Alexandrov A.S.,Theory of Superconductivity - From Weak to Strong Coupling
Подождите немного. Документ загружается.

156
Strong-coupling theory
+
F
7
DUELWUDU\
XQLWV
%(&
%&6
77
F
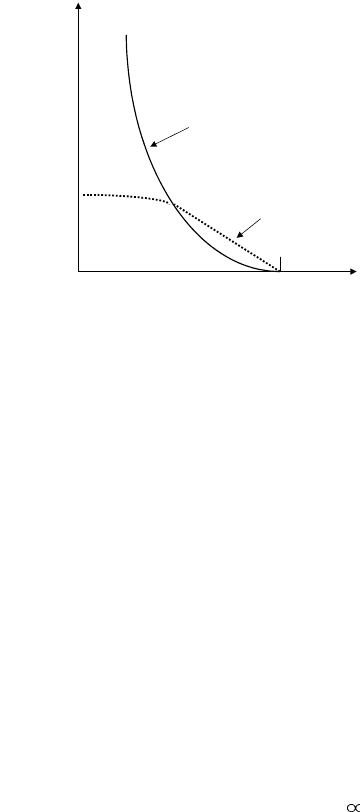
Figure 4.13. BEC critical field of a CBG compared with the H
c2
(T ) of BCS
superconductors.
bipolaron binding energy is much larger than their T
c
. Bosons are condensed
at T = 0 no matter what their energy spectrum is. Hence, in the CBG model,
H
c2
(0) =∞(figure 4.13). For composed bosons, like bipolarons, the pair-
breaking limit is given by µ
B
H
c2
(0) ≈ ,sothatH
c2
(0) H
p
.
4.7.9 Symmetry of the order parameter
The anomalous Bogoliubov average
F
ss
(r
1
, r
2
) =
s
(r
1
)
s
(r
2
)
is the superconducting order parameter both in the weak- and strong-coupling
regimes. It depends on the relative coordinate ρ = r
1
− r
2
of two electrons
of the pair and on the centre-of-mass coordinate R = (r
1
+ r
2
)/2. Hence, its
Fourier transform, f (k, K ), depends on the relative momentum k and on the
centre-of-mass momentum, K. In the BCS theory, K = 0 (in a homogeneous
superconductor) and the Fourier transform of the order parameter is proportional
to the gap in the quasi-particle excitation spectrum, f (k, K )
k
(section 2.2).
Hence, the symmetry of the order parameter and the symmetry of the gap are the
same in the weak-coupling regime. Under the rotation of the coordinate system,
k
changes its sign if the Cooper pairing is unconventional (section 2.10). In this
case, the BCS quasi-particle spectrum is gapless.
In the bipolaron theory, the symmetry of the BEC is not necessarily the
same as the ‘internal’ symmetry of a pair [123]. While the latter describes the
transformation of f (k, K ) with respect to the rotation of k, the former (‘external’)
symmetry is related to the rotation of K . Therefore, it depends on the bipolaron
band dispersion but not on the symmetry of the bound state. As an example, let
us consider a tight-binding bipolaron spectrum comprising two bands on a square
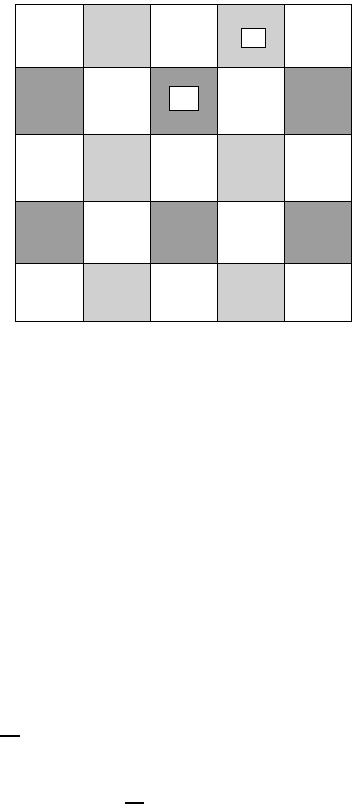
Bipolaronic superconductivity
157
Figure 4.14. d-wave condensate wavefunction, in the Wannier representation. The order
parameter has different signs in the shaded cells and is zero in the blank cells.
lattice with the period a = 1:
E
x
K
= t cos(K
x
) − t
cos(K
y
) (4.269)
E
y
K
=−t
cos(K
x
) + t cos(K
y
).
They transform into one another under π/2 rotation. If t, t
> 0, ‘x’ bipolaron
band has its minima at K = (±π, 0) and the y-band at K = (0, ±π).These
four states are degenerate, so that the condensate wavefunction ψ
s
(m) in the site
space, m = (m
x
, m
y
), is given by
ψ
s
(m) = N
−1/2
K=(±π,0),(0,±π)
b
K
e
−iK ·m
. (4.270)
where b
K
=±
√
n
s
are c-numbers at T = 0. The superposition (4.270) respects
the time-reversal and parity symmetries, if
ψ
±
s
(m) =
√
n
s
[cos(πm
x
) ± cos(πm
y
)]. (4.271)
The two order parameters (equation (4.271)) are physically identical because
they are related by the translation transformation, ψ
+
s
(m
x
, m
y
) = ψ
−
s
(m
x
, m
y
+
1). Both have a d-wave symmetry-changing sign, when the lattice is rotated by
π/2 (figure 4.14). The d-wave symmetry is entirely due to the bipolaron energy
dispersion with four minima at K = 0. When the bipolaron spectrum is not
degenerate and its minimum is located at the point of the Brillouin zone, the
condensate wavefunction is s-wave with respect to the centre-of-mass coordinate.
The symmetry of the gap has little to do with the symmetry of the order parameter
in the strong-coupling regime. The one-particle excitation gap is half of the
158
Strong-coupling theory
bipolaron binding energy, /2, and does not depend on any momentum in zero
order of the polaron bandwidth, i.e. it has an ‘s’-wave symmetry. In fact, due
to a finite dispersion of polaron and bipolaron bands (sections 4.3 and 4.6), the
one-particle gap is an anisotropic s-wave. A multi-band electron structure can
include bands only weakly coupled with phonons which could overlap with the
bipolaronic band (see also part 2). In this case, the CBG coexists with the Fermi
gas just as
4
He bosons coexist with
3
He fermions in the mixture of He-4 and He-3.
The one-particle excitation spectrum of such mixtures is gapless.

PART 2
APPLICATIONS TO HIGH-T
c
SUPERCONDUCTORS

Chapter 5
Competing interactions in unconventional
superconductors
5.1 High-T
c
superconductors: different concepts
Nowadays there are many complex high-T
c
superconductors including copper
[1, 127] and a few other oxides, doped fullerenes M
x
C
60
[128, 129] (M is an
alkali metal) and the more recently discovered magnesium diborade MgB
2
[130]
with a critical temperature above 30 K, figure 5.1.
These discoveries have broken all constraints on the maximum T
c
predicted
by conventional theory of low-temperature superconducting metals and their
alloys. As highlighted by Simon [132], the canonical theory has not provided
a ‘materials’ aspect in the search for new high-T
c
superconductors. Nevertheless,
in a phenomenological sense, any superconductivity can just be treated as a
consequence of the formation of pairs and their condensation—even within the
BCS framework, as was noted by Ginzburg [133] in 1968 (chapter 1). At any
value of the e–ph interaction superconductivity is a correlated many-body state of
pairs which is well described by BCS theory in the weak-coupling regime λ 1,
where pairing takes place in the momentum space due to the Pauli exclusion
principle (collective pairing) and by the bipolaron theory in the strong coupling
regime, λ
1, where pairing is individual (upper half-plane in figure 5.2). Hence,
knowledge of the carrier-pairing mechanism and of the nature of the normal state
is central to an understanding of high-T
c
supeconductivity.
In general, the bosonic field, which ‘glues’ two carriers together, can not only
be ‘phononic’, as in BCS and bipolaron theory, but also ‘excitonic’ [133, 134],
‘plasmonic’ [135, 136] and/or of magnetic origin [137, 138]. BCS theory like
any mean-field theory is rather universal, so that it describes the cooperative
quantum phenomenon of superconductivity well even with these non-phononic
attraction mechanisms, if the coupling is weak (see left-hand side of figure 5.2).
If the coupling is strong, magnetic interaction could result in the spin–bipolaron
formation as suggested by Mott [139]. The main motivation behind these concepts
161
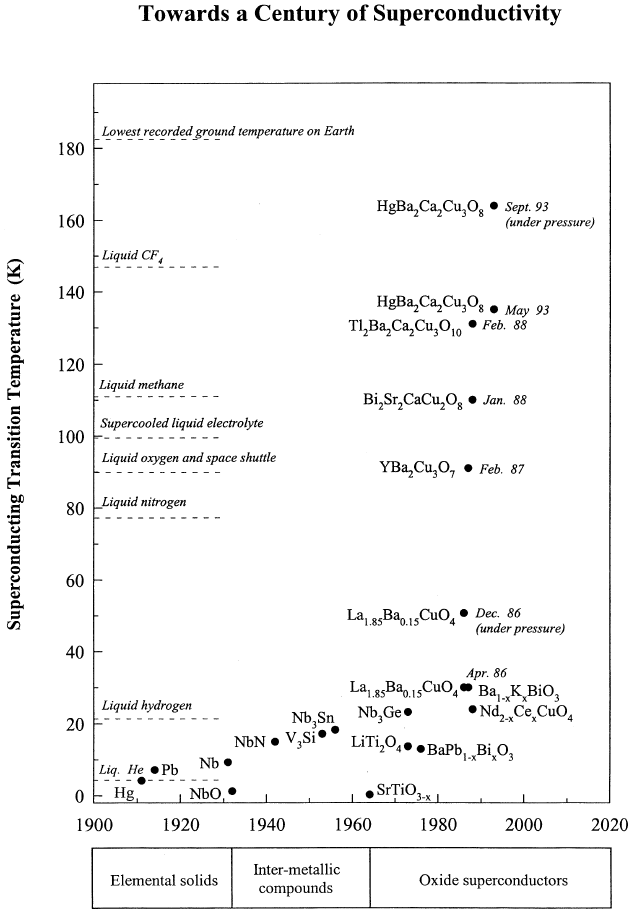
162
Competing interactions in unconventional superconductors
Figure 5.1. Towards a century of superconductivity. A plot of the evolution of the
superconducting transition temperature, from 1911, to the present situation. We also
include the characteristic temperatures of a variety of cryogenic liquids, as well as the
lowest recorded ground temperature on Earth (−89.2
◦
C) [131].
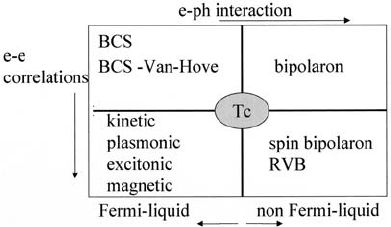
High-
T
c
superconductors: different concepts
163
Figure 5.2. BSC-like theories of high-T
c
superconductors with the electron–phonon (top)
and electron–electron (bottom) interactions (left-hand side). Right-hand side: non-Fermi
liquid theories with electron–phonon (top) and electron–electron (bottom) correlations.
is that high T
c
might be achieved by replacing low-frequency acoustic phonons
in conventional BCS theory by higher-frequency bosonic modes, such as the
electron plasmon (chapter 3) or by spin waves (pseudomagnons). However, the
Coulomb pseudopotential creates a serious problem for any of these non-phononic
mechanisms. With the increasing energy scale of the bosonic field, the retardation
of the effective attraction vanishes and the Tolmachev logarithm (section 3.5)
cannot be applied. Then the direct Coulomb repulsion would definitely prevent
any pairing in these models because the exchange electron–electron interaction is
always weaker than direct repulsion. The problem is particularly grave in novel
superconductors, where the carrier density is low, and the screening of the direct
Coulomb repulsion is poor.
Therefore, some authors [140, 141] dogmatized that the interaction in novel
superconductors is essentially repulsive and unretarded and that it also provides
high T
c
without any phonons. A motivation for this concept can be found
in the earlier work by Kohn and Luttinger [57], who showed that the Cooper
pairing of repulsive fermions is possible. But the same work clearly showed
that the T
c
of repulsive fermions is extremely low, well below the mK scale
(section 3.6). Nevertheless, BCS and BCS-like theories (including the Kohn–
Luttinger consideration) rely heavily on the Fermi-liquid model of the normal
state. This model fails in many high-temperature superconductors (chapter 6).
There are no obvious apriorireasons for discarding the dogma, if the normal
state is not the Fermi liquid. There is little doubt that strong on-site repulsive
correlations (Hubbard U) are an essential feature of the cuprates. Indeed all
undoped parent compounds are insulators with an insulating gap of about 2 eV
or so. But if the repulsive correlations are weak, one would expect a metallic
behaviour for the half-filled d-band of copper in cuprates or, at most, a much
smaller gap caused by lattice and spin distortions (i.e. due to charge and/or spin
density waves [142]). Therefore, it is the strong on-site repulsion of d-electrons
in cuprates which results in their parent ‘Mott’ insulating state (section 5.2).

164
Competing interactions in unconventional superconductors
Differing from conventional band-structure insulators with completely filled or
empty Bloch bands, the Mott insulator arises from a potentially metallic half-filled
band due to the Coulomb blockade of electron tunnelling to neighbouring sites,
if U > zT(a) [143]. The insulator is antiferromagnetic with one hole and spin-
1
2
per site. In using this model, we have to realize that the insulating properties of
the Mott insulator do not depend on the ordering of the spins; they persist above
the N´eel temperature and arise because the on-site Coulomb repulsion is larger
than the half-bandwidth.
When on-site correlations are strong and dimensionality is low, there is an
alternative to the usual Fermi-liquid description proposed by Anderson [140].
In Anderson’s resonating-valence-bond (RVB) theory, the ground state supports
‘topological solitons’ (the so-called spinons and holons), such as occur in one-
dimensional models like the one-dimensional Hubbard model (see later). The
main idea is that an electron injected into a two-dimensional layer decays
into a singlet charge e component (holon) and a spin-
1
2
component (spinon)
and, conversely, must form again in order to come out. This is the case
for one-dimensional repulsive electrons, which form the so-called Luttinger
liquid in one dimension. Bose quasi-particles imply a condensate. However,
there is no experimental evidence for a charge e superfluid. Therefore, in
the so-called interlayer RVB extension of the model [144], it was suggested
that the superconductivity of copper-based high-T
c
materials is due to holon-
pair tunnelling between the copper–oxygen planes. There is no single-particle
coherent tunnelling between two spinon–holon planes above T
c
.However,
there is a coherent two-particle tunnelling between them below T
c
. Then the
corresponding kinetic energy should be responsible for the BCS-like pairing at
temperatures below T
c
≈ t
2
⊥
/t and for the plasma-like gap, observed in the c-
axis conductivity. Here t and t
⊥
are the in-plane and out-of-plane renormalized
hopping integrals, respectively. Anderson argued that the existence of the upper
Hubbard band (section 5.2) would necessarily lead to the Luttinger liquid even in
two dimensions, as opposed to the Fermi liquid. While the interlayer RVB model
was found to be incompatible with experiments [145], the basic idea of spin and
charge separation had been worked out in great detail [146]. The microscopic
Hubbard Hamiltonian [147]
H = T (a)
mn,s
[c
†
ms
c
ns
+ H.c.]+U
m
ˆn
m↑
ˆn
m↓
(5.1)
was proposed to justify the RVB concept, where mn are the nearest neigbours.
The Hamiltonian describes the antiferromagnetic Mott insulator at half filling
when U > D. In the strong correlation limit, U T (a), the doubly occupied
sites take a large Coulomb energy and the Hubbard Hamiltonian can be reduced
to the so-called t–J model [148]
˜
H = T (a)
mn,s
[˜c
†
ms
˜c
ns
+ H.c.]+J
mn
(
ˆ
S
m
ˆ
S
n
−
1
4
ˆn
m
ˆn
n
) (5.2)
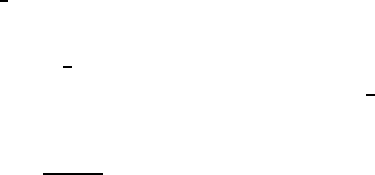
High-
T
c
superconductors: different concepts
165
where the projected electron operators ˜c
m↑
= c
m↑
(1 −ˆn
m↓
) act in the subspace
without double occupancy, ˆn
m
=ˆn
m↑
+ˆn
m↓
,
ˆ
S
α
m
=
1
2
ss
˜c
†
ms
(τ
α
)
ss
˜c
ms
are three components of the on-site spin-
1
2
operator (α = 1, 2, 3) and τ
α
are the
Pauli matrices as in equation (3.73). The second term in
˜
H describes the spin-
1
2
Heisenberg antiferromagnet with the exchange energy
J =
4T
2
(a)
U
(5.3)
for the nearest neigbours. The exchange energy leads to spin polarons similar to
lattice polarons but dressed with magnetic fluctuations rather than with phonons.
However, the non-fermionic commutation relations for the projected electron
operators lead to an additional kinematic interaction between carriers doped into
the Mott insulator. As a result an analytical solution of the t–J model is a very
hard problem but not in the dilute limit. In this limit a doped hole dressed by
antiferromagnetic spin fluctuations propagates coherently in a narrow band of
the order of J T (a) like a small polaron. One could believe that the same
spin fluctuations, which dress a single hole, mediate a superconducting pairing of
spin polarons due to an effective attractive interaction of the order of J .Tobe
consistent with the N´eel temperature of parent undoped cuprates and with their
spin-wave excitation spectrum, J should be of the order of 0.1 eV. The magnetic
singlet pairing might be effective in the d-channel (section 2.10), where the
direct Coulomb repulsion is diminished due to the d-wave symmetry of the BCS
order parameter
k
. The possibility of d-wave BCS-like superconductivity in
the two-dimensional Hubbard model near half-filling was suggested by Scalapino
et al [149] concurrently with the discovery of novel superconductors. There is
now a variety of phase-sensitive experiments, which support the unconventional
symmetry of the order parameter in some cuprates, while other experiments
appear to contradict this symmetry (see section 8.2).
Independent of any experimental evidence, the Hubbard U and t–J models
share an inherent difficulty in determining the order. While some groups
claimed that they describe high-T
c
superconductors at finite doping, other authors
could not find any superconducting instabilty without an additonal (i.e. e–ph)
interaction [150]. Therefore, it has been concluded that models of this kind
are highly conflicting and confuse the issue by exaggerating the magnetism
rather than clarifying it [151]. In our view [12], the problem with the RVB-like
concepts of high-T
c
is that for them to be valid, the diffractive scattering between
holes of cuprate superconductors needs to be absent. A known case where
diffractive scattering is absent is that of one-dimensional interacting fermion
systems. But in one-dimensional interacting fermion systems the scattering is
non-diffractive due to topological reasons. In two and higher dimensions, one
