Alexandrov A.S.,Theory of Superconductivity - From Weak to Strong Coupling

Подождите немного. Документ загружается.

256

Quantum statistics and Boltzmann kinetics

Since cosh

−2

(x /2) falls exponentially for |x| > 1, only a narrow region of the

order of a few T in the vicinity of the Fermi energy contributes to the integral.

Provided that K

(E) is non-singular in the neighbourhood of E = µ, we can

expand it in a Taylor series around E = µ to obtain



∞

−∞

dEN(E)

β(E−µ)

+ 1

∞



p=0

∂

(µ)

dµ

. (B.24)

Here the dimensionless coefﬁcients a

are given by

p−1



∞

−∞

cosh

(x )

. (B.25)

Only terms with even p contribute because a

= 0 for odd p. The coefﬁcients

with p

2 are usually written in terms of Riemann’s zeta function as

=[2 − 2

2−p

]ζ(p) (B.26)

where

ζ(z) =

(1 − 2

1−z

)(z)



∞

z−1

+ 1

(z)



∞

z−1

− 1

(B.27)

and (z) is the gamma function. The zero-order coefﬁcient of the Taylor

expansion is obtained by a straightforward integration:



∞

cosh

(x )

= 1. (B.28)

At low temperatures, we can keep only this, p = 0, and the quadratic terms

with p = 2anda

= π

/6. In particular, for the total energy (r = 1) in any

dimensions, we obtain

U(T ) ≈ 2



dEN(E)E +

[N(µ) + µN



(µ)] (B.29)

where N



(µ) ≡ dN(µ)/dµ and for the particle density (r = 0)

n ≈ 2



dEN(E) +



(µ). (B.30)

The chemical potential differs from the Fermi energy by small terms of the order

of T

, so we write

U(T ) ≈ U(0) + 2E

N(E

)(µ − E

) +

[N(E

) + E



)]

n ≈ n(0) + 2N(E

)(µ − E

) +



) (B.31)

Ideal Fermi gas

257

where U (0) and n(0) are the total energy and the particle density at zero

temperature. To calculate the speciﬁc heat at constant density, we take n to be

temperature independent (n = n(0)) and ﬁnd that

µ = E

−



)/N(E

). (B.32)

Then the total energy becomes

U(T ) ≈ U(0) +

N(E

) (B.33)

and the speciﬁc heat of a degenerate electron gas is, therefore,

(T ) =

2π

TN(E

). (B.34)

The lattice contribution to the speciﬁc heat falls off as the cube of the temperature

and at very low temperatures, it drops below the electronic contribution, which is

linear in T . In practical terms, one can separate these two contributions plotting

C(T )/T against T

. Thus one can ﬁnd γ ≡ 2π

N(E

)/3 and the DOS at the

Fermi level by extrapolating the C(T )/ T curve linearly down to T

= 0and

noting where it intercepts the C(T )/T axis.

B.3.3 Pauli paramagnetism, Landau diamagnetism and de Haas–van

Alphen quantum oscillations

Let us consider a two-dimensional ideal electron gas with the parabolic energy

dispersion E

= (k

)/2m on a lattice in an external magnetic ﬁeld H directed

along z. The energy levels of electrons are quantized (see section 1.6.4) as

= ω(n + 1/2) + µ

σ H (B.35)

where each level is g-fold degenerate with g = L

eH/2π and the last term

takes into account the splitting of the levels due to the spin with σ =±1. Here

ω = eH/m is the Larmour frequency and µ

= e/(2m

) is the Bohr magneton.

The band mass m might be different from the free-electron mass m

. The DOS

becomes a set of narrow peaks:

N(E) = d

∞



n=0

δ[E − ω(n + 1/2) ∓ µ

H ] (B.36)

rather than a constant. This sharp oscillatory structure of the DOS imposed by

the quantization of levels in a magnetic ﬁeld combined with a step-like Fermi–

Dirac distribution at low temperatures leads to the famous de Haas and van

Alphen oscillations of magnetization of two- and three-dimensional metals with

258

Quantum statistics and Boltzmann kinetics

the magnetic ﬁeld. We consider an open system (grand canonical ensemble) with

a ﬁxed chemical potential independent of the applied ﬁeld. It is described by the

thermodynamic potential  = F −µN =−T ln Z. The magnetization of the gas

M(T, H ) =−

∂

∂ H

. (B.37)

For non-interacting fermions, the partition function Z is the product

equation (B.14) and

 =−dT

∞



n=0



σ =±1



1 + exp

µ − ω(n + 1/2) −µ

σ H



. (B.38)

We calculate this sum by applying the Poisson formula for an arbitrary real

function F(n):

∞



n=0

F(n) =



∞

−δ

dxF(x ) + 2

∞



r=1

Re F

(B.39)

where F

is the transform of F(x ),



∞

−δ

dxF(x )e

2πirx

(B.40)

and δ →+0. The Poisson formula is derived by Fourier transforming the sum of

δ-functions

(x ) =

∞



n=−∞

δ(x − n).

This sum is periodic as a function of x with the period 1. Hence, we can expand

it into the Fourier series

(x ) =

∞



r=−∞



2πirx

where the Fourier coefﬁcients are





1/2

−1/2

dx e

−2πirx

(x ) = 1.

Multiplying the Fourier expansion of (x),

∞



n=−∞

δ(x − n) =

∞



r=−∞

2πirx

(B.41)

by F(x ) and integrating it with respect to x from −δ to +∞ we obtain the Poisson

formula (B.39). Applying the formula for F(n) =−gT



σ =±1

ln{1 +exp[(µ −

ω(n + 1/2) −µ

σ H )/T ]} yields

 = 

+ 

(B.42)

Ideal Fermi gas

259

where



=−

2π



σ =±1



∞

dE ln



1 + exp

µ − E − µ

σ H



(B.43)

is the ‘classical’ part containing no orbital effect of the magnetic ﬁeld but only

spin splitting and



=−2dT Re

∞



r=1



σ =±1



∞

dx e

2πirx



1 + exp

µ − ω(x + 1/2) − µ

σ H



(B.44)

is the ‘quantum’ part due to the quantization of the orbital motion. Taking

the derivative of 

, we obtain the Pauli paramagnetic contribution to the

magnetization

= µ

↓

− N

↑

) (B.45)

where

↑↓

2π



∞

exp



E±µ

H−µ



+ 1

is the number of electrons with spin parallel (↑) or antiparallel (↓) to the magnetic

ﬁeld. Performing the integration for T  T

with the Fermi step-function, we

ﬁnd that

= 2µ

HN(E

) (B.46)

where N(E

) = L

m/(2π) is the two-dimensional zero-ﬁeld DOS. The

spin susceptibility χ

= ∂ M

/∂ H is positive and essentially independent of

temperature. It is proportional to the DOS at the Fermi level:

= 2µ

N(E

). (B.47)

This result is actually valid for any energy band spectrum of a metal. Now let

us calculate the quantum part 

. Integrating equation (B.44) twice by parts, we

obtain



= 

+ 

dHvA

(B.48)

where



dω

2π

∞



r=1



σ =±1

1 + exp



ω/2+µ

σ H −µ



(B.49)

and



dHvA

dω

8π

∞



r=1



σ =±1



∞

2πirx

cosh



ω(x+1/2)+µ

σ H −µ



. (B.50)

260

Quantum statistics and Boltzmann kinetics

At all temperatures and ﬁelds of interest, µ  E

 T and µ  ω,sothat



(eH)

2mπ

ζ(2). (B.51)

Here we applied the series representation of the Riemann’s function ζ(2) =



∞

r=1

−2

= π

/6. Differentiating this expression twice with respect to H yields

the diamagnetic (Landau) orbital susceptibility

=−

∂



∂ H

=−





(B.52)

which might be larger or smaller than the paramagnetic Pauli term depending on

the band mass. The remaining part 

dHvA

is responsible for the de Haas–van

Alphen (dHvA) effect. The main contribution to the integral in equation (B.50)

yields the region of x  µ/ω  1, so that we can replace the lower limit in the

integral by −∞. Then using



∞

−∞

iαt

cosh

(t/2)

4πα

sinh(πα)

we obtain



dHvA

∞



r=1

cos



+ πr



(B.53)

where the amplitudes of the Fourier harmonics are

eHT

πr sinh(2π

rT/ω)

cos



πmr



(B.54)

and f = 2πmµ/e is the frequency of oscillations with respect to the inverse

magnetic ﬁeld 1/H . The oscillating part 

dHvA

is small compared with the

‘classical’ part 

as 

dHvA

/

 (ω/µ)

at any temperature. However, due

to the high frequency of oscillations, the oscillating part of magnetization at zero

temperature is larger than the monotonic part as µ/ω,

dHvA

=−

∂

dHvA

∂ H

≈−

∞



r=1

sin



+ πr



. (B.55)

The period of oscillations with the inverse magnetic ﬁeld of the ﬁrst harmonic

(r = 1),

(1/H ) =

2πe

. (B.56)

It directly measures the cross section of the Fermi surface S = πk

,where

= (2mµ)

1/2

is the Fermi momentum. With increasing temperature, the

oscillating part of magnetization falls exponentially as

−2π

rT/ω

. (B.57)

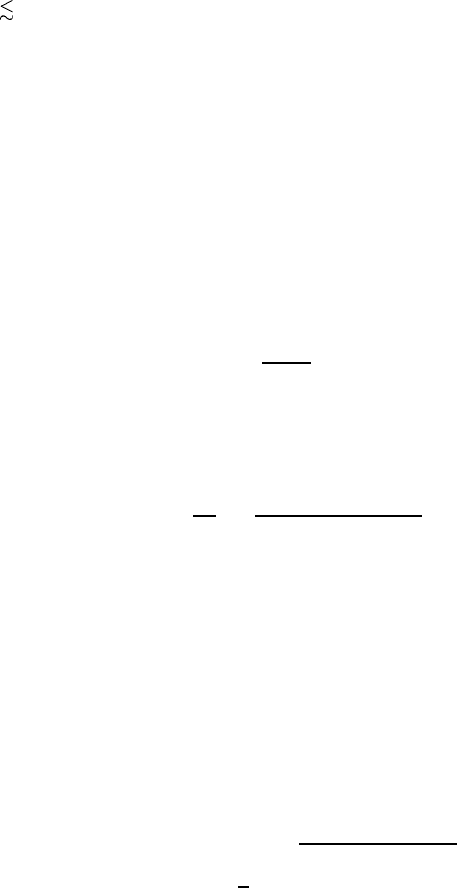
Ideal Bose gas

261

The scattering of electrons by impurities also washes away dHvA oscillations as

soon as the scattering rate is large enough compared with the Larmour frequency,

ωτ

1. The ratio equation (B.52) of the Landau diamagnetism and the Pauli

paramagnetism does not depend on a particular distribution function and remains

the same even for non-degenerate electrons. However, the dHvA effect can be

observed only at low temperatures (T  ω) in clean metals with a well-deﬁned

Fermi surface. The observation of dHvA oscillations is considered to be the

ultimate evidence for a Fermi liquid.

B.4 Ideal Bose gas

B.4.1 Bose–Einstein condensation temperature

Let us consider N

non-interacting Bose particles with zero spin in a cubic box

of the volume L

under periodic boundary conditions. The one-particle energy

spectrum is given by

4π

2mL



j =1

(B.58)

where n

are positive integers including zero and k = (2π/L){n

, n

} is the

wavevector. The chemical potential of a gas µ(T, n) as a function of temperature

and boson density n = N

is determined using the ‘density sum rule’:

n =



exp[β(E

− µ)]−1

(B.59)

where β = 1/T . This equation has a negative solution for µ at any temperature.

If µ is negative, every term of the sum is ﬁnite. When L, N

→∞but n is ﬁnite

(the so-called thermodynamic limit), the one-particle energy spectrum becomes

continuous. In this limit the contribution of every individual term to the sum is

negligible (as 1/N ) compared with n. Hence, we can replace the sum by the

integral using the DOS. However, if the chemical potential approaches zero from

below, a relative contribution of the term with k = 0andE

= 0 could be ﬁnite.

Taking into account this singular contribution we replace the sum by the integral

for all but the ground state. Let us take the number of bosons in the ground state

per unit of volume as n

(T ). Then equation (B.59) is written as

n = n

(T ) +



∞

N(E)

exp[β(E − µ)]−1

(B.60)

where N(E) = m

3/2

1/2

/(π

√

2).Ifthevalueofµ is of the order of level

spacing 1/(mL

) → 0, one can put µ = 0 in this equation. Calculating the

integral, we obtain the density of bosons in the ground state (i.e. the condensate

density),

(T ) = n[1 − (T / T

)

3/2

] (B.61)

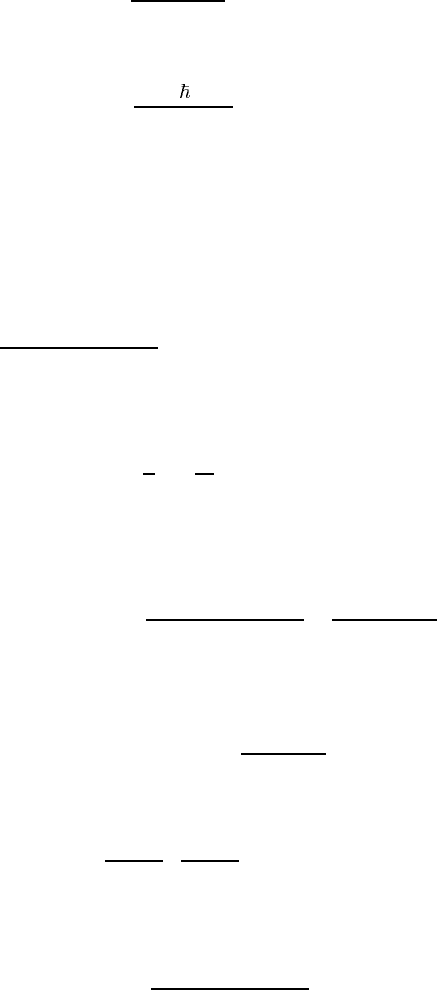
262

Quantum statistics and Boltzmann kinetics

where

= 2π



ζ(3/2)m

3/2



2/3

(B.62)

is the Bose–Einstein condensation temperature. Here ζ(3/2) = 2.612. In

ordinary units, we have

3.313

2/3

. (B.63)

B.4.2 Third-order phase transition

To satisfy equation (B.60), the condensate density n

(T ) should be zero at

temperatures T > T

, where the chemical potential is negative. In this

normal state, the population of every individual level remains microscopic. At

temperatures far above T

, the gas is classical. Here the Bose–Einstein distribution

is almost the same as the Maxwell–Boltzmann distribution,

exp[β(E − µ)]−1

≈ exp[β(µ − E)] (B.64)

if T  T

. The magnitude of the chemical potential is logarithmically large

compared with the temperature in this limit,

µ =−

T ln

. (B.65)

When the temperature approaches T

from above, the chemical potential becomes

small, and ﬁnally zero at and below T

. To obtain its normal state value in the

vicinity of T

, we rewrite the density sum rule as

n[1 −(T / T

)

3/2



∞

dEN(E)



exp[β(E − µ)]−1

−

exp[β E]−1



(B.66)

The function under the integral can be expanded for small E and µ, so that the

integral becomes

n[1 −(T / T

)

3/2

]=T µ



∞

N(E)

E(E − µ)

. (B.67)

The remaining integral yields

µ =−

9π



T − T



(B.68)

for 0 < T − T

 T

. Now we can calculate the free energy and the speciﬁc heat

near the transition. The total energy of the gas per unit of volume is

U =



∞

dEN(E)

exp[β(E − µ)]−1

. (B.69)

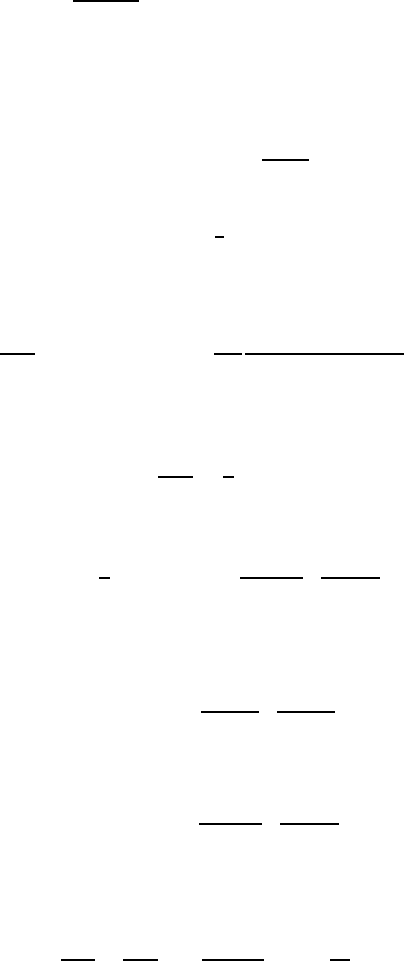
Ideal Bose gas

263

In the condensed state below T

, the chemical potential is zero and

U = U

(T ) =

3ζ(5/2)

2ζ(3/2)

nT(T / T

)

3/2

≈ 0.128m

3/2

5/2

. (B.70)

Remarkably the energy density of the condensed phase does not depend on

the particle density. This is because the macroscopic part of particles in the

condensate does not contribute to the energy. The free energy density

F(T ) =−T



U(T )

(B.71)

is found to be

(T ) =−

(T ). (B.72)

The speciﬁc heat C

(T ) = 5U

(T )/(2T ) is proportional to T

3/2

in the condensed

phase. Differentiating equation (B.69) with respect to the chemical potential, one

obtains

∂U

∂µ

=−



∞

dEN(E)E

∂

∂ E

exp[β(E − µ)]−1

(B.73)

in the normal state. We can take µ = 0 on the right-hand side of this equation

because the chemical potential is small just above T

. Then integrating by parts,

we ﬁnd that

∂U

∂µ

n (B.74)

and

(T ) = U

(T ) +

nµ = U

(T ) −

27π



T − T



. (B.75)

Using equation (B.71), we arrive at the free-energy density in the normal state

close to the transition as

(T ) = F

(T ) +

9π



T − T



(B.76)

and the normal state speciﬁc heat

(T ) = C

(T ) −

27π



T − T



. (B.77)

While the speciﬁc heat of the ideal Bose gas is a continuous function (see

ﬁgure 7.4), its derivative has a jump at T = T



∂C

∂T

−

∂C

∂T



27π

≈ 3.66

. (B.78)

264

Quantum statistics and Boltzmann kinetics

B.5 Boltzmann equation

An external perturbation can drive a macroscopic system out of the thermal

equilibrium. The single-particle distribution function f (r, k, t) in real r and

momentum k space is no longer a Fermi–Dirac or a Bose–Einstein distribution. It

satisﬁes the celebrated Boltzmann kinetic equation. The equation can be derived

by considering a change in the number of particles in an elementary volume dr dk

of the phase space, which is given at the time t by f (r, k, t) dr dk. Particles with

the momentum k move with the group velocity v = dE

/dk. Their number in

the elementary volume dr dk changes due to their motion. The change during an

interval of time dt due to the motion in real space is given by

δ N

=[f (x, y, z, k, t) − f (x + dx , y, z, k, t)]v

dt dy dz dk

+[f (x , y, z, k, t) − f (x , y + dy, z, k, t)]v

dt dx dz dk

+[f (x , y, z, k, t) − f (x , y, z + dz, k, t)]v

dt dx dy dk.

Not only do the coordinates but also the momentum k change under the inﬂuence

of a force F(r, t). The change in the number of particles during the interval dt

due to the ‘motion’ in the momentum space is

δ N

=[f (r, k

, k

, t) − f (r, k

+ dk

, k

, t)]

dt dk

+[f (r, k

, k

, t) − f (r, k

, k

+ dk

, k

, t)]

dt dk

+[f (r, k

, k

, t) − f (r, k

, k

+ dk

, t)]

dt dk

where

k = F(r, t). As a result, the change in the number of particles in the

elementary volume of the phase space is

δ N = δ N

+ δN

. (B.79)

This change can also be expressed via a time derivative of the distribution function

as δN = (∂ f /∂t) dt dr dk, which ﬁnally yields

∂ f (r, k, t)

∂t

+ v · ∇

f (r, k, t) + F(r, t) · ∇

f (r, k, t) = 0. (B.80)

The force acting upon a particle is the sum of the external force and the internal

force of other particles of the system. Splitting the last term in equation (B.80)

into the external and internal contributions, we obtain equation (1.4), where the

collision integral describes the effect of the internal forces.

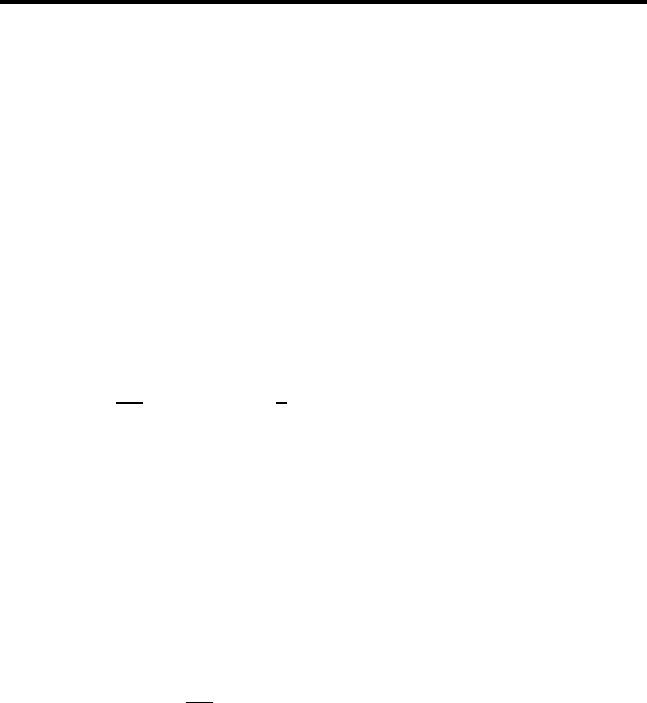
Appendix C

Second quantization

C.1 Slater determinant

Let us consider a quantum mechanical system of N identical particles with

amassm,aspins and the interaction potential between any two of them

V (r

− r

). There is also an external ﬁeld V (r).Herer

are three position

coordinates {x

, y

, z

}, i, j = 1, 2, 3,...,N. The system is described by

a many-particle wavefunction (q

, q

,...,q

), which satisﬁes the stationary

Schr¨odinger equation:





i=1



−



+ V (r

)





i=1



j =i

V (r

− r

)



(q

, q

,...,q

)

= E(q

, q

,...,q

) (C.1)

where q

≡ (r

,σ

) is a set of position r

and spin σ

coordinates. The spin

coordinate (z-component of spin s

) describes the ‘internal’ state of the particle,

which might be a composite particle like an atom. We can readily solve this

equation for non-interacting particles when V (r

− r

) = 0. In this case, many-

particle eigenstates are products of one-particle wavefunctions,



, q

,...q

) = u

) ×···×u

) (C.2)

where u

(q) is a solution of a one-particle Schr¨odinger equation



−

 + V (r)



(r,σ)= 

(r,σ) (C.3)

k is a set of one-particle quantum numbers, for example the wavevector k and s

if the particles are free, or of n, k and s

, if V (r) is periodic (appendix A). Direct

substitution of equation (C.2) into equation (C.1) yields the total energy of the

system, which is the sum of one-particle energies,



i=1



. (C.4)

265