Alexandrov A.S.,Theory of Superconductivity - From Weak to Strong Coupling
Подождите немного. Документ загружается.

66
Weak coupling theory
Then using equation (2.194), we obtain
G(k,ω) = P
u
2
k
ω −
k
+
v
2
k
ω +
k
−iπ tanh
ω
2T
[u
2
k
δ(ω−
k
) +v
2
k
δ(ω+
k
)]
(2.199)
where we have applied the relation 1/(ω +iδ) = P(1/ω) −iπδ(ω).Herethefirst
term is understood as the principal value of the integral when integrating with
respect to ω. For zero temperature, tanh(ω/2T ) = sign(ω) and
G(k,ω) =
u
2
k
ω −
k
+ iδ
+
v
2
k
ω +
k
− iδ
(2.200)
as it should (see equation (2.165)).
2.16 Microscopic derivation of the Ginzburg–Landau
equations
Using the Green’s function formalism near T
c
, Gor’kov [45] derived the
Ginzburg–Landau equations from BCS theory. Let us consider the BCS
superconductor in a stationary magnetic field with the vector potential A(r).
The Fourier transform of equations (2.183) and (2.184) with respect to the
thermodynamic time yields
iω
n
+
[∇ + ie A(r)]
2
2m
+ µ
ω
n
(r, r
) = δ(r − r
) + (r)
+
ω
n
(r, r
),
−iω
n
+
[∇ − ie A(r)]
2
2m
+ µ
+
ω
n
(r, r
) =−
∗
(r)
ω
n
(r, r
) (2.201)
where
ω
n
(r, r
) =
1/T
0
dτ (r, r
,τ)exp(iπ nTτ)
+
ω
n
(r, r
) =
1/T
0
dτ
+
(r, r
,τ)exp(iπnTτ)
and
∗
(r) =−2E
p
T
ω
n
+
ω
n
(r, r).
If the temperature is close to T
c
, the order parameter is small. Then we can
expand the GFs in powers of (r). The zero-order GF is a temperature GF of
an ideal Fermi gas,
(n)
ω
n
(r, r
) in the magnetic field, which satisfies the following
equation:
iω
n
+
[∇ + ie A(r)]
2
2m
+ µ
(n)
ω
n
(r, r
) = δ(r − r
). (2.202)
Microscopic derivation of the Ginzburg–Landau equations
67
If there is no magnetic field,
(n)
ω
n
(r, r
) =
(0)
ω
n
(r − r
) and its Fourier transform
is found as
(0)
(k,ω
n
) =
1
iω
n
− ξ
k
. (2.203)
Transforming back to real space we obtain
(0)
ω
n
(ρ) =
1
(2π)
3
dk
e
ik·ρ
iω
n
− ξ
k
(2.204)
where ρ = r − r
. Calculating the integral over the angles in the polar spherical
coordinates yields
(0)
ω
n
(ρ) =
1
(2π)
2
iρ
∞
0
k dk
e
ikρ
− e
−ikρ
iω
n
− ξ
. (2.205)
We can replace k by k = k
F
+ξ/v
F
because only the states near the Fermi surface
contribute to the integral in equation (2.205), when ω
n
is of the order of T
c
E
F
.
The result is
(0)
ω
n
(ρ) =
m
(2π)
2
iρ
∞
−∞
dξ
e
ik
F
ρ
e
iξρ/v
F
− e
−ikρ
e
−iξρ/v
F
iω
n
− ξ
. (2.206)
Using the contour in the upper or lower half-plane, we obtain
(0)
ω
n
(ρ) =−
m
2πρ
exp[isign(ω
n
)k
F
ρ −|ω
n
|ρ/v
F
]. (2.207)
The Fourier component of the normal state GF falls exponentially with the
characteristic length
ρ ≈ ξ
0
(2.208)
where ξ
0
= v
F
/(2π T
c
) is the zero temperature coherence length. GFs in a
magnetic field are not translation invariant and the exact calculation of the normal
(n)
ω
n
(r, r
) using equation (2.202) is a challenging problem. But we can assume
that the spatial variations of the vector potential are small over the characteristic
distance ξ
0
. If we neglect these variations, then the field operator in the magnetic
field can be readily expressed via the free-electron operator. It satisfies the
equation of motion
∂ψ
s
(r,τ)
∂τ
=
[∇ + ie A(r)]
2
2m
+ µ
ψ
s
(r,τ). (2.209)
If A(r) is a constant then the solution is
ψ
s
(r,τ) =
k
c
ks
exp[i(k − e A) · r − ξ
k
τ)] (2.210)
68
Weak coupling theory
which is verified by direct substitution into equation (2.209). Hence, if the vector
potential varies slowly, the GF differs from the zero-field GF only by the phase:
(n)
ω
n
(r, r
) = e
−ie A(r)·ρ (0)
ω
n
(ρ). (2.211)
This quasi-classical approximation with respect to the magnetic field is applied
if the phase change over the distance ξ
0
is small. |A(r)| varies by Bξ
0
over
this distance, and the phase changes by eBξ
2
0
. The maximum field is (see
section 1.6.4)
B
H
c2
≈
0
2πξ
2
0
T
c
− T
T
c
(2.212)
so that the quasi-classical approximation is applied, if
T
c
− T
T
c
1. (2.213)
It is the very same region where the Ginzburg–Landau theory is applied. Using
the normal state GF in the magnetic field (equation (2.211)), we can transform
Gor’kov equations (2.201) into the integral form:
ω
n
(r, r
) =
(n)
ω
n
(r, r
) +
dx
(n)
ω
n
(r, x)(x)
+
ω
n
(x, r
) (2.214)
+
ω
n
(r, r
) =−
dx
(n)
−ω
n
(x, r)
∗
(x)
ω
n
(x, r
).
Now we expand
ω
n
(r, r
) to the terms of the second order and
ω
n
(r, r
) to the
terms of the third order in (r) inclusive:
ω
n
(r, r
) =
(n)
ω
n
(r, r
) −
dx
d y
(n)
ω
n
(r, x)(x)
×
(n)
−ω
n
( y, x)
∗
( y)
(n)
ω
n
( y, r
) (2.215)
+
ω
n
(r, r
) =−
dx
(n)
−ω
n
(x, r)
∗
(x)
(n)
ω
n
(x, r
)
+
dx
d y
dz
(n)
−ω
n
(x, r)
∗
(x)
(n)
ω
n
(x, y)( y)
×
(n)
−ω
n
(z, y)
∗
(z)
(n)
ω
n
(z, r
).
Using this expansion, we obtain the following equation for the order parameter:
∗
(r) = 2E
p
T
ω
n
dx
(n)
−ω
n
(x, r)
∗
(x)
(n)
ω
n
(x, r)
− 2E
p
T
ω
n
dx
d y
dz
(n)
−ω
n
(x, r)
∗
(x)
(n)
ω
n
(x, y)( y)
×
(n)
−ω
n
(z, y)
∗
(z)
(n)
ω
n
(z, r). (2.216)
Microscopic derivation of the Ginzburg–Landau equations
69
Let us consider the kernel in the first term
K (x − r) = T
ω
n
(n)
−ω
n
(x, r)
(n)
ω
n
(x, r) = K
(0)
(x − r)e
−2ie A(r)·(x−r)
(2.217)
where
K
(0)
(x − r) = T
m
2
(2πρ)
2
ω
n
exp[−2|ω
n
|ρ/v
F
]
=
m
2
T
(2πρ)
2
sinh(2π Tρ/v
F
)
(2.218)
and ρ =|x − r|.ThekernelK
(0)
(ρ) has a characteristic radius about ξ
0
, while
(x) changes over a much larger distance of the order of ξ(T ) ξ
0
near T
c
.
Therefore in the integral over x we can expand all quantities near the point x = r
up to the second order in ρ inclusive:
dx K
(0)
(x − r)e
−2ie A(r)·(x−r)
∗
(x)
≈
∗
(r)
dρ K
(0)
(ρ) +
dρ K
(0)
(ρ)ρ ·[∇
r
− 2ie A(r)]
∗
(r)
+
1
2
3
i, j=1
dρ K
(0)
(ρ)ρ
i
ρ
j
∂
∂r
i
− 2ieA
i
∂
∂r
j
− 2ieA
j
∗
(r).
(2.219)
Here the second term is zero because the function under the integral is odd. The
remaining integrals are:
dρ K
(0)
(ρ) = T
ω
n
dρ
(0)
−ω
n
(ρ)
(0)
ω
n
(ρ)
= T
k
ω
n
1
ω
2
n
+ ξ
2
k
= N(E
F
)
ω
D
0
dξ
tanh(ξ/2T )
ξ
(2.220)
and
dρ K
(0)
(ρ)ρ
i
ρ
j
=
δ
ij
3
dρ K
(0)
(ρ)ρ
2
=
δ
ij
3
v
2
F
4(π T )
2
N(E
F
)
∞
0
dx
x
2
sinh x
=
δ
ij
3
7ζ(3)v
2
F
8(π T )
2
N(E
F
).
(2.221)
We cut the divergent integral in equation (2.220) at |ξ |=ω
D
as usual and
introduce the DOS at the Fermi level: N (E
F
) = mk
F
/(2π
2
). The second term
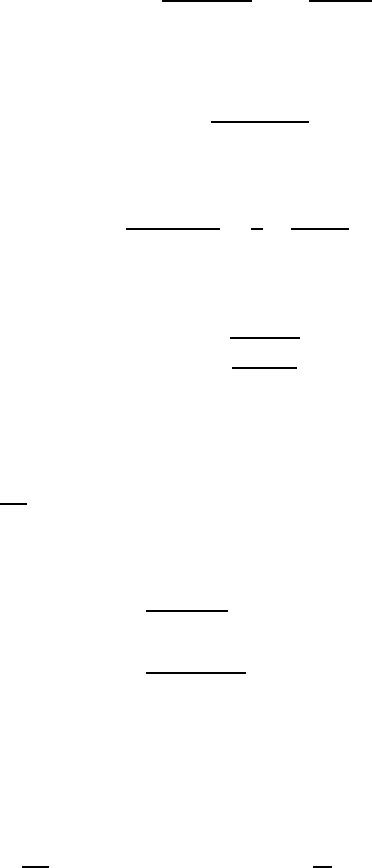
70
Weak coupling theory
on the right-hand side of equation (2.216) is cubic in (r). Here we can neglect
the space variations of (x) and the phase due to the vector potential under the
integrals. Then applying the Fourier transform of the zero-field ideal gas GF, we
obtain the following expression for this term:
−2E
p
T
∗
(r)|(r)|
2
k
ω
n
1
(ω
2
n
+ ξ
2
k
)
2
=−λ
7ζ(3)
8(π T )
2
∗
(r)|(r)|
2
.
(2.222)
We note that T
c
is determined from the linearized BCS equation:
1 = λ
ω
D
0
dξ
tanh(ξ/2T
c
)
ξ
.
Then close to T
c
, we can expand it as
ω
D
0
dξ
tanh(ξ/2T )
ξ
≈
1
λ
+
T
c
− T
T
c
(2.223)
where we use the integral, equation (B.28) from the appendix. Introducing the
‘condensate wavefunction’ φ(r) as
φ(r) = (r)
7ζ(3)n
e
8π
2
T
2
c
(2.224)
and collecting all integrals in equation (2.216), we obtain the phenomenological
Ginzburg–Landau equation (section 1.6.1):
−
1
4m
[∇ +i2e A(r)]
2
φ(r) + β|φ(r)|
2
φ(r) =−αφ(r).
Now the coefficients α and β are determined microscopically as
α =
12π
2
T
c
7ζ(3)mv
2
F
(T − T
c
) (2.225)
β =
12π
2
T
2
c
7ζ(3)mv
2
F
n
e
.
Here n
e
= k
3
F
/(3π
2
) is the electron density. Finally let us show that the second GL
equation for supercurrent follows from the expansion of GFs (2.215) in powers
of (r). The current–density operator can be expressed in terms of the field
operators:
ˆ (r) =
s
ie
2m
(∇
r
−∇
r
)|
r
→r
†
s
(r
)
s
(r) −
e
2
m
A(r)
†
s
(r)
s
(r)
.
(2.226)
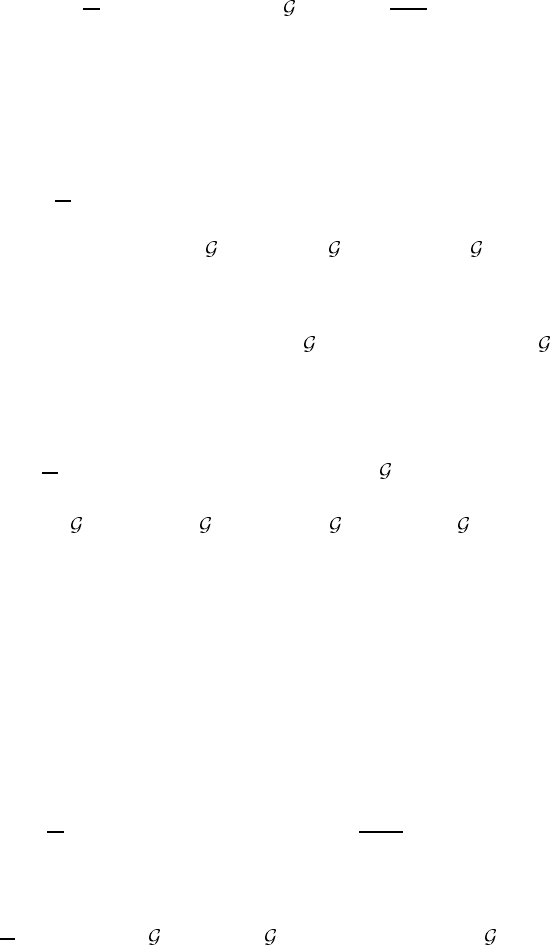
Microscopic derivation of the Ginzburg–Landau equations
71
Its expectation value is
j(r) =
ie
m
(∇
r
−∇
r
)|
r
→r
T
ω
n
ω
n
(r, r
) −
e
2
n
e
m
A(r). (2.227)
There is no current in the normal state in the stationary magnetic field, and the
first (normal) term in the expansion of GFs (equation (2.215)) cancels the second
term on the right-hand side of equation (2.227). The remaining supercurrent is
quadratic with respect to the order parameter:
j(r) =
ie
m
(∇
r
−∇
r
)|
r
→r
T
×
ω
n
dx
d y
(n)
ω
n
(r, x)(x)
(n)
−ω
n
( y, x)
∗
( y)
(n)
ω
n
( y, r
).
(2.228)
The normal state GF in the magnetic field
(n)
ω
n
(r, r
) = exp(−ie A · ρ)
(0)
ω
n
(ρ)
is the product of a slowly varying phase exponent and the zero-field GF, which
oscillates in real space with the electron wavelength (≈1/k
F
). Differentiating the
oscillating part yields
j(r) =
ie
m
T
ω
n
dx
d y (x)
∗
( y)e
2iA·(x−y)
(0)
−ω
n
(|y − x|)
×[
(0)
ω
n
(|r − x|)∇
r
(0)
ω
n
(|y − r|) −
(0)
ω
n
(|y − r|)∇
r
(0)
ω
n
(|r − x|)].
(2.229)
Expanding the slowly varying order parameter and the magnetic phase exponent
in powers of |y − r| and |x − r|, which are of the order of ξ
0
,
(x) ≈ (r) + (x − r) · ∇(r)
∗
( y) ≈ (r) + ( y − r) · ∇
∗
(r)
exp[2ie A ·(x − y)]≈1 + 2ie A · (x − y)
we obtain
j(r) =
ie
m
C[
∗
(r)∇(r) − (r)∇
∗
(r)]−
4e
2
C
m
|(r)|
2
A(r). (2.230)
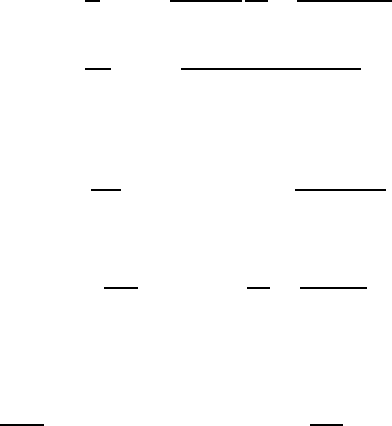
Here the constant C is given by
C =
T
3
ω
n
dx
d y
(0)
−ω
n
(|y − x|)
(0)
ω
n
(|r − x|)[(x − y) ·∇
r
]
(0)
ω
n
(|y − r|).
(2.231)
72
Weak coupling theory
Using the Fourier transform of the ideal gas GF in calculating the integrals over
x and y in C leads to
C =
T
3
ω
n
k
1
iω
n
+ ξ
k
∂
∂ k
· k
1
(iω
n
− ξ
k
)
2
=
T
3m
ω
n
k
k
2
(iω
n
+ ξ
k
)(iω
n
− ξ
k
)
3
. (2.232)
Integrating with respect to k yields
C =
2k
2
F
3m
N(E
F
)T
ω
n
∞
−∞
dξ
ω
2
n
− ξ
2
(ω
2
n
+ ξ
2
)
3
(2.233)
and we obtain
C =
k
2
F
π
6m
N(E
F
)T
ω
n
1
ω
3
n
=
7ζ(3)n
e
16π
2
T
2
c
.
Replacing the order parameter in equation (2.230) by the ‘condensate
wavefunction’ leads to the second GL equation (see equation (1.40)):
j(r) =
ie
∗
2m
∗∗
[φ
∗
(r)∇φ(r) − φ(r)∇φ
∗
(r)]−
e
∗2
m
∗∗
A(r)|φ(r)|
2
(2.234)
where e
∗
= 2e and m
∗∗
= 2m are the charge and mass of the Cooper pair,
respectively.
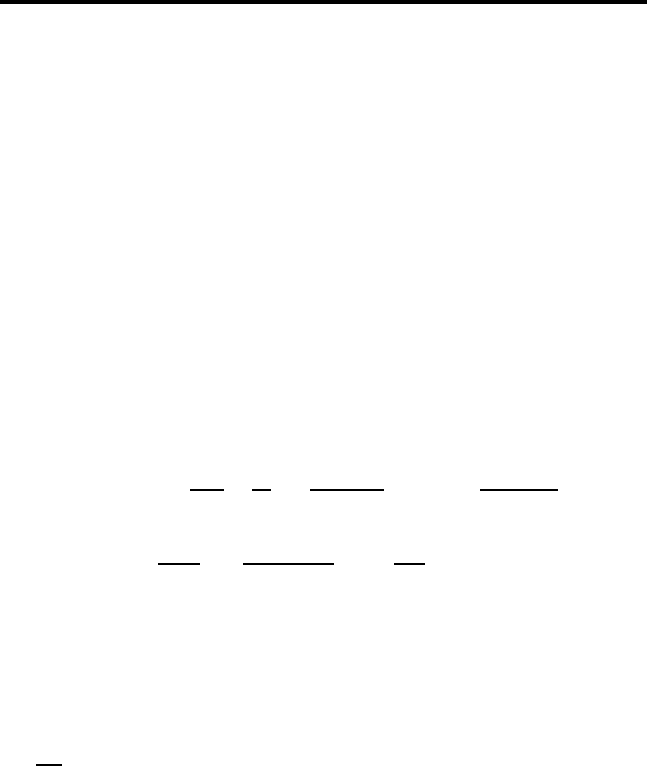
The BCS theory is a mean-field approximation, which is valid if the volume
occupied by the correlated electron pair is large compared with the volume per
electron. As an example, in aluminium the size of the pair (i.e. the coherence
length at T = 0) is about ten thousand times larger than the distance between
electrons. The Cooper pairs in the BCS superconductor disappear above T
c
and the one-particle excitations are fermions. When the coupling constant λ
increases, the critical temperature increases and the coherence length becomes
smaller. Hence, one can expect some deviations from the BCS behaviour in the
intermediate coupling regime. At the first stage, deviations from BCS theory arise
in two ways: (1) the BCS approximation for the interaction between electrons
does not provide an adequate representation of the retarded nature of the phonon
induced attraction; and (2) the damping rate becomes comparable with the quasi-
particle energy. Both the retardation effect and the damping are taken into account
in the Eliashberg extension of the BCS theory to the intermediate coupling regime
[36], which is discussed in the next chapter. However, if λ is larger than 1, the
Fermi liquid becomes unstable even in the normal state above T
c
because of the
polaron collapse of the electron band (chapter 4). Here we encounter qualitatively
different physics [11].
Chapter 3
Intermediate-coupling theory
3.1 Electron–phonon interaction
The attraction between electrons in BCS theory is the result of an ‘overscreening’
of their Coulomb repulsion by vibrating ions. When the interaction between
ion vibrations and electrons (i.e. the electron–phonon interaction) is strong, the
electron Bloch states are affected even in the normal phase. Phonons are also
affected by conduction electrons. In doped insulators (like high-temperature
superconductors), ‘bare’ phonons are well defined in insulating parent compounds
but a separation of electron and phonon degrees of freedom might be a problem
in a metal. Here we have to start with the first-principle Hamiltonian describing
conduction electrons and ions coupled by the Coulomb forces:
H =−
i
∇
2
i
2m
e
+
e
2
2
i=i
1
|r
i
− r
i
|
− Ze
2
ij
1
|r
i
− R
j
|
+
Z
2
e
2
2
j =j
1
|R
j
− R
j
|
−
j
∇
2
j
2M
(3.1)
where r
i
, R
j
are the electron and ion coordinates, respectively, i = 1,...,N
e
;
j = 1,...,N; ∇
i
= ∂/∂r
i
, ∇
j
= ∂/∂ R
j
, Ze is the ion charge and M
is the ion mass. The system is neutral and N
e
= ZN. The inner electrons
are strongly coupled to the nuclei and follow their motion. Hence, the ions
can be considered as rigid charges. To account for their high-energy electron
degrees of freedom we can replace the elementary charge in equation (3.1) by
e/
√
∞
,where
∞
is the phenomenological high-frequency dielectric constant.
One cannot solve the corresponding Schr¨odinger equation perturbatively because
the Coulomb interaction is strong. The ratio of the characteristic Coulomb energy
to the kinetic energy is r
s
= m
e
e
2
/(4πn
e
/3)
1/3
≈ 1 for the electron density
n
e
= ZN/V = 10
23
cm
−3
(further we take the volume of the system as V = 1,
unless specified otherwise). However, we can take advantage of the small value
73
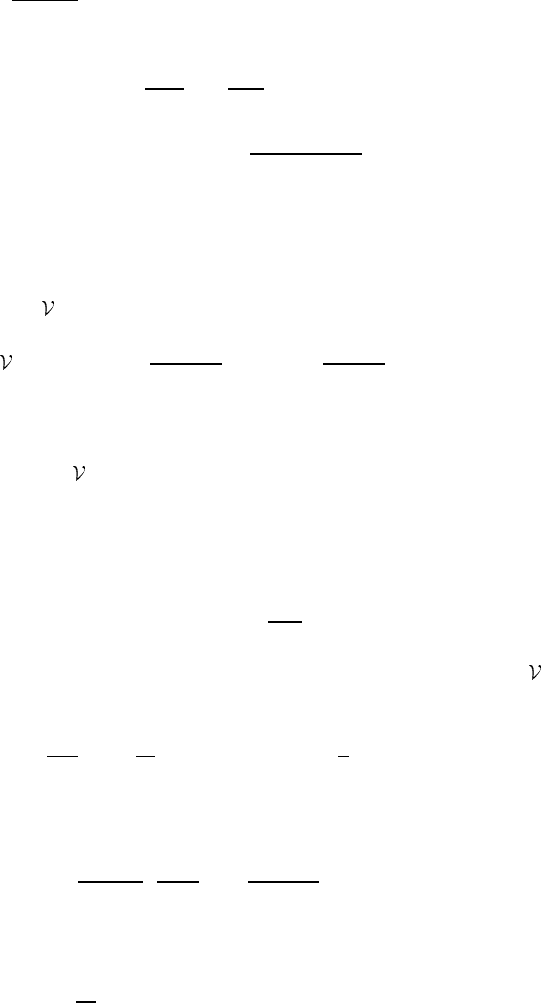
74
Intermediate-coupling theory
of the adiabatic ratio m
e
/M < 10
−3
. The ions are heavy and the amplitudes
|u|
√
1/Mω
D
of their vibrations near the equilibrium R
0
≡ l are much
smaller than the lattice constant a = N
−1/3
:
|u|
a
≈
m
e
Mr
s
1/4
1. (3.2)
In this estimate we take the characteristic vibration frequency ω
D
of the order
of the ion plasma frequency ω
i
=
4π NZ
2
e
2
/M. Because the vibration
amplitudes are small we can expand the Hamiltonian in powers of |u| up to
quadratic terms inclusive. Any further progress requires a simplifying physical
idea, which is to approach the ground state of the many-electron system via
a one-electron picture. This is called the local density approximation (LDA),
which replaces the Coulomb electron–electron interaction by an effective one-
body potential
(r):
(r) =−Ze
2
j
1
|r − R
j
|
+ e
2
dr
n(r
)
|r − r
|
+ µ
ex
[n(r)] (3.3)
where µ
ex
[n(r)] is the exchange interaction, usually calculated numerically
or expressed as µ
ex
[n(r)]=−βn
1/3
(r) with the constant β in a simple
approximation.
(r) is the functional of the electron density n(r) =
s
†
s
(r)
s
(r). As a result, the Hamiltonian takes the form in the second
quantization:
H = H
e
+ H
ph
+ H
e−ph
+ H
e−e
(3.4)
where
H
e
=
s
dr
†
s
(r)
−
∇
2
2m
e
+ V (r)
s
(r) (3.5)
is the electron energy in a periodic crystal field V (r) =
l
v(r −l) which is (r)
calculated at R
j
= l and with the periodic electron density n
(0)
(r +l) = n
(0)
(r),
H
ph
=
l
−
∇
2
u
2M
+u
l
·
∂
∂l
dr n
(0)
(r)V (r)
+
1
2
l,m,α,β
u
lα
u
mβ
D
αβ
(l −m)
(3.6)
is the vibration energy. Here α, β = x, y, z and
D
αβ
(l − m) =
∂
2
∂l
α
∂m
β
Z
2
e
2
2
l
=m
1
|l
− m
|
+
dr n
(0)
(r)V (r)
(3.7)
is a dynamic matrix. The electron–phonon interaction is given by
H
e−ph
=
l
u
l
·
∂
∂l
dr
s
†
s
(r)
s
(r) − n
(0)
(r)
V (r)
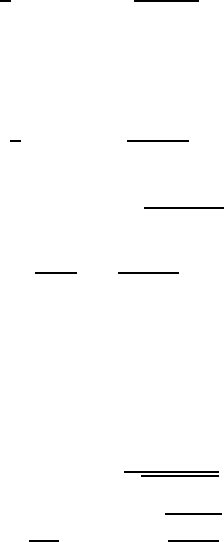
Electron–phonon interaction
75
+
1
2
l,m,α,β
u
lα
u
mβ
∂
2
∂l
α
∂m
β
dr
s
†
s
(r)
s
(r) − n
(0)
(r)
V (r)
(3.8)
and the electron–electron correlations are described by
H
e−e
=
1
2
dr
dr
e
2
|r − r
|
ss
†
s
(r)
†
s
(r
)
s
(r
)
s
(r)
−
dr
dr
e
2
n
(0)
(r
)
|r − r
|
+µ
ex
[n
(0)
(r)]
s
†
s
(r)
s
(r)
+
Z
2
e
2
2
l=m
1
|l − m|
. (3.9)
We include the electrostatic repulsive energy of nuclei in H
e−e
, so that the average
of H
e−e
is zero in the Hartree approximation.
The vibration Hamiltonian H
ph
is a quadratic form and, therefore, can
be diagonalized with a linear canonical transformation for the displacement
operators
u
l
=
q,ν
e
qν
2NMω
qν
d
qν
exp(iq · l) + H.c. (3.10)
∂
∂u
l
=
q,ν
e
qν
Mω
qν
2N
d
qν
exp(iq · l) − H.c.
where q is the phonon momentum, d
qν
is the phonon (Bose) annihilation
operator, e
qν
and ω
qν
are the unit polarization vector and the phonon frequency,
respectively, of the phonon mode ν.ThenH
ph
takes the following form
H
ph
=
q,ν
ω
qν
(d
†
qν
d
qν
+ 1/2) (3.11)
if the eigenfrequencies ω
qν
and the eigenstates e
qν
satisfy
Mω
2
qν
e
α
qν
=
β
D
αβ
q
e
β
qν
(3.12)
and
q
e
∗α
qν
e
β
qν
= Nδ
αβ
. (3.13)
The last equation and the bosonic commutation rules [d
qν
d
†
q
ν
]=δ
νν
δ
qq
follow
from (∂/∂u
α
l
)u
β
l
− u
β
l
(∂/∂u
α
l
) = δ
αβ
.Here
D
αβ
q
=
m
exp(iq · m)D
αβ
(m) (3.14)
