Astakhov V. Tribology of Metal Cutting

Подождите немного. Документ загружается.

Design of Experiments in Metal Cutting Tests 297

Therefore, the design matrix normal system is a diagonal system. Another important

consequence of this property is the mutual independence of the estimated value of the

regression coefﬁcients that signiﬁcantly simpliﬁes their calculations.

The model considered is linear with respect to the response but non-linear with respect

to the factors. The non-linearity in such a context means that the interactions of the

factors are taken into consideration so that they can be estimated quantitatively. Only

when b

=0 and b

123

=0, then the resultant model is linear with respect to both the

factors and interactions.

If a model is non-linear then one should realize that its non-linearity shows up in the

dependence of a factor on the level of other factor, i.e. interaction of these two factors

takes place. The complete block DOE allows quantitative evaluation of such interactions.

The complete block 2

DOE allows to obtain evaluations for eight regression coefﬁcients

, b

and b

123

. However, if one tries to obtain the regression

coefﬁcients of the squared factors (b

, b

, etc.) of regression then one ﬁnds that it is not

possible because columns x

, x

and x

coincide with each other and with x

. Because

these columns become indistinguishable, it is impossible to say what b

estimates in

this case.

5.4.3 Example 1: experimental study of the roughness and roundness of the drilled holes

Obtaining the mathematical model. Consider the use of DOE in the experimental study

of the inﬂuence of three parameters: cutting speed ν(x

), feed f (x

) and the cutting ﬂuid

ﬂow rate Q(x

) on the roughness ∆(y

) and roundness ρ(y

) of the machined hole in

gundrilling. A 2

DOE, complete block is used. According to Eq. (5.18), the mathematical

model for this case can be written as follows:



y =b

123

(5.21)

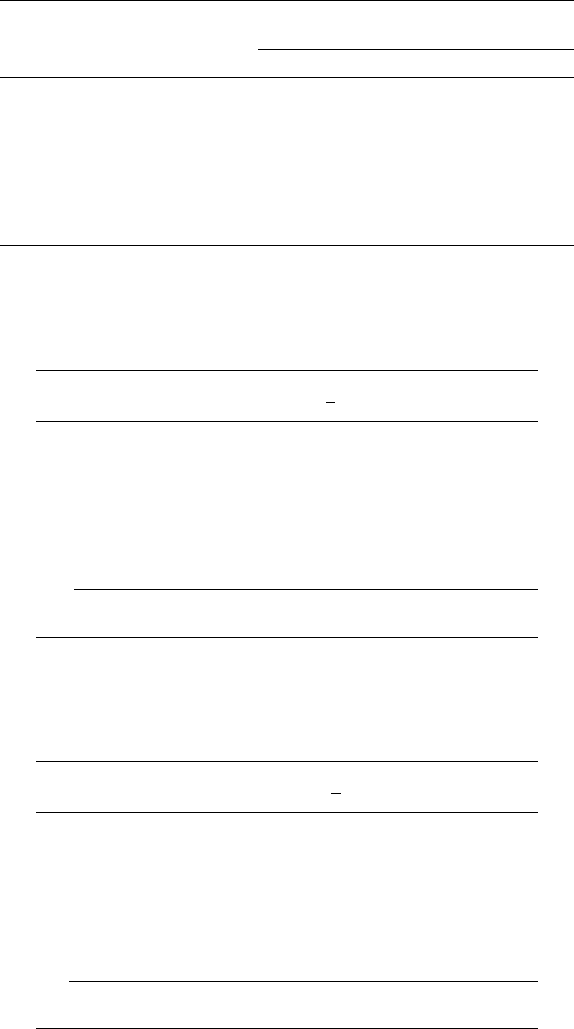
The levels of the factors and intervals of factor variations are shown in Table 5.8.

At each point of the design matrix (Table 5.7), the tests were replicated three times

(r =3). The sequence of the tests was arranged using a generator of random numbers.

The experimental results for roughness and for roundness are shown in Tables 5.9 and

5.10, respectively.

The orthogonality of the design matrix simpliﬁes the calculation of the regression

coefﬁcients, which can be calculated as

u=1

, (5.22)

where i =1, 2, ...,k is the number of factor, m is the number of points of the design

matrix m =8 and

is the mean response at point u of the design matrix (averaged

298 Tribology of Metal Cutting

Table 5.8. The levels of factors and their intervals of variation (roughness

and roundness tests).

Levels of factors Notation ν(m/min) f (mm/rev) Q(l/min)

(n(rpm)) (f

(mm/min)) (G(min))

Basic 0 100 0.07 60

(1670.92) (116.96) (16)

Interval of variation ∆x

15 0.02 20

(250.64) (101.93) (5.33)

Upper +1 115 0.09 80

(1921.56) (172.94) (21.33)

Lower −1 85 0.05 40

(1420.28) (71.01) (10.67)

Table 5.9. Test results (roughness of machined holes (µm)).

Average response Row variance

1 0.57 0.44 1.00 0.67 0.0859

2 0.44 0.38 0.42 0.41 0.0009

3 0.34 0.21 0.15 0.23 0.0095

4 0.22 0.49 0.22 0.31 0.0243

5 0.93 0.49 0.20 0.54 0.1351

6 0.22 0.47 0.21 0.30 0.0217

7 0.17 0.24 0.17 0.19 0.0017

8 0.33 0.21 0.32 0.29 0.0044

{

}

u=1

/8=0.03543

Table 5.10. Test results (roundness of machined holes (µm)).

Average response Row variance

1 4.000 2.900 5.300 4.067 1.508

2 4.000 2.300 2.600 2.967 0.832

3 4.500 4.900 6.000 5.133 0.603

4 3.000 1.200 4.200 2.800 4.560

5 5.500 4.000 5.200 4.900 0.603

6 3.200 3.500 3.000 3.233 0.063

7 3.500 2.200 3.000 2.900 0.430

8 4.500 2.500 2.500 3.167 1.333

{

}

u=1

/8=1.245

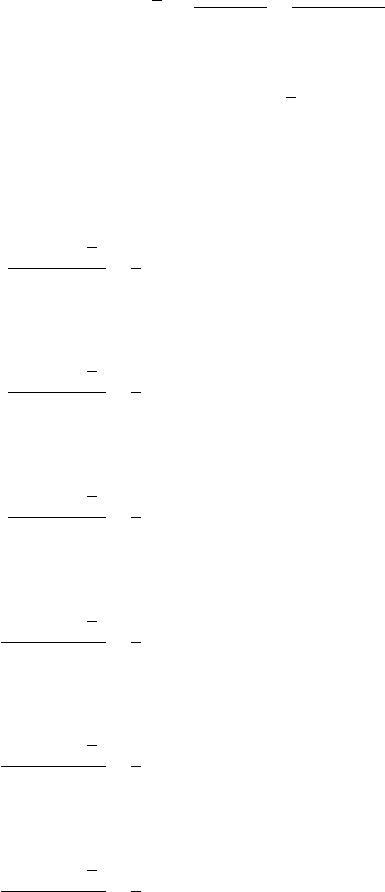
Design of Experiments in Metal Cutting Tests 299

over r repetitions) as

j=1

(5.23)

Because each factor (except for x

) is varied at two levels (+1 and −1), the calculations

are done by attributing to the entries of column

the signs of the entries of the column for

the corresponding factor, followed by algebraic summation of these entries. A regression

coefﬁcient is obtained by dividing the results by the number of plan point.

In the case examined

u=1

(

−0.67+0.41−0.23+0.31−0.54+0.30−0.19+0.29

)

=−0.04125

u=1

(

−0.67−0.41+0.23+0.31−0.54−0.30+0.19+0.29

)

=−0.1125

u=1

(

−0.67−0.41−0.23−0.31+0.54+0.30+0.19+0.29

)

=−0.0375

u=1

12u

(

0.67−0.41−0.23+0.31+0.54−0.30−0.19+0.29

)

=0.0850

u=1

13u

(

0.67−0.41+0.23−0.31−0.54+0.30−0.19+0.29

)

=0.0050

u=1

23u

(

0.67+0.41−0.23−0.31−0.54−0.30+0.19+0.29

)

=0.0225

300 Tribology of Metal Cutting

123

u=1

123u

(

−0.67+0.41+0.23−0.31+0.54−0.30−0.19+0.29

)

=0.0000

Calculation of b

is conducted using the same rule

u=1

(

0.67+0.41+0.23+0.31+0.54+0.30+0.19+0.29

)

=0.3675

Substituting these calculated regression coefﬁcients into Eq. (5.22), one obtains a

mathematical model in the transformed variables as

y =0.3675−0.0412x

−0.1125x

−0.0375x

+0.0850x

−0.0050x

+0.0225x

(5.24)

Statistical examination of the results obtained. Because DOE emanates from the sta-

tistical nature of the process considered, the mathematical model obtained should be

analyzed carefully. The objective of this analysis is dual: on one hand it is necessary to

extract the maximum information from the collected data; on the other hand, the reli-

ability and accuracy of the results obtained should be veriﬁed. The following procedure

for the examination of experimental data has been developed for metal cutting studies:

Calculation of the row variance and the variance of the response. The result of

each test run contains certain test error which is lowered by conducting the test several

times (r

) under the same test conditions, i.e. in each row of the test matrix. The row

variances are calculated using the data from Table 5.9 as

j=1



−y



−1

(5.25)

The results are shown in Table 5.9.

The variance of the response, s

{y}, is the arithmetic average of m different variants of

tests (that is the average variance); in other words

{

}

u=1

j=1



−y



m(r−1)

(5.26)

Design of Experiments in Metal Cutting Tests 301

The calculation of the variance of the response, s

{y}, is shown in Table 5.9. The

calculated variance is valid only when the raw variances are homogeneous.

Examination of the variance homogeneity. Because the errors obtained in the exper-

imental data in machining tests have normal distribution, the homogeneity test is

conducted using the statistical criteria of Fisher (F-criterion), Cochran and Bartlett.

Although the F-criterion is widely used for this purpose, one should remember that,

in general, it cannot be used when the number of variances under study is more than

two because this criterion takes into consideration only the maximum and minimum

variances and thus ignores the others. When the number of test repetitions at each point

of the design matrix is the same for all points, the Cochran’s criterion should be used

instead. This criterion is calculated as the maximum variance s

max

to the sum of all

variances. In the case considered

exp

0.1352

0.2834

=0.47 (5.27)

Using the table of Cochran numbers [17], the critical Cochran number is found to be

=0.61 for the degrees of freedom for the maximum variance f

−1=3−1=2

and the total degree of freedom of the variance f

=m×r =8×3=24 at 5% level of

signiﬁcance. Because G

exp

, the variances are considered to be homogeneous.

Examination of the signiﬁcance of the model coefﬁcients. Signiﬁcance testing

of each model coefﬁcient is evaluated independently using the t-criterion (Student’s

criterion). When using the complete factorial experiment, the conﬁdence intervals for all

the coefﬁcients of the model should be of equal width. First, the variance of regression

coefﬁcient, s

} is to be determined. When the number of repetitions (r

) is the same

for each point of the design matrix, this variance can be calculated using the following

formula:

{

}

{

}

(5.28)

with f

(

−1

)

, the number of degrees of freedom.

In the case considered, s

{

}

=0.03543/8·3=1.46×10

−3

or s

{

}

=0.038

Next step is to calculate the t-criterion for each model coefﬁcient as

{

}

(5.29)

In the considered case: t

=9.67, t

=1.05, t

=2.96, t

=0.99, t

=2.24, t

=0.13

and t

=0.59. The critical value of the t-criterion, t

is determined with f

(

−1

)

(

3−1

)

=16 degrees of freedom at a signiﬁcant level of α =5% using the statistical

tables. It was found that t

=1.74. If t

then coefﬁcient b

is considered to be

insigniﬁcant and thus b

=0. In the case considered, coefﬁcients b

, b

and b

are

found to be insigniﬁcant.

302 Tribology of Metal Cutting

The conﬁdence interval for each signiﬁcant coefﬁcient can also be determined as having

length 2∆b

where

∆b

{

}

=1.74×0.038=0.06612 (5.30)

As shown, all the coefﬁcients have the same conﬁdence interval. Coefﬁcient b

is con-

sidered to be signiﬁcant if its absolute value is more than half of the conﬁdence interval,

i.e. when

> ∆b

. The comparison of the results of Eq. (5.31) with the absolute values

of the remaining coefﬁcients shows that they all are still signiﬁcant.

The mathematical model can be re-written now including only the signiﬁcant coefﬁcients



y =0.3675−0.1125x

+0.085x

(5.31)

To obtain the model in real value of variables, it is necessary to substitute the

transformation given by Eq. (5.4) into Eq. (5.32)



y =0.3675−0.1125

ν−100

+0.085

ν−100

f −0.07

0.02

(5.32)

or ﬁnally



y =2.7446−0.0198ν−33.9583f +0.2833νf (5.33)

Equation (5.34) reveals that for the selected upper and lower limits of the factors, the

surface roughness in gundrilling depends not only on the cutting speed and feed singly,

but also on their interaction.

As follows from the foregoing analysis, the cutting ﬂuid ﬂow rate is found to be an

insigniﬁcant parameter for the selected limits of this factor. It is a common problem

at this stage of the statistical analysis that a particular factor (and the corresponding

regression coefﬁcient), which was thought to be signiﬁcant before testing, is found to be

insigniﬁcant. This situation might occur due to the following:

• The selected zero level ˜x

is too close to the point of a local extremum of factor ˜x

so that

∂





˜x



∂˜x

=0 (5.34)

• The selected interval of variation ∆˜x

is not sufﬁciently wide to detect the inﬂuence

of this factor on the response.

• Factors and/or interaction corresponding to the regression coefﬁcient under consid-

eration do not have a functional relationship with the response.

Design of Experiments in Metal Cutting Tests 303

• The large error occurred during the test is due to the presence of uncontrollable

variables.

When it is believed that the factor being considered has a signiﬁcant inﬂuence on the

response, then:

• The basic (zero) value of the factor should be changed.

• The interval of factor variation should be increased.

• The experiment should be repeated with reduced errors due to uncontrollable

variables.

In the case considered, the inﬂuence of cutting ﬂuid ﬂow rate (Q) is found to be insignif-

icant. This can be explained by a narrow range of the interval of variation of this factor.

But this interval cannot be increased because its upper limit is restricted by the cutting

ﬂuid pressure available in the machine while its lower limit is restricted by reliable chip

removal. Therefore, it can be concluded that the cutting ﬂuid ﬂow rate does not affect

the roughness of the machined hole within the realizable working regimes.

Adequacy of the model. The next step is to check the adequacy of the model obtained.

To do that, the differences between the predicted responses by the obtained model and

the mean of the experimentally obtained responses at each point of the design matrix

should be determined and analyzed. These differences are used to calculate the residual

variance, or variance of adequacy (s

). When the number of repetitions (r

) is the same

at each point of the design matrix then

m−n

u=1

(¯y−



)

, (5.35)

where n is the number of terms in Eq. (5.34) including the free term,

is the mean

response at u row of the design matrix and



is the response at the same point calculated

using Eq. (5.34).

The variance of adequacy is determined with the following degrees of freedom

=m−n =8−4 =4 (5.36)

The examination of the model adequacy includes the computation of the ratio “the vari-

ance of adequacy (s

)/the variance of the response (s

{

}

).” The procedure includes the

use of the F-criterion of Fisher as

F =

{

}

(5.37)

If the calculated value of F < F

, where F

is determined from the statistical table

for F-criterion under f

degrees of freedom and the selected level of signiﬁcance

(α%), then the model is considered as adequate. Otherwise, the model is considered

304 Tribology of Metal Cutting

to be inadequate. If the variance of adequacy (s

) does not exceed variance of the

response (s

{

}

), then F ≤1soF < F

is valid for any degrees of freedom.

In the case considered

m−n

u=1



−





3×0.0282

8−3

=0.01692 (5.38)

Because in the case considered s

{

}

(0.01692< 0.03543), the model adequacy is

obvious even without using the F-criterion calculations.

The examination of the model adequacy is possible only when f

> 0, i.e. when the

number of estimated coefﬁcients of the model (n) is less than the number of the points

in the design matrix (m). If, however, n =m then f

=0, i.e. no degrees of freedom

left to check the adequacy of the model. Experience, however, shows that fortunately in

practice, some regression coefﬁcients are always found to be insigniﬁcant [9].

Using the above-described procedure and data from Table 5.10, the following mathemat-

ical model for the roundness of the gundrilled hole is obtained

∆R

(

µm

)

=−20.044+0.238ν+396f +0.462Q−3.960νf

−0.005νQ−6.600fQ+0.066νf Q (5.39)

As shown, the roundness (surface ﬁnish) of the drilled holes depends not only on the

regime parameters but also on their interactions. Even though the cutting speed, feed

and cutting ﬂuid ﬂow rate have signiﬁcant inﬂuence of roundness, they cannot be judged

individually due to their interactions.

5.4.4 Example 2: tool life and cutting forces

There are certain cases in metal cutting experiments, where the mathematical models of

the response have special formats widely accepted and used in literature [18,19]. Some

of these formats may not be fully suitable for the statistical analysis. Therefore, a certain

transformation of a particular model to bring it into a format acceptable for DOE might

be necessary. The best example is the tool life model, which normally has the following

form:

T =Cν

, (5.40)

where T is tool life (min) and C is a constant mainly attributed to the properties of the

work material.

If Eq. (5.41) is selected as a mathematical model of the response, then in order to apply

the described methods of DOE, some transformation of this equation is needed. Taking

Design of Experiments in Metal Cutting Tests 305

the logarithms of both sides of this equation, a new mathematical model is obtained as

{

}

=β

+β

, (5.41)

where E

{

}

is the mathematical expectation of true tool life in the logarithmic scale,

and x

are logarithms of ν, f and d

, respectively, and β

,β

and β

are

coefﬁcients to be statistically estimated.

It should be explained here that the transformation described is not very strict from the

statistical viewpoint. First, it is not clear what happens to the experimental error included

in the original model. Second, the estimates for the coefﬁcient can be biased [2]. Third,

if the errors in Eq. (5.42) are multiplicative, then no problem can be expected. However,

if these errors are additive, there could be a problem if the experimentalist tries to isolate

the error term. Nonetheless, the practice of metal cutting studies has shown that such a

transformation may be accepted in most practical cases.

The regression equation for Eq. (5.42) can be written as



y =b

, (5.42)

where



y is the estimator for E

{

}

in Eq. (5.42), b

and b

are the estimators for

,β

and β

, respectively.

Equation (5.43) is an empirical model of tool life. To determine the model coefﬁcients,

factorial DOE can be used. If expression

(

lnx

imax

−lnx

imin

)

(5.43)

is selected to be a new scale unit then the transformation of the true values of the factor ˜x

to the transformed value of this factor (x

) will be basically the same as before (Eq. (5.4))

(

lnx

−lnx

imax

)

lnx

imax

−lnx

imin

+1 (5.44)

The results of the coordinate transformations are shown in Table 5.11. In this table, d

the width of the outer cutting edge.

The design matrix and the experimental results are shown in Table 5.12. Repeating the

above-discussed procedure, the regression equation was obtained as



y =4.04−0.96x

−0.29x

+0.13x

(5.45)

The statistical data evaluation should be conducted in a speciﬁc sequence. First of all,

one need to verify that the equation of the ﬁrst order (Eq. (5.46)) is statistically sufﬁcient

to describe the phenomenon under study. To do this, the null hypothesis should be

veriﬁed, i.e. it has to be checked out that the sum of all regression coefﬁcients

306 Tribology of Metal Cutting

of the second order terms x

is equal to zero. For the case under consideration: b

4.04; the mean response at the zero-level (point No. 9 of the matrix (Table 5.12)) is

=4.27; the variance of the response s

{

}

=0.25. Then b

−y

=4.04−4.23<s

{

}

Therefore, the quadratic effects are statistically negligible.

Substituting Eq. (5.45) into Eq. (5.46) one can obtain

y =9.55−1.37lnx

−0.41lnx

+0.19lnx

(5.46)

or in the common exponential form

T =

9.55

0.19

1.37

0.14

(5.47)

Table 5.11. Levels of factors and their intervals of variation (tool life and cutting

force tests).

Levels of factors Notation V (m/min) f (mm/rev) d

(mm)

lnx

Basic 0 125 4.83 0.125 −2.08 2.5 0.92

Interval of variation ∆x

75 4.32 0.075 −2.59 2.0 0.69

Upper (+) +1 200 5.30 0.200 −1.61 4.5 1.5

Lower (−) −1 50 3.91 0.050 −3.00 0.5 −0.69

Table 5.12. Design matrix and experimental results (tool life test).

Point x

Tool life (T) (min) (ln T)

1 +− −−95.0 156.0 132.0 128.0

4.55 5.04 4.88 4.82

2 ++ −−25.031.023.026.3

3.22 3.43 3.12 3.26

3 +− +−135.0 129.085.0116.3

4.90 4.85 4.44 4.75

4 ++ +−14.016.022.017.3

2.64 2.77 3.09 2.83

5 +− −+162.0 264.0 185.0 203.6

5.08 5.57 5.21 5.29

++ −+45.078.040.054.3

3.80 4.35 3.69 3.95

7 +− ++143.0 215.0 170.0 176.0

4.96 5.36 5.13 5.15

8 ++ ++10.08.012.010.0

2.30 2.08 2.48 2.30

9 1 0 0 0 124.068.045.079.0

4.81 4.22 3.80 4.27

4.04 −0.96 −0.29 0.13