Blank B.E., Krantz S.G. Calculus: Single Variable
Подождите немного. Документ загружается.

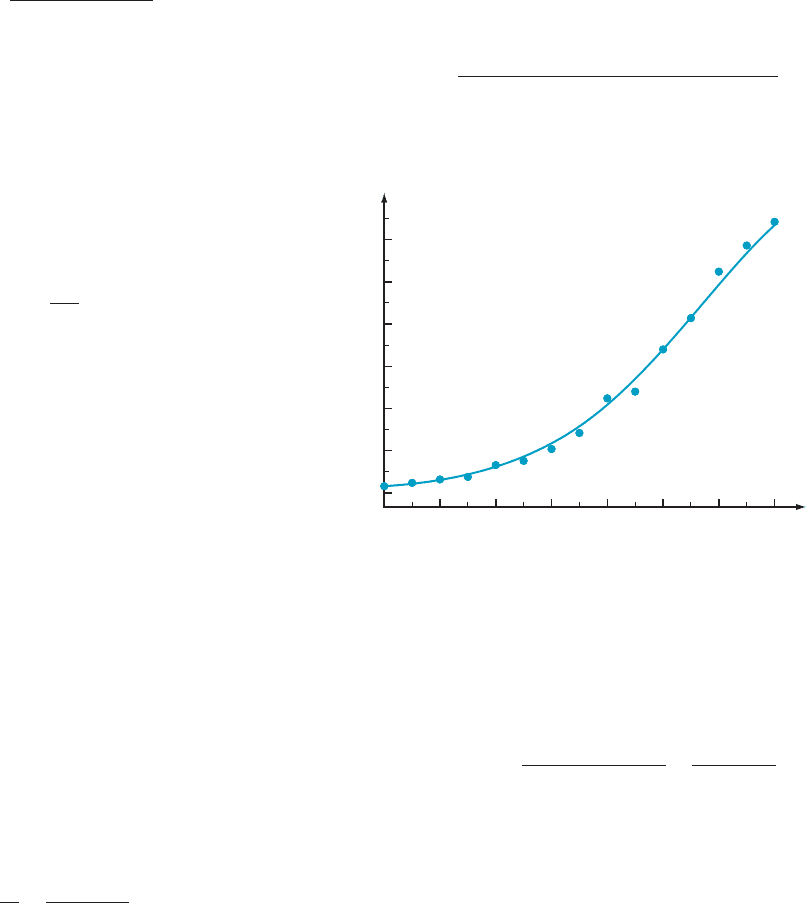
and
E
00
ðTÞ5
2
ðα=2 2 1Þβ 2 T
ðT 1 βÞ
4
EðTÞ:
Deduce that E is an increasing function of T with a sig-
moidal (or S-shaped) graph. What is the point of inflec-
tion of the graph of E?
92. Suppose that h
N
, k,andq are positive constants with q , 1.
Show that the Chapman-Richards function, defined by
hðtÞ5 h
N
1 2 expð2qktÞ
1=q
;
is the solution of the initial value problem
h
0
ðtÞ5 k hðtÞ
h
N
hðtÞ
q
2 1
; hð0Þ5 0;
which is used in forest management to model tree growth.
Here t represents time, and h measures tree height (or
some other growth indicator).
Calculator/Computer Exercises
c In each of Exercises 93296, solve the given initial value
problem for y(x). Determine the value of y(2). b
93. dy/dx 5 (1 1 x
2
) /(1 1 y
4
) y(0) 5 0
94. dy/dx 5 (x
2
) /(1 1 sin( y)) y(0) 5 0
95. y
2
dy/dx 5 (1 1 y)/(1 1 2x) y(0) 5 0
96. dy/dx 5 x/(1 1 e
y
) y(0) 5 0
97. The weight y of a goose is determined as a function of
time by the initial value problem dy=dt 5 0:09
ffiffi
t
p
ð5000 2 yÞ; yð0Þ5 175 when t is measured in weeks
after birth, and y is measured in grams. In how many
weeks does the goose reach half of its mature weight?
98. Suppose that in a certain ecosystem, x 100 denotes the
number of predators, and y 1000 denotes the number of
prey. Suppose further that the relationship between the
two population sizes satisfies the following Lotka-
Volterra equation:
dy
dx
5
y ð6 2 2xÞ
x ð4y 2 5Þ
:
Separate variables, and solve this equation. If the initial
prey population is 1500, and the initial predator popula-
tion is 200, what are the possible sizes of the population of
prey when the predator size is 300?
99. As the result of pollution reduction in the Great Lakes
region, certain species of wildlife that had been
threatened have made a comeback. Figure 13 contains a
plot of the number P of double-crested cormorant nests in
the Great Lakes region for the years 1979 through 1993.
A logistic growth curve has been superimposed. In this
exercise, you will obtain the formula for the logistic curve
PðtÞ5
P
0
P
N
P
0
1 ðP
N
2 P
0
Þexp
2k P
N
ðt 2 1979Þ
:
Use P
N
5 44000 for the carrying capacity, and use P
0
5
P(1979) 5 800 for the value of the initial population.
a. For t 5 1984, 1985, ..., 1988, use a central difference
quotient to approximate P
0
(t). For example,
P
0
ð1980Þ
Pð1981Þ2 Pð1979Þ
1981 2 1979
5
1600 2 800
2
:
The logistic differential equation P
0
(t) 5 kP(t)(P
N
2
P(t)) then permits an estimate for k. Average the five
estimates to get a working value of k.
b. Using the value of k obtained in part a, calculate P(t)
for t 5 1990, ...,1993. (Although the observed values
for these years appear in Figure 13, they were not used
to obtain the model. Comparing the predicted values
with the observed values is a reality check for the
model.)
c. Plot the logistic growth curve for 1979 # t # 1993.
d. According to your logistic model, about when did the
number of nests reach 43,000?
1993
800
1200
1600
3300
1900
19811979 1983
3800
5200
7100
12000
17000
20700
26200
29300
32100
11200
1985 1987 19911989
10000
5000
15000
20000
25000
30000
m Figure 13 Cormorant nests (19791993)
7.6 First Order Differential Equations—Separable Equations 605
7.7 First Order Differential Equations—Linear Equations
In the preceding section, you learned a method for solving the first order differ-
ential equation
dy
dx
5 Fðx; yÞ
when F(x, y) 5 g(x) h( y). There are several other methods that are similarly
adapted to other particular forms assumed by F(x, y). In this section, we will study
the case in which F(x, y) 5 q(x) 2 p(x) y.
Solving Linear
Differential Equations
We say that a first order differential equation of the form
dy
dx
5 qðxÞ2 pðxÞy
is linear. We often write a linear equation in the standard form
dy
dx
1 pðxÞy 5 qðxÞ: ð7: 7 :1Þ
This algebraic step does not separate variables, but it does eliminate the y variable
from the right side of the equation, and it prepares the left side for multiplication
by an “integrating factor” followed by a decisive application of the Product Rule
for differentiation.
THEOREM 1
Suppose that p(x ) and q(x) are continuous functions. Let P(x)be
any antiderivative of p(x). Then the general solution of the linear equation
dy
dx
1 pðxÞy 5 qðxÞ
is
yðxÞ5 e
2PðxÞ
Z
e
PðxÞ
qðxÞdx 1 Ce
2PðxÞ
; ð7:7:2Þ
where C is an arbitrary constant.
Proof. The
presence of both y
0
(x) and y(x) in the sum on the left side of equation
(7.7.1) leads us to consider the Product Rule for differentiation. If u is a function of
x that is never 0, then we have
d
dx
ðuyÞ5 u
dy
dx
1
du
dx
y 5 u
dy
dx
1
1
u
du
dx
y
:
The idea is to find a function u so that the factor of y on the right side of the
preceding equation matches the factor of y in (7.7.1). That is, we want to find a
function u such that
1
uðxÞ
du
dx
5 pðxÞ:
606 Chapter 7 Applications of the Integral

As we learned in Section 7.6, we solve this separable differential equation by
rewriting it as
Z
1
u
du 5
Z
pðxÞdx:
Because P(x) is an antiderivative of p(x), the general solution of the preceding
equation is ln(|u|) 5 P(x) 1 C
0
, where C
0
is a constant of integration. Because any
particular solution u will serve our purpose, we simplify the algebra by choosing a
solution u with u(x) . 0andC
0
5 0. With these choices, we have ln(u) 5 P(x), or
u(x) 5 e
P(x)
. This is our integrat ing factor. On multiplying each side of equation
(7.7.1) by e
P(x)
, we obtain
pðxÞe
PðxÞ
y 1 e
PðxÞ
dy
dx
5 e
PðxÞ
qðxÞ:
Thus
d
dx
e
PðxÞ
y
5
d
dx
e
PðxÞ
y 1 e
PðxÞ
dy
dx
5 P
0
ðxÞe
PðxÞ
y 1 e
PðxÞ
dy
dx
5 pðxÞe
PðxÞ
y 1 e
PðxÞ
dy
dx
5 e
PðxÞ
qðxÞ:
In other words, e
P(x)
y is an antiderivative of e
P(x)
q(x). That is,
e
PðxÞ
y 5
Z
e
PðxÞ
qðxÞdx 1 C;
from which formula (7.7.2) is obtained on divi ding each side by e
P(x)
. ’
⁄ EXAMPLE 1 Solve the initial value problem
dy
dx
1
2y
x
5 4x; yð2Þ5 3:
Solution The
given differential equation is linear: It has the form of equation
(7.7.1) with p(x) 5 2/x and q(x) 5 4x. Because
Z
pðxÞdx 5
Z
2
x
dx 5 2lnðjxjÞ1 C;
and because we may choose any antiderivative of p(x)forP(x), we set P(x) 5 2ln(|x|).
We note from the initial condition that the positive number 2 must belong to the
interval on which the required solution y(x) is defined. This interval of definition
cannot contain any negative number because, if it did, then 0 would be in the interval.
However, we see that the differential equation is not even defined when x 5 0, so it
cannot hold when x 5 0. Thus the domain of definition of y is limited to positive values.
We may therefore dispense with the absolute value function and take P(x) 5 2ln(x).
Thus
e
PðxÞ
5 e
2lnðxÞ
5 e
lnðx
2
Þ
5 x
2
and equation (7.7.2) become
yðxÞ5 x
22
Z
x
2
4xdx1 C x
22
5
4
x
2
Z
x
3
dx 1
C
x
2
5
4
x
2
x
4
4
1
C
x
2
5 x
2
1
C
x
2
:
Because y(2) 5 2
2
1 C/2
2
5 4 1 C/4, the initial condition tells us that 4 1 C/4 5 3. It
follows that C 524, and y(x) 5 x
2
2 4/x
2
. ¥
7.7 First Order Differential Equations—Linear Equations 607
⁄ EXAMPLE 2 Solve the initial value problem
x
dy
dx
1 y 5 sinðxÞ; yðπ=2Þ5 2:
Solution To
put this equation into the form of equation (7.7.1), we divide by x and
obtain
dy
dx
1
1
x
y 5
sinðxÞ
x
:
We set p(x) 5 1/x, q(x) 5 sin(x)/x , and take P( x ) 5 ln(x) for an antiderivative of
p(x). (The reasoning for taking ln(x) instead of ln(|x|) 1 C is similar to that
of Example 1.) It follows that e
P(x)
5 e
ln(x)
5 x and equation (7.7.2) become
yðxÞ5 x
21
Z
x
sinðxÞ
x
dx 1 C x
21
5
1
x
Z
sinðxÞdx 1
C
x
5
C 2 cosðxÞ
x
:
Because y(π/2) 5 2, we have
C 2 cosðπ=2Þ
π=2
5 2;
or C 5 π. Thus
yðxÞ5
π 2 cosðxÞ
x
: ¥¥
⁄ EX
AMPLE 3 Find the general solution of the linear differential equation
dy
dx
1 tanðxÞy 5 secðxÞ
0 , x ,
π
2
:
Do not use equation (7.7.2). Instead, use the technique found in the proof of
Theorem 1.
Solution We
recall that ln(sec(x)) is an antid erivative of tan(x). In the notation of
Theorem 1, q(x) 5 sec(x), p(x) 5 tan(x), and P(x) 5 ln(sec(x)). We multiply each side
of the given differential equation by e
P(x)
. Observing that e
P(x)
5 e
ln(sec(x))
5 sec(x),
we obtain
secðxÞ tanðxÞy 1 secðxÞ
dy
dx
5 sec
2
ðxÞ;
or
d
dx
secðxÞ
y 1 secðxÞ
dy
dx
5 sec
2
ðxÞ;
or
d
dx
secðxÞy
5 sec
2
ðxÞ:
It follows that
secðxÞy 5
Z
sec
2
ðxÞdx 5 tanðxÞ1 C;
608 Chapter 7 Applications of the Integral
or
y 5
tanðxÞ
secðxÞ
1
C
secðxÞ
5 sinðxÞ1 C cos ðxÞ: ¥¥
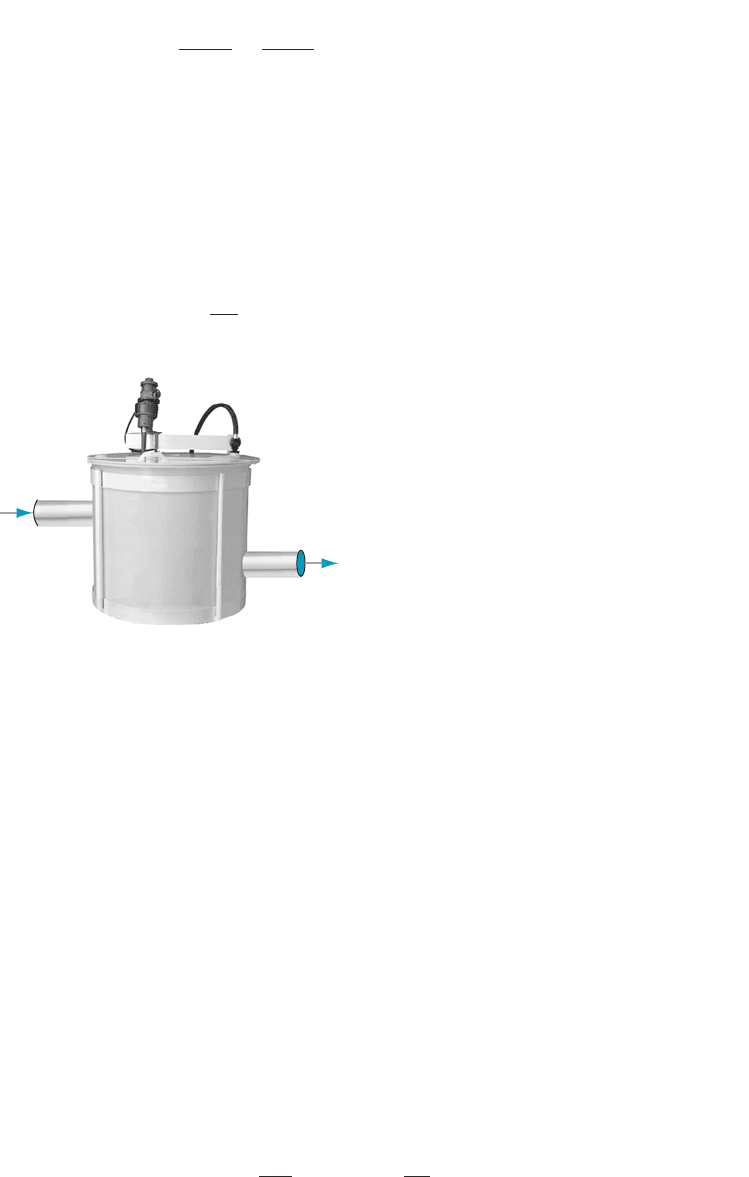
An Application: Mixing
Problems
Mixing problems occur frequently in biology, chemistry, medicine, and pharma-
cology. In a basic mixing problem, a container contains a substance in solution.
There is an inlet to the co ntainer as well as an outlet (see Figure 1). When fluid
enters and exits the tank through these channels, the solution in the tank can
become enriched or diluted. If m is the mass of the dissolved sub stance, then the
basic equation becomes
dm
dt
5 ðrate inÞ 2 ðrate outÞ: ð7:7:3Þ
To apply the theory of linear equations, we must assume that the solution is
thoroughly mixed at all times so that the concentration of the solution is homo-
geneous throughout the container. (Nearly all mathematical models involve some
approximation. Many involve a compromise between accuracy and simplicity. A
completely accurate model that is too difficult to solve may do us little good. By
simplifying the model somewhat, we may be able to obtain a useful solution
without sacrificing too much accuracy.)
⁄ EX
AMPLE 4 A 200 gallon tank is filled with a salt solution initially con-
taining 10 pounds of salt. An inlet p ipe brings in a solu tion of salt at the rate of 10
gallons per minute. The concentratio n of salt in the incoming solution is 1 2 e
2t/60
pounds per gallon when t is measured in minutes. An outlet pipe prevents overflow by
allowing 10 gallons per minute to flow out of the tank. How many pounds of salt are in
the tank at time t? Long term, about how many pounds of salt will be in the tank?
Solution Let m(t)
be the amount of salt in the tank at time t. To calculate the
amounts of salt entering the tank through one pipe and exiting through another, we
multiply the rate at which the fluid enters and exits by the appropriate
concentrations. The rate at which salt enters the tank is
10
gal
min
ð1 2 e
2t=60
Þ
lb
gal
;
Fluid in
ACME
Fluid out
-210
-
-190
-
170
-
-150
-
130
-
-110
-
-90
-
-70
-
-50
G
A
L
L
O
N
S
m Figure 1
7.7 First Order Differential Equations—Linear Equations 609

or 10 ð1 2 e
2t=60
Þ pounds per min ute. The rate at which salt leaves the tank is
10
gal
min
m
200
lb
gal
;
or m/20 pounds per minute. Equation (7.7.3) becomes
dm
dt
5 10
1 2 e
2t=60
2
m
20
;
or
dm
dt
1
1
20
m 5 10
1 2 e
2t=60
;
which is a linear differential equation. Of course, the use of t rather than x as an
independent variable does not affect the applicability of Theorem 1. We simply use
t instead of x and set p(t) 5 1/20 and q(t) 5 10(1 2 e
2t/60
). Then P(t) 5 t/20 and
equation (7.7.2) gives us
mðt Þ5 e
2t=20
Z
e
t=20
10
1 2 e
2t=60
dt 1 Ce
2t=20
5 10 e
2t=20
Z
e
t=20
2 e
t=30
dt 1 Ce
2t=20
;
or
mðtÞ5 100
2 2 3e
2t=60
1 Ce
2t=20
:
Because m(0) 5 10, we have 10(20 2 30e
20/60
) 1 Ce
20/20
5 10, or 10(20 2 30) 1
C 5 10, or C 5 110. Thus
mðt Þ5 100
2 2 3e
2t=60
1 110e
2t=20
:
Notice that
lim
t-N
100
2 2 3e
2t=60
1 110e
2t=20
5
100ð2 2 3 0Þ1 110 0
5 200:
Thus the amount of salt in the tank approaches 200 pounds. Figure 2 plots the
increasing quantity of salt for the first five hours of the process.
¥
An Application:
Electric Circuits
Figure 3 is the schematic diagram of a simple electric circuit involving an elec-
tromotive force of E(t) volts (such as produced by a battery or a generator), a
resistor with a resistance of R ohms (Ω), and an inductor with inductance of L
y
t
200
150
100
50
100
y 200
0 200 300
(
minutes
)
m(t) 100(2 3e
t/60
)110e
t/20
m Figure 2
610 Chapter
7 Applications of the Integral

henrys (h). It is a consequence of Kirchhoff’s voltage law that the current I
(measured in amperes) satisfies
L
dI
dt
1 R I 5 EðtÞ:
⁄ EX
AMPLE 5 In the circuit of Figure 3, what is I(t)ifL 5 3 h, R 5 6 Ω,and
E(t) 5 12 cos(2t)V?
Solution After
dividing by L and substituting the given values, we obtain
dI
dt
1 2 I 5 4 cosð2tÞ:
In the notation of Theorem 1 (with I an d t taking the roles of y and x, respectively),
we have q(t) 5 4 cos(2t), p(t) 5 2, P(t) 5 2t, and e
P(t)
5 e
2t
. Theorem 1 tells us that
the integral
Z
e
PðtÞ
qðtÞdt 5
Z
e
2t
cosð2tÞdt
arises in the solution. To evaluate this integral, we make the substitution x 5 2t,
dx 5 2dt, which, by Example 5 of Section 6.1 in Chapter 6, results in
1
2
Z
e
x
cosðxÞdx; or
1
4
e
x
sinðxÞ1 cosðxÞ
1 C. On resubstituting, we obtain
Z
e
PðtÞ
qðtÞdt 5
Z
e
2t
cosð2tÞdt 5
1
4
e
2t
sinð2tÞ1 cosð2tÞ
1 C:
Equation (7.7.2) of Theorem 1 then gives us
IðtÞ5 e
2PðtÞ
Z
e
PðtÞ
qðtÞdt 1 Ce
2PðtÞ
5 4e
22t
Z
e
2t
cosð2tÞdt 1 Ce
22t
5 cosð2tÞ1 sinð2tÞ1 Ce
22t
: ¥
Linear Equations with
Constant Coefficients
Linear equations with constant coefficients arise frequen tly in applications. These
differential equations have the form dy/dt 1 βy 5 α. By rewriting this equation in
the form dy/dt 5 α 2 βy, we see that it is a separable differential equation (in
addition to being linear). Although we may solve it by using the techniques we
learned in Section 7.6, we will simplify the calculation by appealing to Theorem 1.
THEOREM 2
Suppose that α and β are constants with β 6¼0. Then the linear
differential equation
dy
dt
1 βy 5 α ð7:7:4Þ
has general solution
yðtÞ5
α
β
1 Ce
2βt
: ð7:7:5Þ
The initial value pro blem
dy
dt
1 β y 5 α; yð0Þ5 y
0
ð7:7:6Þ
has unique solution
yðtÞ5
α
β
1
y
0
2
α
β
e
2βt
: ð7:7 :7Þ
L
R
E
m Figure 3
7.7 First Order Differential Equations—Linear Equations 611
Proof. Equation (7.7.4) is the special case of equation (7.7.1) that results from
setting q(t) 5 α and p(t) 5 β. Because P(t) 5 βt is an antiderivative of p(t), equation
(7.7.2) tells us that equation (7.7.4) has solution
yðtÞ5 e
2βt
Z
e
βt
α dx 1 Ce
2βt
5
α
β
1 Ce
2βt
;
which establishes equation (7.7.5). If y(0) 5 y
0
, then α/β 1 Ce
2β 0
5 y
0
,or
C 5 y
0
2 α/β. Substituting this formula for C into equation (7.7.5) results in
formula (7.7.7). ’
We will illustrate Theorem 2 with several different applications. In a text book,
it
is useful to quote formulas such as (7.7.5) and (7.7.7) to avoid consecutive
repetitions of what would be essentially the same calculation. Nevertheless, a
student will find it more useful to understand the general technique of solving
equation (7.7.4) than to memorize the actual solution. Many equations and for-
mulas appear in calculus textbooks. The study of calculus is simplified by mastering
the basic ones and learning the techniques that treat the variants.
Newton’s Law for
Temperature Change
Suppose that T is the temperature of an object that is surrounded by a body (e.g.,
water, air, etc.) at const ant temperature T
N
—the reason for using this subscript will
become apparent. Newton’s Law states that the rate of change of T is proportional
to the difference between T and T
N
. That is,
dT
dt
5 k ðT
N
2 TÞ; ð7:7:8Þ
where the co nstant of proportionality, k, is positive. Note that if T (t) , T
N
, then the
right side of equation (7.7.8) is positive and therefore 0 , T
0
(t). As a result, T(t)
increases, and we refer to Newton’s Law of Heating. If, instead, T
N
, T(t), then
T
0
(t) , 0 and T decreases. In this case, we refer to Newton’s Law of Cooling.
THEOREM 3
Suppose that T
0
5 T(0) is the temperature of an object when it is
placed in an environment that has constant temperature T
N
. Suppos e that the
temperature T(t) of the object is governed by Newton’s law of temperature
change. That is, suppose that T(t) is a solution of equation (7.7.8). Then
TðtÞ5 T
N
1 ðT
0
2 T
N
Þe
2kt
ð0 # t , NÞ: ð7:7:9Þ
Proof. The
right side of equation (7.7.8) can be rew ritten as k T
N
2 k T,or
α 2 βT with α 5 k T
N
and β 5 k. With these values of α and β, equation (7.7.8)
becomes
dT
dt
1 βT 5 α:
Formula (7.7.7) gives a formula for the unique solution of the initial value problem:
TðtÞ5
α
β
1
T
0
2
α
β
e
2βt
5
kT
N
k
1
T
0
2
kT
N
k
k
e
2kt
5 T
N
1 ðT
0
2 T
N
Þe
2kt
: ’
612 Chapter 7 Applications of the Integral

Notice that e
2kt
-0ast-N and therefore
lim
t-N
TðtÞ5 lim
t-N
T
N
1 ðT
0
2 T
N
Þe
2kt
5 T
N
1 ðT
0
2 T
N
Þ lim
t-N
e
2kt
5 T
N
1 ðT
0
2 T
N
Þ 05 T
N
:
Thus as t-N, the temperature of the object approaches the ambient temperature
T
N
.
⁄ EX
AMPLE 6 A thermometer is at room temperature (20.0
C). One min-
ute after being placed in a patient’s throat, it reads 38.0
C. One minute later, it
reads 38.3
C. Is this second reading an accurate measure (to three significant digits)
of the patient’s temperature?
Solution Let T(t)
denote the temperature of the thermometer at time t with t 5 0
corresponding to the moment it is placed in the patient’s throat. Let T
N
denote the
actual temperature of the patient. Then,
TðtÞ5 T
N
1 ðT
0
2 T
N
Þe
2kt
with T
0
5 20. Although there are two unknowns (k and T
N
) in this problem, we are
given two observations with which to determine them :
38 5 Tð1Þ5 T
N
1 ð20 2 T
N
Þe
2k
and
38:3 5 Tð2Þ5 T
N
1 ð20 2 T
N
Þe
22k
: ð7:7:10 Þ
The first of these eq uations may be written as
38 2 T
N
20 2 T
N
5 e
2k
: ð7:7:11Þ
Because e
22k
equals (e
2k
)
2
, we may replace e
22k
in equation (7.7.10) with the
square of the left side of (7.7.11). The result is
38:3 5 T
N
1 ð20 2 T
N
Þ
38 2 T
N
20 2 T
N
2
:
This equation simplifies to a linear equation in the unknown T
N
, which we solve to
find T
N
5 38.305 . . . . The reading at t 5 2 is therefore an accurate meas ure of the
patient’s temperature to three significant digits.
¥
INSIGHT
In Example 6, we may calculate e
2k
5 0.016662 . . . from equation (7.7.11)
and the previously calculated T
N
5 38.305. When we substitute the values of T
0
, T
N
, and
e
2k
into equation (7.7.9), we find that T( t) 5 38.305 2 18.305 (0.016662)
t
. The graph of
T(t) in the window [0, 2] 3 [30, 40] (see Figure 4a) indicates that T(t) is very close to T
N
after t 5 1.3. A closer look using the window [1.5, 2.5] 3 [38.266, 38.305] reveals that T(t)
does continue to increase, but very slowly (Figure 4b). This phenomenon is familiar to
anyone who has watched the readout of a digital thermometer while waiting for its beep.
The Linear Drag Law We consider vertical motion above Earth’s surface. Let y(t) denote the height of an
object above Earth’s surface at time t and v 5 dy/dt the velocity. The force R of air
resistance (or air drag ) can often be approximated by a linear velocity law:
RðvÞ52k v: ð7:7:12Þ
T
t
40
35
30
25
0.5 1.0 1.5 2.0
T(t) T
(T
0
T
)e
Kt
m Figure 4a
T
t
38.304
38.305
38.302
2.5 3.0
m Figure 4b
7.7 First Order Differential Equations—Linear Equations 613
Here k is a positive constant whose units are mass 3 time
21
. Notice that the minus
sign in formula (7.7.12) ensures that R(v)andv have opposite signs. This means
that the direction of air drag is opposite to the direction of motion. (In upward
motion, y is increasing, v 5 y
0
is positive, and R(v) 52kv is negative, hence a
downward force. In downward motion, y is decreasing, v 5 y
0
is negative, and
R(v) 52kv is positive, hence an upward force.)
⁄ EX
AMPLE 7 Let v(t) denote the velocity at time t of an object of mass m
that is dropped from a great height. Assuming that air resistance is given by the
formula (7.7.12), determine v(t). What is the behavior of v(t) for large t?
Solution Denot
ing the acceleration due to gravity by the positive constant g,we
write the downward force of gravity as 2m g. The equation of motion is therefore
m:
dv
dt
mass 3 acceleration
|fflfflfflfflfflfflfflfflfflfflffl{zfflfflfflfflfflfflfflfflfflfflffl}
Total Force
52k v
|fflffl{zfflffl}
Resistive Force
1 ð2m gÞ
|fflfflfflfflffl{zfflfflfflfflffl}
Force of gravity
:
On dividing by m and bringing v to the left side, we obtain the initial value problem
dv
dt
1
k
m
v 52g; vð0Þ5 0:
This initial value problem is of the form (7.7.6) with unknown function v instead of y.
To complete the correspondence, we set α 52g, β 5 k/m,andy
0
5 v(0) 5 0 in (7.7.6).
The solution to our initial value problem is then obtained by using these values for the
constants and replacing y with v in equation (7.7.7). We obtain
vðtÞ5
α
β
1
y
0
2
α
β
e
2βt
|fflfflfflfflfflfflfflfflfflfflfflfflfflfflffl{zfflfflfflfflfflfflfflfflfflfflfflfflfflfflffl}
right side of ð7:7:7Þ
5
2g
k=m
1
0 2
ð2gÞ
k=m
e
2kt=m
;
or
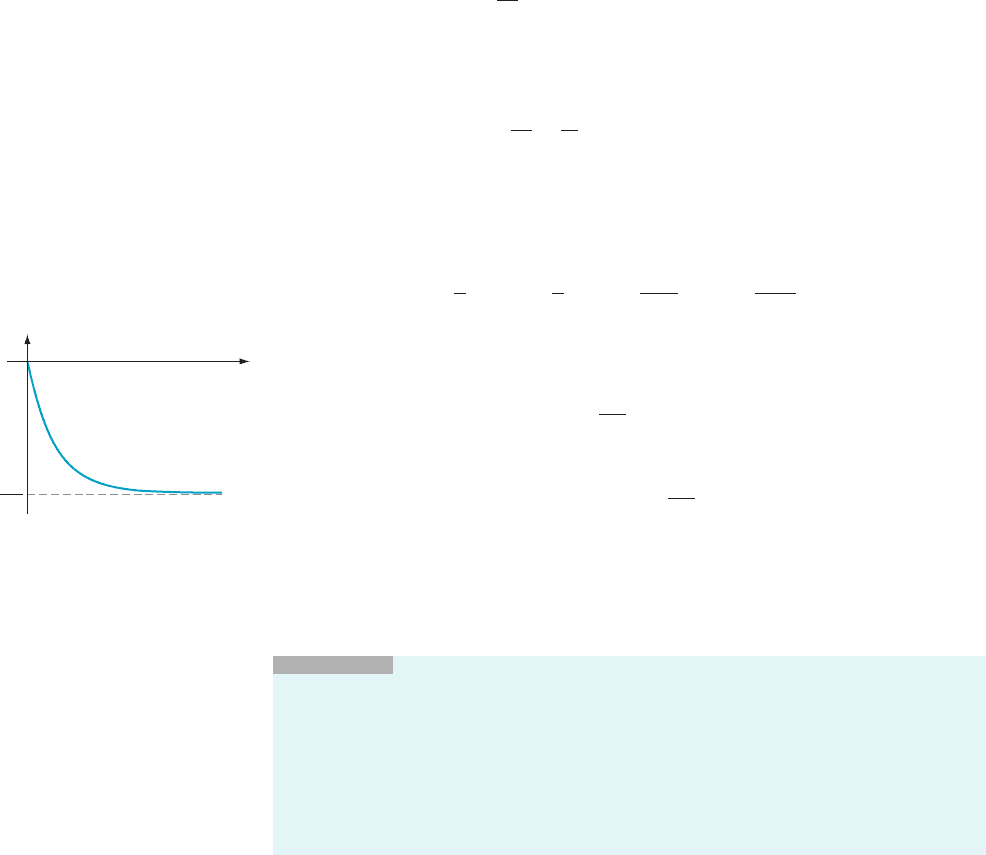
vðtÞ52
mg
k
1 2 e
2kt=m
: ð7:7:13Þ
From this formula, we can calculate
lim
t-N
vðtÞ52
mg
k
:
The quantity 2mg/k is said to be the terminal velocity of the falling object. This
terminology is somewhat misleading: Terminal velocity is approached arbitrarily
closely but is not attained. Figure 5 shows the graph of v and its horizontal
asymptote v 52mg /k.
¥
INSIGHT
One of the reasons that students from so many different backgrounds
study calculus is its wide applicability. Mathematicians will often state a theorem in a
general form (such as the formulation of Theorem 2) in order to maximize the theorem’s
scope. We have already seen that the differential equation studied in Theorem 2 applies
to problems concerning heat transfer and motion. Here are four more applications of the
same differential equation.
1. A drug delivered intravenously to a patient enters his bloodstream at a constant
rate. The drug is broken down and eliminated from the patient’s body at a rate that
v(t)
t
v
mg
K
m Figure 5 Plot of velocity dur-
ing a fall under gravity, assuming
the linear drag law
614 Chapter 7 Applications of the Integral
