Galdi G.P. An Introduction to the Mathematical Theory of the Navier-Stokes Equations: Steady-State Problems
Подождите немного. Документ загружается.

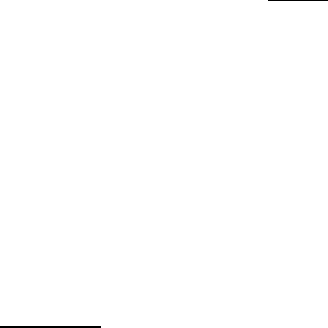
936 XIII Steady Navier–Stokes Flow in Domains with Unbounded Boundaries
they exist without restriction on the coefficient of kinematical viscosity ν or
on the flux Φ. This nice circumstance is due to the fact that, in the case under
consideration, for any η > 0 there is a solenoidal vector a = a(x; η) ∈
b
D
1,2
0
(Ω)
carrying the flux Φ such that
Z
Ω
u · ∇a · u
≤ η
Z
Ω
∇u : ∇u, for all u ∈ D(Ω). (XIII.7.1)
The validity of (XIII.7.1), in turn, is made possible by the fact that the “out-
lets” to infinity for the domain (XIII.5.1) have an unbounded cross section.
The existence of such fields a(x; η) was discovered by Ladyzhenskaya & Solon-
nikov (1977, §2). It should be remarked that the method used by these authors
to construct the fields a(x; η) applies to doma ins whose outlets to infinity Ω
i
are more general than those of domain (XIII.5.1); in fact, the only condition
required is that each Ω
i
contains a semi-infinite cone; cf. also Solonnikov &
Pileckas (1 977, Lemma 4), Solonnikov (1981), and Solonnikov (1983, §2.4)
The construction of the field a(x; η) is the object of the next lemma. As
the reader will recognize, this construction resembles the one given in Lemma
IX.4. 2 f or the case of a flow in a bounded domain.
Lemma XIII.7.1 Let Ω be as i n (XIII.5.1) and let η > 0. Then there exists
a solenoidal vector field a = a(x; η) ∈ C
∞
(Ω) vanishing in a neighborhood of
∂Ω such that
(i) |a(x)| ≤ M|x|
−2
; |∇a(x)| ≤ M|x|
−3
, for all sufficiently large |x|;
(ii) a ∈
b
D
1,2
0
(Ω);
(iii)
Z
S
a
3
= 1;
(iv) a verifies (XIII.7.1).
Proof. Let
b =
1
2π|x
0
|
2
(−x
2
, x
1
, 0) .
Clearly,
∇ × b = 0
∇· b = 0
)
in Ω − {0}.
We next introduce a system of cylindrical co ordinates (r, θ, x
3
) with the origin
at the center of the unit disk C = {|x
0
| < 1} which, without loss, we have as-
sumed to be strictly contained in S. The three unit vectors w ill be denoted by
e
r
, e
θ
, and e
3
, respectively. By a sim ple computation based on the properties
of b we find
Z
∂S
b × n ·e
3
= −1, (XIII.7.2)
where n is the exterior unit normal to ∂S.
1
In fact, introducing
1
Since S is locally Lipschitz, n exists a.e. on ∂S; cf. Lemma II.4.1.

XIII.7 Aperture Domain: Existence and Uniqueness 937
w = (b
2
, −b
1
, 0)
we have
∇
0
· w = 0 in ω = S − C,
where ∇
0
is the divergence operator restricted to the variables x
0
. Thus, by
the divergence theorem,
Z
∂S
w · n =
Z
∂C
w · e
r
.
Since for any vector s = (s
1
, s
2
, 0), w · s = −b × s · e
3
, it follows that
−
Z
∂S
b × n · e
3
= −
Z
∂C
b × e
r
· e
3
=
1
2π
Z
2π
0
(sin
2
θ + cos
2
θ)dθ = 1,
which shows (XIII.7.2). Now, let ρ = ρ(x) denote the regularized distance of
x from ∂Ω. By Lemma III.6.1 we obtain, in particular,
δ(x) ≤ ρ(x) ≤ k
1
δ(x)
|D
α
ρ(x)| ≤ k
2
[δ(x)]
1−|α|
, 0 ≤ |α| ≤ 2
(XIII.7.3)
where δ(x) = dist (x, ∂Ω) and k
1
, k
2
are independent of x. Let γ denote the
x
3
-axis and set
d = dist (∂S, γ).
Let σ, ψ be smooth nondecreasing functions of the real variable t such that
σ(t) =
d
2
if t ≤ d/2
t if t ≥ d
and
ψ(t) =
(
0 if t ≤ 0
1 if t ≥ 1.
Finally, we set
ζ(x) = ψ
ε ln
σ(|x
0
|)
ρ(x)
, ε ∈ (0, 1). (XIII.7.4)
In view of the property of the function ψ, we at once recognize that
ζ(x) =
(
0 if σ(|x
0
|) ≤ ρ(x)
1 if σ(|x
0
|) ≥ ρ(x)e
1/ε
(XIII.7.5)
which implies, i n particular,
supp (∇ζ) ⊂
n
x ∈ Ω : ρ(x) ≤ σ(|x
0
|) ≤ ρ(x)e
1/ε
o
. (XIII.7.6)

938 XIII Steady Navier–Stokes Flow in Domains with Unbounded Boundaries
Moreover, setting
C = {x ∈ Ω : |x
0
| < d/2}
it is im mediately seen that
ζ(x) = 0, for all x ∈ C. (XIII.7.7)
In fact, for x ∈ C we have
δ(x) ≥ d − |x
0
| ≥ d/2
which, by (XIII.7.3), in turn yields
ρ(x) ≥ d/2, for all x ∈ C. (XIII.7.8)
In addition, if x ∈ C, from the definition of the function σ we derive
σ(|x
0
|) = d/2, for all x ∈ C. (XIII. 7.9)
Thus, from (XIII.7.8) and (XI II.7.9) we find
ρ(x) ≥ σ(|x
0
|), for all x ∈ C,
which, by (XIII.7.5), implies (XII I .7.7). Also, setting
N
ε
=
x ∈ Ω : δ(x) ≤
de
−1/ε
2k
1
,
we have
ζ(x) = 1, for all x ∈ N
ε
. (XIII.7.10)
In fact, since σ is nondecreasing,
σ(|x
0
|) ≥ d/2,
and so, by (XIII.7.5), we have ζ = 1 whenever
ρ(x) ≤
d
2
e
−1/ε
,
which, by (XIII.7.3) i mplies (XIII. 7.10). The next task is to prove an esti-
mate for ∇ζ and D
2
ζ at large distances. To this end, we observe that, by a
straightforward computation, we obtain
∂ζ
∂x
k
= εψ
0
S
k
,
∂
2
ζ
∂x
k
∂x
l
= ε
2
ψ
00
S
k
S
l
+ εψ
0
∂S
k
∂x
l
where the prime means differentiation with respect to the argument involved
and

XIII.7 Aperture Domain: Existence and Uniqueness 939
S
k
= σ
0
∂|x
0
|
∂x
k
1
σ(|x
0
|)
−
1
ρ(x)
∂ρ(x)
∂x
k
∂S
k
∂x
l
=
σ
00
σ(|x
0
|)
−
(σ
0
)
2
σ
2
(|x
0
|)
∂|x
0
|
∂x
k
∂|x
0
|
∂x
l
+
σ
0
σ(|x
0
|)
∂
2
|x
0
|
∂x
k
∂x
l
+
1
ρ
2
(x)
∂ρ
∂x
k
∂ρ
∂x
l
−
1
ρ(x)
∂
2
ρ
∂x
k
∂x
l
.
Therefore, observing that
|ψ
0
|, |ψ
00
|, |σ
0
|, |σ
00
| ≤ M,
for some M independent of x, by virtue of (XIII.7.3), (XIII.7.6), and (XIII.7.7)
we conclude that
|∇ζ(x)| ≤ εc
1
δ
−1
(x)
|D
2
ζ(x)| ≤ c
2
δ
−2
(x)
)
for all x ∈ Ω, (XIII.7.11)
with c
1
and c
2
independent of x. The field a is introduced by the following
relation
a = ∇ × (ζb). (XIII.7.12)
Clearly, a is solenoidal and, in view of (XIII.7.7) it is also in C
∞
(Ω). From
the identity ∇ × (ζb) = ∇ζ × b + ζ∇ × b a nd the properties of b we have
a = ∇ζ × b (XIII.7.13)
from which, and with the help of (XIII.7.10), it follows tha t
a(x) = 0, for all x ∈ N
ε
. (XIII.7.14)
Furthermore, we have
Z
S
a
3
=
Z
S
(D
1
(ζb
2
) −D
2
(ζb
1
))
and so, by the divergence theorem, (XIII.7.2), and (XIII. 7.10), we infer
Z
S
a
3
=
Z
∂S
ζ(b
2
n
1
− b
1
n
2
) = −
Z
∂S
b × n · e
3
= 1,
which proves property (iii). From (XIII.7.11) and (XIII.7.13) we find
|a(x)| ≤
c
3
δ(x)|x
0
|
|∇a(x)| ≤ c
4
1
δ(x)|x
0
|
2
+
1
δ
2
(x)|x
0
|
all x ∈ supp (a) ⊂ supp (∇ζ).
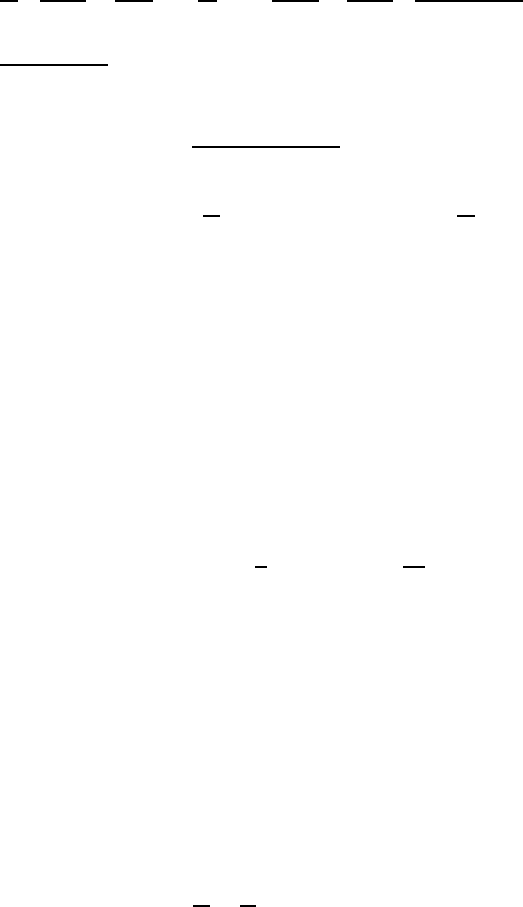
940 XIII Steady Navier–Stokes Flow in Domains with Unbounded Boundaries
If we take |x| sufficiently large, |x| > R (say), from (XIII.7.6) and the definition
of σ we have
δ(x) ≤ c
5
|x
0
| ≤ c
6
δ(x), x ∈ supp (a).
Therefore, for all x ∈ supp (a) with |x| > R, we find
|a(x)| ≤
c
3
2
1
δ
2
(x)
+
1
|x
0
|
2
=
c
3
2
2 +
|x
0
|
2
δ
2
(x)
+
δ
2
(x)
|x
0
|
2
1
δ
2
(x) + |x
0
|
2
≤
c
7
δ
2
(x) + |x
0
|
2
and, likewise,
|∇a(x)| ≤
c
8
(δ
2
(x) + |x
0
|
2
)
3/2
.
Observing that δ
2
(x) ≥ x
2
3
, property (i) follows from these latter inequalities.
Using (i) and the fact that a ∈ C
∞
(Ω
0
), for all bounded Ω
0
with Ω
0
⊂ Ω, we
deduce, in particular, that
Z
Ω
|∇a(x)|
2
< ∞.
Therefore,
a ∈ D
1,2
(Ω)
and since a is solenoidal and by (XIII.7.14) it vanishes i n a neighborhood of
∂Ω, we may conclude, with the help of a standard “cut-off” argument, the
validity of statement (ii) in the lemma. It remains to prove condition (iv). We
begin to observe that, gi ven η > 0, using integration by parts and the Schwarz
inequality, f or all u ∈ D(Ω),
Z
Ω
u · ∇a · u
=
Z
Ω
u · ∇u ·a
≤
η
2
Z
Ω
∇u : ∇u +
1
2η
Z
Ω
u
2
a
2
holds. As a consequence, to prove (iv), we w ill show tha t, given an arbitrary
small λ > 0, we may select ε in such a way that
Z
Ω
u
2
a
2
< λ
Z
Ω
∇u : ∇u. (XIII.7 .15)
To this end for a fixed h > 0, we split R
3
+
into the following three regions (an
analogous splitting holds for R
3
−
):
R
1
=
x ∈ R
3
+
: x
3
> h, x
0
∈ S
R
2
=
x ∈ R
3
+
: x
3
< h, x
0
∈ S
R
3
= R
3
+
−
R
1
∪ R
2
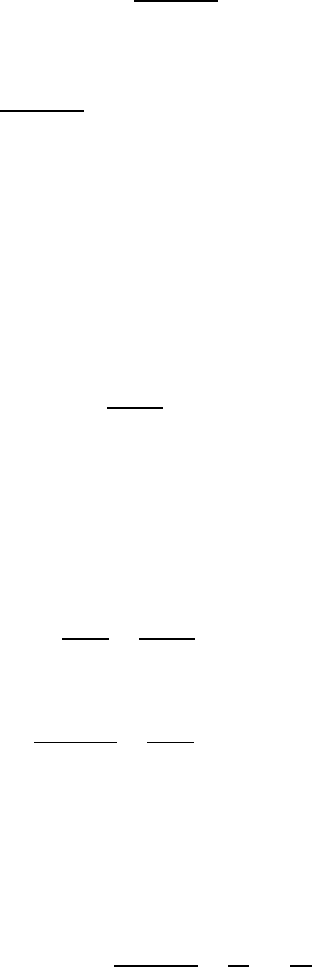
XIII.7 Aperture Domain: Existence and Uniqueness 941
and denote by
e
R
i
, i = 1, 2, 3, the intersection of R
i
with the support o f a.
By virtue of (XIII.7.11)
1
, (XIII.7.13), a nd the definition of b, we find
Z
e
R
i
u
2
a
2
≤ cε
Z
e
R
i
u
2
δ
2
(x)|x
0
|
2
, i = 1, 2, 3. (XIII.7.1 6)
with c independent of ε. We wish to show the inequali ty
Z
e
R
i
u
2
δ
2
(x)|x
0
|
2
≤ c
1
Z
Ω
∇u : ∇u, i = 1, 2, 3 (XIII. 7.17)
which, with the help of (XIII.7.16), implies
Z
R
3
+
u
2
a
2
≤ 3cc
1
ε
Z
Ω
∇u : ∇u.
Since an a nalog ous reasoning holds for R
3
−
, we conclude the validity of
(XIII.7.15) and complete the proof of the lemma. It remains to show the
inequality (XIII.7.17). To this end, we notice that, for functions u ∈ D(Ω)
the following estimate holds
Z
Ω
u
2
x
2
3
+ 1
≤ c
2
Z
Ω
∇u : ∇u, (XIII.7.18)
with c
2
= c
2
(S); cf. Exercise XIII.7.1. In view of (XIII.7.7), we have
|x
0
| ≥ d/2, for a ll x ∈
e
R
1
. (XIII.7.19)
Moreover, since
δ(x) ≥ x
3
≥ h for all x ∈
e
R
1
,
it follows that
1
δ
2
(x)
≤
c
3
x
2
3
+ 1
, for all x ∈
e
R
1
(XIII.7.20)
with c
3
= c
3
(h). Thus, from (XIII.7.18)–(XIII.7.20) we infer that
Z
e
R
1
u
2
δ
2
(x)|x
0
|
2
≤
4c
2
c
3
d
2
Z
Ω
∇u : ∇u. (XIII.7.21)
Let us next consider the region
e
R
3
. For all x ∈
e
R
3
,
δ(x) = x
3
|x
0
| ≥ d,
and so
Z
e
R
3
u
2
δ
2
(x)|x
0
|
2
≤
1
d
2
Z
e
R
3
u
2
x
2
3
.
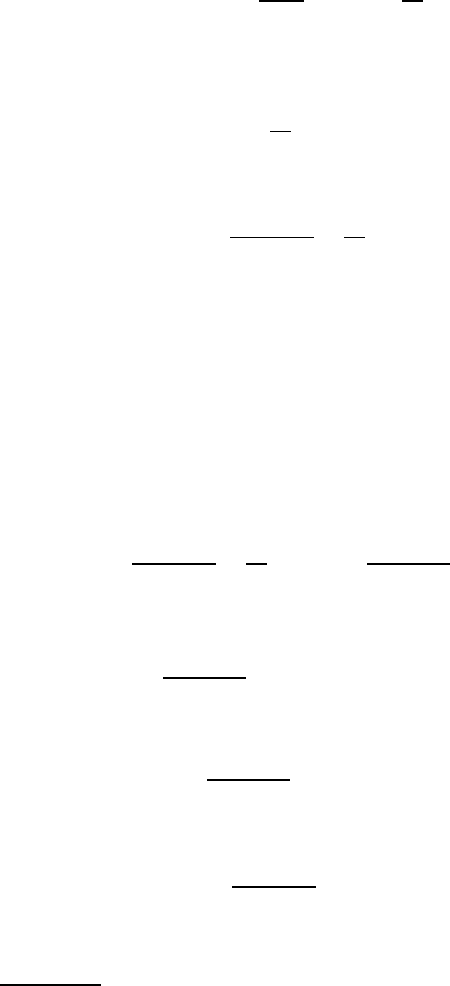
942 XIII Steady Navier–Stokes Flow in Domains with Unbounded Boundaries
However, using the elementary inequality
2
Z
∞
0
f
2
(t)
t
2
≤ 4
Z
∞
0
df
dt
2
, (XIII.7.22)
holdi ng for (sufficiently smooth) functions vanishing in a neighborho od of zero
and infinity, we at once obtain
Z
e
R
3
u
2
x
2
3
≤ 4
Z
Ω
∇u : ∇u
and, as a consequence,
Z
e
R
3
u
2
δ
2
(x)|x
0
|
2
≤
4
d
2
Z
Ω
∇u : ∇u. (XIII.7.23 )
It remains to estimate the integral over the regio n
e
R
2
. To this end, setting
δ
1
(y) = di st (y, ∂S) y ∈ S,
for a ll x ∈
e
R
2
we find that
δ
2
(x) = x
2
3
+ δ
2
1
(x
0
)
|x
0
| ≥ d/2
which, in turn furnishes
Z
e
R
2
u
2
(x)
δ
2
(x)|x
0
|
2
≤
4
d
2
Z
h
0
dx
3
Z
S
u
2
(x
0
, x
3
)
δ
2
1
(x
0
)
dx
0
. (XIII.7.24)
Since S is locally Lipschitz and u ∈ W
1,2
0
(S), we may use Lemma III.6.3 to
obtain
Z
S
u
2
(x
0
, x
3
)
δ
2
1
(x
0
)
dx
0
≤ c
Z
S
∇u : ∇udx
0
(XIII.7.25)
with c = c(S). Integrating (XIII.7.25) over x
3
∈ [0, h) delivers
Z
h
0
dx
3
Z
S
u
2
(x
0
, x
3
)
δ
2
1
(x
0
)
dx
0
≤ c
Z
Ω
∇u : ∇u. (XII I.7.26)
Using (XIII.7.26) in conjunction with (XIII.7.24) allows us to conclude
Z
e
R
2
u
2
(x)
δ
2
(x)|x
0
|
2
≤ c
9
Z
Ω
∇u : ∇u.
Estimate (XIII.7.17) then follows from this inequality, (XIII.7.21), and (XIII.7.23)
and therefore, by what we observed, the proof of the lemma i s complete. ut
2
For the (simple) demonstration of (XIII.7.22), see the proof of Lemma III. 6.3.

XIII.7 Aperture Domain: Existence and Uniqueness 943
Exercise XIII.7.1 Show inequality (X III.7.18), for all u ∈ D(Ω). Hint: Estab-
lish (XIII.7.18) for Ω a half-space. Then use a “cut-off” argument together with
inequality (II.5.18).
The result just shown permits us to obtain the following.
Theorem XIII.7.3 Given arbitrary Φ ∈ R, problem (XIII.5.2) admits at
least o ne corresponding generalized solution v. This solution obeys the energy
inequality:
|v|
2
1,2
≤ −
p
∗
Φ
ν
where p
∗
= p
+
−p
−
and p
±
are the constants associated by Lemma XIII.5.1
to the pressure field p corresponding to v.
Proof. We look for a solution of the form
v = u + Φa
where a = a(x; η) is the vector constructed i n Lemma XIII.7.1 corresponding
to a certain value of η that will be specified later, and u ∈ D
1,2
0
(Ω) obeys
ν(∇u, ∇ϕ) − (u ·∇ϕ, u) = −Φ(u ·∇a, ϕ) + Φ(a · ∇ϕ, u)
−Φ
2
(a · ∇a, ϕ) − νΦ(∇a, ∇ϕ).
(XIII.7.27)
It is clear that v satisfies all requirements of Definition XIII.5.1. As in anal-
ogous circumstances, a solution to (XIII.7.27) will be determined via the
Galerkin method. Let {ϕ
k
} ⊂ D(Ω) denote a sequence of functions whose
linear hull is dense in D
1,2
0
(Ω) and satisfying the properties listed in Lemma
VII.2.1. We set
u
m
=
m
X
k=1
ξ
km
ϕ
k
where the coefficients are required to sati sfy the foll owing system
ν(∇u
m
, ∇ϕ
k
) − (u
m
·∇ϕ
k
, u
m
) = −Φ(u
m
· ∇a, ϕ
k
) + Φ(a · ∇ϕ
k
, u
m
)
−Φ
2
(a · ∇a, ϕ
k
) − νΦ(∇a, ϕ
k
)
(XIII.7.28)
with k = 1, . . . , m. Existence to the algebraic system (XIII.7.28) for each
m ∈ N can be established exactly as in Theorem IX.3.1, provided we show a
suitable bound for |u
m
|
1,2
. To obtain such a bound, we multi ply (XIII.7.28 )
by ξ
km
and sum over the index k from one to m. Observing that
(u
m
·∇u
m
, u
m
) = (a · ∇u
m
, u
m
) = 0, (XIII.7.29)
we find
ν| u
m
|
2
1,2
= −Φ(u
m
·∇a, u
m
) −Φ
2
(a ·∇a, u
m
) −νΦ(∇a, ∇u
m
). (XIII.7.30)
944 XIII Steady Navier–Stokes Flow in Domains with Unbounded Boundaries
Using the Schwarz and Cauchy inequalities, we deduce for all η > 0
Φ
2
(a ·∇a, u
m
) = −Φ
2
(a · ∇u
m
, a) ≤
Φ
4
2η
kak
4
4
+
η
2
|u
m
|
2
1,2
−νΦ(∇ a, ∇u
m
) ≤
ν
2
Φ
2
2η
|a|
2
1,2
+
η
2
|u
m
|
2
1,2
.
(XIII.7.31)
Moreover, by Lemma XIII. 7.1,
−Φ(u
m
· ∇a, u
m
) ≤ η|Φ||u
m
|
2
1,2
. (XIII.7.32)
Thus, choosing
η <
ν
(1 + |Φ|)
,
and recalling that a ∈ L
4
(Ω) ∩ D
1,2
(Ω), we may use (XIII.7.29)–(XIII.7.32)
and Lemma IX.3.2 to show existence to (XIII.7.28) for all m ∈ N. Moreover,
we also find that
|u
m
|
2
1,2
≤ c
1
(Φ, ν). (XIII.7.33)
Employing (XIII.7.33) together with the weak compactness of the spaces
D
1,2
0
(Ω) we may select from {u
m
} a sequence, denoted again by {u
m
}, and a
vector field u ∈ D
1,2
0
(Ω) such that
u
m
w
→ u in D
1,2
0
(Ω)
u
m
→ u in L
2
(Ω
0
),
(XIII.7.34)
for any bounded dom ain Ω
0
⊂ Ω. By these convergence properties and taking
advantage of the properties of the functions {ϕ
k
}, we use a by-now-standard
procedure to show that u solves (XIII.7.28) and that, as a consequence, v is
a weak solution to (XIII.5.2). It remains to show that v satisfies the energy
inequality. For this purpose, setting
v
m
= u
m
+ Φa,
from (XIII.7.3 0), after a simple manipulation we find
ν| v
m
|
2
1,2
= −Φ(v
m
· ∇a, v
m
) + νΦ(∇a, ∇v
m
) (XIII.7.35)
where use has been made of the property
(v
m
· ∇a, a) = 0.
With the help o f the convergence conditi ons (XIII.7.34), it is easily shown
that
lim
m→∞
(v
m
· ∇a, v
m
) = (v · ∇a, v). (XIII.7.36)
Actually, we have
|(v
m
· ∇a, v
m
) − (v · ∇a, v)| ≤ |((v
m
−v) ·∇a, v
m
)|
+|(v · ∇a, (v
m
−v))|
≡ I
1
(m) + I
2
(m).
XIII.7 Aperture Domain: Existence and Uniqueness 945
Recalling that for R sufficiently large
|∇a(x)| ≤ c|x|
−3
, |x| > R, (XIII.7.37)
we find
I
1
(m) ≤
Z
Ω
|∇a|
4/3
|v
m
− v|
2
1/2
Z
Ω
|∇a|
2/3
v
2
m
1/2
≤ c
1
Z
Ω
v
2
m
|x|
2
1/2
Z
Ω
|∇a|
4/3
|v
m
− v|
2
1/2
.
Thus, from this inequality, (II.6. 10), and (XIII.7.33) it follows that
I
1
(m) ≤ c
2
Z
Ω
|∇a|
4/3
|v
m
−v|
2
1/2
(XIII.7.38)
with c
2
independent of m. Setting Ω
R
= Ω ∩ B
R
, Ω
R
= Ω − Ω
R
, from
(XIII.7.37) we have
Z
Ω
|∇a|
4/3
|v
m
−v|
2
≤
Z
Ω
R
|∇a|
4/3
|v
m
− v|
2
+
Z
Ω
R
|∇a|
4/3
|v
m
−v|
2
≤ c
3
Z
Ω
R
|v
m
−v|
2
+
c
4/3
R
2
Z
Ω
R
|v
m
− v|
2
|x|
2
and so, again by (II.6.10) and (XIII.7.33), we find
I
1
(m) ≤ c
4
Z
Ω
R
|v
m
− v|
2
+
c
5
R
2
with c
4
and c
5
independent of m. Taking the lim sup as m → ∞ at both sides
of this latter relation and bearing in mind (XIII.7.34)
2
, by the arbitrarity of
R we conclude that
lim sup
m→∞
I
1
(m) = 0,
that is,
lim
m→∞
I
1
(m) = 0.
Likewise, we show
lim
m→∞
I
2
(m) = 0
and (XIII.7.36 ) is completely established. Let us now take the lim inf as
m → ∞ of both sides of (XIII.7.35). Empl oying (XIII.7.36 ), (XIII.7.34)
1
,
and Theorem II.2.4 we obta in
ν| v|
2
1,2
≤ −Φ(v · ∇a, v) + νΦ(∇a, ∇v). (XIII.7.39)
