Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


8.3 PR08LEMS 223
8.18.
if
f(x)
=
g(x
+
a),
show that
j(k)
=
e-iakg(k).
8.19. For a > 0 find the Fourier transform
of
f(x)
=
e-
alxl.
Is
j(k)
symmetric?
Is it real? Verify the uncertaioty relations.
8.20. The displacement of a damped harmonic oscillator is given by
{
Ae- atei""'t
if t > 0
r» = 0 if 0'
1 t < .
Fiod jew) and show that the frequency distribution jj(w)1
2
is given by
- 2 A
2
I
If(w)1
=
-2
( )2 +
2'
7l'ltJ-lVO
a
convolution
theorem
8.21. Prove the convolution
theorem:
i:
f(x)g(y
-x)dx
=
i:
j(k)g(k)e
ikY
dk.
What will this give when y = O?
Parseval's
relation
8.22. Prove
Parseval's
relation
for Fourier transforms:
i:
f(x)g*(x)dx
=
i:
j(k)g*(k)dk.
In
particular, the norm
of
a
function-with
weightfunctionequalto
I-is
iovariant
under Fourier transform,
8.23. Use the completeness relation 1
=
La
In) (nl and sandwich it between [x)
and (x'] to find an expression for the Dirac delta function in terms of an iofinite
seriesof
orthonormal
functions.
8.24. Use a Fouriertransform io three dimensions to find a solution of the Poisson
equation: V
2<1>(r)
=
-4np(r).
8.25. For 9'(x) =
8(x
-
x'),
find q;(y).
8.26. Show that
jet)
=
f(
-t).
8.27. The Fouriertransform
of
a distribution 9' is given by
00
I
q;(t) =
I:
_8
'
(t - n).
n=on!
What is 9'(x)? Hiot: Use ql(x) = 9'(
-x)
8.28.
For
f
(x)
=
LZ~o
akxk,
show that
n
j(u)
=
y'2;I:
i
ka
k
8
(k)(u),
k~O
where

224 8.
FOURIER
ANALYSIS
Additional Reading
1. Courant, R. and Hilbert, D. Methods
of
Mathematical Physics, vol. I, In-
terscience, 1962.
The
classic
book
by two masters. This is a very readable
book
written specifically for physicists. Its treatment
of
Fourier series and
transforms
is very
clear.
2. DeVries, P.A First Course in Computational Physics, Wiley, 1994. A good
discnssion
of
the fast Fouriertransforminclnding someillnstrativecomputer
programs.
3. Reed, M.,
and
Simon', B. Fourier Analysis, Self-Adjointness, Academic
Press, 1980. Second volume
of
a four-volume series. A comprehensive ex-
position
of
Fourier analysis
with
emphasis on operator theory.
4. Richtmyer,
R. Principles
of
Advanced Mathematical Physics, Springer-
Verlag, 1978. A two-volume
book
on mathematical physics written in a
formal style, but very useful
due
to its comprehensiveness and the large
number
of
examples drawnfrom physics. Chapter4 discusses Fourieranal-
ysis
and
distributions.

Part III _
Complex Analysis

j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j
j

9 _
Complex Calculus
Complex analysis, just like real analysis, deals with questions
of
continuity, con-
vergence of series,
differentiation,
integration,
andso
forth.
The
reader
is
assumed
to have been exposed to the algebra of complex numbers.
9.1 ComplexFunctions
A complex function is a map f : C --> C, and we write
f(z)
= w, where both
z and w are complex numbers.' The map f
can
be geometrically thought of as a
correspondencebetweentwo complexplanes, the
z-plane and the w-plane. The w-
plane has a real axis and an imaginary axis, whichwe
can
call u and v,respectively.
Both
u and v are real functions
of
the coordinates
of
z, i.e., x and y. Therefore, we
may write
f(z)
=
u(x,
y) +
iv(x,
y).
(9.1)
This equation gives a unique point
(u, v) in the w-plane for each point (x,
y)
in the z-plane (see Figure 9.1). Under
f,
regions of the z-plane are mapped onto
regions of the w-plane.
For
instance, a curve in the z-plane may be mapped into a
curve in the w-plane. The following example illustrates this point.
9.1.1. Example. Letus
investigate
the
behavior
ofacoupleof
elementary
complex
func-
tions.
In
particular,
we shall look attheway a line y = mx in thez-plane is
mapped
into
curves
inthe
w-plane.
1
Strictly
speaking,
we shouldwritef :S -e- C whereS is a subsetof thecomplex
plane.
Thereasonis
that
most
functions
arenot
defined
forthe
entire
setofcomplex
numbers,
so
that
the
domain
ofsuch
functions
is not
necessarily
C.Weshallspecify
the
domain
onlywhenit is absolutely
necessary.
Otherwise,
we use thegeneric
notation
f :C -e- C, even
though
f is
defined
onlyonasubsetof C.

228 9.
COMPLEX
CALCULUS
f
z
(x,y) w
(u,v)
Figure 9.1 A map from the z-plane to the w-plane.
(a) For
w=
I(z)
=z2, we have
w = (x
+iy)2
=x
2
-
i
+2ixy,
with
u(x,
y) = x
2
- y2 and
v(x,
y) =
2xy.
For y =
mx,
i.e., for a line in the z-plane
withslopem, these
equations
yieldu =
(1-
m
2)x2
andv =
2mx
2.
Eliminating
x inthese
equations, we find v =
[2m(1
- m
2)]u.
This is a line passing through the origin of the
w-plane [see Figore 9.2(a)]. Note that the angle the image line makes with the real axis
ofthe
w-plane is twice the angle the original line makes with the x-axis. (Shnw this!). (b)
The
function
w =
fez)
= e
Z
= e
x
+
iy
gives
u(x,
y) = eXcos y andvex, y) = eXsiny.
Substituting
y=
mx,
we
obtain
u = eX
cosmx
andv =
ex
sinmx.
Unlike
part
(a), we
cannot
eliminate
x to
find
v as an explicit
function
of u,
Nevertheless,
the lastpairof
equations
areparametric equations of a
curve,
whichwe canplotin a
uv-plane
as
shown
inFigore9.2(b).
III
Limits of complex functions are defined in terms
of
absolute values. Thus,
lim
z
-+
a
f(z)
= wo means that given any real number f > 0, we can find a
corresponding real number 8
>
Osuchthatlf(z)-wol
< s whenever Izc-u] < 8.
Similarly, we say that a function
f is cnntinunus at z= a iflirn
z
-+
a
f(z)
=
f(a).
9.2 AnalyticFunctions
The derivative of a complex function is defined as usual:
9.2.1.Definitinn.
Let
f :
iC
-->
iC
be a complexfunction. The derivative
of
f at
zo
is
df
I = lim
f(zo
+
L>z)
-
f(zo)
,
dz Zo 6.z-+0 .6.z
provided that the limitexists
and
is independent
of
L>z.

9.2
ANALYTIC
FUNCTIONS
229
y
z2
a
-+
x
(aJ
y
e
Z
a
-+
x
(bJ
2a
u
2+4im
1
+im
Example
Illustrating
path
dependence
of
derivative
Figure9.2 (a)The
map
z2
takes
alinewithslopeanglea
and
maps
ittoalinewithtwice
theangle
in the
w-plane.
(b)Themape
Z
takes
thesameline
and
mapsitto a
spiral
inthe
w-plane.
In this definition "independent
of
/),.z" means independent
of
/),.X and /),.y (the
components
of
Az) and, therefore, independent
of
the direction
of
approach to
zoo
The restrictions
of
this definition apply to the real case as well. For instance, the
derivative
of
f(x)
= [x] at x = 0 does
not
exisr'
because it approaches +I from
the right
and
- I from the left.
It
can easilybe shownthatallthe formalrules
of
differentiationthat apply to the
real case also apply to the complexcase.
For
example,
if
f
and
g are differentiable,
then
f ± g, f g,
and-as
long as g is
not
zero-
f / g are also differentiable,
and
their derivatives are given by the usual rules
of
differentiation.
9.2.2,
Example.
Letus examinethe derivative of
fez)
= x
2
+
2ii
at z = I +i:
df
I = lim
f(1
+i +Ll.z)-
f(t
+i)
dz
z=l+i
6.z~O
..6.z
. (I +/),.x)2+
2i(t
+Ll.y)2 - t - 2i
= hm
.8.x--*O
Ax +
if:!..y
.6.y~O
= lim
2Ll.x+4iLl.y+(~x)2+2i(Ll.y)2.
8x~O
Ax
+
l.6..y
.6.y~O
Let us approachz = I +i along the line y - I =
m(x
- I). Then
Ll.y
= m
Sx,
and the
limityields
df
I = lim 2/),.x+4imLl.x +(/),.x)2 +
2im
2(Ll.x)2
dz
z=l+i
.6.x~O
Ax +im
Sx
20
ne
can
rephrase
thisandsaythatthe
derivative
exists.butnotin
terms
of
ordinary
functions,
rather,
interms of generalized
functions-in
this case 8(x)---discussedin Chapter6.
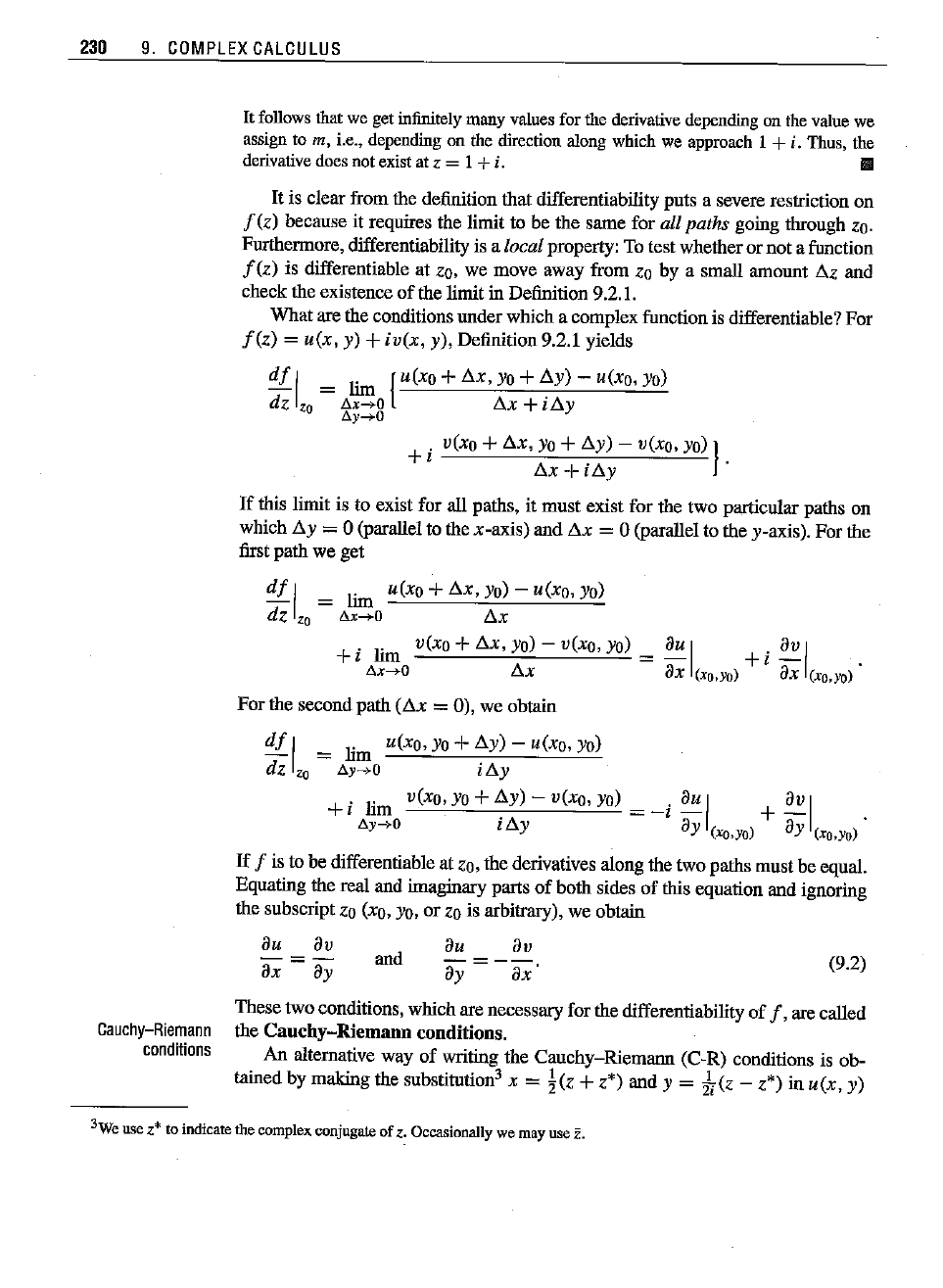
230
9.
COMPLEX
CALCULUS
It follows
that
we getinfinitely manyvaluesforthe
derivative
depending
on thevaluewe
assignto
m, i.e.,
depending
on the
direction
along which we
approach
1 +i, Thus,the
derivative
doesnotexistatz = 1+i, III
It
is clear from the definition that differentiability pnts a severe restriction on
fez)
because it requires the limit to be the saroe for all paths going through
zoo
Furthermore, differentiability is a local property: To test whetheror not a function
fez)
is differentiable at zo. we move away from zo by a small aroount
Az
and
check the existence
of
the limit in Definition 9.2.1.
What are the conditions under which a complex function is differentiable? For
fez)
= u(x, y) +
iv(x,
y), Definition 9.2.1 yields
df
I = lim
{u(x
o
+AX,
YO
+
Ay)
- u(xo,
YO)
dz
zo
8x-+O
Ax+iAy
dy~O
. v(xo + AX,
YO
+
Ay)
- v(xo, YO)}
+1 .
Ax
+
iAy
If
this limit is to exist for all paths, it must exist for the two particular paths on
which
Ay
=0 (parallel to the x-axis) and
Ax
=0 (parallel to the y-axis). For the
first path we get
df
I = lim u(xo +AX,
Yo)
- u(xo, Yo)
dz
zo
llx-+O
~x
.
Ii
v(xo +AX,
YO)
- v(xo,
YO)
au I .av I
+1 m = - +1 - .
L\x-+O !:i.x
ax
(xo,Yo)
ax
(xo,yo)
For the second path
(Ax
= 0), we obtain
df
I = lim u(xo,
Yo
+
Ay)
- u(xo,
YO)
dz
eo
8y-+O
i
Ay
. I' v(xo, Yo+
Ay)
- v(xo,
YO)
. au I av I
+1 rm
=-1
-
+-
8y-+O
iAy
ay
(xo,Yo)
ay
(XO,Yo)
.
If
f is to be differentiable at zo, the derivatives along the two paths must be equal.
Equating the real and imaginary parts
of
both sides of this equation and ignoriog
the subscript zo (xo, Yo,or zo is arbitrary), we obtain
au av
ax = ay
and
(9.2)
These two conditions, whichare necessary for the differentiability
of
f,
are called
Cauchy-Riemann
the
Cauchy-Riemann
conditions.
conditions
An alternative way of writing the Cauchy-Riemann (C-R) conditions is ob-
tained by making the substitution' x =
!(z
+z*) and y =
11
(z - z") in
u(x,
y)
3Weusez"to
indicate
thecomplex
conjugate
of z.Occasionally we mayuse
z.

9.2
ANALYTIC
FUNCTIONS
231
and
vex,
y),
using the chain rule to write
Equation
(9.2) in terms
of
z and
z",
substituting
the
results in !L =
~
+i
~
and
showing that Equation (9.2) is
az* az* az*
equivalent to
the
single equation
af/az*
=
O.
This
equation says that
9.2.3. Box.
Iff
is to be differentiable, it must be independent
of
z".
If
the derivative
of
f exists,
the
arguments leading to Equation (9.2) implythat
the derivative can be expressed as
Expression
forthe
derivative
ofa
differentiable
complex
function
df
au'
av av au
- =
-+i-=
--i-.
dz ax ax
ay ay
(9.3)
The
C-R
conditions assure us that these two equations are equivalent.
The
following example illustrates the differentiability
of
complex functions.
9.2.4. Example. Letus
determine
whether
ornotthefollowing
functions
are
differen-
tiable:
(a) Wehave alreadyestablishedthat
fez)
= x
2
+ 2i
y2
is not differentiableat z =
1+
t.
We can now show
that
it is has no
derivative
at any pointin
the
complex
plane
(ex-
cept at the
origin).
This is easily seen by noting
that
U = x
2
and v = 2y2, and
that
au/ax
= 2x
of.
av/ay
= 4y, and the firstCauchy-Riemann conditionis not satisfied. The
secondC-R
condition
is
satisfied,
but
that
is not
enough.
Wecan alsowrite
fez)
in terms of z andz":
fez)
=
G(Z
+z*)t
+2i
[;i
(z -
z*)t
=
1(1-
2i)(z2
+z*2)
+
!(l
+2i)zz*.
f (z) hasanexplicit
dependence
on
z".
Therefore,
it is not
differentiable.
(b) Nowconsider
fez)
=x
2
- y2 +
2ixy,
for which u = x
2
- i and v = 2xy. TheC-R
conditionsbecome
au/ax
=2x =
av/ay
and
au/ay
=
-2y
=
-av/ax.
Thus,
fez)
may
be
differentiable.
Recall
that
theC-R
conditions
areonlynecessary
conditions;
wehavenot
shown(botwe will, shortly)that they are also sufficient.
Tocheck the dependenceof
f on z", sobstitntex = (z +
z*)/2
and y = (z -
z*)/(2i)
in u andv to show
that
f (z) = z2,and
thus
there
is noz"
dependence.
(c) Let
u(x,
y) =eXcosy and vex, y) =eXsiny. Then
au/ax
=eXcosy =
av/ay
and
aulay
=
_ex
siny =-avlax,
and
theC-Rconditions are
satisfied.
Also,
fez)
= e" cosy +ie" siny =eX(cosy +i siny) =
e"e
ly
= e
x
+
iy
=e
Z,
and
there
is no z"
dependence.
The
requirement
of
differentiability is very restrictive:
The
derivative
must
exist along infiuitely
many
paths. On
the
other hand,
the
C-R
conditions
seem
deceptively mild:
They
are derived for
only
two paths. Nevertheless, the two paths
are, in fact, truerepresentatives
of
all paths; thatis, the
C-R
conditionsare
not
only
necessary,
but
also sufficient:

232 9.
COMPLEX
CALCULUS
9.2.5. Theorem. The function
f(z)
=
u(x,
y) +
iv(x,
y) is differentiable in a
region
of
the complex plane ifand only ifthe Cauchy-Riemann conditions,
. au av
=
ax ay
and
au av
=
ay ax
(or, equivalently,
af/az*
= 0), are satisfied and all first partial derivatives
of
u
and v are continuous in that region. In that case
df
au . av av .au
-=-+Z-=--z-.
dz ax ax ay ay
Proof
We have already shown the
"only
if"
part. To show the
"if"
part, note that
if
the derivative exists at all, it
must
equal (9.3). Thus, we have to show that
lim
f(z
+
M)
-
f(z)
= au +i av
..6..z--+o
llz
ax ax
or, equivaleutly, that
I
f (z + AZ) - f (z) _
(au
+iav)1
<f
Az ax ax
By
defiuition,
whenever
IAzl < 8.
f(z
+
Az)
-
f(z)
=
u(x
+AX, y +
Ay)
+
iv(x
+AX, y +
Ay)
-
u(x,
y) -
iv(x,
y).
Sinceu andv have
continuous
first
partial
derivatives,
we can
write
au au
u(x
+AX, y +Ay) =
u(x,
y) +ax AX + ay Ay +
flAx
+
81
Ay,
av av
v(x
+AX, y +
Ay)
=
v(x,
y) +
-Ax
+
-Ay
+
f2Ax
+82Ay,
ax ay
where
sj
, ez, 8J, and 82are realnumbersthatapproachzeroas Ax and Ay approach
zero.Usingtheseexpressions, we can
write
f(z
+
Az)
-
f(z)
= (aU +i
av)
AX +i
(-i
au +
av)
Ay
ax ax ay ay
+
(fl
+
if2)Ax
+
(81
+i82)Ay
(
au
av)
= ax
+i
ax
(Ax+iAy)+fAx+8Ay,
where f
==
er +iez. 8
==
81+i82, and we used the C-R conditions in the last step.
Dividing both sides by Az = Ax +iAy, we
get
f(z
+
Az)
-
f(z)
_ (aU +i
av)
=
fAX
+8
Ay.
Az ax ax Az Az
