Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations

Подождите немного. Документ загружается.

9.7

PROBLEMS

263

9.7 Problems

9.1. Show that the function w =

l/z

maps the straight line y = a in the z-plane

onto a circle in the w-plane with radius

l/(2Ial)

and center (0,

l/(2a)).

9.2. (a) Using the chainmle,find

af/az*

and

af/az

in terms of partial derivatives

with respect to

x and y.

(b) Evaluate

af/az*

and

af/az

assuming that the Cauchy-Riemann conditions

hold.

9.3. Show that when z is represented by polar coordinates, the derivative

function

f(z)

can be written as

=e-

(au

+iav),

aT aT

where U and V are the real and imaginary parts of

f(z)

written in polar coor-

dinates. What are the C-R conditions in polar coordinates? Hint: Start with the

C-R conditions in Cartesian coordinates and apply the chain IDleto them using

x =

TCOSO

and y =T sinO.

9.4. Show that

d/dz(lnz)

liz.

Hint: Find

u(x,

y) and

v(x,

y) for ln z and

differentiate them.

9.5. Show that sin z and cos z have only real roots.

9.6. Show that

(a) the sum and the prodnct

two entire functions are entire, and

(b) the ratio of two entire functions is analytic everywhere except at the zeros of

the

denominator.

9,7. Given that u =2Aln[(X

+yZ)I/Z], show that v =2A

tan"!

(yf

x),

where u

and v are the real and imaginary parts of an analytic function

w(z).

9.8.

w(z)

is any complex potential, show that its (complex) derivative gives the

components of the electric field.

9.9. (a) Show that the flnx through an element

area da

the lateral surface

of a cylinder (with arbitrary cross section) is

d¢

= dz(IEI

ds)

where

is an arc

length along the equipotential surface.

(b) Prove that lEI

Idw/dzl

av/as

where v is the imaginary part

the com-

plex potential, and

s is the parameter describing the length along the equipotential

curves.

flux per unit z-length

_¢-

v(Pz)

V(PI)

- Zl

for any two points PI and Pz on the cross-sectional curve

the lateral surface.

Conclude that the total flux

per

unit z-length through a cylinder (with arbitrary

264 9.

COMPLEX

CALCULUS

cross section) is

[v],

the total change in v as one goes around the curve.

(d) Using Gauss's law, show that the capacitance per unit length for the capacitor

consisting of the two conductors with potentials

and

charge per unit length _

[v]j4rr

potential difference -

IU2

Uti'

9.10. Using Equation (9.7)

(a) find the equipotential curves (curves

constant u) and curves

constant v

for two line charges of equal magnitude and opposite signs located at y = a and

y =

-a

in the xy-plane.

(b) Show that

z=a(sin;),.

+iSinh;Jj(COSh;),.

-cos

2:)

by solving Equation (9.7) for z and simplifying.

sinh(uj2)") with centers at (0, a coth(uj2)")), and that the curves of constant v

are circles

radii

sin(vj2)") with centers at (a cot(vj2)"), 0).

9.11.

this problem, you will find the capacitance per unit length of two cylin-

dtical conductors of radii Rt and R2 the distance between whose centers is D by

looking for two line charge densities +),. and -),. such that the two cylinders are

two

the equipotential surfaces. FromProblem 9.10, we have

Ri = sinh(u;j2),.) ' Yi =a coth(u;j2)"),

i =

1,2,

where Yjand

are the locations of the centers

the two conductors on the y-axis

(which we assume to connect the two centers).

U21

(a) Show that D = IYt -

Y21

cosh 2)" - R2cosh

2)"

(b) Square both sides and use

cosh(a

-b)

= cosh a

coshb

sinha

sinhb

and the

expressions for the

R's

and the

y's

given above to obtain

(

Ut - U2)

IRr+R~-D21

cosh = .

2)"

2RtR2

concenttic cylinders.

(d) Find the capacitance per unit length

a cylinder and a plane, by letting one

the radii, say

Rj,

go to infinity while h

Rj - D remains fixed.

9.7

PROBLEMS

265

9.12. Use Equations (9.4) and (9.5) to establish the following identities.

(a) Re(sinz) = sin x

coshy,

(b) Re(cosz) =

cosxcoshy,

cosy,

(d) Re(coshz) = cosh x

cosy,

(e) I

sind

= sin

x + sinh

(f)

Istnh z]? = sinh

x + sin

9.13. Find all the zeros of sinh z and cosh z.

9.14. Verify the following hyperbolic identities.

Im(sinz)

= cos x sinh y.

Im(cosz)

= -

sinx

sinhy.

Im(sinhz)

= cosh x

siny.

Im(coshz) =

sinhxsiny.

1coszl

= cos

x + sinh

1coshzl

= sinh

x + cos

(a) cosh

Z - sinh

Z = 1.

(b)

COSh(ZI

Z2)

COShZl

cosh

+ sinh zj sinh

zj.

COShZ2

COShZl

sinh z-.

(d)

cosh2z

=cosh

z + sinh

sinh2z

= 2 sinh zcosh z,

taoh

Zj + tanh Z2

(e) tanhfzj +Z2) = 1+tanh zj

taohZ2'

9.15. Show that

(a)

taoh(!:)

sinhx+isiny

2 cosh x + cos y

9.16. Find all values of z such that

(

sinhx-isiny

(b) coth - = .

coshx

- cos Y

(a) e

-3.

(b) e

= 1+

i./3.

= 1.

9.17. Show that le-zi < 1

and only

Re(z) >

9.18. Show that both the real and the imaginary parts of an aoaiytic function are

harmonic.

9.19. Show that each of the following functions-s-call each one

u(x,

y)-is

har-

monic, and find the function's harmonic partner,

v(x,

y),

such that

u(x,

y) +

iv(x, y) is analytic.

(a)x

3xi.

(b)

cosy.

2 2 2

(d)e-

Ycos2x. (e) e

-X

cos2xy.

(I)

eX(x

cosy

- y

siny)

+ 2 sinh y sin x + x

3xi

+ y.

266

COMPLEX

CALCULUS

9.20. Prove the following identities.

(u)

cos-

Z =

-i

In(z ± J

I).

(c)

tan-

z =

---,

- .

,+z

(e)

sinh-

z = In(z ± J

I).

(b)

sin-

z =

-i

In[iz ±

~)].

(d)

cosh-

z = In(z ±

~).

(f)

tanh-I

Z).

I-z

9.21. Find the curve defined by each of the following equations.

(u) z = I -

it,

0::; t ::; 2.

3:n:

=u(cost+isint),

"2::;

t::;

(b) z = t +

-00

< t <

00.

(d)

z = t +-,

-00

< t <

9.22. Provide the details of the

proof

of part (a) of Proposition 9.3.1. Prove part

cf>

(b) by showing that

f(z)

= z' =

iy'

is analytic and

-2

=0, then

ax ay

cf>

+--0

axil

ay'2 - .

9.23. Let

f(t)

u(t)

iv(t)

be a (piecewise) continuous complex-valued

function of a real variable

t defined in the interval a

::;

t ::; b. Show that

F(t)

U(t)

V(t)

is a function such that

[dt

f(t),

then

f(t)

= F(b) -

F(u).

This is the fundamental theorem of calculus for complex variables.

9.24. Find the value of the integral

Icr(z +2)/z]dz, where C is (a) the semicircle

z = 2e

for 0 ::; e

::;

; (b) the semicircle z = 2e'&,for:n: ::; e

::;

2:n:,

and (c)

thecirclez = 2e

(),

for

-]i

.:5

Jr.

9.25. Evaluatethe integra1I

dz/(z-I-i)

where y is (a)the line joining

= 2i

and

= 3, and (b) the broken path from

to the origin and from there to

Z2.

9.26. Evaluate the integral

zm(z*)ndz, where m and nare integers and C is the

circle

[z]

= I taken counterclockwise.

9.27. Let C be the boundary

the square with vertices at the points z =0, z =I,

z =I +i, and z =i with counterclockwise direction. Evaluate

(5z

+2)dz

and

9.7

PROBLEMS

267

9.28. Let Cl be a simple closed contour. Deform Cl into

anew

contourC2io such

a way that C

1 does not encounter aoy singularity of ao aoalytic function f io the

process. Show that

f(z)dz

= J

f(z)dz.

Tel

That is, the contour cao always be deformed iota simpler shapes (such as a circle)

aod the iotegral evaluated.

9.29. Use the result of the previous problem to show that

dz.

2,,;

aod J (z - I -

i)m-1dz

= 0 for m =

±I,

±2,

...

fCZ-

when C is the boundary of a square with vertices at z =0, z =2, z = 2 +2;, aod

z = 2;, taken counterclockwise.

9.30. Use Equation (9.12) aod the bioomial expaosion to show that

dz (z'") =

mzm-l.

9.31. Evaluate

'.fc

dZ/(Z2

- 1) where C is the circle lzl = 3 iotegrated io the

positive sense. Hint: Deform C iota a contour

that bypasses the singularities

the iotegraod.

9.32. Show that when f is aoalytic witbio aod on a simple closed contour C aod

is noton C, then

f'(z)

dz = J

f(z)

dz .

z -

(z- ZO)2

9.33.

Let

C be the boundary

a square whose sides lie along the lioes x =

±3

aod y =

±3.

For the positive sense

integration, evaluate each of the following

integrals.

J e

J cosz d

(b)

Z(Z2

+10) dz: (e) f

(z _

~)(Z2

-10)

coshz icosz

(e)

-4-

dz.

(f)

-3

dz:

c z c z

eZ i cosz

(h) dz:

(i)

--.-

dz:

c (z -

;,,)2

C Z +

(k) J

sinhz

dz: (I) J

eoshz

dz;

(z - ;,,/2)2 f

(z - ;,,/2)2

for - 3 < a < 3. (n) J Z2 dz:

- 2)(Z2 - 10)

(a)

dz:

z - i,,/2

sinhz

(d)

-4-dz.

c z

cosz d

(g)

c (z - ;,,/2)2 .

(j)

J 2 e

dz:

-5z

taoz

(m) dz

c (z -

a)2

268 9.

COMPLEX

CALCULUS

9.34.

Let

C be the circle lz- i1 = 3 integrated in the positive sense. Find the

valne of each

the following integrals.

(a)

+rr

•

(d)

:rc

(z2 +9)2 .

sinhz

(b)

(Z2

+rr

j2 dz,

coshz

(e) Y

(Z2

+rr

dz:

(c)

:rc

(f)

- 3z +4 dz,

c z

2 - 4z + 3

9.35. Show that Legendre polynomials (for [x] <

can be represented as

(_I)n

(1-

z2)n

Pn(x) = +1 dz;

n(2rri)

c (z -

x)"

where C is the unit circle around the origin.

9.36.

Let

I be analytic within and on the circle

given by [z - zol =

and

integratedin the positive sense. Show that

Cauehy's

inequalityholds:

nlM

I(n)

(zo)1 ::::

where M is the maximum value

I/(z)1 on

Yo.

9.37. Expand

sinhz

in a Taylor series about the point z =

9.38. What is the largest circle within which the Maclaurin series for tanh Z con-

verges to

tanhz?

9.39. Find the (unique) Laurentexpansionofeach of the following functions about

the origin for its entire region

analyticity.

(d)

sinhz-z

(h) (Z2

1)2'

(e)

--'z2'--(-'-I---z-'--).

-4

(g) ---Z--9'

z -

(f)

---Z--I'

z -

(a) .

(z - 2)(z - 3)

(e) (I _

z)3'

(i)

_z_.

z-I

9.40. Show that the following functions are entire.

{

_ 1 2

(a)

I(z)

-----;:-

- z for Z

2 for z

{

(b)

I(z)

= I z

for z

for z = O.

{

cosz

(e)

I(z)

- rr

-I/rr

for z

±rr/2,

for z = ±rr/2.

9.7

PROBLEMS

269

9.41.

Let

I be analytic at zo and I (zo) =

(zo) = ... =

I(k)

(eo) =

Show

that the following function is analytic at zo:

I(z)

(z - ZO)k+l

g(z) =

flk+ll(zo)

(k +I)!

for z

zo.

for z =

zoo

9.42. Obtain the first few nonzero terms

the Laurent series expansion of each

of the following functions about the origin. Also find the integral of the function

along a small simple closed contour encircling the origin.

(a)

-.-.

smz

(e) 3 .

6z+z

-6sinhz

(b) I

cosz

(f)

-2-'-'

Z smz

I-coshz

(g) e

(d)

--,--

z - sinz

Additional Reading

I. Churchill, R. and Verhey, R. Complex Variables

and

Applications, 3rd ed.,

McGraw-Hill, 1974. An introductory text on complex variables with many

examples

and

exercises.

2. Lang, S. Complex Analysis, 2nd ed., Springer-Verlag, 1985. An extremely

well-written book by a master expositor. Although the book has a formal

tone, the clarity of exposition and the use of many examples make this book

very readable.

10 _

Calculus

Residues

One of the mostpowerfultools made available by complex analysis is the theory

residues, which makes possible the routine evaluation

certain definite integrals

that are impossibleto calculateotherwise.The derivation, application, and analysis

of this tool constitute the main focus

this chapter.

the preceding chapter we

saw examples in which integrals were related to expansion coefficients of Laurent

series. Here we will develop a systematicway of evaluating both real and complex

integrals.

10.1

Residues

Recall that a singular point

f :

--*

is a point at which f fails to be

analytic.

in addition, there is some neighborhood

zo in which f is analytic

at every point (except

course at zo itself), then zo is called an isolatedsingu-

isolated

singuiarity

Iarity

Almost all the singularities we have encountered so far have been

isolated singularities. However, we will see

later-when

discussing multivalued

functions-that

singularities that are not isolated do exist.

Let

be an isolated singularity

Then there exists an r > 0 such that

within the "annular" region 0 < [z - zoI < r, the function f has the Laurent

expansion

)

( n

Z =

L-

anz-zo)

=L-an(Z-ZO)

+--+

)2+'"

n=-oo

n=O Z - zo (z - zo

where

1 J

f(~)d~

an = 2".;

- zo)n+l

and

(10.1)

10.1

RESIDUES

271

In particular,

hi = 2

f(~)

d~,

7T:Z

where C is any simple closed contour around zo. traversed in the positive sense,

on and interior to which f is analytic except at the point

itself. The complex

number

hj,

which is essentially the integral

f(z)

along the contour, is called

residue

defined

the

residue

of f at the isolated singular point zo.

is important to note that the

residue is independent of the contour C as long as

is the only isolated singular

pointwithin C.

Pierre AlphonseLaurent (1813-1854) gradoated fromtheEcole

Po1ytechniqoe

nearthe

top

his class

and

became

asecond

lieutenant

inthe

engineering

corps.

Onhis

return

from

the warin Algeria, he took

part

in the effortto

improve

the portat Le

Havre,

spending

six

years

there

directing

various

parts

of theproject

Laurent's

superior

officers

admired

the

breadth

of his

practical

experience

andthe good

judgment

afforded

the

young

en-

gineer.

During

this

period

wrote

his

first

scientific

paper,

onthe

calculus

variations.

and

submitted

it to the

French

Academy

of Sciences for the

grand

prixin

mathematics.

Unfortunately thecompetition had

already

closed(allbough thejudgeshadnotyetdeclared

winner),

and

Laurent's

submission

wasnotsuccessful.

However,

the

paper

impressed

Cauchy

that

recommended

its

publication,

also

without

success.

Thepaperfor which

Laurentis

mostwell

known

suffered

asimilarfate. Inithe

described

general

formofa

theorem

earlier

proven

Cauchy

for the

power

series

expansion

function.

Laurentrealized

that

onecould

generalize

this

result

to holdin

any

annularregion

between

two

singular

discontinuous

points

by usingbothpositive and

negative

powers

inthe

series,

thus

allowing

treatment

regions

beyond

the

first

singular

discontinuous

point.

Again,

Cauchy

argued

for the

paper's

publication

without

success. The

passage

oftime

provided

just

reward,

however,

andtheuseof

Laurent

series

became

fundamental

toolin

complex

analysis.

Laurent

later

workedinthe

theory

oflight

waves

and

contended

with

Cauchy

overthe

interpretation

ofthe

differential

equations

the

latter

had

fonnulated

explain

the

behavior

light.

Little

came

ofhis

work

inthis

area.

however,

and

Laurent

diedattheageof

forty-

two,_a

captain

serving

onthe

committee

fortifications

Paris.

His widow

pressed

havetwo

of his

papers

read

tothe

Academy,

onlyoneofwhichwas

published.

We use the notation

Res[f(zo)]

to denote the residue of f at the isolated

singular point

zoo

Equation (10.1) can then be written as

f(z)

dz =

2ni

Res[f(zo)]·

What

there are several isolated singular points within the simple closed

contour C? The following theorem provides the answer.

residue

theorem

10.1.1.

Theorem.

(the residue theorem) Let C be a positively oriented simple

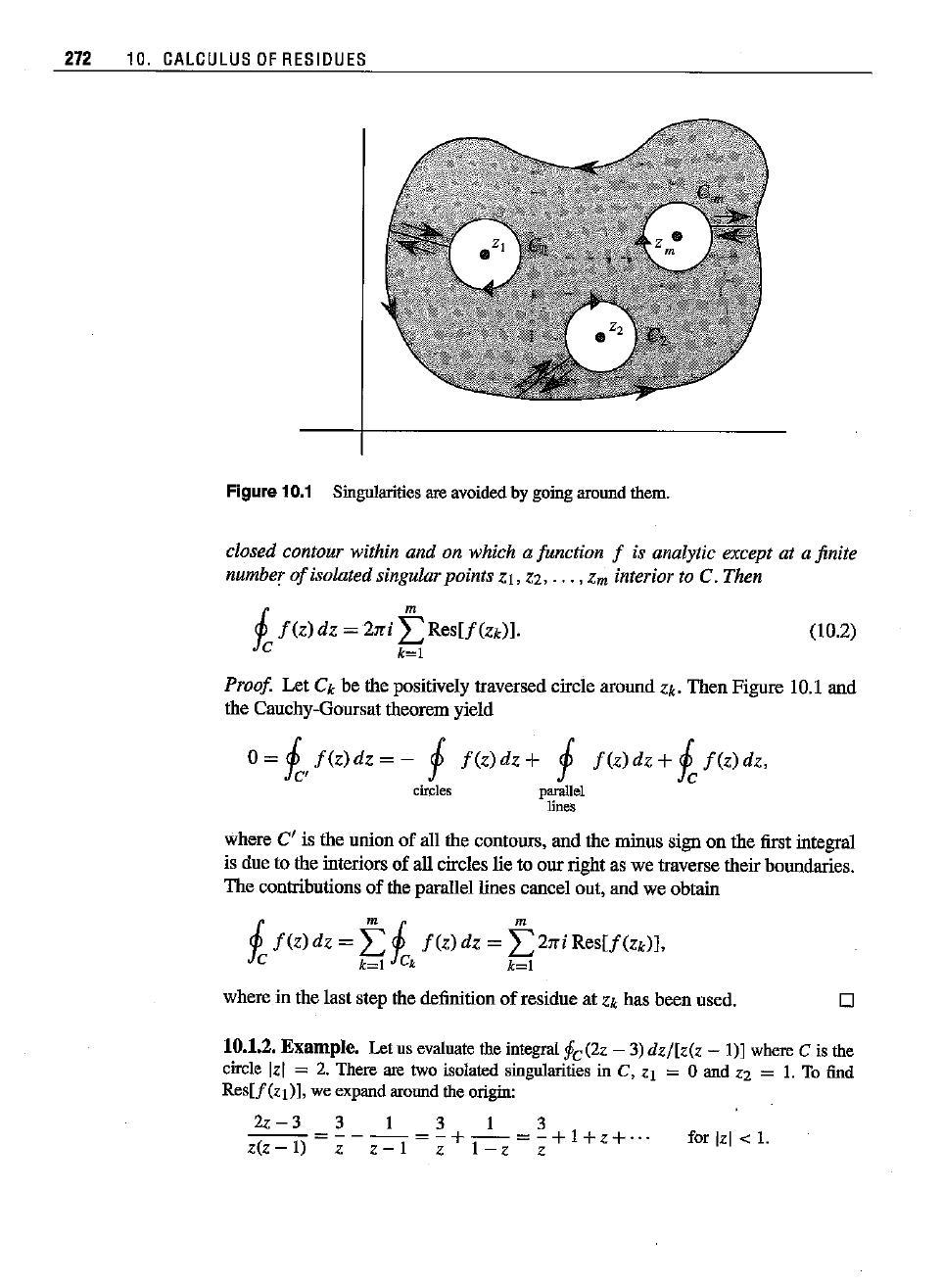
(10.2)

272 10.

CALCULUS

RESIDUES

Figure 10.1 Singularities

are

avoidedby goingaroundthem.

closed contour within and on which a function f is analytic except at a finite

number

isolated singularpoints Zl ,

Z2,

...

interior to C. Then

f(z)dz=2,..itReS[f(Zk)].

fc k=1

Proof

Let

Ck be the positively traversed circle around Zk.

Then

Figure 10.1

and

the Cauchy-Goursat theorem yield

!cJ(Z)dZ

= - f

f(z)dz

+ f

f(z)dz

+!c

f(z)dz,

circles

parallel

lines

where

is the union

all the contours,

and

the minus sign on the first integral

is due to the interiors

all circles lie to

our

right as we traverse their boundaries.

The

contributious

theparallel lines cancel out,

and

we obtain

f(z)

dz = t i

f(z)

= t 2,..i Res[f(Zk)],

k=l

where in thelast step thedefinition

residue at

has been used.

10.1.2.

Example.

Letus evaluatethe integral:fc(2z - 3)dz/[z(z - 1)] where C is the

circle

Izi

= 2. There

are

two isolated singularitiesin C, Zl = 0 and

= I. To find

Res[f(ZI)l. we expandaroundthe origin:

2z-3

3 I 3 I 3

---

= - -

= - +

= - +1+z+... for

[z]

z(z - I) z z- I z 1 - z z