Hatano Y., Katsumura Y., Mozumder A. (Eds.) Charged Particle and Photon Interactions with Matter - Recent Advances, Applications, and Interfaces

Подождите немного. Документ загружается.

40 Charged Particle and Photon Interactions with Matter

InFigure 3.9, the DCSs for elastic scattering of electrons are presented for 3-hydroxytetrahydro-

furan (3-hTHF) (Vizcaino etal., 2008) compared to the DCS for tetrahydrofuran (THF) (Colyer

etal., 2007). In this gure, DCS measured with a crossed-beam method are compared with a

theoretical calculation. These two molecules have a similar structure and are common analogues

to the deoxyribose sugar component in DNA. The experimental results show no essential differ-

ence between the elastic-scattering cross sections for 3-hTHF and THF. This is in contrast with

the dissociative attachment experiment, where a large difference is found in the two molecules.

3.3.3.2 measurements

of d

over the Full r

ange

of s

cattering

ngles

The electron-beam method has a limitation of scattering angles covered for the following three

reasons. First, it is difcult to extend measurements to backward scattering, usually beyond a

maximum scattering angle of about 160°, because of the geometrical restriction of the electron

analyzer. Second, it is difcult to distinguish the electrons elastically scattered at 0° from the

unscattered incident electrons. Finally, good angular and energy resolutions require a minimiza-

tion of magnetic elds present, such as the Earth’s magnetic eld and the elds due to the residual

magnetization of electron-analyzer materials. The magnetic eld, if any, inuences electrons of

low kinetic energies.

To overcome these difculties, an innovation has been proposed and implemented (Read and

Channing, 1996). The basic idea is to introduce into a collision region a suitably controllable mag-

netic eld generated by several electromagnetic coils. The eld guides those electrons scattered

at inaccessible forward- and backward-scattering angles to a direction acceptable to the analyzer.

Acombination of the coils is designed so that the magnetic eld does not leak outside the collision

region.

This technique is called a magnetic angle changer (MAC).

3-hTHF present data

6.5 eV elastic

THF data

3-hTHF Schwinger calc.

THF Schwinger calc.

0 40 80

Scattering angle(deg.)

DCS (10

–16

/sr)

120 160

Figure 3.9 DCS for elastic electron scattering at 6.5 eV. The experimental data are shown for 3-hTHF

(lled circles) compared with those for THF (open circles). The lines are the corresponding theoretical values.

(Reprinted

from Vizcaino, V. etal., New J. Phys., 10, 053002-1, 2008. With permission.)

Electron Collisions with Molecules in the Gas Phase 41

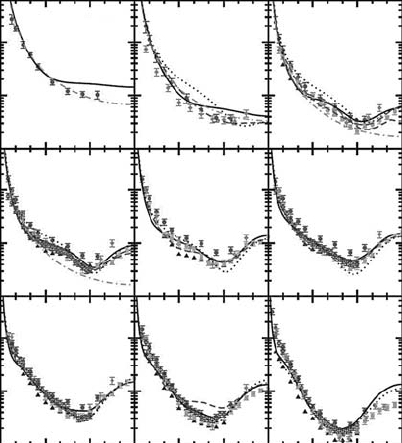
Cho etal. (2004) measured an absolute value of DCS for elastic scattering by H

O at incident

electron energies between 5 and 40eV. They obtained the DCS over the scattering angles from

10° to 180°, using an MAC. Extrapolating their DCS in the forward directions, Cho etal. obtained

an integral elastic cross section (ICS). The resulting values, however, are very small at electron

energies below about 20 eV, compared with theoretical results. (See the discussion by Itikawa and

Mason, 2005.) In 2008, Khakoo etal. (2008) reported their measurement. They obtained the elastic

DCS for H

O at incident energies of 1–100eV for scattering angles from 5° to 130° (Figure 3.10).

To obtain integral cross sections, they extrapolated their DCS in the following manner. Due to the

strong dipole moment of water molecules, the electrons are dominantly scattered in the forward

direction. Therefore, the DCS, particularly at low incident energies, should have a very sharp peak

in the vicinity of 0°. Khakoo etal. carefully considered this fact in their extrapolation in the small-

angle region. In the backward direction, they referred to the measurement by Cho etal. The result-

ing integral cross section of Khakoo etal. becomes larger than that of Cho etal. and consistent with

the

theoretical value (on which the recommended value in Figure 3.3 is based) (Figure 3.11).

Cho

etal. (2008) measured the elastic DCS in the backward directions also for CH

. In Figure 3.12,

the DCS at 5eV for CH

are presented for the full angular range. The experimental data are compared

with theoretical values. It is noted that in the backward direction, theory cannot reproduce the experi-

mental result at all. From the denition (3.4), momentum-transfer cross sections are much affected by

large-angle scattering. The DCS measurement in the backward direction, such as that shown in Figure

3.12, is critical to evaluate accurate values of momentum-transfer cross sections.

3.3.3.3 e

lectron s

pectroscopy

at h

igh

esolution

Once properly normalized, the electron energy-loss spectra easily enable the evaluation of DCSs for

any collision process. To assign each energy-loss peak to a specic elastic or inelastic process, the

energy resolution of the electron beam should be sufciently high. A polyatomic molecule normally

has complicated energy levels of molecular rotation and vibration. In the analysis of the energy-loss

0.1

0 60

6 eV 8 eV 10 eV

15 eV 20 eV 30 eV

1 eV 2 eV 4 eV

120 180 60 120

Scattering angle(deg.)

Cross section (10

–16

/sr)

180 60 120 180

Figure 3.10 Elastic electron scattering DCSs for H

O at various impact energies. The lled circles are

those measured by Khakoo etal. Other details are given in Khakoo etal. (2008). (Reprinted from Khakoo, M.A.

etal.,

Phys. Rev. A, 78, 052710-1, 2008. With permission.)

42 Charged Particle and Photon Interactions with Matter

spectra of molecules, a high-resolution experiment is intrinsically needed. In the following text,

excitations

of rotational, vibrational, and electronic states are discussed separately.

3.3.3.3.1

Deduction of Rotational Excitation Cross Section

When an electron approaches a molecule, it feels an electric force induced by a nonspheri-

cal charge distribution of the molecule. When the electron is located far from the molecule,

for instance, the electron–molecule interaction is represented by the interaction between the

electron and the electric multipole (i.e., dipole, quadrupole,…) moments of the molecule. These

0.1

0 30 60

Scattering angle(deg.)

DCS (10

–16

/sr)

90 120 150 180

Figure 3.12 DCS for elastic electron scattering from CH

at 5eV. Filled circles are the data measured by

Cho etal. Lines are the corresponding theoretical results. See Cho etal. (2008). (Reprinted from Cho, H. etal.,

J. Phys. B: At. Mol. Opt. Phys.,

41, 45203-1, 2008. With permission.)

100

1 10 100

Electron energy (eV)

Cross section(10

–16

)

Figure 3.11 ICS and TCSs for H

O. Filled circles with error bars are those derived by Khakoo et al.

Inverted triangles are the TCSs recommended by Itikawa and Mason. For further details, see Khakoo

etal. (2008).

(Reprinted from Khakoo, M.A. etal., Phys. Rev. A, 78, 052710-1, 2008. With permission.)

Electron Collisions with Molecules in the Gas Phase 43

moments include an electrostatic moment (due to initial molecular charge distribution) and

an induced moment (due to polarization by the approaching electron). Through nonspherical

interaction, the electron exerts a torque on the molecule, leading to rotational excitation (or de-

excitation). Rotational excitation occurs over a wide range of collision energy. Unfortunately,

the electron-beam method at present is hardly capable of resolving individual energy levels of

rotation (except for hydrogen and hydride molecules). Measurements so far have dealt with an

envelope of a rotational structure in an energy-loss spectrum, which is analyzed numerically

for gross information with the deconvolution procedure (Jung et al., 1982). Thus, in almost

all cases, only theoretical calculations produce rotational cross sections. The theoretical cross

sections for H

O obtained by Tennyson’s group are shown in Figure 3.13 (Faure etal., 2004).

Acomparison of rotational excitations for H

, N

, HCl, H

O, and CH

are presented in Figure

3.14 (Itikawa, 2007).

3.3.3.3.2

Resonant Vibrational Excitation

An electron approaching a molecule exerts also a force causing changes in internuclear distances

due to the deformation of the electron charge density in the molecule. This leads to vibrational

excitation of the molecule. In the CH

molecule, which is tetrahedral, there are four normal

modes of vibration with frequencies of ν

, ν

, and ν

, whose excitation energies are 0.362,

0.190, 0.374, and 0.162 eV, respectively. Normally, it is difcult to separate ν

and ν

modes and

and ν

modes in the energy-loss measurement (however, see below). Figure 3.15 (C

urík etal.,

2008) shows the combined cross sections for the excitations of the ν

and ν

modes (designated

as ν

+ ν

) and ν

and ν

modes (as ν

+ ν

). A resonance feature emerges in the vibrational cross

section at around 7 eV, as shown in Figure 3.15 (C

urík etal., 2008). This is the so-called shape

resonance, which is thought of as a temporary bound state of an electron in the molecule. The

bound state is formed by an effective potential well. This well might be, for instance, due to the

combination of the (repulsive) centrifugal force for a certain orbital angular momentum and an

(attractive) molecular potential. The temporary bound state may be viewed as an excited state

of a negative ion, that is, the system of the neutral molecule plus the incoming electron. If the

–1

0.001 0.01

0–3

0–2

0–0

0–1

e+H

O rot (theory)

0.1 1

Electron energy (eV)

Cross section (10

–16

)

Figure 3.13 Rotational excitation cross sections for H

O, calculated by Tennyson’s group (Faure etal.

2004). (Reprinted from Itikawa, Y. and Mason, N.J., J. Phys. Chem. Ref. Data, 34, 1, 2005. With permission.)

44 Charged Particle and Photon Interactions with Matter

temporary bound state is sufciently stable against autodetachment, and has a lifetime much

longer than the period of a molecular vibration, then the nuclei experience forces different from

those in the original molecule. This causes a conversion of a part of the electron’s energy to the

nuclear vibrational motion. This resonant mechanism of vibrational excitation can sometimes

enhance the corresponding cross section by orders of magnitude over the nonresonant case.

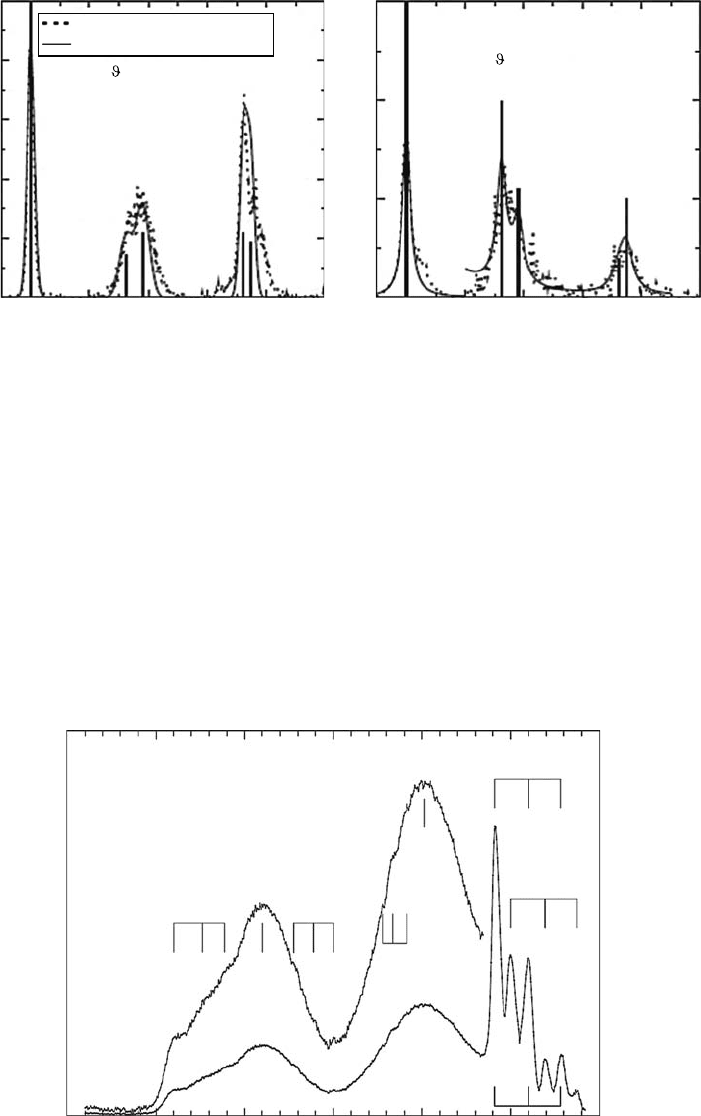
Finally, Figure 3.16 shows the energy-loss spectra in which individual vibrational modes are

partially resolved. It is noted that a theory conrms the relative magnitudes of the vibrational

cross sections.

–1

–2

0.001 0.01 0.1

0–2

O 0–1

0–2

Rotational cross section

HCl 0–1

0–3

1 10

Electron energy (eV)

Cross section (10

–16

)

100

Figure 3.14 Comparison of rotational cross sections for H

, N

, HCl, H

O, and CH

. Theoretical values for

the lowest transitions from the ground state are shown. (Reprinted from Itikawa, Y., Molecular Processes in

Plasmas: Collisions of Charged Particles with Molecules, Springer, Berlin, Germany, 2007. With permission.)

0.1

0.08

0.06

0.04

0.02

+ν

Tanaka et al. (1983)

Bundschu et al. (1997)

Shyn (1991)

Present experiment

DMR SE

DMR SEP

5 10 15 20 0 5 10

Collision energy(eV)Collision energy(eV)

Differential cross section (Å

/sr)

15 20

Figure 3.15 DCS (at θ = 90°) for the vibrational excitation of CH

. Combined cross sections for the excita-

tions of the rst and third modes and for the second and fourth modes are shown. Both are the cross sections

for the excitation from the ground to the rst excited states of the respective modes. For details, see C

urík etal.

(2008). (Reprinted from C

urík, R. etal., J. Phys. B: At. Mol. Opt. Phys., 41, 115203-1, 2008. With permission.)

Electron Collisions with Molecules in the Gas Phase 45

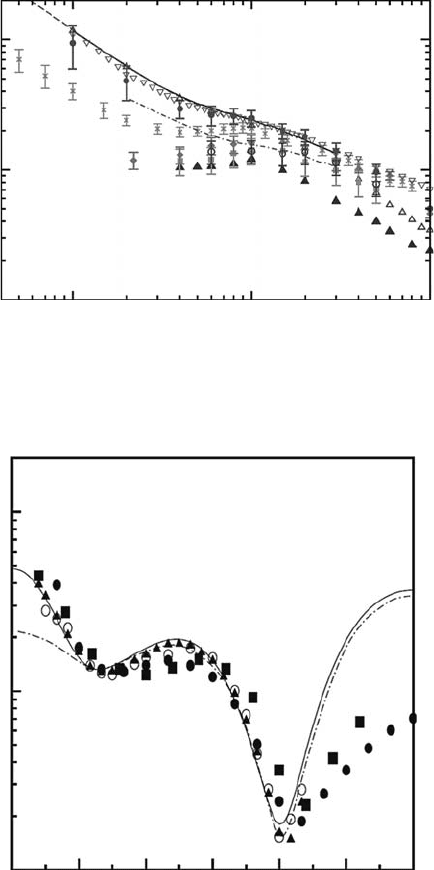
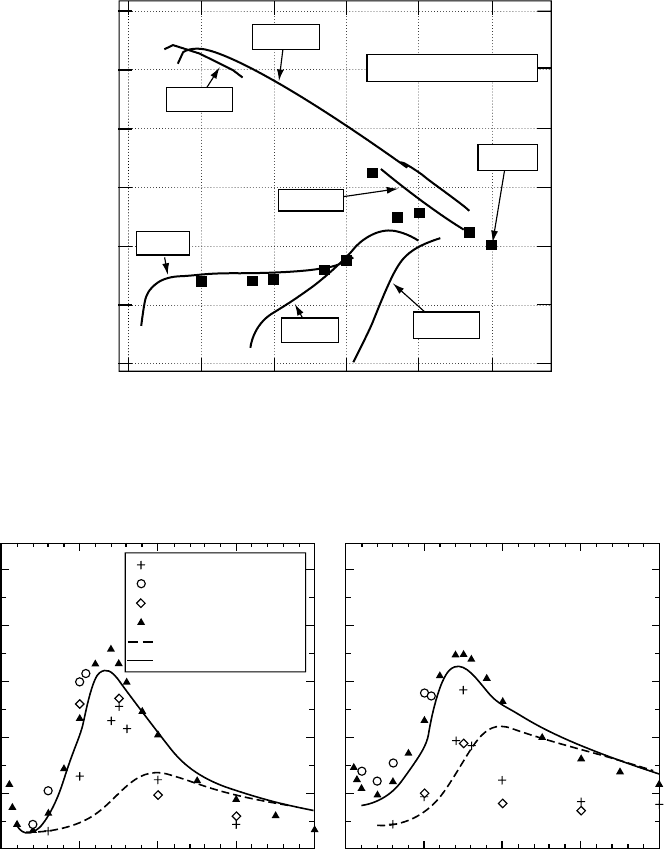
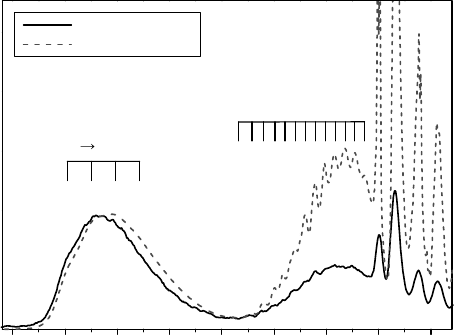
3.3.3.3.3 Energy-Loss Spectra for Electronic Excitation of H

Figure 3.17 shows the threshold electron spectrum for H

O (Jureta, 2005). This spectrum was

obtained by a high-resolution electron spectrometer combined with the penetrating eld method for

scattered electrons with energies close to zero electron volts. This technique is sensitive to observ-

ing a resonance feature. In the H

O molecule, one clearly sees resonant structures emerging near

the thresholds of the rst and the second band. These structures are not observed in the ordinary

energy-loss spectra (Figure 3.18) (Kato etal., 2005). Note that resonances are important in the DEA

producing

negative-ion fragments (see Section 3.3.4.3).

is shown in Section 3.3.1, a summary of the available cross-section data for electron scat-

tering from H

O was given by Itikawa and Mason (2005). These authors noted that “to date no

0.1

0.08

0.06

0.03

0.02

0.01

0.04

0.02

0 100 200

Present experimental spectra

DMR SEP spectra

+ν

×40

×20

=5 eV, = 90°

=20 eV, = 90°

300

Energy loss(meV)

Differential cross section (Å

/sr)

400 500 0 100 200

Energy loss(meV)

300 400 500

Figure 3.16 Energy-loss spectra of CH

at a scattering angle θ = 90° for incident electrons of 5 and 20eV.

Vertical bars represent theoretical values of the corresponding DCSs. (Reprinted from C

urík, R. etal., J. Phys. B:

At. Mol. Opt. Phys.,

41, 115203-1, 2008. With permission.)

6 7

(Times 3)

(

)

(

)

(R)

(V)

0 1 2

1 2

’

’=

Resonances

Incident electron energy (eV)

Yield of threshold electrons(arb. units)

9 10 11

Figure 3.17 Threshold electron-impact spectrum of H

O in the energy region of 5.2–10.8eV. (Reprinted

from

Jureta, J.J., Eur. Phys. J. D, 32, 319, 2005. With permission.)

46 Charged Particle and Photon Interactions with Matter

electron-beam measurements have reported absolute values of the (electronic state) excitation cross

section of H

O.” This fact prevented Itikawa and Mason from providing a recommended set of

values, which they considered to be a serious problem since “electronic excitation is important

in planetary atmospheres, plasmas, and radiation chemistry.” As a consequence, they noted that

“experiments and rened theory are urgently needed.” The results of more recent experiments

described

here serve as one step in trying to overcome this deciency.

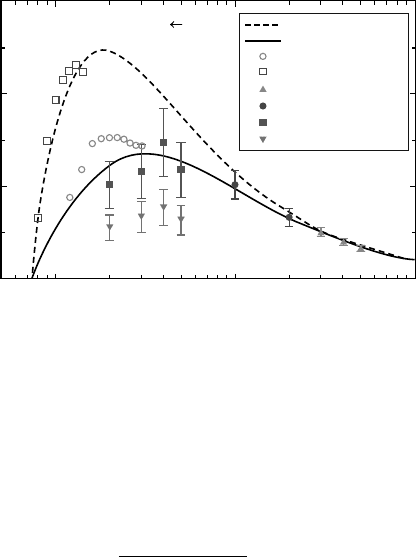
With

the use of a high-resolution technique, Tanaka and his collaborators obtained the energy-

loss spectra of H

O. One example is shown in Figure 3.18 in comparison with the optical absorp-

tion spectrum. The rst broadband with no structure is located from 6.8 to 8.5eV, leading to the

dissociation channel. The second with a small vibration structure on the left side and with a sharp

spike due to the Rydberg states on the right is seen from 8.5 to 11eV. From this and other spectra,

the authors successfully obtained cross sections for the excitations of low-lying six electronic states

of H

O (Thorn etal., 2007a,b; Brunger etal., 2008). As is shown by Itikawa and Mason, there are a

number of theoretical calculations reporting the excitation cross sections. In the above papers, the

newly obtained experimental cross sections are compared with these calculations to nd a large

disagreement

between theory and experiment.

3.3.3.3.4

Application of the Scaled Born Model in Optically Allowed Transition

An excitation of the Ã

electronic state of water is an important channel for the production of the

OH (X

Π) radical, as well as for the emission of the atmospheric Meinel bands. As a part of their

series of the work on electron-impact electronic excitations (see Section 3.3.3.3.3), Thorn etal. mea-

sured the cross section for the Ã

state (Thorn etal., 2007a). Their measurement was performed

over incident energies of 20–200 eV and scattering angles of 3.5°–90°. This is the rst time for

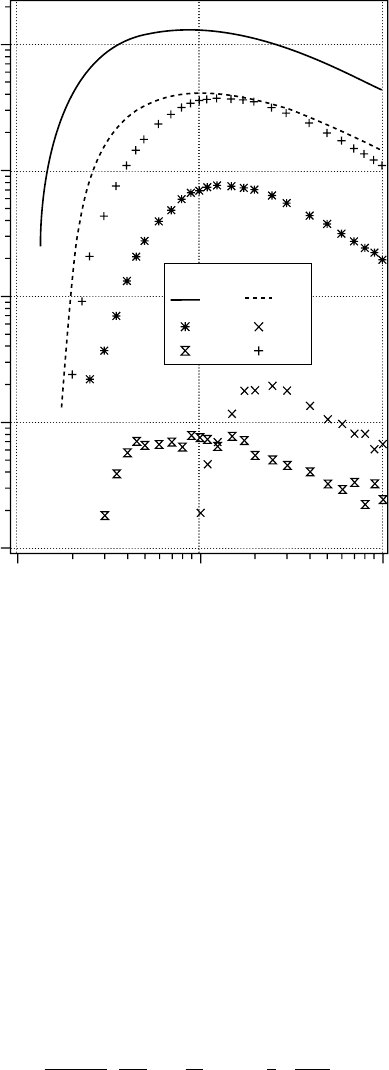
such data to be reported in the literature. To test the accuracy of the experimental data, Thorn etal.

deduced the optical oscillator strength (OOS) for the Ã

–

transition. First they calculated

the generalized oscillator strength (GOS) from the DCS measured at 100 and 200eV. In the limit

of zero momentum transfer, they obtained the OOS from the GOS. The resulting OOS was found

to agree well with the value derived from the photoabsorption experiment. Then they applied the

scaled

Born method to make the data on the excitation cross section more comprehensive.

is well known that the Born approximation gives fairly well an excitation cross section for

optically allowed states at high collision energies. Modifying the Born result, Kim developed a

6.5 7.0 7.5 8.0 8.5 9.0 9.5 10.0 10.5

1280 Å

Intensity (arb. units)

Loss energy (eV)

30 eV, θ=3°

/3sa

;

Limao VUV data

Figure 3.18 Electron energy-loss spectrum of H

O at an impact energy of 30eV for a scattering angle of 3°.

The result from Kato et al., 2005 is compared with the VUV photoabsorption data obtained by Limao (cited

Mota et al., 2005).

Electron Collisions with Molecules in the Gas Phase 47

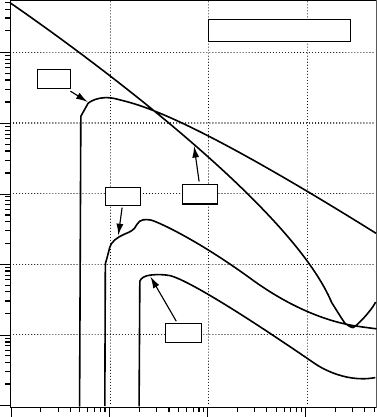
new, simple method of calculation of the excitation cross section of atoms over the entire range of

collision energy (Kim, 2001). He extended this to the case of a molecular target. It is called the BEf-

scaled

Born method (Kim, 2007). The BEf-scaled Born cross section, σ

BEf

, is given by

σ σ

BEf

accur

Born

( )

( )T

f T

f T B E

+ +

(3.10)

where

is the energy of the incident electron

B is the binding energy of the electron being excited

is the excitation energy

The

OOS is denoted by f. The quantity f

accur

is an accurate value obtained from experiments or

calculations with accurate wave functions, and f

Born

is the value obtained from calculation with the

wave function employed in the calculation of the unscaled Born cross section, σ

Born

. The f-scaling

process has the effect of replacing the wave function used for σ

Born

with an accurate one. BE scaling

corrects the deciency of the Born approximation at low T, without losing its well-known validity at

high T. Thorn etal. obtained σ

Born

and f

Born

from their GOS, and then substituted them into Equation

3.10. The resulting σ

BEf

is shown in Figure 3.19 in comparison with the measured values by Thorn

etal. It is clear that the BEf-scaled Born method gives fairly good values of the cross section over

all collision energies. This method is now applied to H

(Kim, 2007; Kato etal., 2008a), CO (Kato

etal., 2007), and CO

(Kawahara etal., 2008). Thus, once the Born cross section is obtained theo-

retically or experimentally, a fairly reasonable cross section can be produced at any incident energy

with

this approach.

3.3.4 croSS SectionS for the production of the Secondary SpecieS

3.3.4.1 ionization Cross section

The ionization cross section is extremely important in the study of radiation sciences. Therefore,

experimental data on the ionization cross section have been accumulated for a very long time. In

total ion measurement, a collection of all product ions should be guaranteed. Particular care must

be taken to avoid discrimination against energetic (fragment) ions. Furthermore, a preference is

0.3

0.2

0.1

0.0

Unscaled Born

BEf-scaled Born

Gil

Gorfinkiel

Lassettre

Sophia

Sophia +Flinders

Flinders

T (eV)

–

onH

O A

exc

(Å

)

Figure 3.19 Comparison of experimental and theoretical integral cross sections for excitation of the

electronic state in H

O. The solid line is the BEf-scaled Born result. For details, see Thorn etal.

(2007a). (Reprinted from Thorn, P.A. etal., J. Chem. Phys., 126, 064306-1, 2007a. With permission.)

48 Charged Particle and Photon Interactions with Matter

placed on the experiment adopting no normalization to other data. To measure partial ionization

cross sections, one can use a parallel-plate apparatus with a time-of-ight (TOF) mass spectrometer

and a position-sensitive detector. The partial ionization cross sections can be made absolute inde-

pendently for each mass number, but, in some cases, are normalized to the total ion measurement.

Recommended data of partial cross sections of H

O are presented for the products of H

, OH

, O

, H

, and H

in Figure 3.20 (Itikawa and Mason, 2005).

Along the increase of research activity in application elds, the need of ionization cross section

is extended to new molecular species, some of which are not accessible to experiment at present. To

produce ionization cross sections, several theoretical methods have been proposed. One of these is

the binary-encounter-Bethe (BEB) model of Kim and his colleagues (Hwang etal., 1996). This is a

simplied version of the BED model (see Section 3.2.3), particularly applicable to molecular targets.

The

ionization cross section in the model is given by

BEB

+ +

−













+ − −

























t u

t t

t1 2

ln ln

(3.11)

where

t = T/B

u = U/B

S a N R B

2 2

( / )

–16

–17

–18

–19

–20

2 3 4 5 6 7 8 2 3 4 5 6 78

100

Electron energy (eV)

Cross section (cm

)

1000

e+H

O ionization

Figure 3.20 Recommended values of partial ionization cross sections of H

O for the production of H

, O

, H

, and H

. (Reprinted from Itikawa, Y. and Mason, N.J., J. Phys. Chem. Ref. Data, 34, 1, 2005.

With

permission.)

Electron Collisions with Molecules in the Gas Phase 49

The quantities U, B, and N are the kinetic and binding energies and the occupation number of

molecular electrons, respectively, and T is the energy of the incident electron. The numerical con-

stants are a

= 0.52918 Å and R = 13.6057eV. (In some cases, a more sophisticated formula with

dipole oscillator strengths is also called a BEB model. See the paper by Hwang etal., 1996.) Note

that formula (3.11) gives the total ionization cross section. The resulting cross sections for small

atoms, a variety of large and small molecules, and radicals are accurate within an error of 5%–20%

from threshold to T ∼ 1 keV (NIST). Examples are shown in Figures 3.21 (Kim and Rudd, 1994) and

3.22 (Kim etal., 1997) for H

O and CH

, respectively.

3.3.4.2 dissociation

to p

roduce

eutral

Fragments

Many

of the neutral fragments of molecular dissociation are active species (e.g., radicals).

Furthermore, most of the fragments have a considerable kinetic energy. Hence, these dissocia-

tion products may play an active role in radiation interaction with matter. When the fragments are

2.5

2.0

1.5

1.0

0.5

0.0

Cross section (10

–20

)

–

on H

T (eV)

Figure 3.21 Total ionization cross section of H

O. The BEB cross section (solid line) is compared

with experimental data. (Reprinted from Kim, Y.-K. and Rudd, M.E., Phys. Rev. A, 50, 3954, 1994. With

permission.)

–

on CH

Rapp

Orient

Duric

Schram

BEB(Adiab.)

BEB(Vert.)

T (eV)

(10

–20

)

Figure 3.22 Total ionization cross section of CH

. The BEB cross section (solid line) is compared with

experimental

data. (Reprinted from Kim, Y.-K. etal., J. Chem. Phys., 106, 1026, 1997. With permission.)