Jeanblanc M., Yor M., Chesney M. Mathematical Methods for Financial Markets
Подождите немного. Документ загружается.


6.4 Constant Elasticity of Variance Process 367
The functions Φ
λ↑
and Φ
λ↓
are
Φ
λ↑
(x)=
√
xK
ν
x
−β
σβ
√
2λ
,Φ
λ↓
(x)=
√
xI
ν
x
−β
σβ
√
2λ
with ν =1/(2β).
Case β<0. One defines X
t
= −
1
σβ
S
−β
t
and one checks that, on the set
t<T
0
(S),
dX
t
= dW
t
+
1
2
β +1
β
1
X
t
dt .
Therefore, X is a Bessel process of negative index 1/(2β) which reaches 0,
hence S reaches 0 too.
The formula for the density of the r.v. X
t
is still valid as long as the
dimension δ of the Bessel process X is positive, i.e., for δ =2+
1
β
> 0(or
β<−
1
2
) and one obtains
P
x
(S
t
∈ dy)=
1
σ
2
(−β)t
x
1/2
y
−2β−3/2
exp
−
x
−2β
+ y
−2β
2σ
2
β
2
t
I
ν
x
−β
y
−β
σ
2
β
2
t
dy .
For −
1
2
<β<0, the process X with negative dimension (δ<0), reaches
0 (see Subsection 6.2.6). Here, we stop the process after it first hits 0, i.e., we
set
S
t
=(σβX
t
)
1/(−β)
, for t ≤ T
0
(X) ,
S
t
=0, for t>T
0
(X)=T
0
(S) .
The density of S
t
is now given from the one of a Bessel process of positive
dimension 4 −(2 +
1
β
)=2−
1
β
, (see Subsection 6.2.6), i.e., with positive index
−
1
2β
. Therefore, for any β ∈ [−1/2, 0[ and y>0,
P
x
(S
t
∈ dy)=
x
1/2
y
−2β−3/2
σ
2
(−β)t
exp
−
x
−2β
+ y
−2β
2σ
2
β
2
t
I
|ν|
x
−β
y
−β
σ
2
β
2
t
dy .
It is possible to prove that
Φ
λ↑
(x)=
√
xI
|ν|
x
−β
σ|β|
√
2λ
,Φ
λ↓
(x)=
√
xK
|ν|
x
−β
σ|β|
√
2λ
.
In the particular case β = 1, we obtain that the solution of dS
t
= σS
2
t
dW
t
is
S
t
=1/(σR
t
), where R is a BES
3
process.
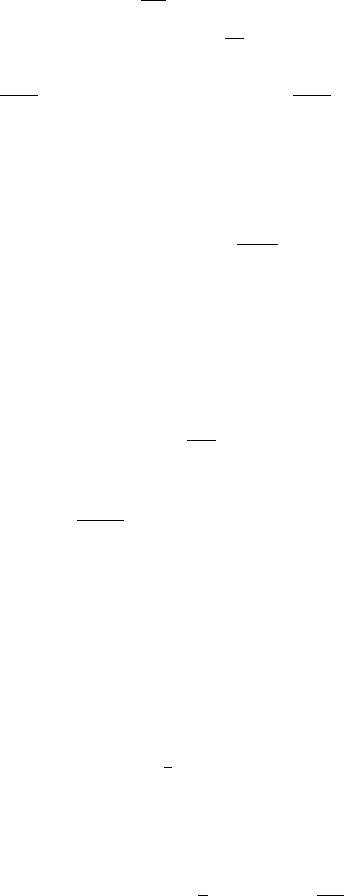
368 6 A Special Family of Diffusions: Bessel Processes
6.4.2 CEV Processes and CIR Processes
Let S follow the dynamics
dS
t
= S
t
(μdt + σS
β
t
dW
t
) , (6.4.2)
where μ =0.Forβ = 0, setting Y
t
=
1
4β
2
S
−2β
t
, we obtain
dY
t
= k(θ −Y
t
)dt + σ
Y
t
dW
t
with k =2μβ, θ = σ
2
2β+1
4kβ
, σ = −sgn(β)σ and kθ = σ
2
2β+1
4β
, i.e., Y follows a
CIR dynamics; hence S is the power of a (time-changed) CIR process.
Let us study the particular case k>0,θ > 0, which is obtained either for
μ>0,β > 0orforμ<0,β <−1/2.
In the case μ>0,β >0, one has kθ ≥ σ
2
/2 and the point 0 is not reached
by the process Y .Inthecaseβ>0, from S
2β
t
=
1
4β
2
Y
t
, we obtain that S does
not explode and does not reach 0.
In the case μ<0,β < −1/2, one has 0 <kθ<σ
2
/2, hence, the point 0 is
reached and is a reflecting boundary for the process Y ;fromS
−2β
t
=4β
2
Y
t
,
we obtain that S reaches 0 and is reflected.
The other cases can be studied following the same lines (see Lemma 6.4.4.1
for related results).
Note that the change of variable Z
t
=
1
σ|β|
S
−β
t
reduces the CEV process
to a “Bessel process with linear drift”:
dZ
t
=
β +1
2βZ
t
− μβZ
t
dt + d
W
t
where
W
t
= −(sgnβ)W
t
. Thus, such a process is the square root of a CIR
process (as proved before!).
6.4.3 CEV Processes and BESQ Processes
We also extend the result obtained in Subsection 6.4.1 for μ = 0 to the general
case.
Lemma 6.4.3.1 For β>0,orβ<−
1
2
, a CEV process is a deterministic
time-change of a power of a BESQ process:
S
t
= e
μt
ρ
c(t)
−1/(2β)
,t≥ 0
(6.4.3)
where ρ is a BESQ with dimension δ =2+
1
β
and c(t)=
βσ
2
2μ
(e
2μβt
− 1).
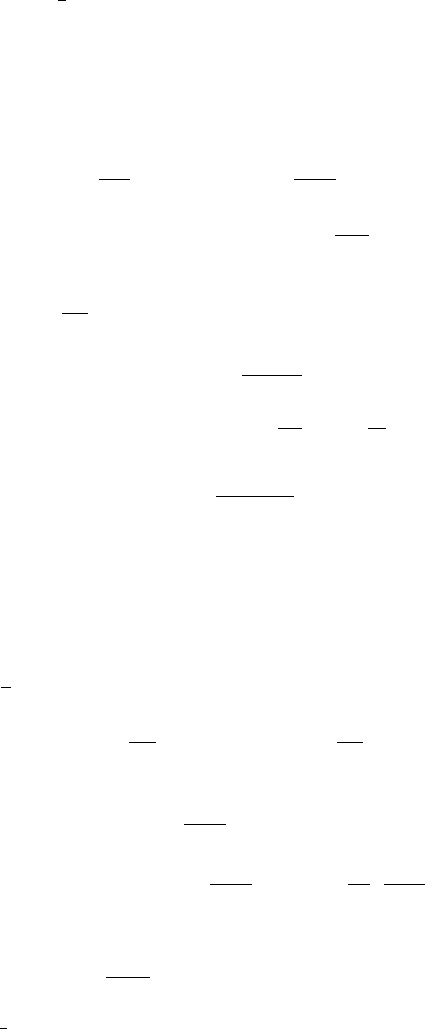
6.4 Constant Elasticity of Variance Process 369
If 0 >β>−
1
2
S
t
= e
μt
ρ
c(t)
−1/(2β)
,t≤ T
0
where T
0
is the first hitting time of 0 for the BESQ ρ.
For any β and y>0, one has
P
x
(S
t
∈ dy)=
|β|
c(t)
e
μ(2β+1/2)t
exp
−
1
2c(t)
x
−2β
+ y
−2β
e
2μβt
× x
1/2
y
−2β−3/2
I
1/(2β)
1
γ(t)
x
−β
y
−β
e
μβt
dy .
Proof: This follows either by a direct computation or by using the fact that
the process Y =
1
4β
2
S
−2β
is a CIR(k, θ) process which satisfies
Y
t
= e
−kt
ρ(σ
2
e
kt
− 1
4k
),t≥ 0 ,
where ρ(·) is a BESQ process with index
2kθ
σ
2
− 1=
1
2β
. This implies that
S
t
= e
μt
4β
2
ρ
σ
2
e
2μβt
− 1
8βμ
−1/(2β)
,t≥ 0 .
It remains to transform ρ by scaling to obtain formula (6.4.3). The density
of the r.v. S
t
follows from the knowledge of densities of Bessel processes with
negative dimensions (see Proposition 6.2.6.3).
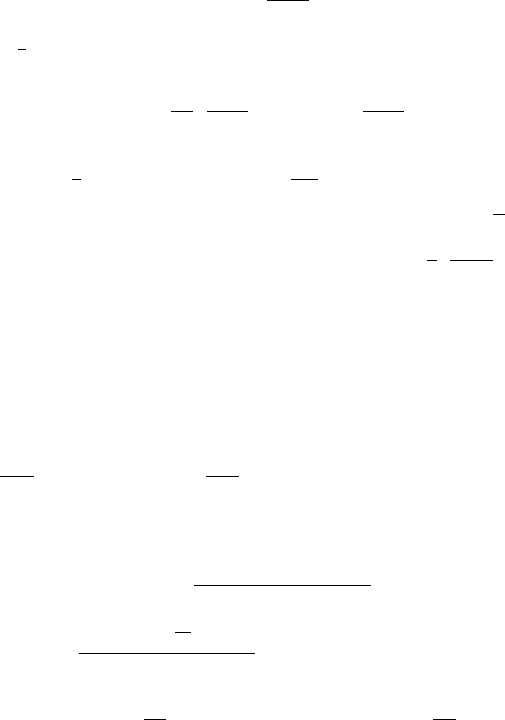
Proposition 6.4.3.2 Let S be a CEV process starting from x and introduce
δ =2+
1
β
.Letχ
2
(δ, α; ·) be the cumulative distribution function of a χ
2
law with δ degrees of freedom, and non-centrality parameter α (see Exercise
1.1.12.5). We set c(t)=
βσ
2
2μ
(e
2μβt
− 1) and y =
1
c(t)
y
−2β
e
2μβt
.
For β>0, the cumulative distribution function of S
t
is
P
x
(S
t
≤ y)=1− χ
2
δ,
x
−2β
c(t)
; y
=1−
∞
n=1
g
n,
x
−2β
2c(t)
G
n +
1
2β
,
1
2c(t)
y
−2β
e
2μβt
where
g(α, u)=
u
α−1
Γ (α)
e
−u
,G(α, u)=
v≥u
g(α, v)1
v≥0
dv .
For −
1
2
>β( i.e., δ>0,β < 0) the cumulative distribution function of
S
t
is
370 6 A Special Family of Diffusions: Bessel Processes
P
x
(S
t
≤ y)=χ
2
δ,
x
−2β
c(t)
; y
.
For 0 >β>−
1
2
(i.e., δ<0), the cumulative distribution function of S
t
is
P
x
(S
t
≤ y)=1−
∞
n=1
g(n −
1
2β
,
1
2c(t)
x
−2β
) G
n,
1
2c(t)
y
−2β
e
2μβt
.
Proof: Let δ =2+
1
β
, x
0
= x
−2β
and c(t)=
βσ
2
2μ
(e
2μβt
− 1).
If ρ is a BESQ
δ
x
with δ ≥ 0, then ρ
t
law
= tY ,whereY
law
= χ
2
(δ,
x
t
)(see
Remark 6.2.2.1). Hence, ρ
c(t)
law
= c(t)Z,whereZ
law
= χ
2
2+
1
β
,
x
−2β
2c(t)
.The
formula given in the Proposition follows from a standard computation.
For δ<0 (i.e., 0 >β>−1/2), from Lemma 6.2.6.1
P
x
(S
t
≥ y, T
0
(S) ≥ t)=Q
δ
x
0
(ρ
c(t)
≥ (e
−μt
y)
−2β
1
{c(t)<T
0
(ρ)}
)
= xQ
4−δ
x
0
(ρ
c(t)
)
1/(2β)
1
{ρ(c(t))≥(e
−μt
y)
−2β
}
.
Setting w =
1
2c(t)
y
−2β
e
2μβt
and z =
1
2c(t)
x
−2β
P
x
(S
t
≥ y)=x(c(t))
1/(2β)
E
Z
1/(2β)
1
{Z≥2w}
= xe
−z
(2c(t))
1/(2β)
∞
n=0
z
n
n!Γ (n +1− 1/(2β))
v≥w
v
n
e
−v
dv
= e
−z
∞
n=0
z
n−
1
2β
n!Γ (n +1− 1/(2β))
v≥w
v
n
e
−v
dv
=
∞
n=0
g
1+n −
1
2β
,z
G(n +1,w)=
∞
n=1
g
n −
1
2β
,z
G(n, w) .
6.4.4 Properties
From the results on Bessel processes, we obtain (recall that in the case of
negative dimension we stop the processes at level 0).
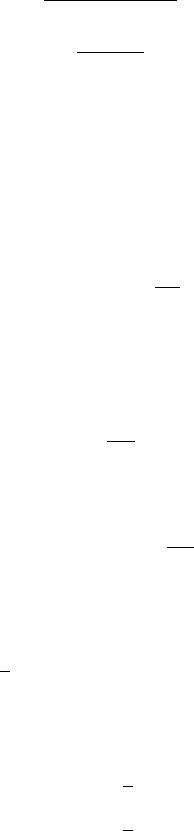
Lemma 6.4.4.1 For β<0, the boundary {0} is reached a.s..
For β<−1/2, {0} is instantaneously reflecting.
For −1/2 <β<0, {0} is an absorbing point.
For 0 <β, {0} is an unreachable boundary.
6.4 Constant Elasticity of Variance Process 371
From the knowledge of the density of S
T
, we can check that, for x>0
P
x
(S
T
> 0) =
γ(−ν, ζ(T ))/Γ (−ν),β<0
1,β>0 ,
E
x
(S
T
)=
xe
μT
,β<0
xe
μT
γ(ν, ζ(T ))/Γ (ν) β>0
where ν =1/(2β), γ(ν, ζ)=
ζ
0
t
ν−1
e
−t
dt is the incomplete gamma function,
and
ζ(T )=
⎧
⎪
⎨
⎪
⎩
μ
βσ
2
(e
2μβT
− 1)
x
−2β
,μ=0
1
2β
2
σ
2
T
x
−2β
,μ=0.
Note that, in the case β>0, the expectation of e
−μT
S
T
is not S
0
, hence, the
process (e
−μt
S
t
,t ≥ 0) is a strict local martingale. We have already noticed
this fact in Subsection 6.4.1 devoted to the study of the case μ =0,usingthe
results on Bessel processes.
Using (6.2.9), we deduce:
Proposition 6.4.4.2 Let X be a CEV process, with β>−1.Then
P
x
(T
0
<t)=G
−1
2β
,ζ(t)
.
6.4.5 Scale Functions for CEV Processes
The derivative of the scale function and the density of the speed measure are
s
(x)=
⎧
⎨
⎩
exp
μ
σ
2
β
x
−2β
,β=0
x
−2μ/σ
2
β =0
and
m(x)=
⎧
⎨
⎩
2σ
−2
x
−2−2β
exp
−
μ
σ
2
β
x
−2β
,β=0
2σ
−1
x
−2+2μ/σ
2
β =0.
The functions Φ
λ↓
and Φ
λ↑
are solutions of
1
2
σ
2
x
2+2β
u
+ μxu
− λu =0
and are given by:
for β>0,
Φ
λ↑
(x)=x
β+1/2
exp
2
cx
−2β
W
k,n
(cx
−2β
),
Φ
λ↓
(x)=x
β+1/2
exp
2
cx
−2β
M
k,n
(cx
−2β
),

372 6 A Special Family of Diffusions: Bessel Processes
for β<0,
Φ
λ↑
(x)=x
β+1/2
exp
2
cx
−2β
M
k,n
(cx
−2β
) ,
Φ
λ↓
(x)=x
β+1/2
exp
2
cx
−2β
W
k,n
(cx
−2β
) ,
where
c =
|μ|
|β|σ
2
,= sign(μβ) ,
n =
1
4|β|
,k=
1
2
+
1
4β
−
λ
2|μβ|
,
and W and M are the classical Whittaker functions (see Subsection A.5.7).
6.4.6 Option Pricing in a CEV Model
European Options
We give the value of a European call, first derived by Cox [205], in the case
where β<0. The interest rate is supposed to be a constant r. The previous
computation leads to
E
Q
(e
−rT
(S
T
− K)
+
)=S
0
∞
n=1
g(n, z)G
n −
1
2β
,w
− Ke
−rT
∞
n=1
g
n −
1
2β
,z
G(n, w)
where g, G are defined in Proposition 6.4.3.2 (with μ = r), z =
1
2c(T )
S
−2β
0
and
w =
1
2c(T )
K
−2β
e
2rβT
.
In the case β>0,
E
Q
(e
−rT
(S
T
− K)
+
)=S
0
∞
n=1
g
n +
1
2β
,z
G(n, w)
− Ke
−rT
∞
n=1
g(n, z)G
n +
1
2β
,w
.
We recall that, in that case (S
t
e
−rt
,t ≥ 0) is not a martingale, but a strict
local martingale.
Barrier and Lookback Options
Boyle and Tian [121] and Davydov and Linetsky [225] study barrier and
lookback options.
See Lo et al. [601], Schroder [772], Davydov and Linetsky [227]and
Linetsky [592, 593] for option pricing when the underlying asset follows a
CEV model.

6.5 Some Computations on Bessel Bridges 373
Perpetual American Options
The price of a perpetual American option can be obtained by solving the
associated PDE. The pair (b, V ) (exercise boundary, value function) of an
American perpetual put option satisfies
σ
2
2
x
2(β+1)
V
(x)+rxV
(x)=rV (x),x>b
V (x) ≥ (K − x)
+
V (x)=K − x, for x ≤ b
V
(b)=−1 .
The solution is
V (x)=Kx
∞
x
1
y
2
exp
r
σ
2
β
(y
−2β
− b
−2β
)
dy, x > b
where b is the unique solution of the equation V (b)=K − b.(SeeEkstr¨om
[295] for details and properties of the price.)
6.5 Some Computations on Bessel Bridges
In this section, we present some computations for Bessel bridges, which are
useful in finance. Let t>0andP
δ
be the law of a δ-dimensional Bessel process
on the canonical space C([0,t], R
+
) with the canonical process now denoted
by (R
t
,t ≥ 0). There exists a regular conditional distribution for P
δ
x
( |R
t
),
namely a family P
δ,t
x→y
of probability measures on C([0,t], R
+
) such that for
any Borel set A
P
δ
x
(A)=
P
δ,t
x→y
(A)μ
t
(dy)
where μ
t
is the law of R
t
under P
δ
x
. A continuous process with law P
δ,t
x→y
is
called a Bessel bridge from x to y over [0,t].
6.5.1 Bessel Bridges
Proposition 6.5.1.1 For a Bessel process R,foreachpair(ν, μ) ∈ R
+
×R
+
,
and for each t>0, one has
P
(ν)
x
[exp( −
μ
2
2
t
0
ds
R
2
s
)|R
t
= y]=
I
γ
(xy/t)
I
ν
(xy/t)
, (6.5.1)
where γ =
ν
2
+ μ
2
.
Equivalently, for a squared Bessel process, for each pair (ν, μ) ∈ R
+
×R
+
,
and for each t>0, one has

374 6 A Special Family of Diffusions: Bessel Processes
Q
(ν)
x
exp
−
μ
2
2
t
0
ds
ρ
s
|ρ
t
= y
=
I
γ
(
√
xy/t)
I
ν
(
√
xy/t)
, (6.5.2)
and, for any b ∈ R,
Q
(ν)
x
exp
−
b
2
2
t
0
ρ
s
ds
|ρ
t
= y
(6.5.3)
=
bt
sinh(bt)
exp
x + y
2t
(1 − bt coth bt)
I
ν
[b
√
xy/sinh bt]
I
ν
[
√
xy/t]
.
Proof: On the one hand, from (6.2.3)and(6.2.10), for any a>0andany
bounded Borel function f,
P
(ν)
x
f(R
t
)exp
−
μ
2
2
t
0
ds
R
2
s
= P
(γ)
x
R
t
x
ν−γ
f(R
t
)
=
∞
0
dy
y
x
ν−γ
f(y)
y
t
y
x
γ
exp
−
x
2
+ y
2
2t
I
γ
(xy/t) .
On the other hand, from (6.2.3), this expression equals
∞
0
dyf(y)P
(ν)
x
exp
−
μ
2
2
t
0
ds
R
2
s
|R
t
= y
y
t
y
x
ν
exp
−
x
2
+ y
2
2t
I
ν
(xy/t)
and the result follows by identification. The squared Bessel bridge case follows
from (6.2.14) and the knowledge of transition probabilities.
Example 6.5.1.2 The normalized Brownian excursion |B|
[g
1
,d
1
]
is a BES
3
bridge from x =0toy = 0 with t = 1 (see equation (4.3.1) for the notation).
6.5.2 Bessel Bridges and Ornstein-Uhlenbeck Processes
We shall apply the previous computation in order to obtain the law of hitting
times for an OU process. We have studied OU processes in Subsection 2.6.3.
Here, we are concerned with hitting time densities for OU processes with
parameter k:
dX
t
= dW
t
− kX
t
dt .
Proposition 6.5.2.1 Let X be an OU process starting from x and T
0
(X) its
first hitting time of 0: T
0
(X):=inf{t : X
t
=0}.
Then P
x
(T
0
(X) ∈ dt)=f (x, t)dt,where
f(x, t)=
|x|
√
2π
exp
kx
2
2
exp
k
2
(t − x
2
coth(kt))
k
sinh(kt)
3/2
.
6.5 Some Computations on Bessel Bridges 375
Proof: The absolute continuity relationship established in Subsection 2.6.3
reads
P
k,0
x
|
F
t
=exp
−
k
2
(W
2
t
− t − x
2
) −
k
2
2
t
0
W
2
s
ds
W
x
|
F
t
and holds with the fixed time t replaced by the stopping time T
a
, restricted
to the set T
a
< ∞, leading to
P
k,0
x
(T
a
∈ dt) = exp
−
k
2
(a
2
− t − x
2
)
W
x
1
{T
a
∈dt}
exp
−
k
2
2
t
0
W
2
s
ds
.
Hence,
P
k,0
x
(T
a
∈ dt) = exp
−
k
2
(a
2
− t − x
2
)
× W
a−x
1
{T
0
∈dt}
exp
−
k
2
2
t
0
(a − W
s
)
2
ds
.
Let us set y = |a − x|. Under W
y
, the process (W
s
,s ≤ T
0
) conditioned by
T
0
= t is a BES
3
bridge of length t,startingaty and ending at 0, therefore
W
a−x
1
{T
0
∈dt}
exp
−
k
2
2
t
0
(a − W
s
)
2
ds
= P
3
y
exp
−
k
2
2
t
0
(a − R
s
)
2
ds
R
t
=0
W
y
(T
0
∈ dt)
where =sgn(a − x). For a = 0, the computation reduces to that of
P
3
y
exp
−
k
2
2
t
0
R
2
s
ds
R
t
=0
which, from (6.5.3)and(A.5.3)isequalto
kt
sinh(kt)
3/2
exp
−
y
2
2t
(kt coth(kt) − 1)
.
Comment 6.5.2.2 The law of the hitting time for a general level a requires
the knowledge of the joint law of
t
0
R
s
ds,
t
0
R
2
s
ds
under the BES
3
-bridge
law. See Alili et al. [10], G¨oing-Jaeschke and Yor [397]andPatie[697].
Exercise 6.5.2.3 As an application of the absolute continuity relationship
(6.3.3)betweenaBESQandaCIR,provethat
k
Q
δ,t
x→y
=
exp
−
k
2
2
t
0
ρ
s
ds
Q
δ,t
x→y
exp
−
k
2
2
t
0
ρ
s
ds
Q
δ,t
x→y
(6.5.4)
376 6 A Special Family of Diffusions: Bessel Processes
where
k
Q
δ,(t)
x→y
denotes the bridge for (ρ
u
, 0 ≤ u ≤ t) obtained by conditioning
k
Q
δ
x
by (ρ
t
= y). For more details, see Pitman and Yor [716].
6.5.3 European Bond Option
Let P (t, T
∗
)=B(r
t
,T
∗
− t) be the price of a zero-coupon bond maturing at
time T
∗
when the interest rate (r
t
,t≥ 0) follows a risk-neutral CIR dynamics,
where B(r, s)=exp[−A(s) − rG(s)]. The functions A and G are defined in
Corollary 6.3.4.3. The price C
EB
of a T -maturity European call option on a
zero-coupon bond maturing at time T
∗
,whereT
∗
>T, with a strike price
equal to K is
C
EB
(r
t
,T − t)=E
Q
exp
−
T
t
r
s
ds
(P (T,T
∗
) − K)
+
|F
t
.
We present the computation of this quantity.
Proposition 6.5.3.1 Let us assume that the risk-neutral dynamics of r are
dr
t
= k(θ − r
t
)dt +2
√
r
t
dW
t
,r
0
= x.
Then,
C
EB
(r, T − t)=B(r, T
∗
− t) Ψ
1
− KB(r, T − t) Ψ
2
(6.5.5)
where
Ψ
1
= χ
2
4kθ
σ
2
,
2φ
2
r exp(γ(T
∗
− t))
φ + ψ + G(T
∗
− T )
;2r
∗
(φ + ψ + G(T
∗
− T ))
,
Ψ
2
= χ
2
4kθ
σ
2
,
2φ
2
r exp(γ(T
∗
− t))
φ + ψ
;2r
∗
(φ + ψ)
,
φ =
2γ
σ
2
(exp(γ(T
∗
− t)) − 1)
,ψ=
k + γ
σ
2
,
γ =
k
2
+2σ
2
,r
∗
= −
A(T
∗
− T )+ln(K)
G(T
∗
− T )
.
Here, χ
2
is the non-central chi-squared distribution function defined in
Exercise 1.1.12.5. The real number r
∗
is the critical interest rate defined by
K = B(r
∗
,T
∗
−T ) for T
∗
>T below which the European call will be exercised
at maturity T .
Finally notice that K is constrained to be strictly less than A(T
∗
− T ), the
maximum value of the discount bond at time T, otherwise exercising would
of course never be done.
Proof: From P (T,T
∗
)=B(r
T
,T
∗
− T ), we obtain
