Klyatskin V.I. Lectures on Dynamics of Stochastic Systems
Подождите немного. Документ загружается.

166 Lectures on Dynamics of Stochastic Systems
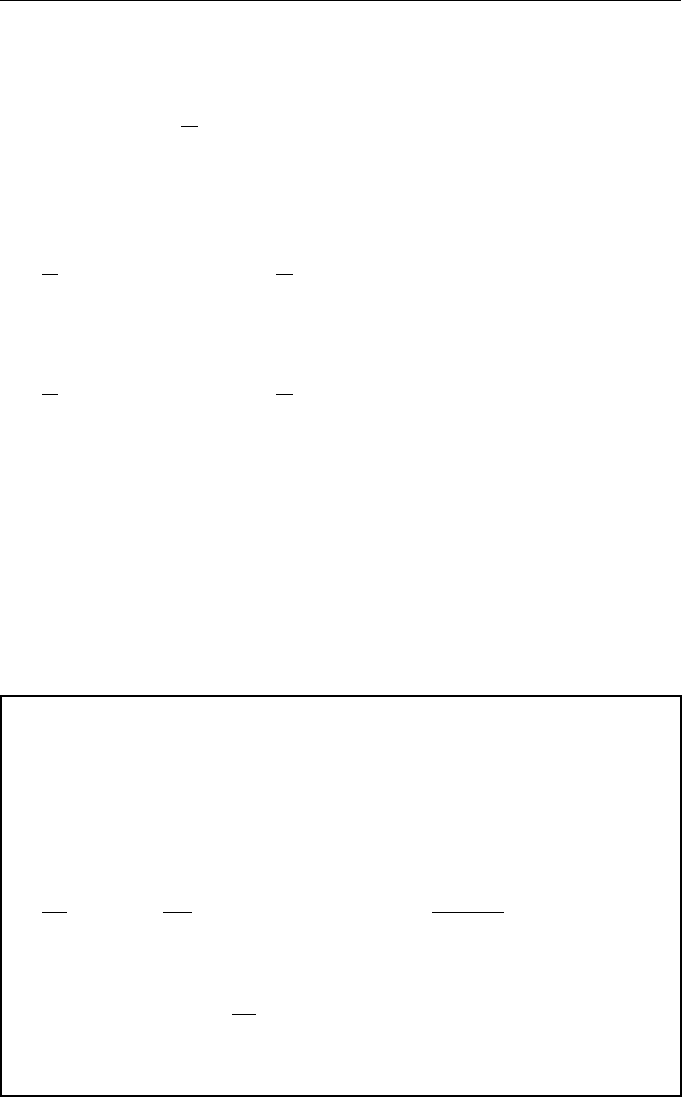
We can expand the logarithm of the characteristic functional 2[t; v(τ )] of delta-
correlated processes in the functional Fourier series
2[t; v(τ )] =
∞
X
n=1
i
n
n!
t
Z
0
dτ K
n
(τ )v
n
(τ ), (6.81)
where K
n
(t) determine the cumulant functions of process z(t). Substituting Eq. (6.81)
in Eq. (6.80), we obtain the equation
d
dt
h
x(t)
i
=
b
A(t)
h
x(t)
i
+
∞
X
n=1
1
n!
K
n
(t)
b
B(t)
n
h
x(t)
i
. (6.82)
If there exists power l such that
b
B
l
(t) = 0, then Eq. (6.82) assumes the form
d
dt
h
x(t)
i
=
b
A(t)
h
x(t)
i
+
l−1
X
n=1
1
n!
K
n
(t)
b
B(t)
n
h
x(t)
i
. (6.83)
In this case, the equation for average value depends only on a finite number of cumu-
lants of process z(t). This means that there is no necessity in knowledge of probability
distribution of function z(t) in the context of the equation for average value; sufficient
information includes only certain cumulants of process and knowledge of the fact that
process z(t) can be considered as the delta-correlated random process. Statistical des-
cription of an oscillator with fluctuating frequency is a good example of such system
in physics.
Problems
Problem 6.1
Starting from the backward Liouville equation (3.8), page 71 describing the
behavior of dynamic system (6.1), page 141 as a function of initial values t
0
and x
0
, derive the backward equation for probability density P(x, t|x
0
, t
0
) =
h
δ(x(t|x
0
, t
0
) − x)
i
.
Solution
∂
∂t
0
+ v(x
0
, t
0
)
∂
∂x
0
P(x, t|x
0
, t
0
) =
˙
2
t
0
t, t
0
;
δ
iδf ( y, τ )
δ
(
x(t|x
0
, t
0
) − x
)
,
where
˙
2
t
0
[t, t
0
; u( y, τ )] =
d
dt
0
ln 8[t, t
0
; u( y, τ )]
and 8[t, t
0
; u( y, τ )] is the characteristic functional of field f (x
0
, t
0
).
General Approaches to Analyzing Stochastic Systems 167
Problem 6.2
Derive the equation for probability density of the solution to the one-dimensional
problem on passive tracer diffusion
∂
∂t
ρ(x, t) + v(t)
∂
∂x
f (x)ρ(x, t) = 0,
where v(t) is the Gaussian stationary random process with the parameters
h
v(t)
i
= 0,
v(t)v(t
0
)
= B(t − t
0
)
B
v
(0) =
D
v
2
(t)
E
and f (x) is the deterministic function.
Instruction Average the Liouville equation (3.19), page 74 that can be rewritten
in the form
∂
∂t
ϕ(x, t; ρ) = −v(t)
∂
∂x
f (x) −
∂f (x)
∂x
1 +
∂
∂ρ
ρ
ϕ(x, t; ρ),
ϕ(x, 0; ρ) = δ(ρ
0
(x) − ρ)
over an ensemble of realizations of random process v(t). Use the Furutsu–
Novikov formula (5.16), page 126 for functional ϕ[x, t; ρ; v(τ )] and take into
account Eq. (6.12), page 145 that assumes in this problem the form
δ
δv(t
0
)
ϕ
[
x, t; ρ; v(τ)
]
=
∂ϕ(x, T(t), ρ)
∂T
θ(t − t
0
)
= −θ(t − t
0
)
∂
∂x
f (x) −
∂f (x)
∂x
1 +
∂
∂ρ
ρ
ϕ(x, t; ρ),
where T[t, v(τ)] =
Z
t
0
dτ v(τ ), is the new “random” time, and θ (t) is the Heav-
iside step function.
Solution
∂
∂t
P(x, t; ρ) =
t
Z
0
dτ B(τ )
∂
∂x
f (x) −
df (x)
dx
1 +
∂
∂ρ
ρ
×
f (x)
∂
∂x
−
df (x)
dx
∂
∂ρ
ρ
P(x, t; ρ),
P(0, x; ρ) = δ(ρ
0
(x) − ρ),
168 Lectures on Dynamics of Stochastic Systems
which can be rewritten in the form
∂
∂t
P(x, t; ρ) =
t
Z
0
dt
0
B(t
0
)
∂
∂x
f
2
(x)
∂
∂x
−
∂
∂x
f (x)
df (x)
dx
∂
∂ρ
ρ
−
df (x)
dx
f (x)
∂
∂x
1 +
∂
∂ρ
ρ
+
df (x)
dx
2
∂
∂ρ
ρ
1 +
∂
∂ρ
ρ
)
P(x, t; ρ),
P(x, 0; ρ) = δ(ρ
0
(x) − ρ).
Remark Note that, if function f (x) varies along x with characteristic scale k
−1
and
is a periodic function (‘fast variation’), then additional averaging over x results
in an equation for ‘slow’ spatial variations
∂
∂t
P(x, t; ρ) =
t
Z
0
dt
0
B(t
0
)
(
f
2
(x)
∂
2
∂x
2
+
df (x)
dx
2
∂
2
∂ρ
2
ρ
2
)
P(x, t; ρ),
P(x, 0; ρ) = δ(ρ
0
(x) − ρ).
If, moreover, the initial condition is independent of x (a homogeneous initial
state), the average probability density will be also independent of x and satisfy
the equation
∂
∂t
P(t; ρ) =
df (x)
dx
2
t
Z
0
dt
0
B(t
0
)
∂
2
∂ρ
2
ρ
2
P(t; ρ), P(0; ρ) = δ(ρ
0
− ρ).
The solution to this equation is the probability density of the lognormal ran-
dom process.
For t τ
0
this equation grades into the equation
∂
∂t
P(t; ρ) = D
ρ
∂
2
∂ρ
2
ρ
2
P(t; ρ), P(0; ρ) = δ(ρ
0
− ρ), (6.84)
where D
ρ
is the diffusion coefficient in ρ-space,
D
ρ
=
df (x)
dx
2
∞
Z
0
dt
0
B(t
0
) = σ
2
τ
0
df (x)
dx
2
,
and τ
0
is the temporal correlation radius of random process v(t). Equation (6.84)
can be rewritten in the form
∂
∂t
P(t; ρ) = D
ρ
∂
∂ρ
ρP(t; ρ) + D
ρ
∂
∂ρ
ρ
∂
∂ρ
ρP(t; ρ), P(0; ρ) = δ(ρ
0
− ρ).
(6.85)
General Approaches to Analyzing Stochastic Systems 169
Features of a logarithmically normal random process will be considered in
detail later, in Sect. 8.3.3, page 200.
Problem 6.3
Derive the equation for the average of the field satisfying the Burgers equation
with random shear
∂
∂t
q(x, t) +
(
q + z(t)
)
∂
∂x
q(x, t) = ν
∂
2
∂x
2
q(x, t), (6.86)
where z(t) is the stationary Gaussian process with correlation function
B(t − t
0
) =
z(t)z(t
0
)
.
Instruction Averaging Eq. (6.86), use the Furutsu–Novikov formula
h
z(t)q(x, t)
i
=
t
Z
0
dτ B(t − τ )
δ
δz(τ )
q(x, t)
,
and the expression
h
q[z(τ ) + η
1
(τ )]q[z(τ ) + η
2
(τ )]
i
= exp
t
Z
0
dτ
1
t
Z
0
dτ
2
B(τ
1
− τ
2
)
δ
2
δη
1
(τ
1
)δη
2
(τ
2
)
×
h
q[z(τ ) + η
1
(τ )]q[z(τ ) + η
2
(τ )]
i
to split correlations (see Lecture 5 and Problem 5.5, page 136) and rewrite these
formulas, in view of Eq. (6.63), in the form
h
z(t)q(x, t)
i
= −
t
Z
0
dτ B(τ )
∂
∂x
h
q(x, t)
i
,
D
q
2
(x, t)
E
= exp
2
t
Z
0
dτ
(
t − τ
)
B(τ )
∂
2
∂η
1
∂η
2
h
q(x, t + η
1
)
i h
q(x, t + η
2
)
i
|
η=0
=
∞
X
n=0
2
n
n!
t
Z
0
dτ
(
t − τ
)
B(τ )
n
∂
n
∂x
n
h
q(x, t)
i
2
.
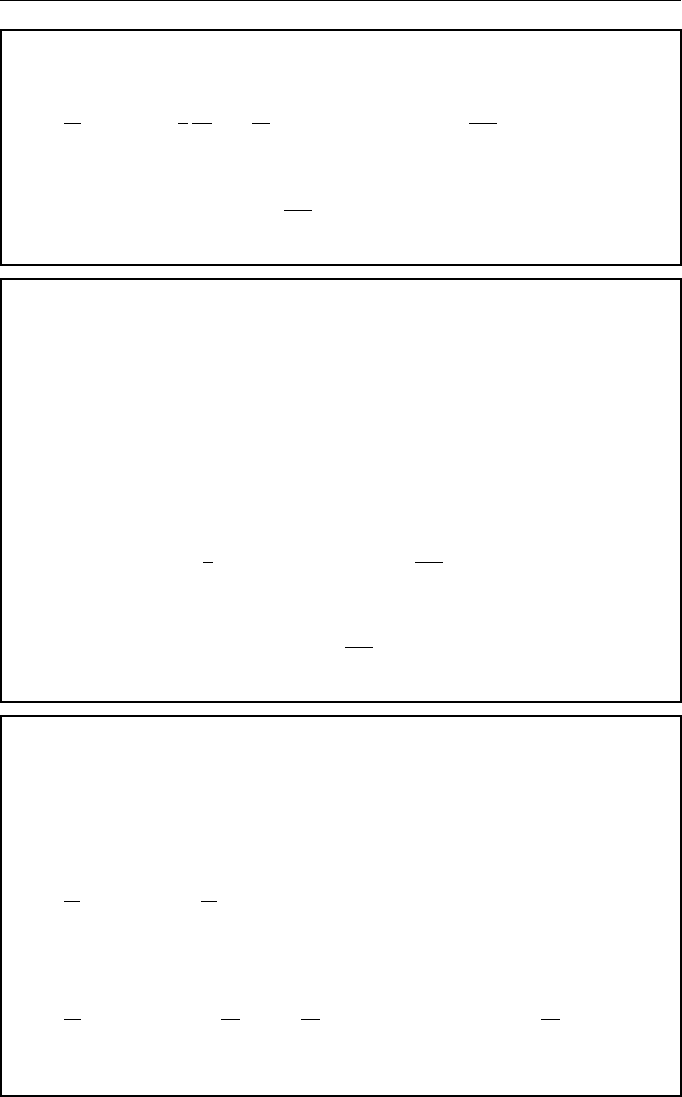
170 Lectures on Dynamics of Stochastic Systems
Solution
∂
∂t
h
q(x, t)
i
+
1
2
∂
∂x
∞
X
n=0
2
n
n!
t
Z
0
dτ (t − τ)B(τ )
n
∂
n
∂x
n
h
q(x, t)
i
2
=
ν +
t
Z
0
dτ B(τ )
∂
2
∂x
2
h
q(x, t)
i
. (6.87)
Problem 6.4
Derive Eq. (6.87) for average field starting from the one-dimensional Burger’s
equation with random drift (6.86) and Eq. (6.57), where z(t) is the stationary
Gaussian process with correlation function
B(t − t
0
) =
z(t)z(t
0
)
.
Instruction Use equality
h
q(x, t)
i
= exp
1
2
t
Z
0
dτ
1
t
Z
0
dτ
2
B(τ
1
− τ
2
)
∂
2
∂x
2
Q(x, t)
= exp
t
Z
0
dτ (t − τ )B(τ )
∂
2
∂x
2
Q(x, t).
Problem 6.5
Under the assumption that random process z(t) is delta-correlated, derive the
equation for probability density of the solution to stochastic equation (1.30),
page 19 describing the stochastic parametric resonance.
Instruction Rewrite Eq. (1.30) in the form of the system of equations
d
dt
x(t) = y(t),
d
dt
y(t) = −ω
2
0
[1 + z(t)]x(t),
x(0) = x
0
, y(0) = y
0
.
(6.88)
Solution
∂
∂t
P(x, y, t) =
−y
∂
∂x
+ ω
2
0
x
∂
∂y
P(x, y, t) +
.
2
t
t; −iω
2
0
x
∂
∂y
P(x, y, t),
P(x, y, 0) = δ(x − x
0
)δ(y − y
0
), (6.89)

General Approaches to Analyzing Stochastic Systems 171
where
.
2
t
[
t; v(τ )
]
=
d
dt
2
[
t; v(τ )
]
, 2
[
t; v(τ )
]
= ln 8
[
t; v(τ )
]
, and 8
[
t; v(τ )
]
is the characteristic functional of random process z(t).
Problem 6.6
Starting from system of equations (6.88), derive the equation for the average of
quantity
A
k
(t) = x
k
(t)y
N−k
(t) (k = 0, . . . , N)
under the assumption that z(t) is the delta-correlated random process.
Solution
d
dt
h
A
k
(t)
i
= k
h
A
k−1
(t)
i
− ω
2
0
(N − k)
h
A
k+1
(t)
i
+
N
X
n=1
1
n!
K
n
B
n
kl
h
A
l
(t)
i
(k = 0, . . . , N),
where K
n
are the cumulants of random process z(t) and matrix
B
ij
= −ω
2
0
(N − i)δ
i,j−1
.
The square of this matrix has the form
b
B
2
ij
= −ω
4
0
(N − i)(N − j + i)δ
i,j−2
and so
forth for higher powers, so that
b
B
N+1
≡ 0.
Problem 6.7
Derive the Fokker–Planck equation for system of equations (6.88) in the case of
the Gaussian delta-correlated process z(t) with the parameters
h
z(t)
i
= 0,
z(t)z(t
0
)
= 2σ
2
τ
0
δ(t − t
0
).
Solution
∂
∂t
P(x, y, t) =
−y
∂
∂x
+ ω
2
0
x
∂
∂y
P(x, y, t) + Dω
2
0
x
2
∂
2
∂y
2
P(x, y, t),
P(x, y, 0) = δ
(
x − x
0
)
δ
(
y − y
0
)
,
(6.90)
where D = σ
2
τ
0
ω
2
0
is the coefficient of diffusion in space {x, y/ω
0
}.
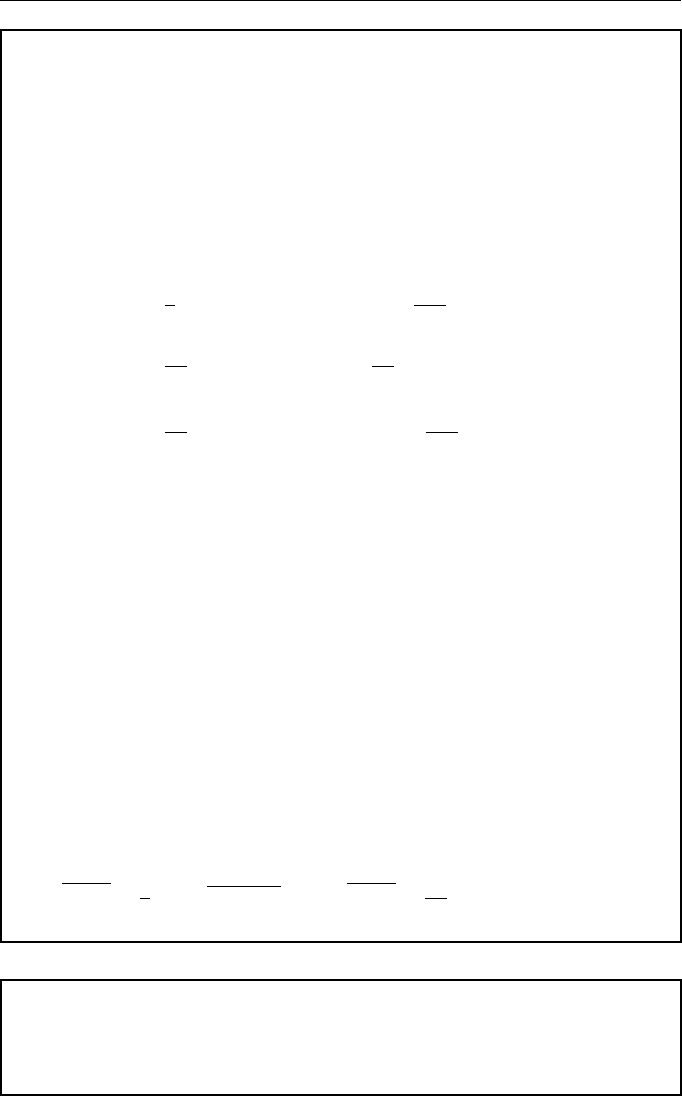
172 Lectures on Dynamics of Stochastic Systems
Problem 6.8
Obtain the solutions for the first- and second-order moments, which follow from
Eq. (6.90) for D/ω
0
1 and x(0) = 0, y(0) = ω
0
.
Solution
h
x(t)
i
= sin ω
0
t,
h
y(t)
i
= ω
0
cos ω
0
t,
which coincides with the solution to system (6.88) for absent fluctuations, and
D
x
2
(t)
E
=
1
2
e
Dt
− e
−Dt/2
cos(2ω
0
t) +
3D
4ω
0
sin(2ω
0
t)
,
h
x(t)y(t)
i
=
ω
0
4
2e
−Dt/2
sin(2ω
0
t) +
D
ω
0
h
e
Dt
− e
−Dt/2
cos(2ω
0
t)
i
,
D
y
2
(t)
E
=
ω
2
0
2
e
Dt
+ e
−Dt/2
cos(2ω
0
t) −
D
4ω
0
sin(2ω
0
t)
(6.91)
Remark Solution (6.19) includes terms increasing with time, which corresponds
to statistical parametric build-up of oscillations in a dynamic system (6.88)
because of fluctuations of frequency. Solutions to a statistical problem (6.88)
have two characteristic temporal scales t
1
∼ 1/ω
0
and t
2
∼ 1/D. The first tem-
poral scale corresponds to the period of oscillations in system (6.88) without
fluctuations (fast processes), and the second scale characterizes slow variations
caused in statistical characteristics by fluctuations (slow processes). The ratio of
these scales is small:
t
1
/t
2
= D/ω
0
1.
We can explicitly obtain slow variations of statistical characteristics of pro-
cesses x(t) and y(t) by excluding fast motions by averaging the corresponding
quantities over the period T = 2π/ω
0
. Denoting such averaging with the over-
bar, we have
x
2
(t)
=
1
2
e
Dt
,
h
x(t)y(t)
i
= 0,
y
2
(t)
=
ω
2
0
2
e
Dt
.
Problem 6.9
Starting from (6.90), derive the third-order equation for average potential energy
of an oscillator
h
U(t)
i
=
x
2
(t)
.

General Approaches to Analyzing Stochastic Systems 173
Solution
d
3
dt
3
h
U(t)
i
+ 4ω
2
0
d
dt
h
U(t)
i
− 4Dω
2
0
h
U(t)
i
= 0, (6.92)
Problem 6.10
Derive the Fokker–Planck equation for the stochastic oscillator with linear
friction
d
dt
x(t) = y(t),
d
dt
y(t) = −2γ y(t) − ω
2
0
[1 + z(t)]x(t),
x(0) = x
0
, y(0) = y
0
,
(6.93)
and the condition of stochastic excitation of second moments.
Solution
∂
∂t
P(x, y, t) =
2γ
∂
∂y
y − y
∂
∂x
+ ω
2
0
x
∂
∂y
P(x, y, t) + Dω
2
0
x
2
∂
2
∂y
2
P(x, y, t),
P(x, y, 0) = δ(x − x
0
)δ(y − y
0
),
where D = σ
2
τ
0
ω
2
0
is the diffusion coefficient. If we substitute in the system of
equations for second moments the solutions proportional to exp{λt}, we obtain
the characteristic equation in λ of the form
λ
3
+ 6γ λ
2
+ 4(ω
2
0
+ 2γ
2
)λ + 4ω
2
0
(2γ − D) = 0.
Under the condition
2γ < D, (6.94)
second moments are the functions exponentially increasing with time, which
means the statistical parametric excitation of second moments.
Problem 6.11
Obtain steady-state values of second moments formed under the action of ran-
dom forces on the stochastic parametric oscillator with friction
d
dt
x(t) = y(t), x(0) = x
0
,
d
dt
y(t) = −2γ y(t) − ω
2
0
[1 + z(t)]x(t) + f (t), y(0) = y
0
.
(6.95)
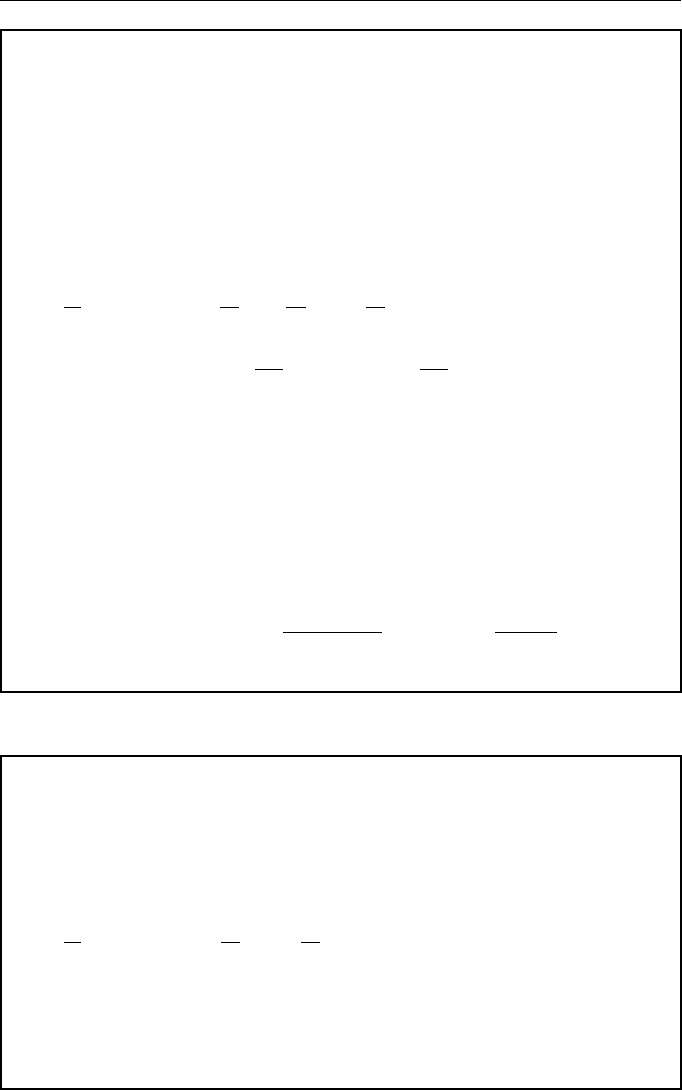
174 Lectures on Dynamics of Stochastic Systems
Here, f (t) is the Gaussian delta-correlated process statistically independent of
process z(t) and having the parameters
h
f (t)
i
= 0,
f (t)f (t
0
)
= 2σ
2
f
τ
f
δ(t − t
0
),
where σ
2
f
is the variance and τ
f
is the temporal correlation radius.
Solution In the case of stochastic system of equations (6.95), the one-time proba-
bility density satisfies the Fokker–Planck equation
∂
∂t
P(x, y, t) =
2γ
∂
∂y
y − y
∂
∂x
+ ω
2
0
x
∂
∂y
P(x, y, t)
+ Dω
2
0
x
2
∂
2
∂y
2
P(x, y, t) + σ
2
f
τ
f
∂
2
∂y
2
P(x, y, t),
P(x, y, 0) = δ
(
x − x
0
)
δ
(
y − y
0
)
.
Consequently, the steady-state solution for second moments exists for t → ∞
under the condition (6.94) and has the form
h
x(t)
i
= 0,
h
y(t)
i
= 0,
h
x(t)y(t)
i
= 0,
D
x
2
(t)
E
=
σ
2
f
τ
f
ω
2
0
(D − 2γ )
,
D
y
2
(t)
E
=
σ
2
f
τ
f
D − 2γ
.
Problem 6.12
Starting from (6.89), derive the Kolmogorov–Feller equation in the case of the
Poisson delta-correlated random process z(t).
Solution
∂
∂t
P(x, y, t) =
−y
∂
∂x
+ ω
2
0
x
∂
∂y
P(x, y, t)
+ ν
∞
Z
−∞
dξp(ξ )P(t; x, y + ξω
2
0
x) − νP(x, y, t).
(6.96)
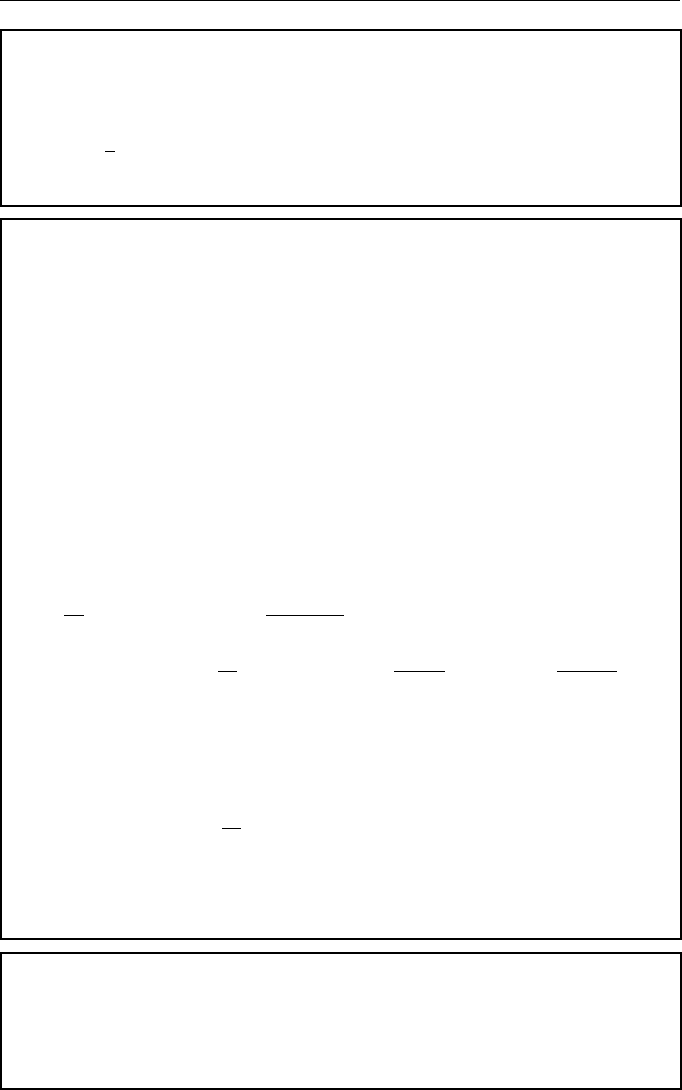
General Approaches to Analyzing Stochastic Systems 175
Remark For sufficiently small ξ , Eq. (6.96) grades into the Fokker–Planck equa-
tion (6.90) with the diffusion coefficient
D =
1
2
ν
D
ξ
2
E
ω
2
0
x.
Problem 6.13
Derive the equation for the characteristic functional of the solution to linear
parabolic equation (1.89), page 39 by averaging Eq. (3.48), page 82.
Solution Characteristic functional of the problem solution
8[x; v, v
∗
] =
ϕ[x; v, v
∗
]
,
where
ϕ[x; v, v
∗
] = exp
i
Z
dR
0
u(x, R
0
)v(R
0
)+u
∗
(x, R
0
)v
∗
(R
0
)
,
satisfies the equation
∂
∂x
8[x; v, v
∗
] =
˙
2
x
x;
δ
iδε(ξ, R
0
)
ϕ[x; v,v
∗
]
+
i
2k
Z
dR
0
v(R
0
)1
R
0
δ
δv(R
0
)
− v
∗
(R
0
)1
R
0
δ
δv
∗
(R
0
)
× 8[x; v, v
∗
], (6.97)
where
˙
2
x
x; ψ(ξ, R
0
)
=
d
dx
ln
*
exp
i
x
Z
0
dξ
Z
dR
0
ε(ξ, R
0
)ψ(ξ, R
0
)
+
is the derivative of the characteristic functional logarithm of field ε(x, R).
Problem 6.14
Starting from Eq. (6.97), derive the equation for the characteristic functional of
the solution to linear parabolic equation (1.89), page 39 assuming that ε(x, R)
is the homogeneous random field delta-correlated in x.
