Arya S.P.S. Introduction to Micrometeorology

Подождите немного. Документ загружается.

118 8 Fundamentals of Turbulence

lence

structure.

These

are

all

based

on statistical analyses of turbulent

fluctuations

observed

the

flow.

The

simplest

measures

fluctuation levels are

the

variances u

[these

three

are

often

combined

to define

the

turbulent

kinetic energy

per

unit

mass

!(u

+ v

+ w

)

()2,

etc.,

and

standard

deviations

= (U

2)1/2,

etc.

The

ratios

standard

deviations of velocity fluctuations to the mean

wind

speed

are

called

turbulence

intensities (e.g., i; = CTjU, i

= CTjU

and

i.; =

w/U),

which

are

measures

of relative fluctuation levels in

different directions (velocity

components).

Observations

indicate that tur-

bulence

intensities

are

typically less

than

10% in

the

nocturnal boundary

layer,

10-20%

in a

near-neutral

surface layer, and

greater

than 20% in

unstable

and

convective

boundary

layers.

Turbulence

intensities are gen-

erally

largest

near

the

surface

and

decrease

with height in stable and near-

neutral

boundary

layers.

Under

convective

conditions, however, the sec-

ondary

maxima

turbulence

intensities often

occur

at the inversion base

and

sometimes

the

middle

the

CBL.

Even

important

turbulent

flows

are

the

covariances

UW,

()w,

etc.,

which

are

directly

and

sometimes referred to as turbulent

fluxes

momentum,

heat,

etc. As

covariances,

they

are averages of

products

two

fluctuating variables

and

depend

on the correlations be-

tween

the

variables involved.

These

can

be positive, negative, or zero,

depending

the

type

of flow

and

symmetry

conditions.

For

example, if U

and

ware

the

velocity fluctuations in

the

and

z directions, respectively,

their

product

will also be a fluctuating quantity but with a nonzero

mean;

uw will be positive if u

and

ware

positively correlated,

and

nega-

tive if

the

two

variables

are

negatively correlated. A

better

measure

of the

correlation

between

two

variables is

provided

by the correlation coeffi-

cient

(8.3)

whose

value always lies

between

-1

and

according to

Schwartz's

inequality luw I"S

CTuCT

Similarly,

one

can

define correlation coefficients

for all

the

covariances

to get an

idea

how

well correlated different

variables

are

in a

turbulent

flow.

There

is still some

order

to be found in an

apparently

chaotic

motion

which is responsible for all the important ex-

change

processes

taking place in

the

flow.

order

to see a

clear

connection

between

covariances and turbulent

fluxes, let us

consider

the

vertical flux

a scalar with a variable concen-

tration (mass

per

unit

volume) c in

the

flow.

The

flux of any scalar in a

given direction is defined as

the

amount

the scalar

per

unit time

per

unit

area

normal to

that

direction.

is quite obvious

that

the

velocity compo-

nent

the

direction

flux is responsible for

the

transport

and

hence for

8.6 Eddies and Scales of Motion

119

the flux.

For

example, it is

easy

to see that in the vertical direction the flux

at any instant is

CIV

and the mean flux with which we are normally con-

cerned is

CIV.

Further

writing C = C + c and

= W +

wand

following

the Reynolds averaging rules, it

can

be shown that

('vi;' = (C +

c)(W

+ w) =

(8.4)

Thus, the total scalar flux

can

be represented as a sum

the mean

transport (transport by mean motion) and the turbulent transport. The

latter, also called the turbulent flux, is usually the dominant transport

term and always of considerable importance in a turbulent flow. If the

scalar

under

consideration is the potential temperature

or enthalpy

pc/),

the corresponding turbulent flux in the vertical is Ow, or

pCpOw.

this way,

the

covariance Ow may be interpreted as the turbulent heat flux

(in kinematic units). Similarly,

uw may be considered as the vertical tur-

bulent flux of horizontal

direction) momentum or, equivalently, the

horizontal

(x direction) flux of vertical momentum, as i,w = wu.

According to

Newton's

second law of motion the rate of change (flux)

of momentum is equal to force

per

unit area or stress, so that one can also

express turbulent stresses as

-puw,

= r

puw,

etc.

(8.5)

These are also called Reynolds stresses, which in most turbulent flows are

found to have much larger magnitudes than the corresponding mean vis-

cous stresses

J.L(aUiaz

+ aWlax),

J.L(aVlaz

+ a

Wlay)

, etc. The latter can

usually be ignored,

except

within the extremely thin viscous sublayers.

8.6

EDDIES

AND

SCALES OF

MOTION

is a

common

practice to speak in terms

eddies when turbulence is

described qualitatively. An eddy is by no means a clearly defined struc-

ture or feature of the flow which

can

be isolated and followed through, in

order

to study its behavior.

is rather an abstract concept used mainly

for qualitative descriptions of turbulence. An eddy may be considered

akin to a vortex or a whirl in common terminology. Turbulent flows are

highly rotational

and

have all kinds of vortexlike structures (eddies) bur-

ied in them. However, eddies are not simple two-dimensional circulatory

motions of the type in an isolated vortex, but are believed to be complex,

three-dimensional structures. Any analogy between turbulent eddies and

vortices

can

only be very rough and qualitative.

On the basis

flow visualization studies, statistical analyses of turbu-

lence data,

and

some of the well-accepted theoretical ideas, it is believed

120 8 Fundamentals of Turbulence

that a turbulent flow consists of a hierarchy of eddies of a wide range of

sizes (length scales), from the smallest

that

can survive the dissipative

action of viscosity to the largest that is allowed by the flow geometry. The

range of

eddy

sizes increases with the Reynolds number of the overall

mean flow.

In particular, for the ABL, the typical range of eddy sizes is

10-

to 10

Of the wide

and

continuous range of scales in a turbulent flow, a few

have a special significance and are used to characterize the flow itself. The

one is the characteristic large-eddy scale or macroscale of turbulence,

which represents the length

(I)

or time scale of eddies receiving the most

energy from the mean flow. The

other

is the characteristic small-eddy

scale or microscale of turbulence, which represents the length

(YJ)

or time

scale of

most

dissipating eddies. The ratio

l/YJ

is found to be proportional

to Re

and is typically 10

for the atmospheric

PBL.

The macroscale, or simply the scale of turbulence, is generally compa-

rable (in

order

of magnitude) to the characteristic scale of the mean flow,

such as the boundary layer thickness or channel depth, and does not

depend on the molecular properties of the fluid. On the other hand, the

microscale depends on the fluid viscosity

u, as well as on the rate of

energy dissipation

e. Dimensional considerations further lead to the defin-

ing relationship

(8.6)

In stationary or steady-state conditions, the rate at which the energy is

dissipated is exactly equal to the rate at which the energy is supplied from

mean flow into turbulence. This leads to an inviscid estimate for the

energy dissipation

/l, where u is the characteristic velocity scale of

turbulence (large eddies), which

can

be defined in terms of the turbulent

kinetic energy

(8.7)

Some investigators define the turbulence velocity scale such that iu

rep-

resents the turbulent kinetic energy (e.g., Tennekes and Lumley, 1972).

has

been

recognized for a long time that in large Reynolds number

flows almost all

the energy is supplied to large eddies, while almost all

of it is eventually dissipated by small eddies. The transfer of energy from

large (energy-containing) to small (energy-dissipating) eddies occurs

through a cascade-type process involving the whole range of intermediate

size eddies. This idea was originally suggested by Lewis Richardson in

1922 in the form

a parody

Problems and Exercises

121

Big whorls have little whorls,

Which feed on their velocity;

And little whorls have lesser whorls,

And so on to viscosity.

This may be considered to be the qualitative picture of turbulence struc-

ture in a nutshell and the concept of energy transfer down the scale. The

actual mechanism and quantitative aspects of energy transfer are very

complicated

and

are outside the scope of this text. Smaller eddies are

assumed to be created through an instability and breakdown of larger

eddies and the energy transfer from larger to smaller eddies presumably

occurs during the breakdown process. Since small eddies are created after

many successive breakdowns of larger and larger eddies, it can be argued

that the former are very far removed from the initial process of the gener-

ation of macroscale turbulence by the mean flow and, hence, can have no

preferred orientation with respect to the direction of mean shear or buoy-

ancy. Thus, the small-scale structure may be expected to have some

universal attributes common to all turbulent flows. This idea of universal

similarity

and

isotropy of small-scale turbulence was first expounded by

A. N. Kolmogorov in

1941

(see Monin and Yaglom, 1971)and has become

the cornerstone of theory and observations of turbulence.

8.7

APPLICATIONS

A knowledge of the fundamentals of turbulence is essential to any

qualitative or quantitative understanding of the turbulent exchange pro-

cesses occurring in the

PBL

as well as in the free atmosphere. The statisti-

cal descriptions in terms of variances and covariances of turbulent fluctu-

ations, as well as in terms of eddies and scales of motion, provide the

bases for further quantitative analyses of observations. At the same time

the basic concepts of the generation and maintenances of turbulence, of

supply, transfer,

and

dissipation

energy, and of local similarity are the

foundation stones of turbulence theory.

PROBLEMS

AND

EXERCISES

1. Discuss the various types of instability mechanisms that might be oper-

ating in the atmosphere and their possible effects on atmospheric mo-

tions.

2. If you have available only the upper air velocity and temperature

122 8 Fundamentals of Turbulence

soundings,

how

would you use this information to determine which

layers

the

atmosphere

might be turbulent?

3. (a) What are the possible mechanisms of generation and maintenance

turbulence in the atmosphere?

(b) What criteria may be used to determine if turbulence in a stably

stratified layer could be maintained or if it would decay?

What

are

the characteristics

turbulence that distinguish it from a

random

wave

motion?

Compare

and

contrast

the time and ensemble averages. Under what

conditions might the two types

averages be equivalent?

6. What is the possible range

turbulence intensities in the atmosphere

and

under

what

conditions might the extreme values occur'?

7. Give all

the

components

of the Reynolds stress and indicate which of

these are really independent.

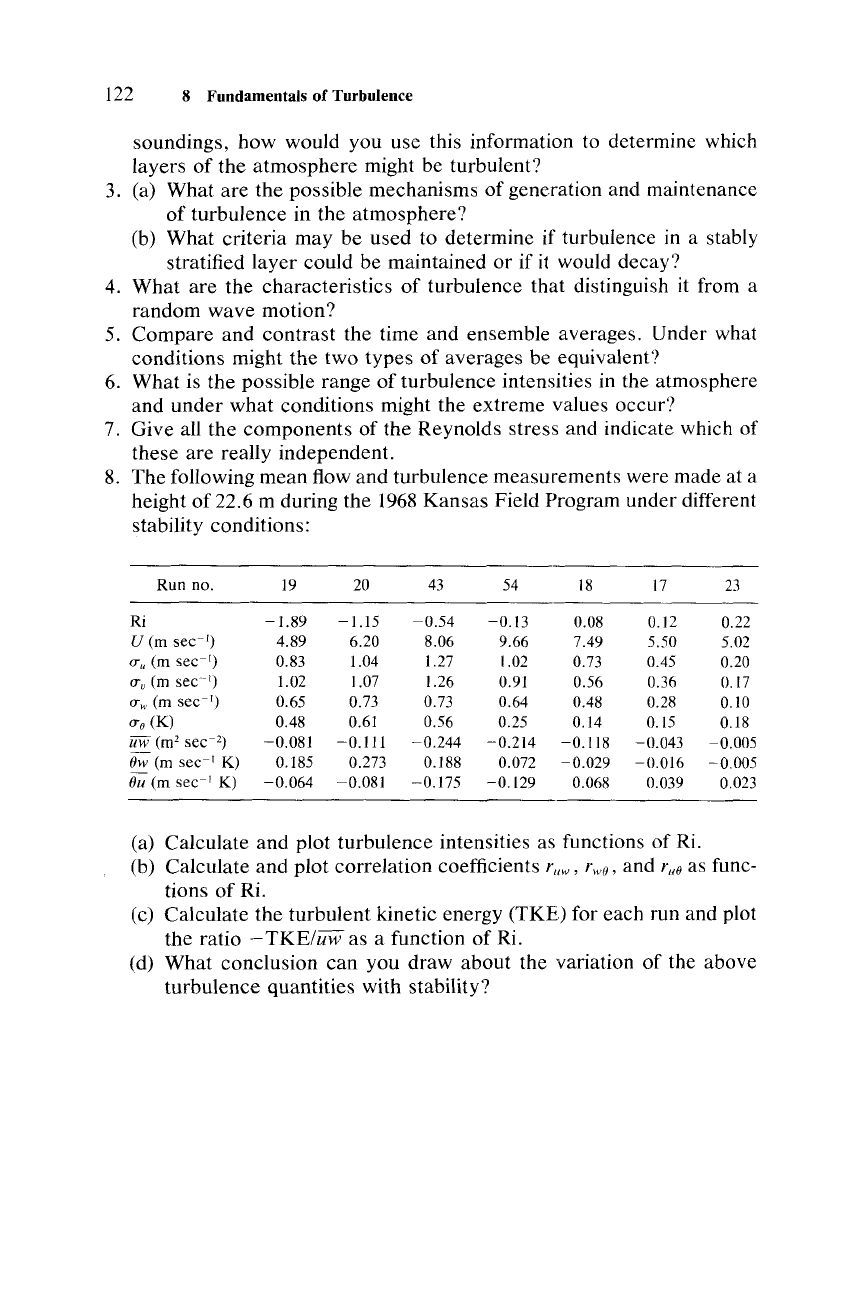
8. The following mean flow and turbulence measurements were made at a

height

of22.6

m during the 1968 Kansas Field Program under different

stability conditions:

Run no.

19 20

54 18

17 23

-1.89

-1.15

-0.54

-0.13

0.08

0.12

0.22

U (m

sec'")

4.89

6.20

8.06 9.66 7.49 5.50

5.02

sec

)

0.83

1.04 1.27

1.02 0.73 0.45

0.20

(T,

sec'")

1.02 1.07 1.26

0.91 0.56 0.36 D.17

(Tw

sec

)

0.65 0.73 0.73 0.64

0.48 0.28

0.10

(To

(K)

0.48

0.61 0.56

0.25 0.14 0.15 0.18

(m'

sec')

-0.081 -0.111

-0.244 -0.214

-0.118

-0.043

-0.005

Ow (m sec

0.185 0.273 0.188

0.072

-0.029 -0.016

-0.005

Ou (m

sec-

-0.064

-0.081

-0.175

-0.129

0.068 D.039 0.023

(a) Calculate and plot turbulence intensities as functions of Ri.

(b) Calculate and plot correlation coefficients

w8,

and r

as func-

tions

Ri.

the ratio

-TKE/uw

as a function

Ri.

(d) What conclusion

can

you

draw

about the variation of the above

turbulence quantities with stability?

Chapter 9

Semiempirical Theories of Turbulence

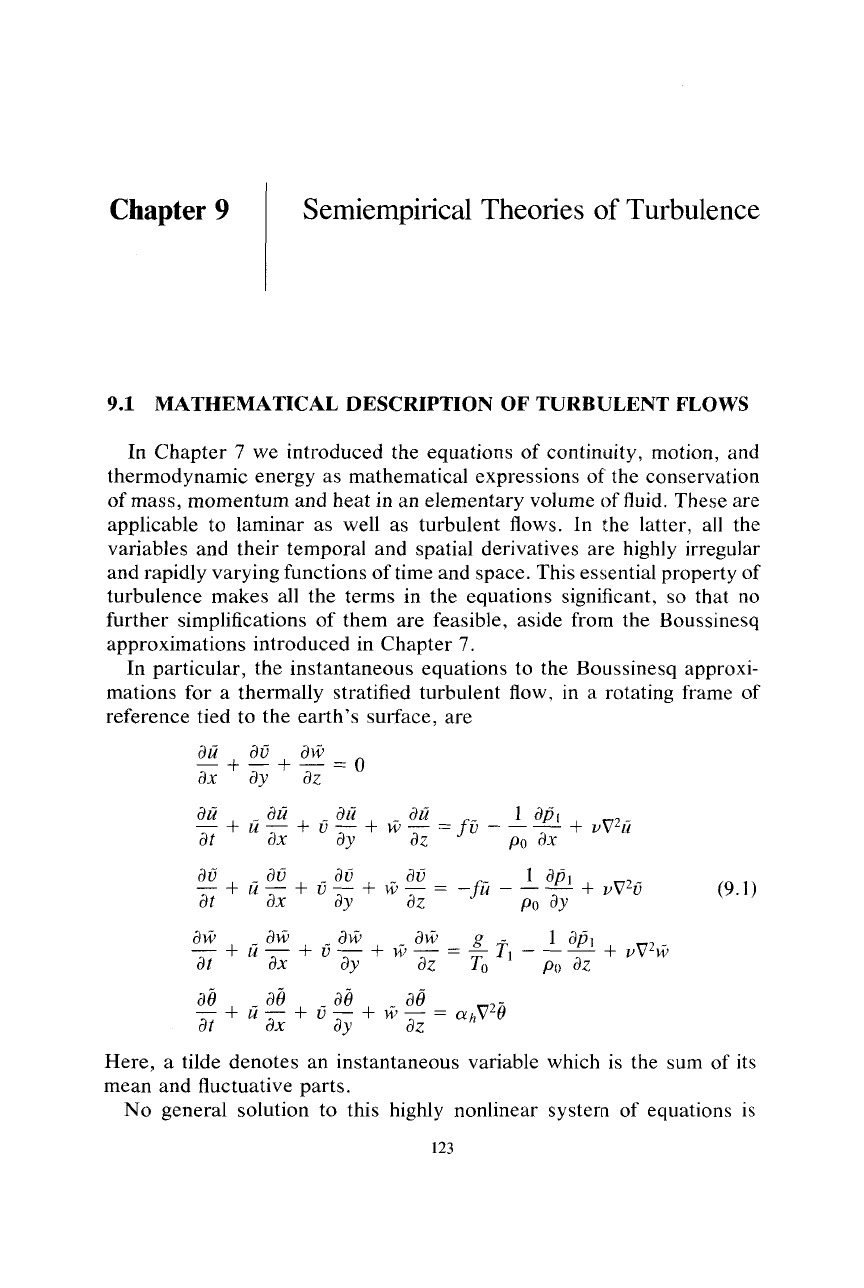
9.1

MATHEMATICAL

DESCRIPTION

TURBULENT

FLOWS

Chapter

7 we introduced the equations of continuity, motion, and

thermodynamic energy as mathematical expressions of the conservation

of mass, momentum and heat in an elementary volume of fluid. These are

applicable to laminar as well as turbulent flows. In the latter, all the

variables and their temporal and spatial derivatives are highly irregular

and rapidly varying functions of time and space. This essential property of

turbulence makes all the terms in the equations significant, so that no

further simplifications of them are feasible, aside from the Boussinesq

approximations introduced in

Chapter

In particular, the instantaneous equations to the Boussinesq approxi-

mations for a thermally stratified turbulent flow, in a rotating frame of

reference tied to the

earth's

surface, are

-+-+-=

_ 1

ap,

- + u - + v - + w - =

- - - + v'i/

_ 1

ap]

- + u - + v - + w - =

-fu

- - - + vV

(9.1)

g - 1

ap]

- + u - + v - + w - = - T] - - - + vV

ae ae

- + it - + u- + w- = Cl:hv

Here,

a tilde denotes an instantaneous variable which is the sum of its

mean and f1uctuative parts.

No general solution to this highly nonlinear system of equations is

123

124 9 Semiempirical Theories of Turbulence

known and there appears to be no hope of finding one through purely

analytical methods.

9.1.1

FULL-TURBULENCE

SIMULATION

In this age of computers, one may think of solving the Navier-Stokes

equations using the brute force method of numerical integration on large

computers. This has

been

called as full-turbulence simulation (FTS) and

has

been

attempted for very low Reynolds number flows. The amount of

computational effort to make a fundamental attack on most turbulent

flows

practical interest is simply prohibitive and outside the range of

even the most sophisticated supercomputers of today. The difficulty

comes from the fact

that

the number of grid points required to resolve all

the turbulent eddies in a flow is of the order of

(lIYJ)3

where

lIYJ

is the ratio

of the largest to the smallest eddy scales. This number is simply mind

boggling (10

2-10

)

for atmospheric turbulent flows and also too large to

be handled for most

other

flows of practical interest. A rough estimate of

the

computer

time required for a full-turbulence simulation of the daytime

PBL

using the latest Cray X-MP machine is millions of years! Still, certain

low Reynolds number flows with

liT)

have been computed this way

and, in the

not

too distant future,

other

turbulent flows of more practical

interest might become amenable to the FTS approach, although still at a

great cost.

9.1.2

LARGE-EDDY

SIMULATION

A more feasible but less fundamental computational approach which

has

been

used in meteorology and engineering fluid mechanics is large-

eddy simulation (LES). This approach attempts to faithfully simulate only

the scales of motion in a certain range (between the smallest grid size and

the dimensions

simulated flow domain), while the unresolved subgrid

scale motions are ignored altogether or are parameterized. The origins of

the

LES

technique lie in the early global weather prediction and general

circulation models in which the permissible grid spacing could hardly

resolve large-scale atmospheric structures.

Large-eddy simulations

turbulent flows, including the PBL, started

with the pioneering work of Deardorff(1970, 1973).The soundness of this

modeling approach in simulating neutral and unstable PBLs, even in the

presence of moist convection and clouds, has been amply demonstrated

by subsequent studies of Deardorff and others. A pressing need is the

extension

the

LES

to the nocturnal boundary layer, including the

morning and evening transition periods, which are poorly understood.

The main difficulty in simulating the stably stratified boundary layer is

9.1 Description of Turbulent Flows

125

that

the

characteristic

large-eddy scale

(I)

becomes too small and most of

the

energy

transfer

and

other

exchange

processes

are overly influenced

dominated

by subgrid scale motions. A successful

LES

simulation of the

NBL

and

transition

processes

should

become

possible in the

near

future,

powerful next-generation

computers

become available.

The

computational

details

large-eddy simulation are outside the

scope

this

introductory

text.

should suffice to say

that

LES

is viewed

as the

most

promising tool for future

research

in turbulence and is ex-

pected

provide

better

understanding

the

transport

phenomena,

well

beyond

the

reach

semiempirical theories

and

most routine obser-

vations. Since it requires an

investment

huge

computer

resources

(there are also a few

other

limitations),

LES

would most likely remain a

research

tool.

would be

very

useful,

course, for generating numerical

turbulence

data

for studying large-eddy

structures,

developing parameter-

izations for simpler models,

and

designing physical experiments (Wyn-

gaard, 1984).

9.1.3

EQUATIONS

MEAN

MOTION

Full-turbulence simulation (FTS) is

based

on the

"primitive"

Navier-

Stokes

equations

motion.

The

large-eddy simulation utilizes the same

equations,

but

averaged

over

the finite grid volume. Numerical integra-

tions

both

systems

yield highly irregular (turbulent) variables as func-

tions of time

and

space. Average statistics, such as means, variances, and

higher

moments,

are

then

calculated from this

"simulated

turbulence"

data. In

most

applications,

however,

only the average statistics are

needed

and

how

they

come

about

from turbulent fluctuations is usually of

little or no

concern.

One

may

ask

"Why

not

use

the

equations

mean

motion to

compute

the

desired average

statistics?"

This question was first

addressed

Osborne

Reynolds toward the end of the nineteenth cen-

tury.

also suggested some simple averaging rules or conditions and

derived

what

came

to be

known

as the Reynolds-averaged equations.

and

are

two

dependent

variables or functions

variables with

mean

valuesJ

and

c is a

constant,

the Reynolds averaging condi-

tions

are

f+g=J+g

;

aflas =

aJlas;

=Jg

If ds = IJds

(9.2)

where

s = x,

v.z,

These

conditions are used in deriving the equations

for

mean

variables from

those

the instantaneous variables. Since, these

126 9 Semiempirical Theories of Turbulence

are strictly satisfied only by ensemble averaging, this type of averaging is

generally implied in theory. The time and space averages often used in

practice can satisfy the Reynolds averaging rules only under certain ideal-

ized conditions (e.g., stationarity and homogeneity of flow), which were

discussed in

Chapter

The usual procedure for deriving Reynolds-averaged equations is to

substitute in

Eq.

(9.1) u = U + u,

()

= V + v, etc., and take their aver-

ages using the Reynolds averaging conditions [Eq.

(9.2)]. Consider first

the continuity equation

a(U

+ u)/ax +

a(V

+ v)/ay + a(W + w)/az = 0 (9.3)

which, after averaging, gives

aU/ax

av/ay

aw/az

= 0 (9.4)

Subtracting Eq. (9.4) from (9.3), one obtains the continuity equation for

the

fluctuating motion

au/ax + av/ay + aw/az = 0

(9.5)

Thus, the form of continuity equation remains the same for instantaneous,

mean, and fluctuating motions. This is not the case, however, for the

equations of conservation of momentum and heat, because of the pres-

ence

nonlinear advection terms in those equations.

Let

us consider, for example, the advection terms in the instantaneous

equation of the conservation of heat

tie = u(ao/ax) + ()(aiJ/ay) + w(ae/az) (9.6a)

which, after utilizing the continuity equation, can also be written in the

form

tie = (a/ax)(uO) + (a/ay)(()O) +

(a/a,,:)(wO)

(9.6b)

Expressing variables as sums of their mean and fluctuating parts in Eq.

(9.6b) and averaging,

one

obtains the Reynolds-averaged advection terms

a a a

-cue)

+ - (ve) + - (we)

ax ay

a--

+ - (uO) +

-(vO)

+ - (wO)

ax ay az

or, after using the mean continuity equation,

(9.7a)

ae ae ae a - a

+ - (uO) + - (ve) + - (wO) (9.7b)

ax ay

9.1 Description

Turbulent Flows 127

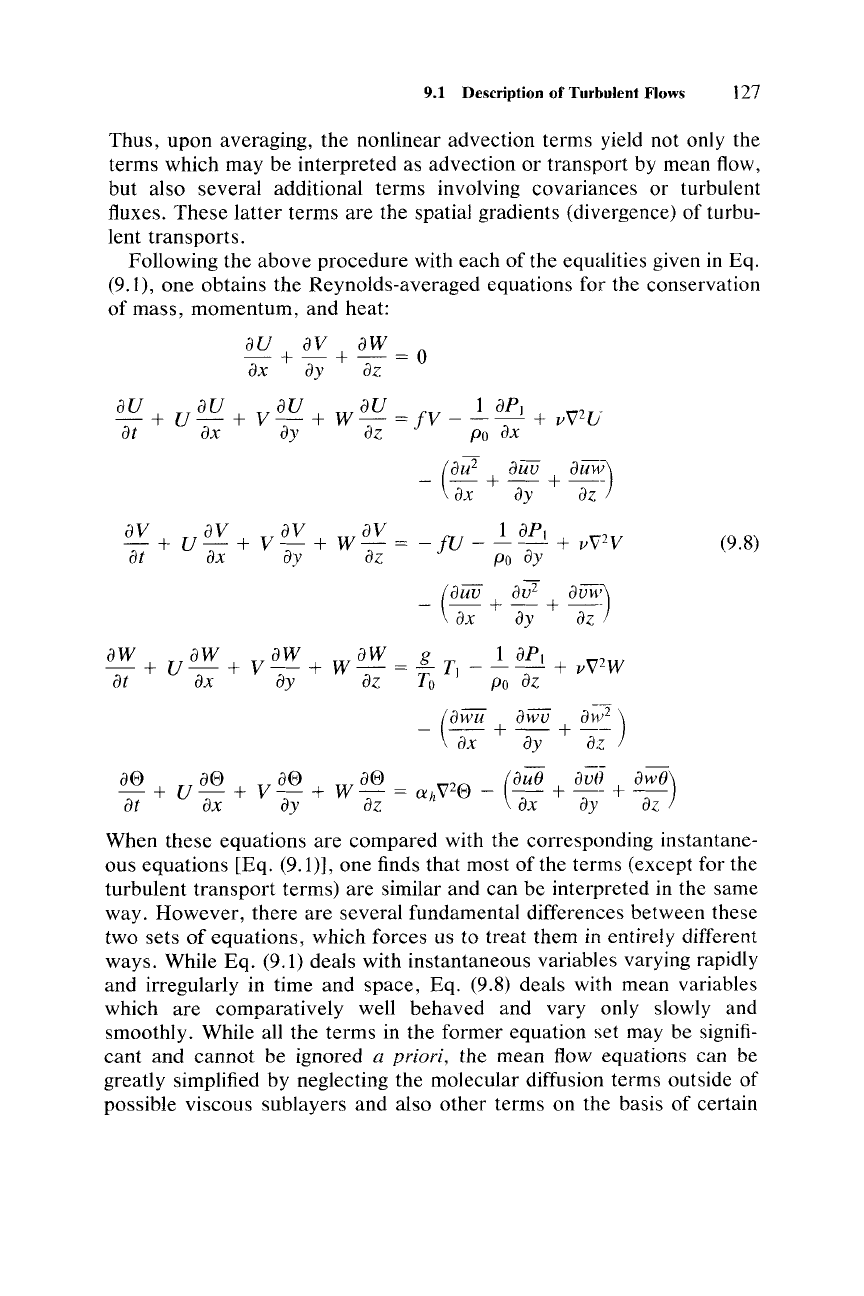
Thus, upon averaging, the nonlinear advection terms yield not only the

terms which may be interpreted as advection or transport by mean flow,

but also several additional terms involving covariances or turbulent

fluxes.

These

latter

terms are the spatial gradients (divergence) of turbu-

lent transports.

Following the above procedure with each of the equalities given in Eq.

(9.1), one obtains the Reynolds-averaged equations for the conservation

of mass, momentum, and heat:

au + av + aw = 0

u au + v au + w au = jV _

ap]

vV2u

(au

auv

aUl1~

ax ay

az-)

av av av av 1

aPt

- + u - + v - + w - = -

- - - + vy

ax ay

(9.8)

(auv

aVI1~

az-;

aw + u aw + v aw+ w aw=

.K-

T] _

aPt

vV2w

(awu

awv

al;~)

ax ay

a0 + u a0 + v a0 + w a0 = (Xh

V20

(aue

aVi~

awe)

When these equations are compared with the corresponding instantane-

ous equations [Eq. (9.1)], one finds that most of the terms (except for the

turbulent

transport

terms) are similar and

can

be interpreted in the same

way. However, there are several fundamental differences between these

two sets

equations, which forces us to treat them in entirely different

ways. While Eq. (9.1) deals with instantaneous variables varying rapidly

and irregularly in time and space, Eq. (9.8) deals with mean variables

which are comparatively well behaved and vary only slowly and

smoothly. While all the terms in the former equation set may be signifi-

cant

and

cannot

be ignored a priori, the mean flow equations can be

greatly simplified by neglecting the molecular diffusion terms outside of

possible viscous sublayers

and

also other terms on the basis of certain