ASM Metals HandBook Vol. 8 - Mechanical Testing and Evaluation

Подождите немного. Документ загружается.

Fig. 4 Rimmed 1008 steel with Lüders bands on the surface as a result of stretching the

sheet just beyond the yield point during forming

Measures of Ductility. Currently, ductility is considered a qualitative, subjective property of a material. In

general, measurements of ductility are of interest in three respects (Ref 1):

• To indicate the extent to which a metal can be deformed without fracture in metalworking operations,

such as rolling and extrusion

• To indicate to the designer the ability of the metal to flow plastically before fracture. A high ductility

indicates that the material is “forgiving” and likely to deform locally without fracture should the

designer err in the stress calculation or the prediction of severe loads.

• To serve as an indicator of changes in impurity level or processing conditions. Ductility measurements

may be specified to assess material quality, even though no direct relationship exists between the

ductility measurement and performance in service.

The conventional measures of ductility that are obtained from the tension test are the engineering strain at

fracture (e

) (usually called the elongation) and the reduction in area at fracture (q). Elongation and reduction in

area usually are expressed as a percentage. Both of these properties are obtained after fracture by putting the

specimen back together and taking measurements of the final length, L

, and final specimen cross section, A

(Eq 5)

(Eq 6)

Because an appreciable fraction of the plastic deformation will be concentrated in the necked region of the

tension specimen, the value of e

will depend on the gage length (L

) over which the measurement was taken

(see the section of this article on ductility measurement in tension testing). The smaller the gage length, the

greater the contribution to the overall elongation from the necked region and the higher the value of e

Therefore, when reporting values of percentage elongation, the gage length should always be given.

Reduction in area does not suffer from this difficulty. These values can be converted into an equivalent zero-

gage-length elongation (e

). From the constancy of volume relationship for plastic deformation, AL = A

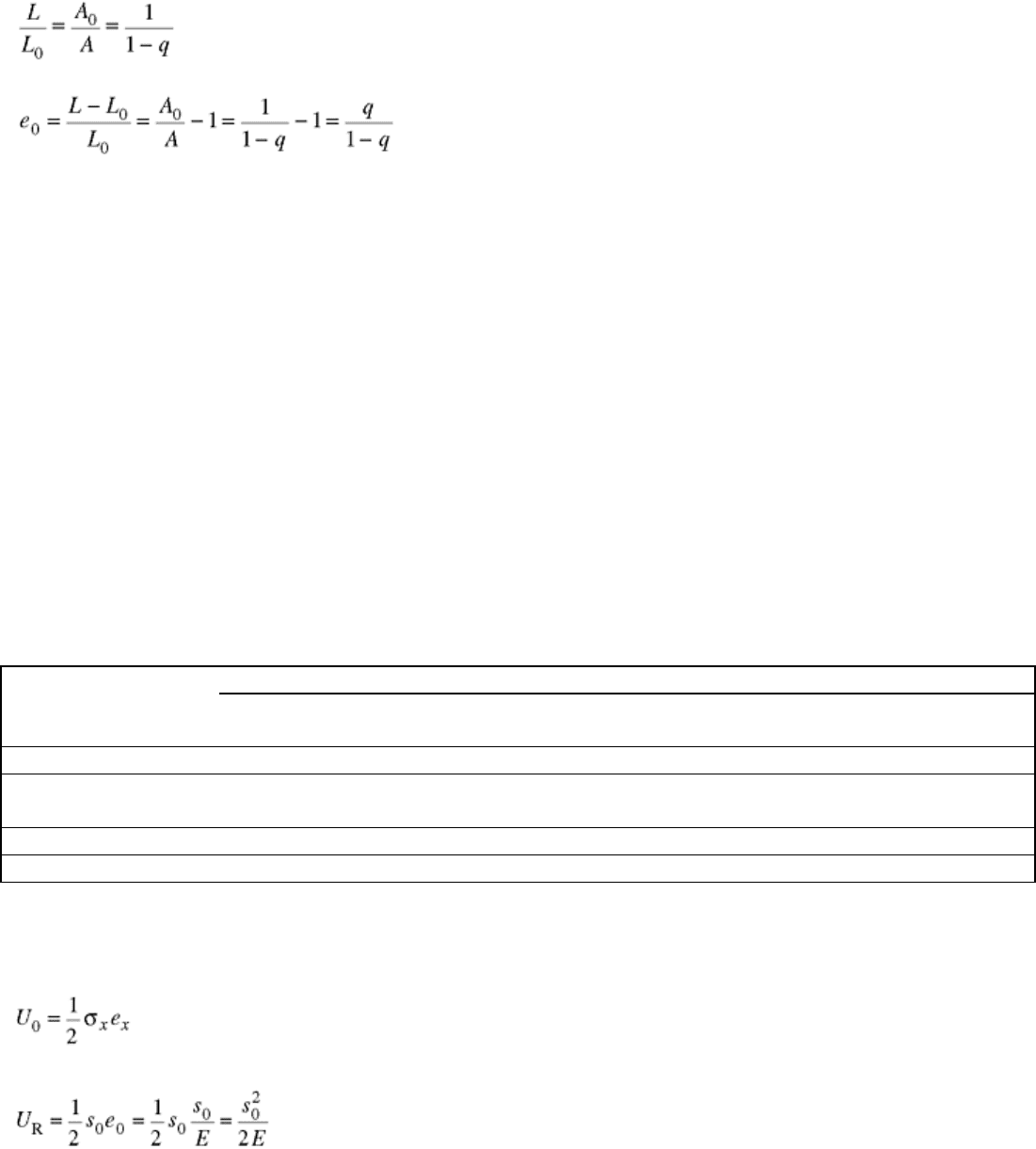
(Eq 7)

This represents the elongation based on a very short gage length near the fracture.

Another way to avoid the complications resulting from necking is to base the percentage elongation on the

uniform strain out to the point at which necking begins. The uniform elongation (e

), correlates well with

stretch-forming operations. Because the engineering stress-strain curve often is quite flat in the vicinity of

necking, it may be difficult to establish the strain at maximum load without ambiguity. In this case, the method

suggested in Ref 2 is useful.

Modulus of Elasticity. The slope of the initial linear portion of the stress-strain curve is the modulus of

elasticity, or Young's modulus, as shown in Fig. 3. The modulus of elasticity (E) is a measure of the stiffness of

the material. The greater the modulus, the smaller the elastic strain resulting from the application of a given

stress. Because the modulus of elasticity is needed for computing deflections of beams and other members, it is

an important design value.

The modulus of elasticity is determined by the binding forces between atoms. Because these forces cannot be

changed without changing the basic nature of the material, the modulus of elasticity is one of the most

structure-insensitive of the mechanical properties. Generally, it is only slightly affected by alloying additions,

heat treatment, or cold work (Ref 3). However, increasing the temperature decreases the modulus of elasticity.

At elevated temperatures, the modulus is often measured by a dynamic method (Ref 4). Typical values of the

modulus of elasticity for common engineering metals at different temperatures are given in Table 1.

Table 1 Typical values of modulus of elasticity at different temperature

Modulus of elasticity GPa (10

psi), at: Material

Room

temperature

250 °C (400

°F)

425 °C (800

°F)

540 °C (1000

°F)

650 °C (1200

°F)

Carbon steel 207 (30.0) 186 (27.0) 155 (22.5) 134 (19.5) 124 (18.0)

Austenitic stainless

steel

193 (28.0) 176 (25.5) 159 (23.0) 155 (22.5) 145 (21.0)

Titanium alloys 114 (16.5) 96.5 (14.0) 74 (10.7) 70 (10.0) …

Aluminum alloys 72 (10.5) 65.5 (9.5) 54 (7.8) … …

Resilience. The ability of a material to absorb energy when deformed elastically and to return it when unloaded

is called resilience. This property usually is measured by the modulus of resilience, which is the strain energy

per unit volume (U

) required to stress the material from zero stress to the yield stress (σ

). The strain energy

per unit volume for uniaxial tension is:

(Eq 8)

From the above definition, the modulus of resilience (U

) is:

(Eq 9)

This equation indicates that the ideal material for resisting energy loads in applications where the material must

not undergo permanent distortion, such as in mechanical springs, is one having a high yield stress and a low

modulus of elasticity.

For various grades of steel, the modulus of resilience ranges from 100 to 4500 kJ/m

(14.5–650 lbf · in./in.

with the higher values representing steels with higher carbon or alloy contents (Ref 5). The cross-hatched

regions in Fig. 5 indicate the modulus of resilience for two steels. Due to its higher yield strength, the high-

carbon spring steel has the greater resilience.

Fig. 5 Comparison of stress-strain curves for high- and low-toughness steels. Cross-

hatched regions in this curve represent the modulus of resilience (U

) of the two

materials. The U

is determined by measuring the area under the stress-strain curve up to

the elastic limit of the material. Point A represents the elastic limit of the spring steel;

point B represents that of the structural steel.

The toughness of a material is its ability to absorb energy in the plastic range. The ability to withstand

occasional stresses above the yield stress without fracturing is particularly desirable in parts such as freight-car

couplings, gears, chains, and crane hooks. Toughness is a commonly used concept that is difficult to precisely

define. Toughness may be considered to be the total area under the stress-strain curve. This area, which is

referred to as the modulus of toughness (U

) is an indication of the amount of work per unit volume that can be

done on the material without causing it to rupture.

Figure 5 shows the stress-strain curves for high- and low-toughness materials. The high-carbon spring steel has

a higher yield strength and tensile strength than the medium-carbon structural steel. However, the structural

steel is more ductile and has a greater total elongation. The total area under the stress-strain curve is greater for

the structural steel; therefore, it is a tougher material. This illustrates that toughness is a parameter that

comprises both strength and ductility.

Several mathematical approximations for the area under the stress-strain curve have been suggested. For ductile

metals that have a stress-strain curve like that of the structural steel, the area under the curve can be

approximated by:

≈ s

(Eq 10)

(Eq 11)

For brittle materials, the stress-strain curve is sometimes assumed to be a parabola, and the area under the curve

is given by:

(Eq 12)

Typical Stress-Strain Curves. Figure 6 compares the engineering stress-strain curves in tension for three

materials. The 0.8% carbon eutectoid steel is representative of a material with low ductility. The annealed 0.2%

carbon mild steel shows a pronounced upper and lower yield point. The polycarbonate engineered polymer has

no well-defined linear modulus, and a large strain to fracture. Note the pronounced difference in stress level at

which yielding is defined, as well as the quite different shape of the stress-strain curves.

Fig. 6 Typical engineering stress-strain curves

Footnote

Reprinted in part from Mechanical Metallurgy, 3rd ed., McGraw-Hill, New York, 1986, p 275–

295, with

permission

References cited in this section

1. G.E. Dieter, Introduction to Ductility, in Ductility, American Society for Metals, 1968

2. P.G. Nelson and J. Winlock, ASTM Bull., Vol 156, Jan 1949, p 53

3. D.J. Mack, Trans. AIME, Vol 166, 1946, p 68–85

4. P.E. Armstrong, Measurement of Elastic Constants, in Techniques of Metals Research, Vol V, R.F.

Brunshaw, Ed., Interscience, New York, 1971

5. H.E. Davis, G.E. Troxell, and G.F.W. Hauck, The Testing of Engineering Materials, McGraw-Hill, New

York, 1964, p 33

Mechanical Behavior Under Tensile and Compressive Loads*

George E. Dieter, University of Maryland

True Stress-True Strain Curve

The engineering stress-strain curve does not give a true indication of the deformation characteristics of a metal

because it is based entirely on the original dimensions of the specimen, and these dimensions change

continuously during the test. Also, ductile metal that is pulled in tension becomes unstable and necks down

during the course of the test. Because the cross-sectional area of the specimen is decreasing rapidly at this stage

in the test, the load required to continue deformation falls off.

The average stress based on the original area likewise decreases, and this produces the fall-off in the

engineering stress-strain curve beyond the point of maximum load. Actually, the metal continues to strain

harden to fracture, so that the stress required to produce further deformation should also increase. If the true

stress, based on the actual cross-sectional area of the specimen, is used, the stress-strain curve increases

continuously to fracture. If the strain measurement is also based on instantaneous measurement, the curve that

is obtained is known as true stress-true strain curve. This is also known as a flow curve because it represents the

basic plastic-flow characteristics of the material.

Any point on the flow curve can be considered the yield stress for a metal strained in tension by the amount

shown on the curve. Thus, if the load is removed at this point and then reapplied, the material will behave

elastically throughout the entire range of reloading.

The true stress (σ) is expressed in terms of engineering stress (s) by:

(Eq 13)

The derivation of Eq 13 assumes both constancy of volume and a homogeneous distribution of strain along the

gage length of the tension specimen. Thus, Eq 13 should be used only until the onset of necking. Beyond the

maximum load, the true stress should be determined from actual measurements of load and cross-sectional area.

(Eq 14)

The true strain, ε, may be determined from the engineering or conventional strain (e) by:

(Eq 15)

This equation is applicable only to the onset of necking for the reasons discussed above. Beyond maximum

load, the true strain should be based on actual area or diameter (D) measurements:

(Eq 16)

Figure 7 compares the true stress-true strain curve with its corresponding engineering stress-strain curve. Note

that because of the relatively large plastic strains, the elastic region has been compressed into the y-axis. In

agreement with Eq 13 and 15, the true stress-true strain curve is always to the left of the engineering curve until

the maximum load is reached.

Fig. 7 Comparison of engineering and true stress-true strain curves

However, beyond maximum load, the high, localized strains in the necked region that are used in Eq 16 far

exceed the engineering strain calculated from Eq 2. Frequently, the flow curve is linear from maximum load to

fracture, while in other cases its slope continuously decreases to fracture. The formation of a necked region or

mild notch introduces triaxial stresses that make it difficult to determine accurately the longitudinal tensile

stress from the onset of necking until fracture occurs. This concept is discussed in greater detail in the section of

this article on instability in tension. The following parameters usually are determined from the true stress-true

strain curve.

The true stress at maximum load corresponds to the true tensile strength. For most materials, necking begins at

maximum load at a value of strain where the true stress equals the slope of the flow curve. Let σ

and ε

denote

the true stress and true strain at maximum load when the cross-sectional area of the specimen is A

. The

ultimate tensile strength can be defined as:

(Eq 17)

and

(Eq 18)

Eliminating P

max

yields:

(Eq 19)

and

(Eq 20)

The true fracture stress is the load at fracture divided by the cross-sectional area at fracture. This stress should

be corrected for the triaxial state of stress existing in the tensile specimen at fracture. Because the data required

for this correction frequently are not available, true fracture stress values are frequently in error.

The true fracture strain, ε

, is the true strain based on the original area (A

) and the area after fracture (A

(Eq 21)

This parameter represents the maximum true strain that the material can withstand before fracture and is

analogous to the total strain to fracture of the engineering stress-strain curve. Because Eq 15 is not valid beyond

the onset of necking, it is not possible to calculate ε

from measured values of e

. However, for cylindrical

tensile specimens, the reduction in area (q) is related to the true fracture strain by:

(Eq 22)

The true uniform strain ε

, is the true strain based only on the strain up to maximum load. It may be calculated

from either the specimen cross-sectional area (A

) or the gage length (L

) at maximum load. Equation 15 may

be used to convert conventional uniform strain to true uniform strain. The uniform strain frequently is useful in

estimating the formability of metals from the results of a tension test:

(Eq 23)

The true local necking strain (ε

) is the strain required to deform the specimen from maximum load to fracture:

(Eq 24)

Footnote

Reprinted in part from Mechanical Metallurgy, 3rd ed., McGraw-Hill, New York, 1986, p 275–

295, with

permission

Mechanical Behavior Under Tensile and Compressive Loads*

George E. Dieter, University of Maryland

Mathematical Expressions for the Flow Curve

The flow curve of many metals in the region of uniform plastic deformation can be expressed by the simple

power curve relation:

σ = Kε

(Eq 25)

where n is the strain-hardening exponent, and K is the strength coefficient. A log-log plot of true stress and true

strain up to maximum load will result in a straight line if Eq 25 is satisfied by the data (Fig. 8).

Fig. 8 Log-log plot of true stress-true strain curve n is the strain-hardening exponent; K is

the strength coefficient.

The linear slope of this line is n, and K is the true stress at ε = 1.0 (corresponds to q = 0.63). As shown in Fig. 9,

the strain-hardening exponent may have values from n = 0 (perfectly plastic solid) to n = 1 (elastic solid). For

most metals, n has values between 0.10 and 0.50 (see Table 2).

Table 2 Values for n and K for metals at room temperature

Metals Condition

MPa ksi

Ref

0.05% carbon steel

Annealed 0.26 530 77 6

SAE 4340 steel

Annealed 0.15 641 93 6

0.6% carbon steel

Quenched and tempered at 540 °C (1000 °F) 0.10 1572 228 7

0.6% carbon steel

Quenched and tempered at 705 °C (1300 °F) 0.19 1227 178 7

Copper

Annealed 0.54 320 46.4 6

70/30 brass

Annealed 0.49 896 130 7

Fig. 9 Various forms of power curve σ = Kε

The rate of strain hardening dσ/dε is not identical to the strain-hardening exponent. From the definition of n:

(Eq 26)

Deviations from Eq 25 frequently are observed, often at low strains (10

-3

) or high strains (ε ≈ 1.0). One

common type of deviation is for a log-log plot of Eq 25 to result in two straight lines with different slopes.

Sometimes data that do not plot according to Eq 25 will yield a straight line according to the relationship:

σ = K(ε

+ ε)

(Eq 27)

can be considered to be the amount of strain that the material received prior to the tension test (Ref 8).

Another common variation on Eq 25 is the Ludwik equation:

σ = σ

+ Kε

(Eq 28)

where σ

is the yield stress, and K and n are the same constants as in Eq 25. This equation may be more

satisfying than Eq 25, because the latter implies that at zero true strain the stress is zero. It has been shown that

can be obtained from the intercept of the strain-hardening portion of the stress-strain curve and the elastic

modulus line by (Ref 9):

(Eq 29)

The true stress-true strain curve of metals such as austenitic stainless steel, which deviate markedly from Eq 25

at low strains (Ref 10), can be expressed by:

σ = Kε

+ +

(Eq 30)

where is approximately equal to the proportional limit, and n

is the slope of the deviation of stress from Eq

25 plotted against ε. Other expressions for the flow curve are available (Ref 11, 12). The true strain term in Eq

25 26 27 28 properly should be the plastic strain, ε

= ε

total

- ε

= ε

total

- σ/E, where ε

represents elastic strain.

Footnote

Reprinted in part from Mechanical Metallurgy, 3rd ed., McGraw-Hill, New York, 1986, p 275–

295, with

permission

References cited in this section

6. J.R. Low and F. Garofalo, Proc. Soc. Exp. Stress Anal., Vol 4 (No. 2), 1947, p 16–25

7. J.R. Low, Properties of Metals in Materials Engineering, American Society for Metals, 1949

8. J. Datsko, Material Properties and Manufacturing Processes, John Wiley & Sons, New York, 1966, p

18–20

9. W.B. Morrison, Trans. ASM, Vol 59, 1966, p 824

10. D.C. Ludwigson, Metall. Trans., Vol 2, 1971, p 2825–2828

11. H.J. Kleemola and M.A. Nieminen, Metall. Trans., Vol 5, 1974, p 1863–1866

12. C. Adams and J.G. Beese, Trans. ASME, Series H, Vol 96, 1974, p 123–126

Mechanical Behavior Under Tensile and Compressive Loads*

George E. Dieter, University of Maryland

Effect of Strain Rate and Temperature

The rate at which strain is applied to the tension specimen has an important influence on the stress-strain curve.

Strain rate is defined as = dε/dt. It is expressed in units of s

-1

. The range of strain rates encompassed by various

tests is shown in Table 3.

Table 3 Range of strain rates in common mechanical property tests

Range of strain rate

Type of test

-8

to 10

-5

-1

Creep test at constant load or stress

-5

to 10

-1

Tension test with hydraulic or screw driven machines

-1

to 10

-1

Dynamic tension or compression tests

to 10

-1

High-speed testing using impact bars

to 10

-1

Hypervelocity impact using gas guns or explosively driven projectiles

Increasing strain rate increases the flow stress. Moreover, the strain-rate dependence of strength increases with

increasing temperature. The yield stress and the flow stress at lower values of plastic strain are more affected by

strain rate than the tensile strength.

If the crosshead velocity of the testing machine is ν = dL/dt, then the strain rate expressed in terms of

conventional engineering strain is:

(Eq 31)

The engineering strain rate is proportional to the crosshead velocity. In a modern testing machine, in which the

crosshead velocity can be set accurately and controlled, it is a simple matter to carry out tension tests at a

constant engineering strain rate.

The true strain rate is given by:

(Eq 32)

Equation 32 shows that for a constant crosshead velocity the true strain rate will decrease as the specimen

elongates or cross-sectional area shrinks. To run tension tests at a constant true strain rate requires monitoring

the instantaneous cross section of the deforming region, with closed-loop control feed back to increase the

crosshead velocity as the area decreases. The true strain rate is related to the engineering strain rate by the

following equation:

(Eq 33)

The strain-rate dependence of flow stress at constant strain and temperature is given by:

σ = C ( )

|ε,T

(Eq 34)

The exponent in Eq 34, m, is known as the strain-rate sensitivity, and C is the strain hardening coefficient. It

can be obtained from the slope of a plot of log σ versus log . However, a more sensitive way to determine m is

with a rate-change test (Fig. 10). A tensile test is carried out at strain rate

and at a certain flow stress, σ

, the

strain rate is suddenly increased to

. The flow stress quickly increases to σ

. The strain-rate sensitivity, at

constant strain and temperature, can be determined from:

(Eq 35)

The strain-rate sensitivity of metals is quite low (<0.1) at room temperature, but m increases with temperature.

At hot-working temperatures, T/T

> 0.5, m values of 0.1 to 0.2 are common in metals. Polymers have much

higher values of m, and may approach m = 1 in room-temperature tests for some polymers.

Fig. 10 Strain-rate change test, used to determine strain-rate sensitivity, m. See text for

discussion

The temperature dependence of flow stress can be represented by:

(Eq 36)

where Q is an activation energy for plastic flow, cal/g · mol; R is universal gas constant, 1.987 cal/K · mol; and

T is testing temperature in kelvin. From Eq 36, a plot of ln σ versus 1/T will give a straight line with a slope

Q/R.

Footnote

Reprinted in part from Mechanical Metallurgy, 3rd ed., McGraw-Hill, New York, 1986, p 275–

295, with

permission

Mechanical Behavior Under Tensile and Compressive Loads*

George E. Dieter, University of Maryland

Instability in Tension

Necking generally begins at maximum load during the tensile deformation of a ductile metal. An exception to

this is the behavior of cold-rolled zirconium tested at 200 to 370 °C (390–700 °F), where necking occurs at a

strain of twice the strain at maximum load (Ref 13). An ideal plastic material in which no strain hardening

occurs would become unstable in tension and begin to neck as soon as yielding occurred. However, an actual

metal undergoes strain hardening, which tends to increase the load-carrying capacity of the specimen as

deformation increases.