Glikin A.E. Polymineral-Metasomatic Crystallogenesis

Подождите немного. Документ загружается.

4.6 Some Methods of Estimation of Reaction Volume Effect 171

localized automorph with a hard central core and a loose periphery is most likely

to occur if an isotherm has a convex trajectory (Fig. 4.1k). It is to be noted that the

above phenomena can only occur in a relatively small quantity of solution, assum-

ing, of course, that after accessing the eutonic point in a congruent system the

replacement stops and excessive matter of the protocrystal remains preserved in

relics. If the amount of a solution is relatively great, the process is characterized by

a short sector of the isotherm and the system state is close to a stationary one, with

formation of an almost homogeneous structure of the replacement products.

The process becomes more complicated in presence of additional compounds

(Fig. 4.1c). The quantity A′ of a dissolved substance makes it possible to generate

a quantity M′ of an additional phase, which requires a quantity B′ of the dissolving

substance. This mechanism is represented by auxiliary dash–dot lines in A′OB

coordinates. At the same time, M′M

part of the extra phase would crystallize,

while the other part OM

would remain in the solution. Further dissolution of B

results in crystallization of the additional substance M, which proceeds until the dot

is attained; quantities of substances undergoing dissolving and salting-out

and M

) can be readily estimated using BOM coordinates (see corresponding

auxiliary dash–dot lines). The total balance of the process is as follows: amount

B′ + B

of the protocrystal dissolves, and M′M

quantity of a new formation crystal-

lizes out. This formal conclusion is only a partial representation of the real process,

which becomes quite complicated due to salting-out followed by dissolution of

substance A according to the sector A′E

, as it was mentioned before.

This process, including a number of uncertainties caused by kinetic effects, is

shown in details in Fig. 4.5.

At the first stage (trajectory A

), the quantity of dissolving protocrystal B is

characterized by the sector OB

, while the amount of the new formation A is

described by the sector A

At the second stage the process proceeds along E

trajectory and its estima-

tion should be performed in A

OM coordinates. At this stage the substance A dis-

solves completely accompanied by continuous dissolution of the substance B and

initiating the salting-out of the substance M. The quantity of the substance M

undergoing salting-out ranges from M

to M

and depends upon the ratio

between the rate of replacement of the substance B and that of the substance A.

These sections are plotted on the abscissa as sectors OA

and A

, which are equal

to section A

; after that it is possible to draw the lines A

and A

, as well

as the lines M

and M

, A

, and A

are parallel to OM, while

and M

are parallel to the abscissa). To reach the position M

it is neces-

sary to dissolve the main quantity B

of the substance B (thus precipitating M

amount of M) and an additional quantity, which is described by the interval B

–

(thus precipitating M

–M

quantity of M). Dissolution of the additional

part of B compensates the movement of the system toward the dot E

that is caused

by the replacement of the substance A.

The additional part of the substance B and a quantity of the substance M corre-

sponding to that part, and the additional ratios of B, M, and A cannot be estimated

precisely, as their reactions are prolonged, and so their volume effect changes in the

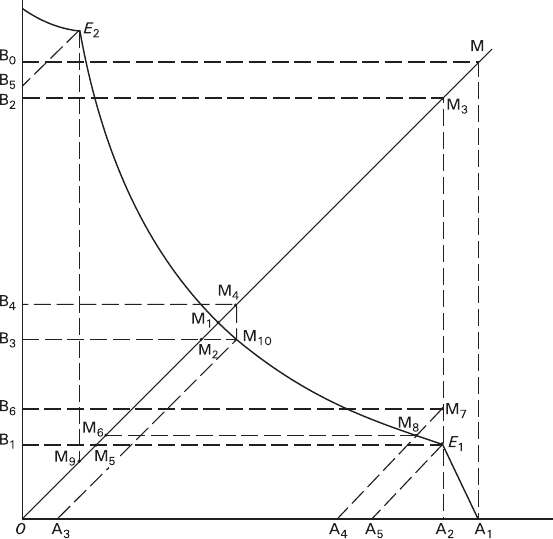
172 4 Physicochemical Analysis of Metasomatic Crystallogenesis

course of the process in accordance with the isotherm curvature. Reaching the

extreme values of B

–M

is possible if an extremely fast secondary replace-

ment takes place completely in the point E

, and the values of B

–M

are

feasible, and if an extremely slow replacement of A proceeds almost completely at

the third stage.

The third stage of the process corresponds to the trajectory M

and all calcula-

tion should be performed in BOM coordinates. Here, amount of dissolving sub-

stance B can be calculated from the sector OB

, while the quantity of precipitating

substance M is described by M

section.

If an insoluble additional compound is present in the system (reactions of type

III; e.g., system KAl(SO

)

·12H

O–BaSO

–BaCl

·2H

O–H

O), the points M

, M

, and E

coincide with zero point of the coordinates. The amount of the new for-

mation precisely corresponds to the section OM and may be calculated from ordi-

nary chemical equations describing exchange reactions.

It is desirable that solubilities plotted on the axes should be expressed as volume

units indicating ratio of a substance volume to a fixed amount of a solvent (e.g.,

1 cm

of a substance per 100 g of H

O or any other solvent having a fixed composition).

Fig. 4.5 Scheme for graphic determination of the volume effect at a multistage replacement of

crystals B in solution of the initial composition A

in a system containing additional compound M

(for a more schematic representation see Fig. 4.1c)

References 173

In a double-salts system (Fig. 4.1a) the axes scales are mutually independent and

can be arbitrary chosen in accordance with the formula composition of the sub-

stances involved, which, if present, should also include water of crystallization. If

the system contains any additional compounds (Figs. 4.1b, c), using this direct

method is impossible. Quantitative indices of all the three axes may be consistent

only when the measurement units of the main axes are expressed in equal molar or

weight units of components, a sum of which makes the formula of the additional

compound. For example, in analyzing replacement of KAl(SO

)

·12H

O alum with

BaSO

in solution of BaCl

·2H

O, the main axes should indicate contents of Ba

and SO

2−

ions determined in equilibrium solutions, while the intermediate axis

should indicate content of BaSO

. To use the diagram it is convenient to convert the

chosen solubility units of each axis into volume contents of substances in their

formula representations. The scales thus obtained would be different, but compat-

ible with each other.

Naturally, graphic representation of multi-type systems must be different, and

exemplary examination of some incongruent systems – systems with several addi-

tional compounds – or quaternary and more complex systems proves this in full

measure. Isomorphic replacement cannot be exactly examined in volume unit coor-

dinates, as the densities of the solid phases vary. Quantitative graphical analysis of

such replacement processes requires plotting special nomograms.

This section was not written to present any analytical algorithms. The examples

cited are to demonstrate a practicality of an important physicochemical approach to

replacement processes. It is to be noted that volume effect of replacement (under the

non-isothermal conditions too) entirely depends upon the equilibrium parameters of

a system. The proposed approach of physicochemical analysis combined with crys-

tallogenetic interpretation of structural-morphological and kinetic phenomena is

supposed to reveal the essence of metasomatic reactions and at present has no valid

alternatives. Of course, known variety of natural physicochemical systems does not

comprise all the possible cases, so only indirect features are available for analysis of

the process mechanisms, its driving forces and characteristic features. However, the

proposed approach provides a solid basis for mineral-genetic examinations. At the

same time, it is necessary to emphasize a difference between volume effect of crystal

replacement, which depends upon equilibrium characteristics of a system, and a

metasomatic change of the entire bulk of a mountain rock, which is substantially

defined by its mechanical characteristics and the process kinetics.

References

Anosov VYa, Ozerova MI, Fialkov YuA (1976) Fundamentals of physicochemical analysis.

Nauka, Moscow (Russ.)

Franke VD, Glikin AE, Kryuchkova LYu et al. (2007) Cocrystallization of isomorphic compo-

nents in solutions and crystal zoning: an example of the (Ba,Pb)(NO

)

series. Geol Ore

Deposits: 49:7:641–647

174 4 Physicochemical Analysis of Metasomatic Crystallogenesis

Glikin AE (1991) Mechanisms of inheriting and losing the structural attributes of rocks in the

course of metasomatism and recrystallization. In: Gelman ML (ed) Metamorphic complexes

of the North-East of USSR, their ore content and geological mapping. Russian Academy of

Science, Magadan (Russ.)

Glikin AE (1995a) On the theory of formation of isomorphic-mixed crystals. Zapiski Vsesoyuz

Miner Obsh 5:125–134 (Russ.)

Glikin AE (1995b) Crystallogenesis and geological-mineralogical sciences – coordination prob-

lems (by example of metasomatism phenomena). Zapiski Vsesoyuz Miner Obsh 4:116–125

(Russ.)

Glikin AE (1996a) The physicochemical aspect of the unsteady state of metasomatic crystal pro-

duction. Geochem Intern 33:8:117–128 (Russ.)

Glikin AE (1996b) About equilibrium supercooled solutions related to formation of isomorphic-

mixed crystals. Zapiski Vsesoyuz Miner Obsh 5:103–111 (Russ.)

Glikin AE (1996c) Modeling of metasomatic crystallogenesis in aqueous-salt systems. Dr Sci

thesis. St. Petersburg State University, St. Petersburg (Russ.)

Glikin AE (2002) Features of rapakivi origin in terms of polymineral-metasomatic crystallogen-

esis. Miner Soc Poland. Special Papers 20:17–19

Glikin AE, Petrov SV (1998) Recrystallization of bimineral aggregates. Zapiski Vseross Miner

Obsh 4:79–88 (Russ.)

Glikin AE, Sinai MYu (1991) Morphological and genetic classification of crystal replacement

products. Zapiski Vsesoyuz Miner Obsh 1:3–17 (Russ.)

Glikin AE, Sinai MYu (2004) Metasomatic formation of poikilitic crystals: experimental mode-

ling. Doklady Earth Sci 396: 4: 563–566

Glikin AE, Kovalev SI, Rudneva EB, et al. (2003) Phenomena and mechanisms of mixed crystal

formation in solutions I. General concept on the example of the system KHC

RbHC

-H

O. J. Cryst Growth 255:150–162

Glikin AE, Kryuchkova LYu, Plotkina YuV (2007) Crystallogenetic grounds of isomorphism:

experimental data and theoretical approach. Zapiski Russ Miner Soc, Crystallogenesis and

Mineralogy, Special Issue 7–35. Nauka, St. Petersburg

Kasatkin IA, Leont’eva OA (1992) Phase equilibria in aqueous salt systems containing isomorphic

components. Inorganic materials 28:6:1169–1172 (Russ.)

Kasatkin IA, Glikin AE, Bradaczek H, et al. (1995) Kinetics of mixed crystal K

(SO

,CrO

)

growth from aqueous solutions. Cryst Res Techn 30:5:659–666

Kirgintsev AN (1976) Sketches on thermodynamics of aqueous salt systems. Nauka, Novosibirsk

(Russ.)

Korzhinskii DS (1970) Theory of metasomatic zoning. Clarendon Press, Oxford

Kryuchkova LYu, Glikin AE, Voloshin AE, et al. (2002) Kinetic and morphological phenomena

of growth and isomorphic replacement of mixed crystals in solutions (in the series (Co,Ni)

(NH

)

(SO

)

·6H

O). Zapiski Vsesoyuz Miner Obsh 3:62–77 (Russ.)

Kulkov and Glikin (2007) Replacement of nickelhexahydrite with retgersite: polymorphic–meta-

somatic structures. Geol Ore Deposits 49:8:159–164

Punin YuO (1964) Recrystallization in aqueous solutions accompanied by grain coarsening.

Zapiski Vsesoyuz Miner Obsh 3:364–367 (Russ.)

Punin YuO (1965) On mechanism of recrystallization. Zapiski Vsesoyuz Miner Obsh 4:459–462

(Russ.)

Putnis A (2002) Mineral replacement reactions: from macroscopic observations to microscopic

mechanisms. Miner Mag 66:5:689–708

Shubnikov AV (1918) Influence of temperature fluctuations upon crystals. J Russ Physicochem

Soc. Phys Branch 50:39–44 (Russ.) (see also in: Zs Krist 1941 54:3–4:417–433)

Sinai MYu, Glikin AE (1989) Formation of case-like and negative pseudomorphs. Geol Explor

4:31–35 (Russ.)

Treivus EB (1977) Elaboration of the state diagrams of multi-component systems. In: Mass crys-

tallization. IREA, Moscow (Russ.)

Treivus EB (1982) A method of depicting the compositions of isomorphic crystals and three-

component solutions equilibrial to them. In: Frank-Kamenetskii VA (ed) Crystallography and

Crystallochemistry. Leningrad State University, Leningrad (Russ.)

Treivus EB (2000) About isothermal diagrams of ternary systems containing binary solid solution.

Vestnik St. Petersburg State University 7 Ser 2:15:14–22 (Russ.)

Treivus EB, Rozhnova GA (1962) Experimental reproduction of metasomatism in water-soluble

salts. Zapiski Vsesoyuz Miner Obsh 2:219–222 (Russ.)

Wyckoff R (1965) Crystal structures 1. Wiley, New York/London/Sidney

Wyckoff R (1966) Crystal structures 3. Wiley, New York/London/Sidney

References 175

Chapter 5

Metasomatic Transformation of Aggregates

5.1 On Replacement and Growth of Monocrystals

in the Course of Transformation of Aggregates

The processes of metasomatic transformation of aggregates can be conveniently

represented as a series of isolated replacements of single individuals. It is obvious

that replacement of individuals in aggregates conforms to the rules discussed above

for single protocrystals (see Chapter 1). Experiments conducted by Dr. M. Yu. Sinai

showed that a compact crystal aggregate behaves in a course of replacement as an

entity transforming either into a single pseudomorph, or a single automorph. It can

be accounted for by formation of a common diffusion field around the aggregate

undergoing dissolution, which induces precipitation of a new formation in the

periphery of the aggregate (pseudomorphic replacement) or at some distance from

the initial aggregation (automorphic replacement). Moreover, if the distance

between the individuals undergoing replacement is sufficient, the morphological

patterns are expected to be complicated as a result of overlapping the areas occu-

pied by the new formations produced around various individuals. Variation of

temperature causes direct processes of growth and dissolution to occur, which

would take place mainly on the border of a compact aggregate, subsequently pen-

etrating its inner part as the structure of the aggregate undergoes loosening. At the

same time, loosening the aggregates should induce an abrupt acceleration of

restructuring the solution.

Thus, metasomatic transformation of aggregates covers all four overelementary

classes of metasomatic crystallogenesis (see Introduction). During pseudomorphic

replacement of aggregates or individuals composing the aggregates, the basic fea-

tures of the initial structure (the first and second classes) should generally remain,

while during automorphic replacement of individuals the overlapping areas of the

products would to some extent conceal the protostructure (mainly, the third and

fourth classes). At the same time, structural-morphological diversity of the product

characteristics can be practically unlimited, being determined by the number and

quantitative ratio of solid reactants, their granulometric composition, spatial distri-

bution, as well as their physicochemical properties and kinetic characteristics of

growth and dissolution. Therefore, in contrast to replacement of monocrystals,

A.E. Glikin, Polymineral-Metasomatic Crystallogenesis, 177

178 5 Metasomatic Transformation of Aggregates

these processes should mostly belong to the reactions of the third and fourth

classes, as their products do not maintain any spatial and spatiotemporal relations

to the initial individuals.

Investigations of aggregate metasomatic replacement have been mainly focused

on the general phenomena and mechanisms of replacement of rock massifs (Irving

1911; Lindgren 1925; Pospelov 1976; Zavaritskii 1950; Korzhinskii 1955, 1970;

Demin et al. 1979; Zaraiskii 1979, 1991; Zaraiskii et al. 1981, 1986; Petrov 1983;

Yardley 1989; Barton et al. 1991; Aleksandrov 1995; and others). These investiga-

tions made the grounds for a diffusion–infiltration theory of metasomatic process

and allowed to formulate valid approaches to such well-known problems as analysis

of changes in total chemical composition of the rocks, construction of metasomatic

columns, and discovering succession of stages in metasomatic processes.

In this connection, a great attention is focused on the volume effect of metaso-

matic process. In contrast to unambiguous volume relations between the educt and

product observed during replacement of monocrystals (see Sects. 1.5.2 and 4.6),

effect of the replacement process upon the total volume of a rock formation cannot

be determined since it is mediated by the forms of mineral replacement, initial rock

porosity, and the rock expansive or compressive properties. Volume-deficit in a

rock massif can be compensated by increase in the rock friability and contraction,

which also can be accompanied by the removal of a new formation from the reac-

tion area. Also, the extreme case of volume compensation, which is formation of

spongy mixed crystals, would affect neither intercrystal space nor the total amount

of rock or any of its blocks. On the contrary, variations of the volume characteristics

of rocks are probably difficult to be evaluated when friability of the rocks increases

in various ways accompanied by contraction and removal of the secondary material.

The volume excess can be expressed as an increased volume of the mixed crystals,

filling up the pores and cracks with secondary crystals, removing the new formation

outside the reaction space, and as expanded dimensions of the massif undergoing

replacement.

Moreover, these processes are very likely to occur in combination

and their results appear to be rather uncertain; for example, removal of a new for-

mation outside the reaction volume can lead to the total volume-deficit, despite the

fact that the reaction balance is volume-excessive.

So, it is important to distinguish analysis of the volume transformations of

individual crystals from examination of rocks undergoing metasomatic transforma-

tions. It is to be noted that all the above-mentioned factors determining the volume

effect are well-known, and any concept implying the volume constancy cannot be

accepted as justifiable.

The most demonstrative example of the massif extension is a volume-excess replacement of

anhydrite with gypsum accompanied by development of local tectonic phenomena (Betekhtin

1961). It is to be noted that reactions of anhydrite transformation into gypsum and those of dehy-

dration of anhydrite (Prodan 1990) are typical reactions of metasomatic replacement and they

always involve salting-out.

5.1 On Replacement and Growth of Monocrystals in the Course 179

Another trend includes studying the metasomatic formation of individuals and

local peculiarities of structures of rocks and vein-shaped bodies (e.g., Ramdohr,

1955; Betekhtin et al. 1958; Atlas… 1976; MacKensie and Guilford 1980;

Adams et al. 1984; Yardley et al. 1990; Barker 1994; Craig and Vangham 1994).

This concept comprises two popular replacement models, namely a solid-phase

model (Lindgren 1925; Grigor’ev 1961) and a three-zone model (Beus 1961;

Pospelov 1973). Numerous investigations encompass uncountable metacrystals,

pseudomorphs, rims, and other specific aggregates, which in some cases allow to

reveal (including reported descriptions, regardless of the questions considered by

authors) some attributes of inheriting the primary aggregate structure by pseudo-

morphs (Lyakhovich 1954), by formations, which here are referred to as local-

ized automorphs (Tatarskii 1939; Velikoslavinskii 1953; Sudovikov 1967;

Adams et al. 1984), by shadowed pseudomorphs (Chesnokov 1974), and

some other formations.

Compositions and spatial distributions of minerals in numerous experimental

samples belonging to various metasomatic columns have been thoroughly investi-

gated; the results obtained have been generalized in the well-known concept of

column zonality (Zharikov and Zaraiskii 1973; Zaraiskii 1991; and others). The

descriptions contain data on some features of aggregate structure and in some cases

on metacrystal morphology (Schouten 1934; Garrels and Dreyer 1952; Ol’shanskii

and Brusilovskii 1958; Pospelov et al. 1961; Treivus and Rozhnova 1962; Zaraiskii

1979; Zaraiskii et al. 1981; Krasnova et al. 1983; Zaraiskii et al. 1986; Zaraiskii

1991; Krasnova and Petrov 1997; and others). Only a few reported works include

crystallogenetic analysis of behavior of individuals (Krasnova et al. 1983;

Krasnova and Petrov 1997).

The forms of metasomatic crystal growth were studied in precipitation experi-

ments using the method of reactant counter diffusion (Henisch 1970; Wilke 1973;

Petrov et al. 1983; Askhabov 1984; Givargizov et al. 1984; and others), which is

one of the experimental analogs of a contact-reaction process. The minerals pre-

pared belong to all mineral classes, the examples including gold (Kratochvil et al.

1968), cinnabar (Murphy et al. 1968), nantokite, miersite, and fluorite (Armington

and O’Connor 1968; Murphy et al. 1968; Kiryanova et al. 1984; Kiryanova and

Glikin 1999), magnetite and structural analogs of garnet (Y

[FeO

]

– Y-Fe-garnet)

and perovskite (YFeO

– Y-Fe-perovskite or orthoferrite) (Mikhailov et al. 1973),

celestine (Ulyanova and Petrov 1971), calcite (Kiryanova et al. 1998; Kiryanova

and Glikin 1999, and some others).

Y-Fe-garnet, Y-Fe-perovskite, fluorite, and calcite were synthesized for

researches of crystal morphogenesis (Mikhailov et al. 1973; Kiryanova et al. 1984).

These substances are interesting examples of metasomatic replacements in aggre-

gates having different primary structures. The experiments differed in having vari-

ous manners of arranging the reactant powders in solutions. The first experimental

series involved divided weights of pure initial reactants (in fluorite preparations the

distance between the reactant portions was about 20 cm, in calcite preparations

it was about 5 cm, and in Y-Fe-garnet and Y-Fe-perovskite preparations it was about

1–2 cm). Another variant comprised contacting portions of pure reactants (Y-Fe-garnet

180 5 Metasomatic Transformation of Aggregates

and Y-Fe-perovskite syntheses). The third series included mixtures of reactants

(Y-Fe-garnet and Y-Fe-perovskite synthesis), and the fourth variant consisted in

reactions of calcite crystals with fluorine-containing solutions. Crystallizations of

fluorite and calcite were conducted via diffusion in water of a number of soluble

salts containing calcium and fluorine (fluorite) or calcium and carbonate ion (cal-

cite) at room temperature and under low-temperature hydrothermal conditions in

solution having various pH values. Joint synthesis of Y-Fe-garnet and Y-Fe-perovskite

was carried out via a reaction of iron and yttrium oxides in hydrothermal solutions

of KOH. In this experimental series, diffusive influx of hydrogen into the solution

caused reduction of initial hematite that resulted in crystallization of magnetite in

association with the above minerals. It is to be reminded that hydrogen metaso-

matism is classified as an independent type of metasomatic process (Rus’ko 1976;

Lazarenko 1979).

It was possible to observe various distributions of new formations of Y-Fe-garnet

and Y-Fe-perovskite in container corresponding to the theory of precipitating the new

formations due to counter diffusion of components (Petrov et al. 1983). When the

reactants were divided, Y-Fe-garnet and Y-Fe-perovskite crystals were formed at the

border between a free volume of the solution and iron oxide, which had a significantly

lower solubility than yttrium oxide. Fluorite was also formed on the surface of the

less-soluble reactant or in relative proximity to it in a free solution volume. When the

reactants were in contact, precipitation of the new formation (Y-Fe-garnet and Y-Fe-

perovskite) was asymmetric in respect to the contact interface, proceeding in the way

analogous to that observed in model bimetasomatic columns (Zaraiskii et al. 1986).

In the case considered, it is possible to conclude that precipitation of the new forma-

tions occurs within the mass of the less-soluble iron oxide at different distances from

the surface of contact. In the mixtures of the initial reactant the new formations pre-

cipitated chaotically within the whole bulk. Degree of hematite replacement with

magnetite (proceeding under the action of hydrogen in accordance with usual scheme

of regenerative process) decreased along the column in downward direction, as the

distance from the top of container where hydrogen penetrated the system via holes in

the cover. Thus, the area of precipitation of the new formation was characterized by

“common” replacement front, which resulted from superposition of diffusive fields

induced by different individuals composing the aggregate. In this case, the aggregate

behaved as a whole entity and spatial coordination between the products and the

individuals undergoing replacement became lost completely, i.e., the system was

found to display all the attributes of the third overelementary class of metasomatic

crystallogenesis (see Introduction).

Synthesized crystals of Y-Fe-garnet, Y-Fe-perovskite, and magnetite looked like

a uniform fragile aggregate or could be distributed in a polycrystalline mass of

unreacted but partially recrystallized initial substances, mainly, in the mass of iron

oxides. Metacrystals could reach 1 mm in size. This result is an illustration for a

model of metasomatic formation of crystals in a solid medium proceeding in

accordance with the three-zone mechanism (Beus 1961; Pospelov 1973).

As a rule, crystals of metasomatic new formations have full-faceted, sometimes

skeletal, form with usual relief elements (growth layers, vicinal hillocks), typical

for the process of free growth from the solution; the same features have been also

detected in natural crystals (Novgorodova 1968). It should be emphasized that

morphological peculiarities of metasomatically formed fluorite and fluorite

obtained in direct growth experiments appear to be the same. It is a direct evidence

of common morphogenesis mechanisms of both types of crystallizations.

Faceting of crystals prepared by means of counter-diffusion method (analog of

bimetasomatic process) can include a small set of crystal forms (e.g., only {111}

for magnetite). Nevertheless, occurrence of some forms, atypical for synthesized

crystals, is also observed rather frequently, for instance: fluorite can develop the

{111}, {100}, {110}, and {112} faces instead of usual {111} and {100} combi-

nation; calcite occurs in the {0001}, {101

1}, {011

2}, and {088

1} faces instead

of the {0001} and {1011

} ones, Y-Fe-garnet forms {112}, {110}, {123}, {130},

and {100} faces instead of {110} and {112}. The number of crystal forms for the

orthorhombic orthoferrite reaches 16. The faceting variety can be explained by

nonstationarity of preparative conditions (Glikin and Glazov 1979; Grunskii

1988; Kasatkin et al. 1995) reflecting a specificity of metasomatic crystallogenesis,

which has been stated to be determined by nonstationary nature of metasomatic reac-

tions (Dolivo-Dobrovol’skii 1967; Glikin 1996a; and others). Of course, this

assumption requires thorough investigation since the variations of the forms can

be induced by usual influence of medium composition, supersaturation, and

temperature.

Metasomatic phenomena can accompany direct growth of polymineral aggre-

gates; it has been discussed in Chapter 2. One of the extreme cases of such a proc-

ess is probably formation of a metastable phase followed by its dissolution caused

by change in the solution composition induced by growth of the other phase (these

processes can be classified as belonging to any one of the two to four overelementary

classes). The metastable phase can be partially dissolved (see Sect. 4.5 and com-

ments to Fig. 4.4) or disappear completely, as it happens, for example, during

evaporation of an aqueous KNO

solution, where a hexagonal modification of

KNO

, having precipitated originally, dissolves, being automorphically replaced

with an orthorhombic modification (these phenomena can be classified as belong-

ing to the third overelementary class). A phase can be dissolved partially or com-

pletely, and then get crystallized again. For example, according to our data,

at the

first stage of the experiments involving Na

–NaNO

–H

O system, evaporation

followed by cooling resulted in formation of an aggregate composed of three to four

alternating polycrystalline zones consisting of Na

and NaNO

. During the

second stage of the experiment, zones of one of the substances dissolved com-

pletely accompanied by continuous growth of the second phase. During the third

stage, both substances precipitated again in a whole bulk of the residual solution

(the fourth-class phenomena).

Growing of crystals via dissolution of polycrystalline substance having another

composition accompanied by a convective transport of the matter to the growth

5.1 On Replacement and Growth of Monocrystals in the Course 181

Ms M. V. Togatova performed the experimental investigations.