Hassani S. Mathematical Methods: For Students of Physics and Related Fields
Подождите немного. Документ загружается.

566 Second-Order Linear Differential Equations
24.2 The Wronskian
To form a basis of solutions, f
1
and f
2
must be linearly independent. It is
important to note that the linear dependence or independence of a number of
functions, defined on the interval [a, b], is a concept that must hold for all x
in [a, b]. Thus, if
α
1
f
1
(x
0
)+α
2
f
2
(x
0
)+···+ α
n
f
n
(x
0
)=0
for some x
0
∈ [a, b], it does not mean that the f ’s are linearly dependent.
Linear dependence requires that the equality holds for all x in [a, b].
The nature of the linear relation between f
1
and f
2
can be determined by
their Wronskian.
Wronskian defined
Definition 24.2.1. The Wronskian of any two differentiable functions f
1
(x)
and f
2
(x) is defined to be
W (f
1
,f
2
; x)=f
1
(x)f
2
(x) −f
2
(x)f
1
(x)=det
⎛
⎝
f
1
(x) f
1
(x)
f
2
(x) f
2
(x)
⎞
⎠
.
If we differentiate both sides of the definition of Wronskian and substitute
from Equation (24.4), we obtain
d
dx
W (f
1
,f
2
; x)=f
1
f
2
+ f
1
f
2
− f
2
f
1
− f
2
f
1
= f
1
(−pf
2
− qf
2
) −f
2
(−pf
1
− qf
1
)
= pf
1
f
2
− pf
1
f
2
= −p(x)W (f
1
,f
2
; x).
We can easily find a solution to this DE:
dW
dx
= −pW ⇒
dW
W
= −pdx ⇒ ln W = −
#
x
c
p(t) dt +lnC,
where c is an arbitrary point in the interval [a, b]andC is the constant of
integration. In fact, it is readily seen that C = W (c). We therefore have
W (f
1
,f
2
; x)=W (f
1
,f
2
; c)e
−
x
c
p(t) dt
. (24.5)
Note that W (f
1
,f
2
; x) = 0 if and only if W (f
1
,f
2
; c) = 0, and that [because
the exponential in (24.5) is positive] W (f
1
,f
2
; x)andW (f
1
,f
2
; c)havethe
same sign if they are not zero. This observation leads to
Box 24.2.1. The Wronskian of any two solutions of Equation (24.4)
does not change sign in the interval [a, b]. In particular, if the Wronskian
vanishes at one point in [a, b], it vanishes at all points in [a, b].
24.3 A Second Solution to the HSOLDE 567
Let f
1
and f
2
be any two differentiable functions that are not necessarily
solutions of any DE. If f
1
and f
2
are linearly dependent, then one is a multiple
of the other, and the Wronskian is readily seen to vanish. Conversely, assume
that the Wronskian is zero. Then f
1
(x)f
2
(x) −f
2
(x)f
1
(x) = 0. This gives
f
1
df
2
= f
2
df
1
⇒
df
2
f
2
=
df
1
f
1
⇒ ln f
2
=lnf
1
+lnC ⇒ f
2
= Cf
1
and the two functions are linearly dependent. We have just shown that differentiability is
important in the
statement of Box
24.2.2.
Box 24.2.2. Two differentiable functions, which are nonzero in the inter-
val [a, b], are linearly dependent if and only if their Wronskian vanishes.
Example 24.2.1. Let f
1
(x)=x and f
2
(x)=|x| for −1 ≤ x ≤ 1. These two
functions are linearly independent in the given interval, because α
1
x + α
2
|x| =0for
all x if and only if α
1
= α
2
= 0. The Wronskian, on the other hand, vanishes for
all − 1 ≤ x ≤ 1:
W (f
1
,f
2
; x)=x
d|x|
dx
−|x|
dx
dx
= x
d|x|
dx
−|x|
= x
d
dx
0
x if x ≥ 0
−x if x ≤ 0
−
0
x if x ≥ 0
−x if x ≤ 0
=
0
x − x =0 ifx>0,
−x − (−x)=0 ifx<0.
This seems to be in contradiction to Box 24.2.2. It is not! Box 24.2.2 assumes that
both functions are differentiable in their common interval of definition. However,
|x| is not differentiable at x =0.
24.3 A Second Solution to the HSOLDE
If we know one solution to Equation (24.4), we can use the Wronskian to
obtain a second linearly independent solution. Let W (x) ≡ W (f
1
,f
2
; x)bethe
Wronskian of the two solutions f
1
and f
2
. Then, by definition and Equation
(24.5), we have
f
1
(x)f
2
(x) − f
2
(x)f
1
(x)=W (x)=W (c)e
−
x
c
p(t) dt
,
where c is an arbitrary point in the interval of interest. Given f
1
(x), this is a
FOLDE in f
2
(x), which can be solved by the method of Subsection 23.3. In
fact, 1/f
2
1
(x) is an integrating factor, and dividing both sides by f
2
1
(x)gives
d
dx
f
2
(x)
f
1
(x)
=
W (x)
f
2
1
(x)
568 Second-Order Linear Differential Equations
ora second linearly
independent
solution can be
found from a
given solution
f
2
(x)
f
1
(x)
= C +
#
x
α
W (s)
f
2
1
(s)
ds = C +
#
x
α
1
f
2
1
(s)
W (c)exp
−
#
s
c
p(t) dt
ds,
where C is an arbitrary constant of integration and α is a convenient point in
the interval [a, b]. Thus,
second solution of
the HSOLDE
obtained from the
first
f
2
(x)=f
1
(x)
(
C + K
#
x
α
1
f
2
1
(s)
exp
−
#
s
c
p(t) dt
ds
)
, (24.6)
where we substituted K for W (c). We do not have to know W (x) (this
would require knowledge of f
2
, which we are trying to calculate!) to obtain
K = W (c). In fact, it is a good exercise for the reader to show that f
2
,asgiven
by (24.6), indeed satisfies Equation (24.4) no matter what K is. Note also
that f
2
(α)=Cf
1
(α). Whenever possible—and convenient—it is customary
to set C = 0 because its presence simply gives a term that is proportional to
the known solution f
1
(x).
Example 24.3.1.
(a) A solution to the SOLDE y
− k
2
y =0ise
kx
. To find a
second solution, we let C =0andK = 1 in Equation (24.6). Since p(x)=0,we
have
f
2
(x)=e
kx
0+
#
x
α
ds
e
2ks
= −
1
2k
e
−kx
+
e
−2kα
2k
e
kx
which, ignoring the second term which is proportional to the first solution, leads
directly to the choice of e
−kx
as a second solution.
(b) The differential equation y
+ k
2
y = 0, which arises in mechanics in the study
of the motion of a mass attached to the end of a spring, has sin kx as a solution.
With C =0,c = α = π/2k,andK =1,weget
f
2
(x)=sinkx
0+
#
x
π/2k
ds
sin
2
ks
= −sin kx cot ks|
x
π/2k
= −cos kx.
Thus, sin kx and cos kx form a basis of solution, and a general solution is of the
form
y(x)=A cos kx + B sin kx,
a result that should be familiar to the reader from introductory physics.
(c) For the solutions in part (a),
W (x)=det
e
kx
ke
kx
e
−kx
−ke
−kx
= −2k
and for those in part (b),
W (x)=det
sin kx k cos kx
cos kx −k sin kx
= −k.
Both Wronskians are constant. This is a special case of a result that holds for all
DEs of the form y
+ q(x)y =0.
24.4 The General Solution to an ISOLDE 569
Most special functions used in mathematical physics are solutions of SOL-
DEs. The behavior of these functions at certain special points is determined by
the physics of the particular problem. In most situations physical expectation
leads to a preference for one particular solution over the other. For example,
although there are two linearly independent solutions to the Legendre DE,
Legendre
differential
equation
d
dx
(1 −x
2
)
dy
dx
+ n(n +1)y =0,
the solution that is most frequently encountered is a Legendre polynomial
P
n
(x) discussed in Chapter 26. The other solution can be obtained by using
Equation (24.6).
24.4 The General Solution to an ISOLDE
We now determine the most general solution of an inhomogeneous SOLDE
(ISOLDE). Let g(x) be a particular solution of
L[y]=y
+ py
+ qy = r(x) (24.7)
and let h(x) be any other solution of this equation. Then h(x) −g(x)satisfies
Equation (24.4) and, by Theorem 24.1.3, can be written as a linear combina-
tion of a basis of solutions f
1
(x)andf
2
(x). It follows that
h(x)=c
1
f
1
(x)+c
2
f
2
(x)+g(x). (24.8)
Box 24.4.1. If we have a particular solution of the ISOLDE of Equation
(24.7) and two basis solutions of the HSOLDE, then the most general
solution of (24.7) can be expressed as the sum of a linear combination of
the two basis solutions and the particular solution.
We know how to find a second solution to the HSOLDE once we know one
solution. We now show that knowing one such solution will also allow us to
find a particular solution to the ISOLDE. The method we use is called the
method of variation of constants.
method of
variation of
constants
Let f
1
and f
2
be the two (known) solutions of the HSOLDE and g(x)the
sought-after solution to Equation (24.7). Write g as g(x)=f
1
(x)v(x)with
v a function to be determined. Substitute this in (24.7) to get a SOLDE for
v(x):
with a solution of
HSOLDE at our
disposal, we can
find a particular
solution of an
ISOLDE.
v
+
p +
2f
1
f
1
v
=
r
f
1
.
This is a first-order linear DE in v
which has a solution of the form (see
Problem 24.6)
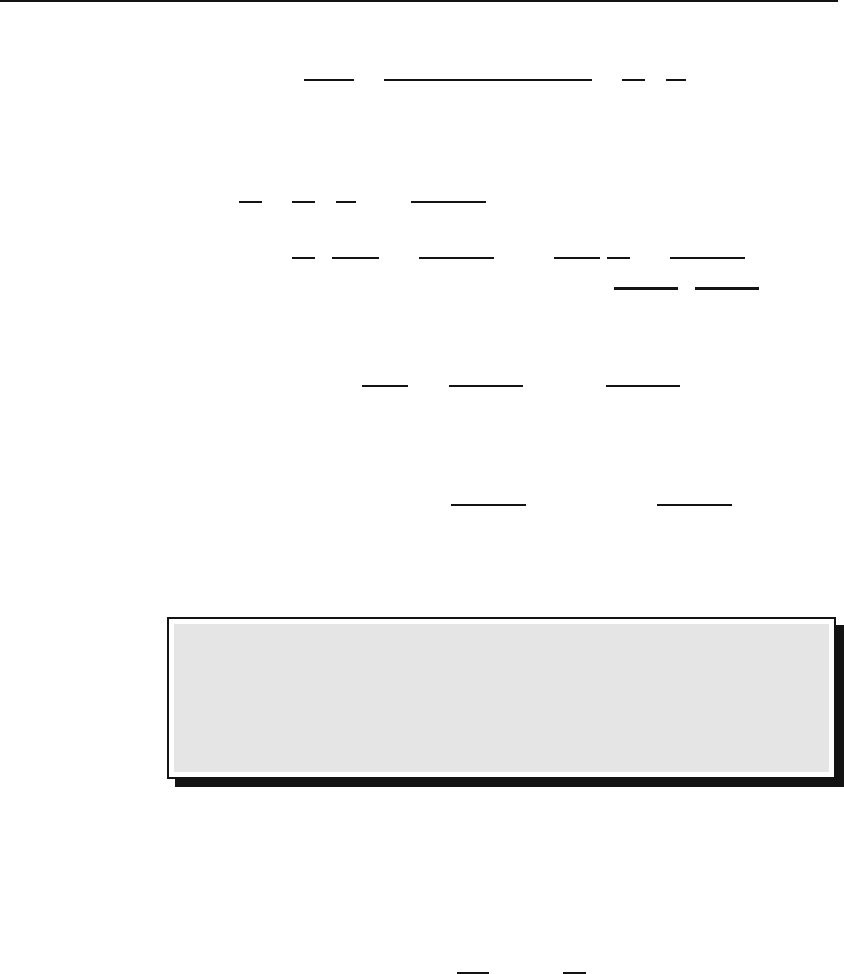
v
=
W (x)
f
2
1
(x)
C +
#
x
a
f
1
(t)r(t)
W (t)
dt
,
570 Second-Order Linear Differential Equations
where W (x) is the (known) Wronskian of Equation (24.7). Substituting
W (x)
f
2
1
(x)
=
f
1
(x)f
2
(x) −f
2
(x)f
1
(x)
f
2
1
(x)
=
d
dx
f
2
f
1
in the above expression for v
and setting C = 0 (we are interested in a
particular solution), we get
dv
dx
=
d
dx
f
2
f
1
#
x
a
f
1
(t)r(t)
W (t)
dt
=
d
dx
f
2
(x)
f
1
(x)
#
x
a
f
1
(t)r(t)
W (t)
dt
−
f
2
(x)
f
1
(x)
d
dx
#
x
a
f
1
(t)r(t)
W (t)
dt
=f
1
(x)r(x)/W (x)
and, by integration,
v(x)=
f
2
(x)
f
1
(x)
#
x
a
f
1
(t)r(t)
W (t)
dt −
#
x
a
f
2
(t)r(t)
W (t)
dt,
where in the last integral, we used t as the variable of integration. This leads
to the particular solution
g(x)=f
1
(x)v(x)=f
2
(x)
#
x
a
f
1
(t)r(t)
W (t)
dt −f
1
(x)
#
x
a
f
2
(t)r(t)
W (t)
dt. (24.9)
Note how symmetric f
1
and f
2
appear in the final result.
It thus follows that
Box 24.4.2. Given a single solution f
1
(x) of the homogeneous equation
corresponding to an ISOLDE, one can use Equation (24.6) to find a second
solution f
2
(x) of the homogeneous equation and Equation (24.9) to find a
particular solution g(x). The most general solution h,willthenbe
h(x)=c
1
f
1
(x)+c
2
f
2
(x)+g(x).
24.5 Sturm–Liouville Theory
We saw in Chapter 22 that the separation of PDEs normally results in ex-
pressions of the form
L[u]+λu =0, or p
2
(x)
d
2
u
dx
2
+ p
1
(x)
du
dx
+ p
0
(x)u + λu =0, (24.10)
where u is a function of a single variable and λ is, a priori, an arbitrary
constant. This is an eigenvalue equation for the operator L just as Equation
(7.17) was an eigenvalue equation for the matrix T. In this section, we try
to learn some properties of this eigenvalue problem, but first we need to
understand the concept of the adjoint of a differential operator.
24.5 Sturm–Liouville Theory 571
24.5.1 Adjoint Differential Operators
In our discussion of the eigenvalues and eigenvectors of matrices in Section 7.4,
symmetric matrices seemed to be special (see Theorem 7.4.1). The analog of a
symmetric matrix in the case of differential operators (DO) is a self-adjoint
differential operator.
The HSOLDE
L[y] ≡ p
2
(x)y
+ p
1
(x)y
+ p
0
(x)y = 0 (24.11)
is said to be exact if it can be written as
exact SOLDE
L[y]=
d
dx
[A(x)y
+ B(x)y]. (24.12)
An integrating factor for L[y] is a function μ(x) such that μ(x)L[y]isexact.
integrating factor
for SOLDE
If an integrating factor exists, then Equation (24.11) reduces to
d
dx
[A(x)y
+ B(x)y]=0 ⇒ A(x)y
+ B(x)y = C,
a FOLDE with a constant inhomogeneous term whose solution is given in
Theorem 23.3.1. Even the ISOLDE corresponding to Equation (24.11) can be
solved, because
μ(x)L[y]=μ(x)r(x) ⇒
d
dx
[A(x)y
+ B(x)y]=μ(x)r(x)
⇒ A(x)y
+ B(x)y =
#
x
α
μ(t)r(t) dt,
which is a general FOLDE. Thus, the existence of an integrating factor com-
pletely solves a SOLDE. It is therefore important to know whether or not a
SOLDE admits an integrating factor.
If the SOLDE is exact, then (24.12) must equal (24.11), implying that
p
2
= A, p
1
= A
+ B,andp
0
= B
. It follows that p
2
= A
, p
1
= A
+ B
,
and p
0
= B
,whichinturngivep
2
−p
1
+p
0
=0.Converselyifp
2
−p
1
+p
0
=0,
then, substituting p
0
= −p
2
+ p
1
in the LHS of Equation (24.11), we obtain
p
2
y
+ p
1
y
+ p
0
y = p
2
y
+ p
1
y
+(−p
2
+ p
1
)y
= p
2
y
− p
2
y +(p
1
y)
=(p
2
y
− p
2
y)
+(p
1
y)
=
d
dx
(p
2
y
− p
2
y + p
1
y),
and the DE is exact. Therefore,
Box 24.5.1. The SOLDE of Equation (24.11) is exact if and only if
p
2
− p
1
+ p
0
=0.
572 Second-Order Linear Differential Equations
A general SOLDE is clearly not exact. Can we make it exact by multi-
plying it by an integrating factor as we did with a FOLDE? An immediate
consequence of Box 24.5.1 is
Box 24.5.2. Afunctionμ is an integrating factor of the SOLDE of Equa-
tion (24.11) if and only if it is a solution of the HSOLDE
M[μ] ≡ (p
2
μ)
− (p
1
μ)
+ p
0
μ =0. (24.13)
We can expand Equation (24.13) to obtain the equivalent equation
p
2
μ
+(2p
2
− p
1
)μ
+(p
2
− p
1
+ p
0
)μ =0. (24.14)
The operator M given by
adjoint of a
second-order linear
differential
operator
M ≡ p
2
d
2
dx
2
+(2p
2
− p
1
)
d
dx
+(p
2
− p
1
+ p
0
) (24.15)
is called the adjoint of the operator L and denoted by M ≡ L
†
. Thisisthe
equivalent of the transpose of a matrix T
t
.
Box 24.5.2 confirms the existence of an integrating factor. However, the
latter can be obtained only by solving Equation (24.14), which is at least as
difficult as solving the original differential equation! In contrast, the integrat-
ing factor for a FOLDE can be obtained by a mere integration [see Equation
(23.13)].
Although integrating factors for SOLDEs are not as useful as their coun-
terparts for FOLDEs, they can facilitate the study of SOLDEs. Let us
first note that the adjoint of the adjoint of a differential operator is the
original operator: (L
†
)
†
= L (see Problem 24.10). This suggests that if
v is an integrating factor of L[u], then u will be an integrating factor of
M[v] ≡ L
†
[v]. In particular, multiplying the first one by v and the second
one by u and subtracting the results, we obtain [see Equations (24.11) and
(24.13)] vL[u] −uM[v]=(vp
2
)u
−u(p
2
v)
+(vp
1
)u
+ u(p
1
v)
,whichcanbe
simplified to
vL[u] −uM[v]=
d
dx
[p
2
vu
− (p
2
v)
u + p
1
uv]. (24.16)
Integrating this from a to b yields
Lagrange
identities
#
b
a
vL[u] −uM[v]
!
dx =
.
p
2
vu
− (p
2
v)
u + p
1
uv
/
b
a
. (24.17)
Equations (24.16) and (24.17) are called the Lagrange identities.
As in the case of matrices, a self-adjoint differential operator (correspond-
ing to a symmetric matrix for which T = T
t
) merits special consideration.
24.5 Sturm–Liouville Theory 573
For M[v] ≡ L
†
[v]tobeequaltoL[v], we must have [see Equations (24.11) and
(24.14)] 2p
2
−p
1
= p
1
and p
2
−p
1
+ p
0
= p
0
. The first equation gives p
2
= p
1
,
which also solves the second equation. If this condition holds, then we can
write Equation (24.11) as L[y]=p
2
y
+ p
2
y
+ p
0
y,or
L[y]=
d
dx
p
2
(x)
dy
dx
+ p
0
(x)y =0.
Can we make all SOLDEs self-adjoint? Let us multiply both sides of
Equation (24.11) by a function w(x), to be determined later. We get the new
DE
w(x)p
2
(x)y
+ w(x)p
1
(x)y
+ w(x)p
0
(x)y =0,
which we desire to be self-adjoint. This will be accomplished if we choose
w(x) such that wp
1
=(wp
2
)
,orp
2
w
+ w(p
2
−p
1
) = 0, which can be readily
integrated to give
w(x)=
1
p
2
exp
#
x
p
1
(t)
p
2
(t)
dt
.
We have just proved the following:
Theorem 24.5.1. The SOLDE of Equation (24.11) is self-adjoint if and only
all SOLDEs can
be made
self-adjoint
if p
2
= p
1
, in which case the DE has the form
d
dx
p
2
(x)
dy
dx
+ p
0
(x)y =0.
If it is not self-adjoint, it can be made so by multiplying it through by
w(x)=
1
p
2
exp
#
x
p
1
(t)
p
2
(t)
dt
.
Example 24.5.2.
(a) The Legendre equation in normal form,
y
−
2x
1 − x
2
y
+
λ
1 − x
2
y =0,
is not self-adjoint. However, we get a self-adjoint version if we multiply through by
w(x)=1− x
2
:
(1 − x
2
)y
− 2xy
+ λy =0, or [(1 − x
2
)y
]
+ λy =0
(b) Similarly, the normal form of the Bessel equation
y
+
1
x
y
+
1 −
n
2
x
2
y =0
is not self-adjoint, but multiplying through by h(x)=x yields
d
dx
x
dy
dx
+
x −
n
2
x
y =0,
which is clearly self-adjoint.
574 Second-Order Linear Differential Equations
24.5.2 Sturm–Liouville System
Now that we know that every SOLDE can be made self-adjoint, let’s apply
the procedure to our starting DE (24.10). If we multiply that equation by the
w(x) of Theorem 24.5.1 it becomes self-adjoint, and can be written as
d
dx
p(x)
du
dx
+[λw(x) − q(x)]u =0 or
L[u]=
d
dx
p(x)
du
dx
− q(x)u = −λw(x)u (24.18)
with p(x)=w(x)p
2
(x)andq(x)=−p
0
(x)w(x). Equation (24.18) is the
standard form of the Sturm-Liouville (S-L) equation.
The appearance of w is the result of our desire to render the differential
operator self-adjoint. It also appears in another context. Write the Lagrange
identity (24.16) for a self-adjoint differential operator L:
uL[v] −vL[u]=
d
dx
{p(x)[u(x)v
(x) − v(x)u
(x)]}. (24.19)
If we specialize this identity to the S-L equation of (24.18) with u = u
1
corresponding to the eigenvalue λ
1
and v = u
2
corresponding to the eigenvalue
λ
2
, we obtain for the LHS
u
1
L[u
2
] − u
2
L[u
1
]=u
1
(−λ
2
wu
2
)+u
2
(λ
1
wu
1
)=(λ
1
− λ
2
)wu
1
u
2
.
Integrating both sides of (24.19) then yields
(λ
1
− λ
2
)
#
b
a
wu
1
u
2
dx = {p(x)[u
1
(x)u
2
(x) −u
2
(x)u
1
(x)]}
b
a
. (24.20)
A desired property of the solutions of a self-adjoint DE is their orthogonality
when they belong to different eigenvalues. This property will be satisfied if
we assume an inner product integral with weight function w(x), and if the
RHS of Equation (24.20) vanishes. There are various boundary conditions
(BC) that fulfill the latter requirement. One such boundary conditions are
separated boundary conditions:
α
1
u(a)+β
1
u
(a)=0,
α
2
u(b)+β
2
u
(b)=0, (24.21)
where α
1
, α
2
, β
1
,andβ
2
are real constants. Another set of appropriate bound-Sturm–Liouville
systems
ary conditions is the periodic BC given by
u(a)=u(b)andu
(a)=u
(b). (24.22)
The collection of the DO and the boundary conditions is called a Sturm–
Liouville (S-L) system.

24.6 SOLDEs with Constant Coefficients 575
Example 24.5.3. For fixed ν the DE
d
2
u
dr
2
+
1
r
du
dr
+
k
2
−
ν
2
r
2
u =0, 0 ≤ r ≤ b (24.23)
transforms into the Bessel equation u
+ u
/x +(1− ν
2
/x
2
)u =0ifwemake
the substitution kr = x. Thus, the solution of the S-L equation (24.23) that is
analytic at r = 0 and corresponds to the eigenvalue k
2
is u
k
(r)=J
ν
(kr)—because
Bessel functions J
ν
(x) are entire functions. For two different eigenvalues, k
2
1
and k
2
2
,
the eigenfunctions are orthogonal if the boundary term of (24.20) corresponding to
Equation (24.23) vanishes, that is, if
{r[J
ν
(k
1
r)J
ν
(k
2
r) − J
ν
(k
2
r)J
ν
(k
1
r)]}
b
0
vanishes, which will occur if and only if J
ν
(k
1
b)J
ν
(k
2
b)−J
ν
(k
2
b)J
ν
(k
1
b) = 0. A com-
mon choice is to take J
ν
(k
1
b)=0=J
ν
(k
2
b), that is, to take both k
1
b and k
2
b as (dif-
ferent) roots of the Bessel function of order ν.Wethushave
b
0
rJ
ν
(k
i
r)J
ν
(k
j
r) dr =
0ifk
i
and k
j
are different roots of J
ν
(kb)=0.
The Legendre equation
d
dx
(1 − x
2
)
du
dx
+ λu =0, where − 1 <x<1,
is already self-adjoint. Thus, w(x) = 1, and p(x)=1− x
2
. Solutions of this DE
corresponding to λ = n(n + 1) are the Legendre polynomials P
n
(x). The bound-
ary term of (24.20) clearly vanishes at a = −1andb = +1, and we obtain the
orthogonality relation:
+1
−1
P
n
(x)P
m
(x) dx =0ifm = n.
The Hermite equation is
u
− 2xu
+ λu =0. (24.24)
It is transformed into an S-L system if we multiply it by w(x)=e
−x
2
. The resulting
S-L equation is
d
dx
e
−x
2
du
dx
+ λe
−x
2
u =0. (24.25)
The function u is an eigenfunction of (24.25) corresponding to the eigenvalue λ if
and only if it is a solution of (24.24). Solutions of this DE corresponding to λ =2n
are the Hermite polynomials H
n
(x). The boundary term corresponding to the two
eigenfunctions u
1
(x)andu
2
(x) having the respective eigenvalues λ
1
and λ
2
= λ
1
is
{e
−x
2
[u
1
(x)u
2
(x) − u
2
(x)u
1
(x)]}
b
a
.
This vanishes for arbitrary u
1
and u
2
if a = −∞ and b =+∞. We can therefore
write
+∞
−∞
e
−x
2
H
n
(x)H
m
(x) dx =0ifm = n.
24.6 SOLDEs with Constant Coefficients
The SOLDEs with constant coefficients occur frequently and their solutions
are easily accessible. In fact, we need not confine ourselves to the second order
equations. The most general nth-order linear differential equation (NOLDE)
with constant coefficients can be written as
L[y] ≡ y
(n)
+ a
n−1
y
(n−1)
+ ···+ a
1
y
+ a
0
y = r(x). (24.26)
The corresponding homogeneous NOLDE (HNOLDE) is obtained by setting
r(x)=0.
