Heiman G. Basic Statistics for the Behavioral Sciences
Подождите немного. Документ загружается.


Although Table 15.1 looks like a two-way ANOVA, it is not analyzed like one.
Instead of testing for main effects, the two-way procedure tests only what is essen-
tially the interaction. Recall that with an interaction, the influence of one variable
depends on the other. The two-way is also called the test of independence because
it tests whether the frequency that participants fall into the categories of one variable
depends on the frequency of falling into the categories on the other variable. Thus, our
study will test whether the frequencies of having or not having a heart attack are inde-
pendent of the frequencies of being Type A or Type B.
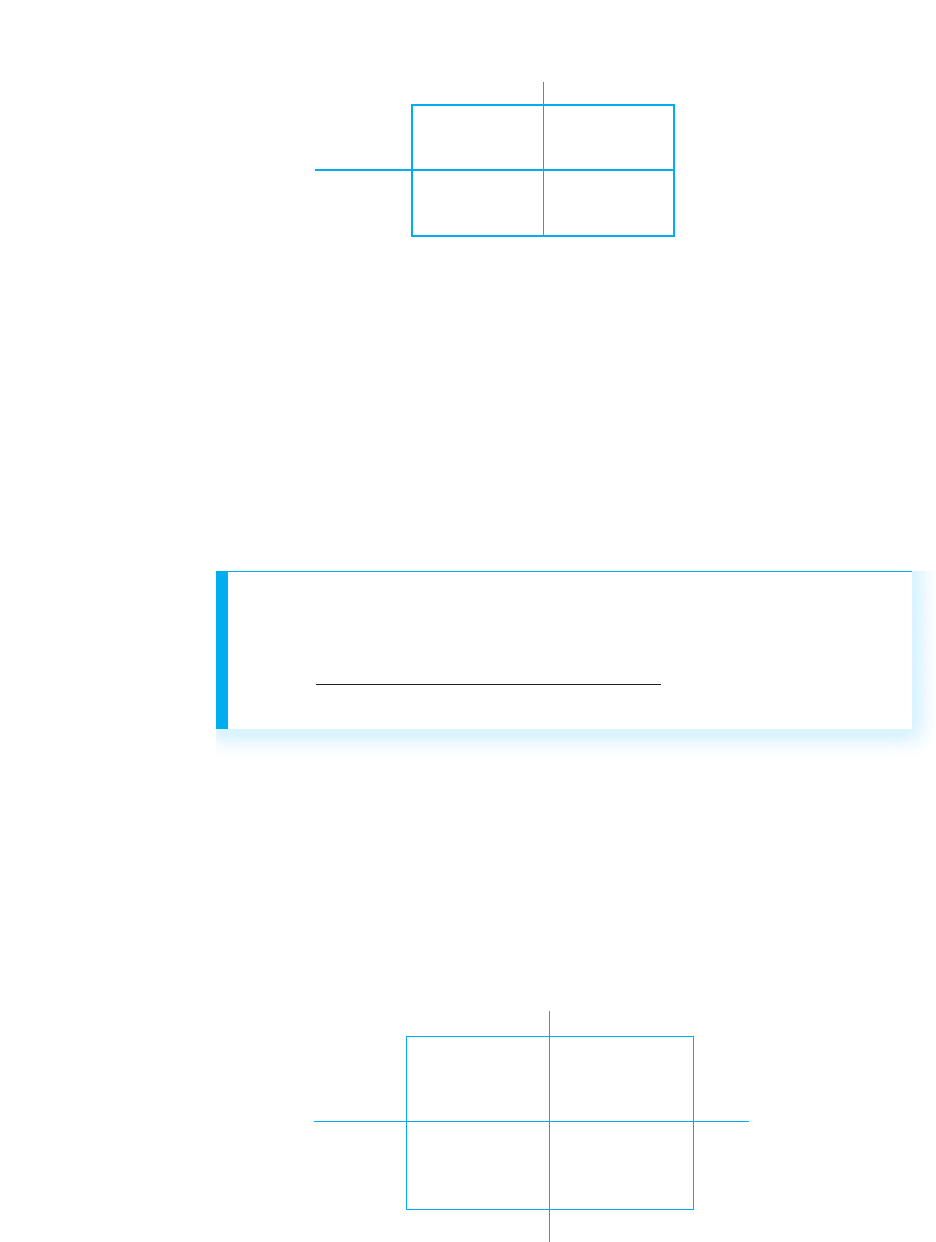
To understand independence, the left-hand matrix in Table 15.2 shows an example of
data we might get if category membership on our variables was perfectly independent.
Here, the frequency of having or not having a heart attack does not depend on the fre-
quency of being Type A or Type B. Another way to view the two-way is as a test of
whether a correlation exists between the two variables. When variables are independ-
ent, there is no correlation, and using the categories from one variable is no help in pre-
dicting the frequencies for the other variable. Here, knowing if people are Type A or
Type B does not help to predict if they do or do not have heart attacks (and the health
categories do not help in predicting personality type).
On the other hand, the right-hand matrix in Table 15.2 shows an example of when
category membership on the two variables is totally dependent. Here, the frequency of
a heart attack or no heart attack depends on personality type. Likewise, a perfect corre-
lation exists because whether people are Type A or Type B is a perfect predictor of
whether or not they have had a heart attack (and vice versa).
But, say that the actual observed frequencies from our participants are those shown
in Table 15.3. There is a degree of dependence here because heart attacks tend to
be more frequent for Type A personalities, while no heart attack is more frequent for
Type B personalities. Therefore, some degree of correlation exists between the vari-
ables. On the one hand, we’d like to conclude that this relationship exists in the popu-
lation. On the other hand, perhaps there really is no correlation in the population, but
by chance we obtained frequencies that poorly represent this. The above translate into
our null and alternative hypotheses. In the two-way , is that category member-
ship on one variable is independent of (not correlated with) category membership on
the other variable. If the sample data look correlated, this is due to sampling error. The
is that category membership on the two variables in the population is dependent
(correlated).
H
a
H
0
2
2
2
2
358 CHAPTER 15 / Chi Square and Other Nonparametric Procedures
TABLE 15.2
Examples of Independence and Dependence
On the left, personality type and heart attacks are perfectly independent. On the right, personality type and heart attacks are
perfectly dependent.
Personality Type
Type A Type B
Heart
Attack
f
o
20
f
o
20
Health
No Heart
Attack
f
o
20
f
o
20
Personality Type
Type A Type B
Heart
Attack
f
o
40
f
o
0
Health
No Heart
Attack
f
o
0
f
o
40
Personality Type
Type A Type B
Heart
Attack
f
o
25
f
o
10
Health
No Heart
Attack
f
o
5
f
o
40
TABLE 15.3
Observed Frequencies as
a Function of Personality
Type and Health
Personality Type
Type A Type B
Heart f
o
25 f
o
10 row
Attack f
e
13.125 f
e
21.875 total 35
(35)(30)/80 (35)(50)/80
Health
No Heart f
o
5 f
o
40 row
Attack f
e
16.875 f
e
28.125 total 45
(45)(30)/80 (45)(50)/80
column column total 80
total 30 total 50 N 80
TABLE 15.4
Diagram Containing f
o
and f
e
for Each Cell
Each f
e
equals the row
total times the column
total, divided by N.
The Two-Way Chi Square 359
Computing the Two-Way Chi Square
Again the first step in computing is to compute the expected frequencies. To do so,
as shown in Table 15.4, first compute the total of the observed frequencies in each col-
umn and the total of the observed frequencies in each row. Also, note , the total of all
observed frequencies.
Each is based on the probability of a participant falling into a cell if the two vari-
ables are independent. For example, for the cell of Type A and heart attack, we deter-
mine the probability of someone in our study being Type A and the probability of
someone in our study reporting a heart attack, when these variables are independent.
The expected frequency in this cell then equals this probability multiplied times .
Luckily, the steps involved in this can be combined to produce this formula.
N
f
e
N
2
For each cell we multiply the total observed frequencies for the row containing the cell
times the total observed frequencies for the column containing the cell. Then divide by
the of the study.
Table 15.4 shows the completed computations of for our study. To check
your work, confirm that the sum of the in each column or row equals the column
or row total.
f
e
f
e
N
The formula for computing the expected frequency
in a cell of a two-way chi square is
f
e
5
1Cell’s row total f
o
21Cell’s column total f
o
2
N

Compute the two-way using the same formula used in the one-way design,
which is
With the data in Table 15.4 we have
As you did previously with this formula, in the numerator of each fraction subtract
from . Then square that difference and then divide by the in the denominator. Then
so
To evaluate , compare it to the appropriate . First, determine the degrees of
freedom by looking at the number of rows and columns in the diagram of your study.
In a two-way chi square, df ()( ).
For our design, is . Find the critical value of in Table
7 in Appendix C. At and , the is 3.84.
Our of 30.56 is larger than , so it is significant. Therefore, envision the sam-
pling distribution back in Figure 15.1, with in the region of rejection. This indicates
that the differences between our observed and expected frequencies are so unlikely to
occur if our data represent variables that are independent in the population, that we reject
that this is what the data represent. If the variables are not independent, then they must
be dependent. Therefore, we accept the that the frequency of participants falling into
each category on one of our variables depends on the category they fall into on the other
variable. In other words, we conclude that there is a significant correlation such that the
frequency of having or not having a heart attack depends on the frequency of being Type
A or Type B (and vice versa). If is not larger than the critical value, do not reject
. Then, we cannot say whether these variables are independent or not.
REMEMBER A significant two-way indicates that the sample data are likely
to represent two variables that are dependent (or correlated) in the population.
2
H
0
2
obt
H
a
2
obt
2
crit
2
obt
2
crit
df 5 1 5 .05
2
12 2 1212 2 125 1df2 3 2
Number of columns 2 1Number of rows 2 1 5
2
crit
2
obt
2
obt
5 30.56
2
obt
5 10.74 1 6.45 1 8.36 1 5.01
f
e
f
o
f
e
1 a
140 2 28.1252
2
28.125
b
2
obt
5 a
125 2 13.1252
2
13.125
b1 a
110 2 21.8752
2
21.875
b1 a
15 2 16.8752
2
16.875
b
2
obt
5 © a
1f
o
2 f
e
2
2
f
e
b
2
obt
360 CHAPTER 15 / Chi Square and Other Nonparametric Procedures
■
The two-way is used when counting the
frequency of category membership on two
variables.
■
The is that category membership for one
variable is independent of category membership for
the other variable.
H
0
2
MORE EXAMPLES
We count the participants who indicate (1) whether
they like or dislike statistics and (2) their gender. The
is that liking/disliking is independent of gender.
The results are
H
0
A QUICK REVIEW
(continued)
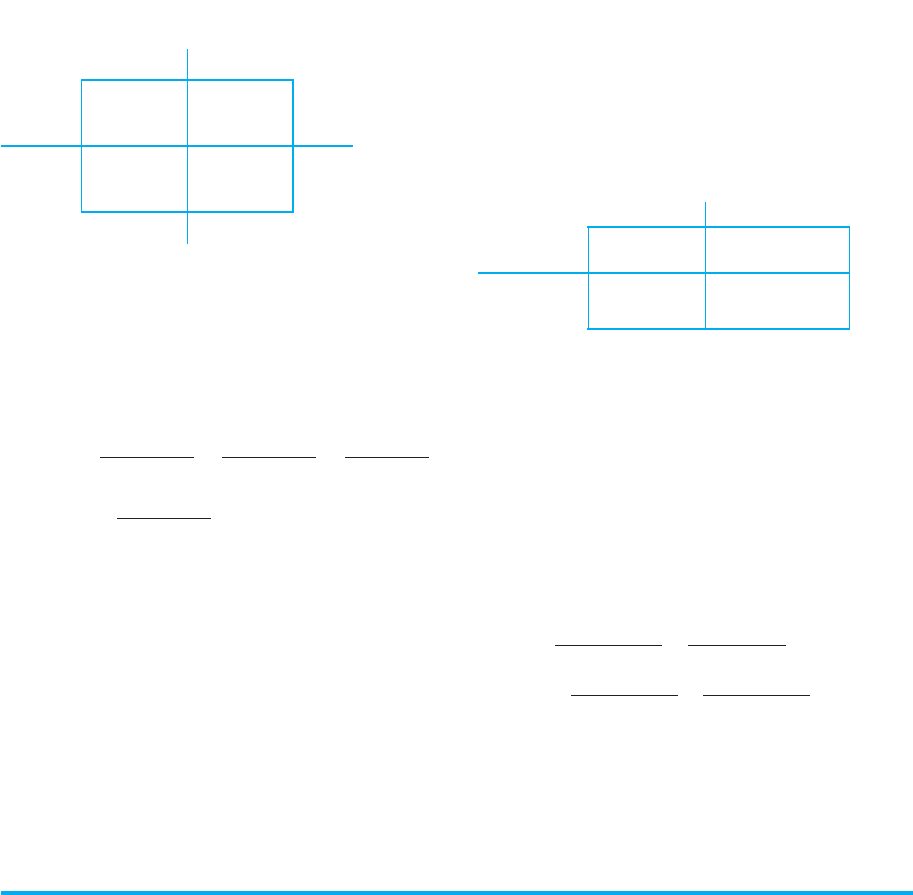
The Two-Way Chi Square 361
Like Dislike
f
o
20 f
o
10
Male
f
e
15 f
e
15
Total f
o
30
f
o
5 f
o
15
Female
f
e
10 f
e
10
Total f
o
20
Total Total
f
o
25 f
o
25 N 50
Compute each :
For male-like:
For male-dislike:
For female-like:
For female-dislike:
With , , so is significant: The
frequency of liking/disliking statistics depends on—is
correlated with—whether participants are male or
female.
For Practice
1. The two-way is used when counting the ______
with which participants fall into the ______ of two
variables.
2
2
obt
2
crit
5 3.84 5 .05
df 5 12 2 1212 2 125 1
2
obt
5 8.334
2
obt
5 25>15 1 25>15 1 25>10 1 25>10
1
115 2 102
2
10
2
obt
5
120 2 152
2
15
1
110 2 152
2
15
1
15 2 102
2
10
f
e
5 12021252>50 5 10
f
e
5 12021252>50 5 10
f
e
5 13021252>50 5 15
f
e
5 13021252>50 5 15
f
e
2. The is that the frequencies in the categories of
one variable are ______ of those of other variable.
3. Below are the frequencies for people who are
satisfied/dissatisfied with their job and who
do/don’t work overtime. What is the in each cell?
Overtime No Overtime
Satisfied f
o
11 f
o
3
Dissatisfied f
o
8 f
o
12
4. Compute .
5. The ______ and ______.
6. What do you conclude about these variables?
Answers
1. frequency; categories
2. independent
3. For satisfied–overtime, ;
for satisfied–no overtime, ;
for dissatisfied–overtime, ;
for dissatisfied–no overtime, .
4.
5. 1; 3.84
6. is significant: The frequency of job satisfaction/
dissatisfaction depends on the frequency of overtime/
no overtime.
2
obt
1
18 2 11.1762
2
11.176
1
112 2 8.8242
2
8.824
5 4.968
2
obt
5
111 2 7.8242
2
7.824
1
13 2 6.1762
2
6.176
f
e
5 8.824
f
e
5 11.176
f
e
5 6.176
f
e
5 7.824
2
crit
5df 5
2
obt
f
e
H
0
Describing the Relationship in a Two-Way Chi Square
A significant two-way chi square indicates a significant correlation between the vari-
ables. To determine the size of this correlation, we have two new correlation coeffi-
cients: We compute either the phi coefficient or the contingency coefficient.
If you have performed a chi square and it is significant, compute the
phi coefficient. Its symbol is , and its value can be between 0 and . Think
of phi as comparing your data to the ideal situations shown back in Table 15.2.
A coefficient of 0 indicates that the variables are perfectly independent. The larger
the coefficient, the closer the variables are to forming a pattern that is perfectly
dependent.
11
2 3 2
362 CHAPTER 15 / Chi Square and Other Nonparametric Procedures
equals the total number of participants in the study.
For the heart attack study, was 30.56 and was 80, so
Thus, on a scale of 0 to , where indicates perfect dependence, the correlation is
between the frequency of heart attacks and the frequency of personality types.
Remember that another way to describe a relationship is to square the correlation
coefficient, computing the proportion of variance accounted for. If you didn’t take the
square root in the above formula, you would have (phi squared). This is analogous
to or , indicating how much more accurately we can predict scores by using the
relationship. Above, , so we are 38% more accurate in predicting the fre-
quency of heart attacks/no heart attacks when we know personality type (or vice versa).
The other correlation coefficient is the contingency coefficient, symbolized by .
This is used to describe a significant two-way chi square that is not a design (it’s
a , a , and so on).3 3 32 3 3
2 3 2
C
2
5 .38
2
r
2
2
.62
1111
5
B
2
obt
N
5
B
30.56
80
5 1.382 5 .62
N
2
obt
N
is the number of participants in the study. Interpret in the same way you interpret
. Likewise, is analogous to .
STATISTICS IN PUBLISHED RESEARCH: REPORTING CHI SQUARE
The results of a one-way or two-way are reported like previous results, except
that in addition to the , we also include the . For example, in our handedness
study, was 50, was 1, and the significant was 18. We report this as
.
To graph a one-way design, label the axis with frequency and the axis with the
categories, and then plot the in each category. With a nominal variable, create a bar
graph. Thus, the graph on the left in Figure 15.2 shows the results of our handedness
study. The graph on the right shows the results of our heart attack study. For a two-way
design, place frequency on the axis and one of the nominal variables on the axis.
The levels of the other variable are indicated in the body of the graph. (This is similar
to the way a two-way interaction was plotted in the previous chapter, except that here
we create bar graphs.)
XY
Xf
o
XY
2
11, N 5 5025 18.00, p 6 .05
2
obt
dfN
Ndf
2
2
C
2
CN
The formula for the phi coefficient is
5
B
2
obt
N
The formula for the contingency coefficient is
C 5
B
2
obt
N 1
2
obt

Nonparametric Procedures for Ranked Data 363
NONPARAMETRIC PROCEDURES FOR RANKED DATA
The is the only common inferential statistic for nominal scores. The only other type
of nonparametric procedure is for when the dependent variable involves rank-ordered
(ordinal) scores. You obtain ranked scores for one of two reasons. First, sometimes
you’ll directly measure participants using ranked scores (directly assigning participants
a score of 1st, 2nd, and so on). Second, sometimes you’ll initially measure interval or
ratio scores, but they violate the assumptions of parametric procedures by not being
normally distributed or not having homogeneous variance. Then you transform these
scores to ranks (the highest raw score is ranked 1, the next highest score is ranked 2,
and so on). Either way, you then compute one of the following nonparametric inferen-
tial statistics to determine whether there are significant differences between the condi-
tions of your independent variable.
The Logic of Nonparametric Procedures for Ranked Data
Instead of computing the mean of each condition in the experiment, with nonparamet-
ric procedures we summarize the individual ranks in a condition by computing the sum
of ranks. The symbol for a sum of ranks is . In each procedure, we compare the
observed sum of ranks to an expected sum of ranks. To see the logic of this, say we
have the following scores:
Condition 1 Condition 2
12
43
56
87
Here, the conditions do not differ, with each containing both high and low ranks. When
the ranks are distributed equally between two groups, the sums of ranks are also equal
(here, is 18 in each). This is true even for two populations. Our is always that
the populations are equal, so with ranked data, is that the sums of ranks for each
population are equal. Therefore, when is true, we expect the sums of ranks from theH
0
H
0
H
0
©R
©R 5 18©R 5 18
©R
2
40
30
20
10
f
0
Handedness
Left Right
45
40
35
30
25
20
15
10
5
f
0
Personality type
Heart attacks
No heart attacks
AB
(a) (b)
FIGURE 15.2
Frequencies of
(a) left- and right-
handed geniuses and
(b) heart attacks and
personality type
364 CHAPTER 15 / Chi Square and Other Nonparametric Procedures
samples to be equal. Thus, the observed above is exactly what we would
expect if is true, so such an outcome supports .
But say the data had turned out differently, as here:
Condition 1 Condition 2
15
26
37
48
Condition 1 contains all of the low ranks, and Condition 2 contains all of the high
ranks. Because these samples are different, they may represent two different popula-
tions. With ranked data says that one population contains predominantly low ranks
and the other contains predominantly high ranks. When our data are consistent with
, the observed sum of ranks in each sample is different from the expected sum of
ranks produced when is true: Here, each does not equal 18.
Thus, the observed sum of ranks in each condition should equal the expected sum if
is true, but the observed sum will not equal the expected sum if is true. Of
course, it may be that is true, but we have sampling error in representing this, in
which case, the observed sum will not equal the expected sum. However, the larger the
difference between the expected and observed sum of ranks, the less likely it is that this
difference is due to sampling error, and the more likely it is that each sample represents
a different population.
In each of the following procedures, we compute a statistic that measures the differ-
ence between the expected and the observed sum of ranks. If we can then reject and
accept , we are confident that the reason the observed sum is different from the
expected sum is that the samples represent different populations. And, if the ranks
reflect underlying interval or ratio scores, a significant difference in ranks indicates that
the raw scores also differ significantly.
Resolving Tied Ranks
Each of the following procedures assumes you have resolved any tied ranks, in which
two participants receive the same rank on the same variable. Say that two people are
ranked 2nd. If not tied, one of them would have been 2nd and the other 3rd. Therefore,
resolve ties by assigning the mean of the ranks that would have been used had there not
been a tie. The mean of 2 and 3 is 2.5, so each tied participant would be given the new
rank of 2.5. Now, in a sense, you’ve used 2 and 3, so the next participant (originally
3rd) is assigned the new rank of 4, the next is given 5, and so on. (If three participants
had tied at 2nd, we’d assign them the mean of 2, 3, and 4, and assign the next person
the rank of 5. And so on.) Doing this makes your sum of ranks work out correctly.
Choosing a Nonparametric Procedure
Each of the major parametric procedures found in previous chapters has a correspon-
ding nonparametric procedure for ranked data. Your first task is to know which non-
parametric procedure to choose for your type of research design. Table 15.5 shows the
name of the nonparametric version of each parametric procedure we have discussed.
H
a
H
0
H
0
H
a
H
0
©RH
0
H
a
H
a
©R 5 26©R 5 10
H
0
H
0
©R 5 18
Nonparametric Procedures for Ranked Data 365
Note: The table includes from Chapter 7 the Pearson , the parametric correlation coef-
ficient, and the Spearman , the nonparametric correlation coefficient for ranks. My
advice is to memorize this table!
The steps in calculating each new nonparametric procedure are described in the fol-
lowing sections.
Tests for Two Independent Samples: The Mann–Whitney
U Test and the Rank Sums Test
Two nonparametric procedures are analogous to the t-test for two independent samples:
the Mann–Whitney test and the rank sums test. Which test we use depends on the
in each condition.
The Mann–Whitney U Test Perform the Mann–Whitney test when the in
each condition is equal to or less than 20 and there are two independent samples of
ranks. For example, say that we measure the reaction times of people to different visual
symbols that are printed in either black or red ink. Reaction times tend to be highly pos-
itively skewed, so we cannot perform the t-test. Therefore, we convert the reaction time
scores to ranks. Say that each is 5 (but unequal ns are acceptable). Table 15.6 gives
the reaction times (measured in milliseconds) and their corresponding ranks.
n
nU
nU
r
S
r
Type of Design Parametric Test Nonparametric Test
Two independent Independent- Mann–Whitney U or rank
samples samples t-test sums test
Two related Related-samples Wilcoxon T test
samples t-test
Three or more Between-subjects Kruskal–Wallis H test
independent ANOVA (Post hoc test: rank
samples (Post hoc test: sums test)
protected t-test)
Three or more Within-subjects Friedman
2
test
repeated- ANOVA (Post hoc test: Nemenyi’s
measures (Post hoc test: test)
samples Tukey’s HSD)
Correlational study Pearson r Spearman r
S
TABLE 15.5
Parametric Procedures
and Their Nonparametric
Counterparts
Red Symbols Black Symbols
㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬 㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬㛬
Reaction Ranked Reaction Ranked
Time Score Time Score
540 2 760 7
480 1 890 8
600 5 1105 10
590 3 595 4
605 6 940 9
n 5 5n 5 5
©R 5 38©R 5 17
TABLE 15.6
Ranked Data from Two
Independent Samples

366 CHAPTER 15 / Chi Square and Other Nonparametric Procedures
To perform the Mann–Whitney test,
1. Assign ranks to all scores in the experiment. Assign the rank of 1 to the lowest
score in the experiment, regardless of which group it is in. Assign the rank of 2 to
the second-lowest score, and so on.
2. Compute the sum of the ranks for each group. Compute for each group.
3. Compute two versions of the Mann–Whitney . First, compute for Group 1,
using the formula
where is the of Group 1, is the of Group 2, and is the sum of ranks
from Group 1. Let’s identify the red symbol condition as Group 1, so from Table
15.6, we have
Second, compute for Group 2, using the formula
Our black symbol condition is Group 2, so
4. Determine the Mann–Whitney . In a two-tailed test, the value of equals
the smaller of or . In the example, and , so .
In a one-tailed test, we predict that one of the groups has the larger sum of ranks.
The corresponding value of or from that group becomes .
5. Find the critical value of in Table 8 of Appendix C entitled “Critical Values of
the Mann–Whitney .” Choose the table for either a two-tailed or a one-tailed test.
Then locate using and . For our example, with a two-tailed test and
and , the is 2.0.
6. Compare to . WATCH OUT! Unlike any statistic we’ve discussed, the
is significant if it is equal to or less than . (This is because the smaller
the , the more likely that is false. In the example, and
, so the samples differ significantly, representing different populations
of ranks. Because the ranks reflect reaction time scores, the samples of reaction
times also differ significantly and represent different populations .
7. To describe the effect size, compute eta squared. If is significant, then ignore the
rule about the ns and reanalyze the data using the following rank sums test to get to .
The Rank Sums Test Perform the rank sums test when you have two independent
samples of ranks and either is greater than 20. To illustrate the calculations, we’ll
violate this rule and use the data from the previous reaction time study.
To perform the rank sums test,
1. Assign ranks to the scores in the experiment. As we did back in Table 15.6, rank-
order all scores in the experiment.
n
2
U
obt
1p 6 .052
U
crit
5 2.0
U
obt
5 2.0H
0
U
obt
U
crit
U
obt
U
crit
U
obt
U
crit
n
2
5 5n
1
5 5
n
2
n
1
U
crit
U
U
U
obt
U
2
U
1
U
obt
5 2.0U
2
5 2.0U
1
5 23.0U
2
U
1
U
obt
U
obt
U
2
5 1521521
515 1 12
2
2 38 5 40 2 38 5 2.0
U
2
5 1n
1
21n
2
21
n
2
1n
2
1 12
2
2 ©R
2
U
2
U
1
5 1521521
515 1 12
2
2 17 5 40 2 17 5 23.0
©R
1
nn
2
nn
1
U
1
5 1n
1
21n
2
21
n
1
1n
1
1 12
2
2 ©R
1
U
1
U
©R
U
Nonparametric Procedures for Ranked Data 367
2. Choose one group and compute the sum of the ranks. Compute for one
group.
3. Compute the expected sum of ranks for the chosen group. Use the
formula
where is the of the chosen group and is the total of the study. We’ll com-
pute for the red symbol group, which had ( ). Thus,
4. Compute the rank sums statistic, symbolized by . Use the formula
where is the sum of the ranks for the chosen group, is the expected
sum of ranks for the chosen group, and are the of the two groups, and is
the total of the study.
For our example, so
5. Find the critical value of in the z-tables (Table 1 in Appendix C). At ,
the two-tailed . (If we had predicted whether the sum of ranks of the
chosen group would be greater than or less than the expected sum of ranks, then
we would use the one-tailed value of either or .)
6. Compare to . If the absolute value of is larger than , then the sam-
ples differ significantly. In our example, and .
Therefore, we conclude that the samples of ranked scores—as well as the
underlying samples of reaction times—differ significantly .
7. Describe a significant relationship using eta squared. Here eta squared is
analogous to . Use the formula
where is computed in the above rank sums test and is the total number of
participants.
In the example, is and is 10, so we have , or . Thus, the
color of the symbols accounts for of the variance in the ranks. Because the ranks
reflect reaction time scores, approximately 53% of the differences in reaction time
scores are associated with the color of the symbol.
.53
.5312.192
2
>9N22.19z
obt
Nz
obt
2
5
1z
obt
2
2
N 2 1
r
2
pb
1p 6 .052
z
crit
5 ;1.96z
obt
522.19
z
crit
z
obt
z
crit
z
obt
21.64511.645
z
crit
5 ;1.96
5 .05z
z
obt
5
210.5
222.92
5
210.5
4.79
522.19
z
obt
5
©R 2 ©R
exp
B
1n
1
21n
2
21N 1 12
12
5
17 2 27.5
B
152152110 1 12
12
©R 5 17
N
Nnsn
2
n
1
©R
exp
©R
z
obt
5
©R 2 ©R
exp
B
1n
1
21n
2
21N 1 12
12
z
obt
©R
exp
5
n
1
1N 1 12
2
5
5110 1 12
2
5
55
2
5 27.5
N is 10n 5 5©R
exp
NNnn
©R
exp
5
n1N 1 12
2
1©R
exp
2
©R
