Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Medrano, Minei, Stark and Terras study Ihara-Selberg zeta functions

Cayley graphs for the Heisenberg group over certain finite rings, and

discuss

corresponding Artin L-function.

This book was prepared

the University of South Florida. Denise

Marks put the book together and handled all correspondence and the

galley proofs. We thank Denise for all she has done for this project.

Working with Denise is always

pleasure.

We take this opportunity and thank all the speakers and participants

in the American Mathematical Society Special Session, all the contribut-

ing authors, and the referees, in making this book

worthy tribute to

Mizan Rahman.

Orlando,

FL,

and Delft,

July

2004.

Mourad

E.H.

Ismail

Erik Koelink

This volume is

dedicated to Mizan

Rahman in recognition

of his contributions to

special functions,

q-series and orthogonal

polynomials.

MIZAN RAHMAN, HIS MATHEMATICS AND

LITERARY WRITINGS

Richard Askey

Department of Mathematics

University of Wisconsin

Madison, Wisconsin 53706

Mourad E.H. Ismail

Department

Mathematics

University of Central Florida

Orlando,

39618

Erik Koelink

Department of Mathematics

Technische Universiteit Delft

Delft, The Netherlands

Mizan studied at the University of Dhaka where he obtained his B.Sc.

degree in mathematics and physics in 1953 and his M.Sc. in applied

mathematics in 1954. He received a

B.A.

in mathematics from Cam-

bridge University in 1958, and a M.A. in mathematics from Cambridge

University in 1963. He was a senior lecturer at University of Dhaka from

1958 until 1962. Mizan decided to go abroad for his Ph.D. He went to

the University of New Brunswick in 1962 and received his Ph.D. in 1965

with a thesis on Kinetic Theory of Plasma using singular integral equa-

tions techniques. After obtaining his Ph.D., Mizan became an assistant

professor at Carleton University, where he spent the rest of his career.

He is currently a distinguished professor emeritus there.

In this article we mainly discuss some of Mizan's mathematical re-

sults which are the most striking and influential, at least in our opinion.

Needless to say, we cannot achieve completeness since Mizan has written

so many interesting papers. The reference item preceded by

refer to

items under "Publications" on Mizan's CV while the ones without

refer to references at the end of this article.

2005

Springer Science+Business Media, Inc.

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

In the early part of his research career Mizan devoted some energy

to questions involving statistical distributions resulting in the papers

CV[5], CV[7] and CV[11]. Mizan spent the academic year 1972173 at

Bedford College, of the University of London on sabbatical and worked

with Mike Hoare. In an e-mail to the editors, Hoare described how the

liberal arts atmosphere of Bedford, set idyllically in Regent Park was

well-suited for Mizan but the down side was that Physics at Bedford

was a small department and "there was little resonance in the heavily

algebracisized Mathematics Department under Paul Cohen." He added

"This hardly seemed to matter, since we were both outsiders from what

was most fashionable at the time."

Hoare's original plan was to study a one-dimensional gas model known

as the Rayleigh piston, but his collaboration with Mizan went way be-

yond this goal. This resulted in CV[9] and CV[12]. Another problem

suggested by Hoare involved urn models which made them soon realize

that the urn models they were investigating were related to birth and

death processes and Jacobi and Hahn polynomials. The result of their

investigations are papers CV[13], CV[17], and CV[18]. Some proba-

bilistic interpretations of identities for special functions were known, but

it was not an active area of research. The Hoare-Rahman papers dealt

with exactly solvable models where the eigenvalues and eigenfunctions

have been found explicitly. Such questions led in a very natural way to

certain kernels involving the Hahn and Krawtchouk polynomials. These

kernels were reproducing kernels which take nonnegative values. More

general bilinear forms involving orthogonal polynomials also appeared.

The question of positivity of these kernels became important and Mizan

started corresponding with R. Askey who, with G. Gasper, was working

on positivity questions at the time and they were very knowledgeable

about these questions. Through Askey and Gasper, Mizan Rahman was

attracted to the theory of special functions and eventually to q-series. He

mastered the subject very quickly and started contributing regularly to

the subject. Within a few years, Mizan had become a world's expert in

the theory of special functions in general and q-series in particular. It is

appropriate here to quote from Mike Hoare's e-mail how he described the

beginning of this activity. Mike wrote "After we had done some work on

(Rayleigh Piston)

. .

happened to mention a problem which I have

been worrying away at for some years. This disarmingly simple notion

arose from energy transfer in chemical kinetics (the Kassell model). Re-

formulated

a discrete 'urn model,' it corresponds to a Markoff chain

for partitioning balls in boxes in which only a subset are randomized in

each event. My eigenvalue solution for the simplest continuous case in

Laguerre polynomials led to probability kernels (which are) effectively

Mizan Rahman, his Mathematics and Literary Writings

the same as those seen in the formulas of Erddyi and Kogbetliantz in the

1930's special function theory." He then added "Once Mizan's interest

was stimulated, he was off and running, with the early series of abstract

papers you well know." Mike Hoare echoed the feelings of those of us

who collaborated with Mizan when he wrote "To see Mizan at work was

an amazing experience. He seldom had to cross anything out and [in]

what seemed no time at all the sheets in his characteristically meticulous

script would be delivered with a modest little gesture of triumph."

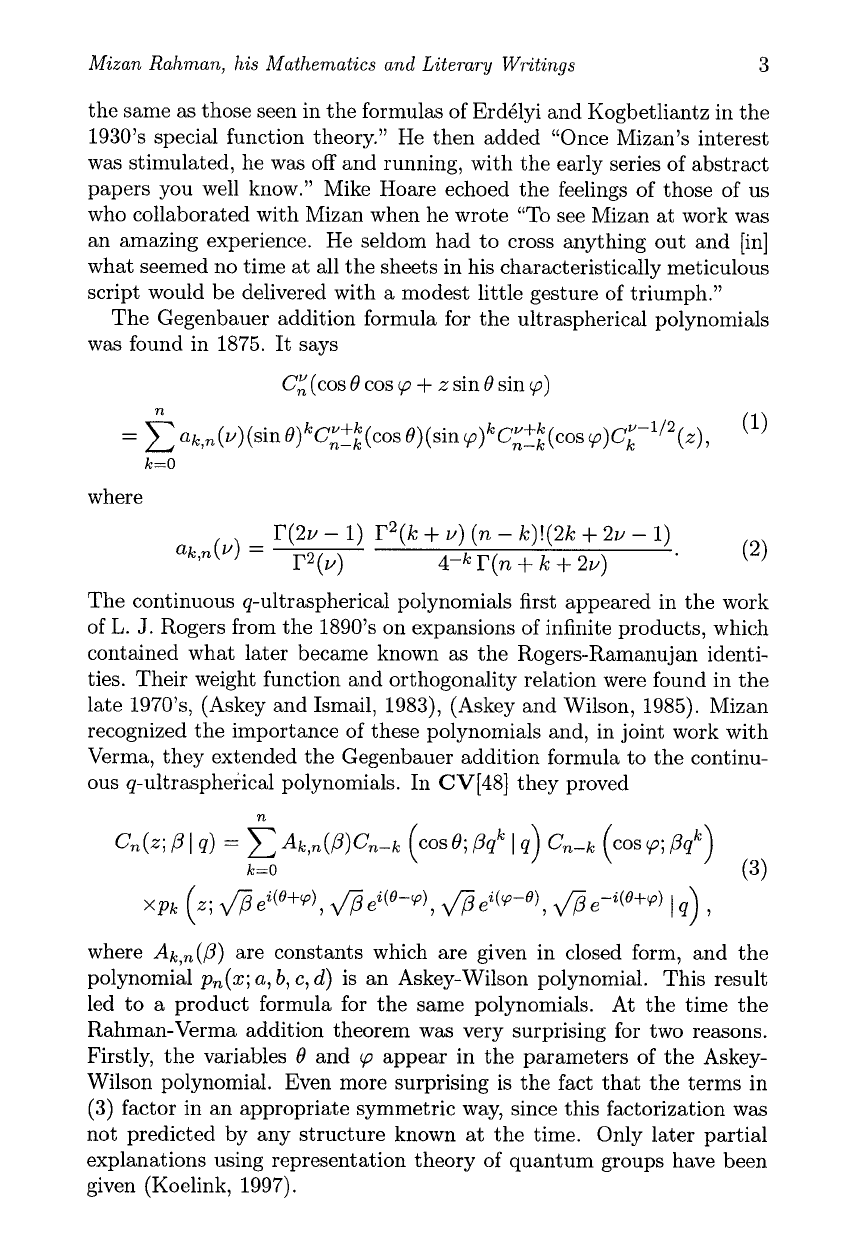
The Gegenbauer addition formula for the ultraspherical polynomials

was found in 1875. It says

C,"

(cos

cos

sin

sin cp)

akYn (v) (sin 6)

(cos 6) (sin cp)

c::;

(COB

CL-"~

(z)

(I)

where

The continuous q-ultraspherical polynomials first appeared in the work

Rogers from the 1890's on expansions of infinite products, which

contained what later became known as the Rogers-Ramanujan identi-

ties. Their weight function and orthogonality relation were found in the

late 1970's, (Askey and Ismail, 1983), (Askey and Wilson, 1985). Mizan

recognized the importance of these polynomials and, in joint work with

Verma, they extended the Gegenbauer addition formula to the continu-

ous q-ultraspherical polynomials. In CV[48] they proved

where Ak,n(P) are constants which are given in closed form, and the

polynomial pn(x; a,

is an Askey-Wilson polynomial. This result

led to a product formula for the same polynomials. At the time the

Rahman-Verma addition theorem was very surprising for two reasons.

Firstly, the variables 6 and

appear in the parameters of the Askey-

Wilson polynomial. Even more surprising is the fact that the terms in

(3)

factor in an appropriate symmetric way, since this factorization was

not predicted by any structure known at the time. Only later partial

explanations using representation theory of quantum groups have been

given (Koelink, 1997).

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Askey (Askey, 1970) raised the question of finding the domain of

(a, p) within

-1,

-1

which makes the linearization coefficients

a(m, n, k) in

m+n

(.,a)

P~~~(X)P~~~)(X)=

a(m,n,k)Pk (4)

k=lm-n)

nonnegative.

Hylleraas (Hylleraas, 1962) showed that the coefficients

a(m, n, k) satisfy a three-term recurrence relation, and showed that the

case

,f3

leads to a closed form solution, as was the case when

For other (a, P) (except

-+),

the coefficients were repre-

sented as double sums, and this expression cannot be used for any of

the applications the writers know except for computing a few of the co-

efficients. In (Gasper, 1970a) and (Gasper, 1970b), G. Gasper used the

recurrence relation of Hylleraas to solve the problem of the positivity

of these coefficients. Mizan started working on extending Gasper's re-

sults to the continuous q-Jacobi polynomials, where the problem is much

more difficult. Rahman CV[27] identified a(m, n, k) as a

gF8

function

and then used the gF8-representation to prove the nonnegativity of the

linearization coefficients. Later Mizan CV[30] used the same technique

to identify the q-analogue of a(m, n,

as a

locpg

and establish its non-

negativity for

(a,

in a certain subset of

(-1,

m).

The linearization coefficients in (4) are integrals of products of three

Jacobi polynomials. Din (Din, 1981) proved that

Pm(x)Pn(x)Qn(x)dx

for m

(5)

-1

where {Pn(x)} are Legendre polynomials and {Qn(x)) are Legendre

functions of the second kind. Askey, Koornwinder and Rahman CV[50]

extended this to the ultraspherical polynomials. Rahman and Shah

CV[39] summed the series, which is dual to (5), namely

F(Q,~)$):=C(~+~/~)P~(COS~)P~(COS~)Q~(COS$),

(6)

n=O

cp,$

7r.

They proved that F(8, cp,$)

for 18

cpl

7r,

but F(8,

cp,

cpl,

27r

and

8+p+$

27r. On the other hand, F(8,

cp,

-all2,

8+cp.

In the above

sin((8

$)/2) sin((8

$)/2)

sin((8

$)/2) sin((cp

8)/2).

(7)

Mizan Rahman, his Mathematics and Literary Writings

They also extended it to the ultraspherical polynomials. In CV[40],

this was extended to the case where the sum involves a product of three

ultraspherical polynomials and an ultraspherical function of the second

kind. In CV[51], Rahman and Verma extended CV[39] in a different

direction where it involved products of two q-ultraspherical polynomials

and a q-ultraspherical function.

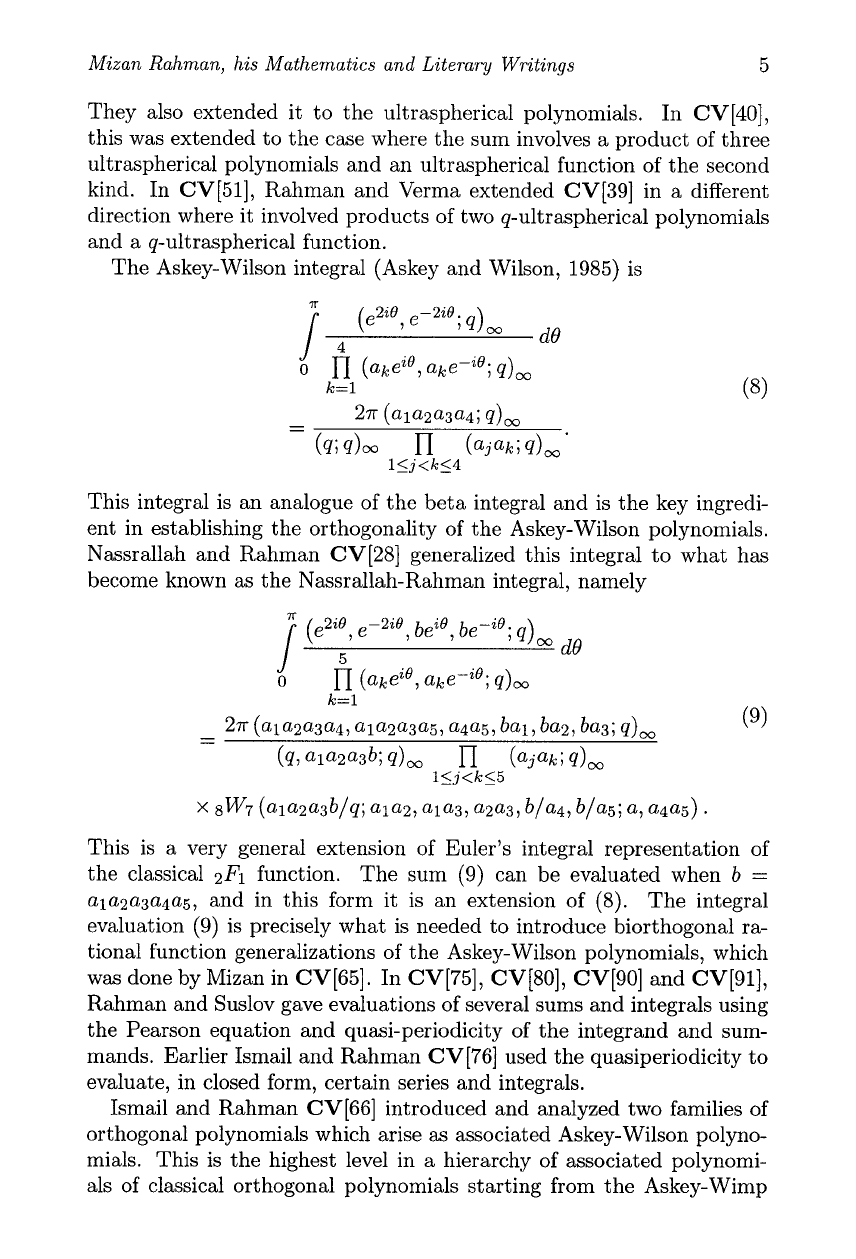
The Askey-Wilson integral (Askey and Wilson, 1985) is

This integral is an analogue of the beta integral and is the key ingredi-

ent in establishing the orthogonality of the Askey-Wilson polynomials.

Nassrallah and Rahman CV[28] generalized this integral to what has

become known as the Nassrallah-Rahman integral, namely

This is a very general extension of Euler's integral representation of

the classical

2F1

function. The sum

(9)

can be evaluated when

ala2a3a4as, and in this form it is an extension of (8). The integral

evaluation (9) is precisely what is needed to introduce biorthogonal ra-

tional function generalizations of the Askey-Wilson polynomials, which

was done by Mizan in CV[65]. In CV[75], CV[80], CV[90] and CV[91],

Rahman and Suslov gave evaluations of several sums and integrals using

the Pearson equation and quasi-periodicity of the integrand and sum-

mands. Earlier Ismail and Rahman CV[76] used the quasiperiodicity to

evaluate, in closed form, certain series and integrals.

Ismail and Rahman CV[66] introduced and analyzed two families of

orthogonal polynomials which arise as associated Askey-Wilson polyno-

mials. This is the highest level in a hierarchy of associated polynomi-

als of classical orthogonal polynomials starting from the Askey-Wimp

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

and Ismail-Letessier-Valent associated Hermite and Laguerre polynomi-

als from the mid-1980's. The Askey-Wilson polynomials are birth and

death process polynomials, so their associated polynomials are also birth

and death process polynomials. The death rate at zero population,

PO,

is either zero or follows the pattern of

p,.

Each definition of po leads

to a family of orthogonal polynomials. Surprisingly, thanks to Mizan's

insight and amazing computational power, one can get not only closed

form representations for both families of polynomials, but also find the

orthogonality measures of both families explicitly. The closed form ex-

pressions represent the polynomials in terms of the Askey-Wilson basis

{(aeie, aeVie; q)n). The coefficients are

lo(p+

No other choice of po

leads to orthogonal polynomials where the coefficients of their expan-

sion in the Askey-Wilson basis is a single sum. The paper also gives a

basis of solutions to the recurrence relation satisfied by the polynomials.

Rahman and Tariq derived a Poisson and related kernels for associated

continuous q-ultraspherical polynomials in CV[86] and reproducing ker-

nels for associated Askey-Wilson polynomials in CV[88]. Earlier, Mizan

CV[78] found generating functions for the Askey-Wilson polynomials. In

CV[41] Mizan gave a q-analogue of Feldheim's kernel (Feldheim, 1941)

which involves q-utraspherical polynomials. An integral representation

analogous to the Weyl fractional integral in Fourier analysis is in CV[99].

Recall the notation

j(t)

(x), where x

t-')

12,

and the

Askey- Wilson operator

where e(x)

x. Ismail raised the question of finding Vgl, a right

inverse to Vq on different

spaces weighted by weight functions of

different classical q-orthogonal polynomials. In CV[79] Ismail, Rahman

and Zhang found an integral representation for Dil on

weighted by

the weight function of the continuous q-Jacobi polynomials. They then

proved that the spectrum of the compact operator Dil is discrete and

described completely the eigenvalues and eigenfunctions. This led to a

generalization of the plane wave expansion in (Ismail and Zhang, 1994).

Ismail and Rahman CV[100] found an integral representation of Vgl

weighted by the Askey-Wilson weight function. The kernel in

this integral representation turned out to be very simple and all the

complications are absorbed in a constant.

Mizan has served the mathematical community very well. He is a reg-

ular referee for many mathematics and physics journals. He co-organized

a major meeting on special functions at the Fields Institute in Toronto

Mizan Rahman, his Mathematics and Literary Writings

in 1994 and co-edited its proceedings. One of the most important ways

a mathematician can serve is in writing books which are needed. Gasper

and Rahman (Gasper and Rahman, 1990) did this, and a second edi-

tion will appear shortly. The new edition will not only have more on

q-series, but will contain a chapter on new work on elliptic hypergeomet-

ric series (F'renkel and Turaev, 1997), a very interesting new extension

of hypergeometric and basic hypergeometric series. This extended the

earlier trigonometric case in (F'renkel and Turaev, 1995). The Gasper-

Rahman book also contains a treatment of the

lops

biorthogonal rational

functions CV[65] and its elliptic extensions. The book started because,

according to George Gasper "Mizan was tired of having to repeatedly

search papers for known formulas involving basic hypergeometric func-

tions that were not contained in the books by Bailey or Slater." Mizan

then suggested that he and Gasper should write an up-to-date book on

basic hypergeometric functions. A first outline of this book dates back

to 1982. Their book has become a much-cited classic, and Mizan and

George have rendered the mathematical community a great service in

writing this book.

Not only did Mizan co-author the definitive book on q-series, but he

also wrote valuable review articles CV[64], CV[87], CV[97] and CV[98].

To the best of our knowledge, CV[98] is the first article which collects

all the recent developments on associated orthogonal polynomials, which

makes it a very valuable reference and teaching source.

Mizan's scientific contributions have been recognized and acknowl-

edged. Part of his dissertation was included in a book on gases and

plasmas by Wu (Wu, 1966).

special session was held in his honor at

the the annual meeting of the American Mathematical Society held in

Baltimore, Maryland. The session was well attended and highly success-

ful. Several speakers expressed their mathematical debt to Mizan and

noted his generosity with his ideas. He has helped younger mathemati-

cians with suggestions and specific ideas on how to overcome certain

hurdles and would not have his name as a joint author of the resulting

paper(s). Mizan's contributions are well-appreciated by people working

in special functions and related areas. R. W. (Bill) Gosper put it well

when he wrote on April 7, 2004 "I can't begin to estimate Mizan Rah-

man's prowess

a q-slinger. All

know is that he alone could 'q' any

hypergeometric identity that I could find. Sometimes the q-form was so

unimaginable that I would have bet money there was none." He then

added "And yet the memory that stands out was not a q.

exhibited to

the usual gang of maniacs a really mysterious-looking infinite trig prod-

uct identity, dug up with Macsyma. It wasn't even obvious that the nth

term converged to

And that gentle man completely stung me with a