Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.


58
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Theorem
5.4.
N+m+l-ks
x([
m-ks
Proof.
Set a
=
q in Corollary 4.3 and invoke Lemma 5.2 for the inner
sum with
t
=
s.
0
6.
Conclusion
The primary object of this paper has been the development of a-
Gaussian polynomials. In light of their natural partition-theoretic in-
terpretation (Proposition 2.7)) it is surprising that they have not been
studied previously. It seems extremely likely that Proposition 2.7 has
already suggested itself to many workers. The first thing one notices is
that for a-Gaussian polynomials there is no lovely product formula like
(1.8) only a less satisfying sum (Proposition 2.8) which reduces to (1.8)
when a
=
q. In addition, the symmetry identity (Andrews, 1976, p. 35,
eq. (3.3.2))
has no simple analog for a-Gaussian polynomials. It may well be that
these two deficits discouraged further investigation especially in light of
the fact that the definition of a-Gaussian polynomials contains a sum
that is not naturally terminating.
A secondary object of this paper has been the study of the polynomial
refinements of "a-generalizations" of Rogers-Ramanujan type identities.
Such studies almost always have in mind (or, at least, in the back of
their mind) the famous Borwein conjecture (Andrews, 1995). Namely, if
then each of An(q), B,(q) and Cn(q) has non-negative coefficients.
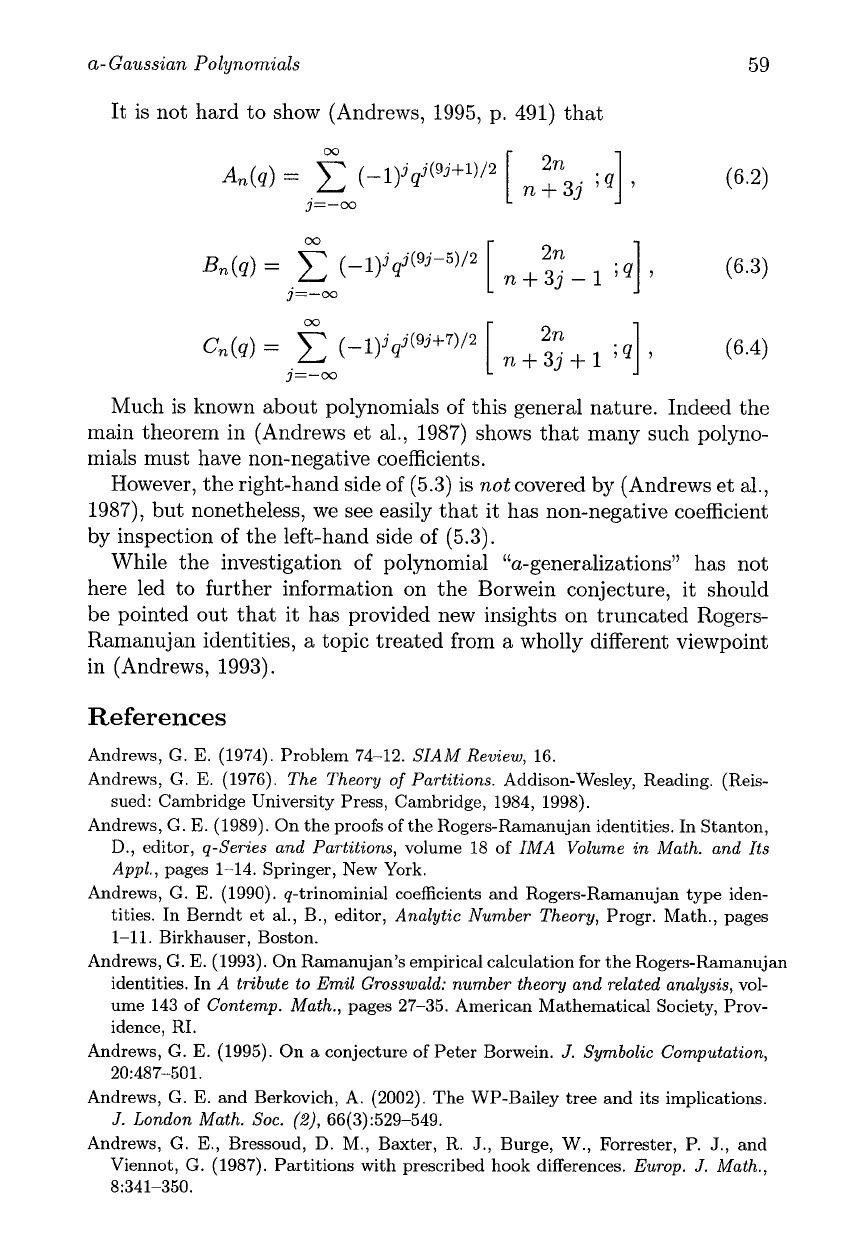
a-Gaussian Polynomials
It is not hard to show (Andrews, 1995, p. 491) that
Much is known about polynomials of this general nature. Indeed the
main theorem in (Andrews et al., 1987) shows that many such polyno-
mials must have non-negative coefficients.
However, the right-hand side of (5.3) is
not
covered by (Andrews et al.,
1987), but nonetheless, we see easily that it has non-negative coefficient
by inspection of the left-hand side of (5.3).
While the investigation of polynomial "a-generalizations" has not
here led to further information on the Borwein conjecture, it should
be pointed out that it has provided new insights on truncated Rogers-
Ramanujan identities, a topic treated from a wholly different viewpoint
in (Andrews, 1993).
References
Andrews,
G.
E.
(1974). Problem 74-12. SIAM Review, 16.
Andrews,
G.
E.
(1976). The Theory of Partitions. Addison-Wesley, Reading. (Reis-
sued: Cambridge University Press, Cambridge, 1984, 1998).
Andrews,
G.
E.
(1989). On the proofs of the Rogers-Ramanujan identities. In Stanton,
D.,
editor, q-Series and Partitions, volume 18 of IMA Volume in Math. and Its
Appl., pages 1-14. Springer, New York.
Andrews,
G.
E.
(1990). q-trinominial coefficients and Rogers-Ramanujan type iden-
tities. In Berndt et al., B., editor, Analytic Number Theory, Progr. Math., pages
1-11.
Birkhauser, Boston.
Andrews,
G.
E.
(1993). On Ramanujan's empirical calculation for the Rogers-Ramanujan
identities. In A tribute to Emil Grosswald: number theory and related analysis, vol-
ume 143 of Contemp. Math., pages 27-35. American Mathematical Society, Prov-
idence, RI.
Andrews,
G.
E.
(1995). On
a
conjecture of Peter Borwein.
J.
Symbolic Computation,
20:487-501.
Andrews,
G.
E.
and Berkovich, A. (2002). The WP-Bailey tree and its implications.
J.
London Math. Soc.
(2),
66(3):529-549.
Andrews,
G.
E.,
Bressoud,
D.
M., Baxter, R. J., Burge, W., Forrester, P. J., and
Viennot,
G.
(1987). Partitions with prescribed hook differences. Europ.
J.
Math.,
8:341-350.

60
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Bailey, W.
N.
(1949). Identities of the Rogers-Ramanujan type. Proc. London Math.
SOC.
(2),
5O:l-10.
Bressoud,
D.
M. (1981a). Solution to problem 74-12. SIAM Review, 23:lOl-104.
Bressoud,
D.
M. (1981b). Some identities for terminating q-series. Math. Proc. Camb.
Phil. SOC., 89:211-223.
Paule, P. (1994). Short and easy computer proofs of the Rogers-Ramanujan identities
and identities of similar type. Electronic
J.
Combin., 1:Research Paper 10, approx.
9 pp. (electronic).
Schur, I. (1917). Ein beitrag zur additiven zahlentheorie. In S.-B. Preuss. Akad.
Wiss., Phys.-Math. Klasse, pages 302-321. (Reprinted: Gesamm, Abhand., Vol.
2, Springer, Berlin, 1973, pp. 117-136).
Stembridge,
J.
(1990). Hall-Littlewood functions, plane partitions, and the Rogers-
Ramanujan identities. Trans. Amer. Math. Soc., 319:469-498.
Warnaar, S.
0.
(2002). Partial sum analogs of the Rogers-Ramanujan identities.
J.
Comb. Th. (A), 99:143-161.
Watson, G.
N.
(1929).
A
new proof of the Rogers-Ramanujan identities.
J.
London
Math. Soc., 4:4-9.
Zeilberger (a.k.a.
S.
B. Ekhad
and
S. Tre),
D.
(1990).
A
purely verification proof of
the first Rogers-Ramanujan identity.
J.
Comb. Th. (A), 54(2):309-311.
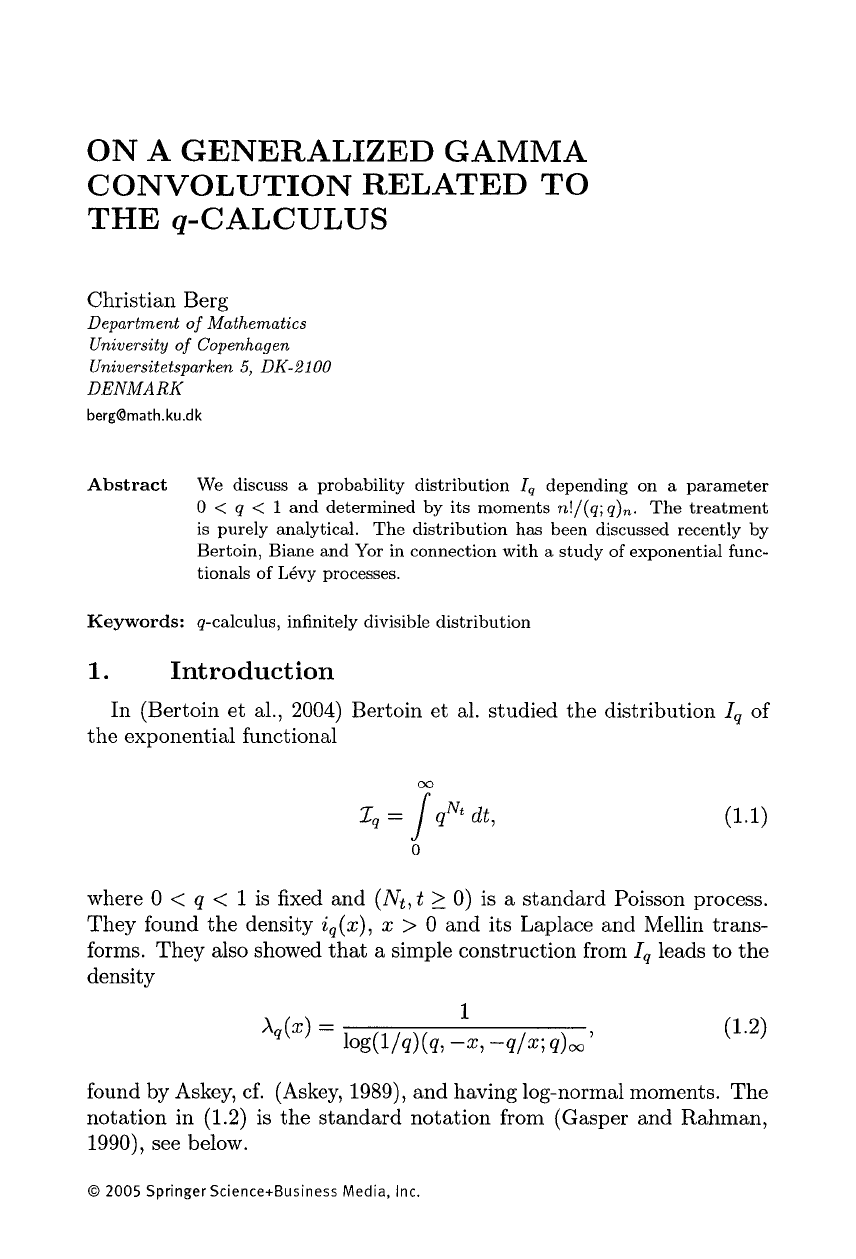
ON A GENERALIZED GAMMA
CONVOLUTION RELATED TO
THE q-CALCULUS
Christian Berg
Department
of
Mathematics
University of Copenhagen
Universitetsparken
5,
DK-2100
DENMARK
berg@math.ku.dk
Abstract
We discuss a probability distribution
Iq
depending on a parameter
0
<
q
<
1
and determined by its moments n!/(q;
q)n.
The treatment
is purely analytical. The distribution has been discussed recently by
Bertoin, Biane and Yor in connection with a study of exponential func-
tional~ of Lkvy processes.
Keywords: q-calculus, infinitely divisible distribution
1.
Introduction
In (Bertoin et al., 2004) Bertoin et al. studied the distribution
I,
of
the exponential functional
Iq
=
J
qN'
dt,
0
where 0
<
q
<
1
is fixed and
(Nt,
t
2
0) is
a
standard Poisson process.
They found the density
i4(x),
x
>
0 and its Laplace and Mellin trans-
forms. They also showed that a simple construction from
Iq
leads to the
density
found by Askey, cf. (Askey, 1989), and having log-normal moments. The
notation in (1.2) is the standard notation from (Gasper and Rahman,
1990), see below.
O
2005
Springer Science+Business Media, Inc.

62
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
The distribution
Iq
has also appeared in recent work of Cowan and
Chiu (Cowan and Chiu, 1994), Dumas et al. (Dumas et al., 2002) and
Pakes (Pakes, 1996).
The proofs in (Bertoin et al., 2004) rely on earlier work on exponential
functionals which use quite involved notions from the theory of stochastic
processes, see (Carmona et al., 1994; Carmona et al., 1997).
The purpose of this note is to give a self-contained analytic treatment
of the distribution
Iq
and its properties.
In Section
2
we define a convolution semigroup (Iq,t)t,O of probabil-
ities supported by [0, m[, and it is given in terms of the corresponding
Bernstein function
f
(s)
=
log(-s; q), with LBvy measure v on 10, m[
having the density
dv
1
00
-
=
-
C
exp (-xqdn)
.
dx x
n=O
The function
1/
log(-s; q), is a Stieltjes transform of a positive measure
which is given explicitly, and this permits us to determine the potential
kernel of
The measure
Iq
:=
Iq,~
is a generalized Gamma convolution in the
sense of Thorin, cf. (Thorin, 197713; Thorin, 1977a). The moment se-
quence of
I,
is shown to be n!/(q; q),, and the nth moment of
IqTt
is a
polynomial of degree n in t. We give a recursion formula for the coeffi-
cients of these polynomials. We establish that
Iq
has the density
A
treatment of the theory of generalized Gamma convolutions can be
found in Bondesson's monograph (Bondesson, 1992). The recent paper
(Biane et al., 2001) contains several examples of generalized Gamma
convolutions which are also distributions of exponential functionals of
LBvy processes.
We shall use the notation and terminology from the theory of basic
hypergeometric functions for which we refer the reader to the monograph
by Gasper and Rahman (Gasper and Rahman, 1990). We recall the q-
shifted factorials
and (z; q)o
=
1. Note that (z; q), is an entire function of z.

On a generalized Gamma convolution related to the q-calculus 63
For finitely many complex numbers zl,
22,
. . .
,
zp we use the abbrevi-
ation
(zl,z2,. zp; q),
=
(21; 4)n (22; 4)n. (~p; q)n.
The q-shifted factorial is defined for arbitrary complex index
X
by
and this is related to Jackson's function
rq
defined by
In Section
3
we introduce the entire function
and use it to express the Mellin transform of
Iq.
the density
Xq
&ven in (1.2) can be written as the product convolution
of
I,
and another related distribution, see Theorem 3.2 below. The
Mellin transform of the density
Xq
can be evaluated as a special case
of the Askey-Roy beta-integral given in (Askey and Roy, 1986) and in
particular we have, see also (Askey, 1989):
The value of (1.5) is an entire function of c and equals h(c)h(l-c)/(q; q),.
The following formulas about the q-exponential functions, cf. (Gasper
and Rahman, 1990), are important in the following:
qn(n-1)/2Zn
Eqb)
=
c
=
(-2; q),, z
E
@.
(a; q)n
(1.7)
n=O
2.
The analytic method
We recall that a function
cp
:
10, m[
I+
[0, m[ is called completely
monotonic, if it is
Cw
and (-l)kcp(k)(~)
2
0 for
s
>
0,
k
=
0,1,. . . .
By the Theorem of Bernstein completely monotonic functions have the
form
00
~(s)
=
J
e-sx dol(x),
(2.1)
0

64
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
where
a
a non-negative measure on [0, m[. Clearly
cp(O+)
=
a([O,
m[).
The equation (2.1) expresses that
cp
is the Laplace transform of the
measure a.
To establish that a probability q on [O,
oo[
is infinitely divisible, one
shall prove that its Laplace transform can be written
I
e-"" dq(x)
=
exp(-
f
(s)), s
>
0,
0
where the non-negative function
f
has a completely monotonic deriv%
tive. If q is infinitely divisible, there exists a convolution semigroup
(qt)t>o of probabilities on [0, m[ such that q1
=
q and it is uniquely
determined by
cf. (Berg and Forst, 1975), (Bertoin, 1996). The function
f
is called the
Laplace exponent or Bernstein function of the semigroup. It has the
integral representation
where
a
>
0 and the LBvy measure
v
on 10,
oo[
satisfies the integrability
condition
J
x/(1+ x) dv(x)
<
oo.
If
f
is not identically zero the convo-
00
lution semigroup is transient with potential kernel
K
=
J
qt dt, and the
0
Laplace transform of
K
is
l/
f
since
The generalized Gamma convolutions q are characterized among the
infinitely divisible distributions by the following property of the corre-
sponding Bernstein function
f,
namely by
f'
being a Stieltjes transform,
i.e. of the form

On a generalized Gamma convolution related to the q-calculus
65
where
a
2
0 and
p
is a non-negative measure on
[O,
XI[.
The relation
between
p
and
v
is that
This result was used in (Berg, 1981) to simplify the proof of a theo-
rem of Thorin (Thorin, 197713)) stating that the Pareto distribution is a
generalized Gamma convolution.
Theorem
2.1.
Let
0
<
q
<
1
be fixed. The function
00
f (s)
=
log(-s; q),
=
C
log(1
+
sqn),
s
t
0
(2.4)
n=O
is a Bernstein function. The corresponding convolution semigroup
((Iq,t)t,o)
consists of generalized Gamma convolutions and we have
00
The potential kernel
K~
=
Iq,t
dt has the following completely mono-
0
tonic density
Xq(x)
=
1
-
q
+
pcpq(Y) dY.
(2.6)
1
where
cp
is the continuous function
Proof.
The function
f
defined by (2.4) has the derivative
showing that
f'
is a Stieltjes transform with
a
=
0,
and
p
is the discrete
measure with mass
1
in each of the points
qen,
n
2
0. In particular
f
is
a Bernstein function with
a
=
0
and L6vy measure given by (1.3).
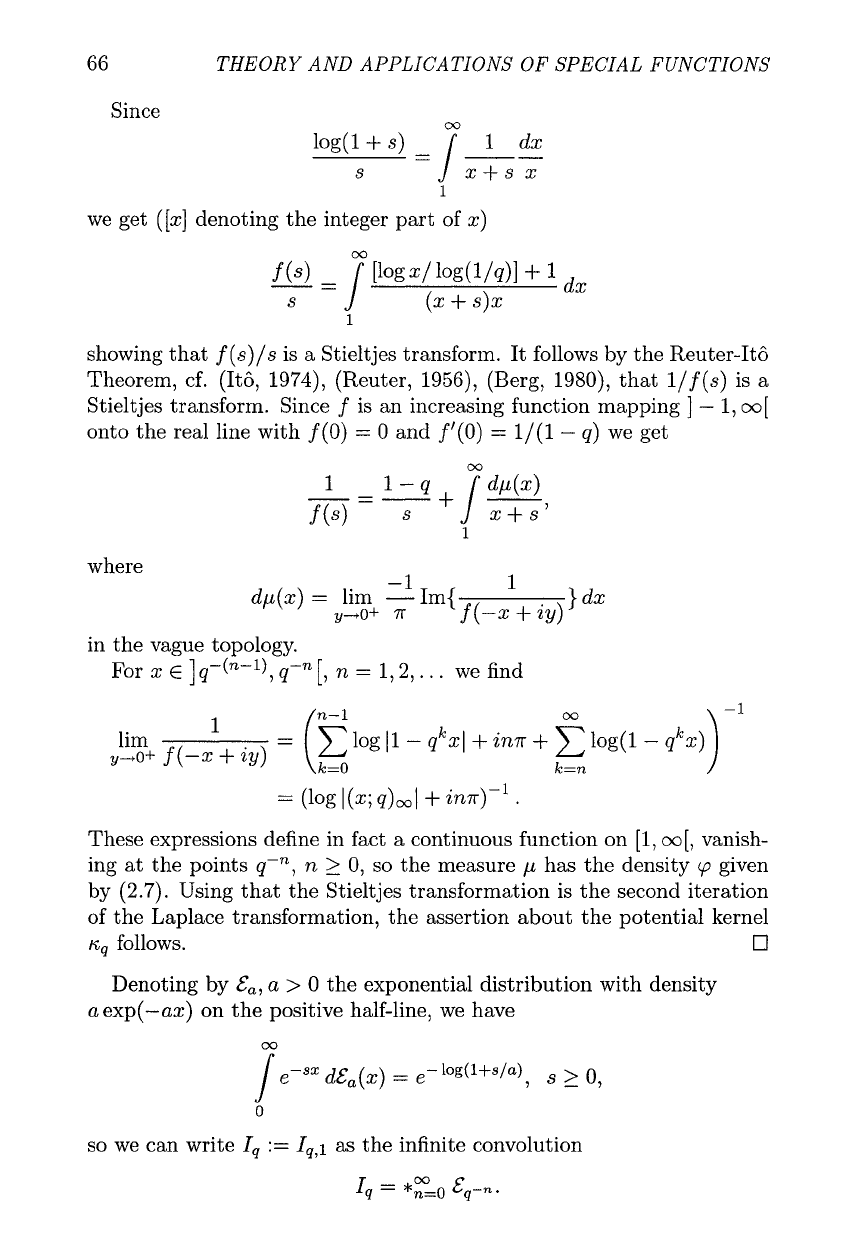
66
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Since
10
+
s)
1
dx
--
s x+s x
1
we get
([XI
denoting the integer part of x)
showing that
f
(s)/s is a Stieltjes transform. It follows by the Reuter-It6
Theorem, cf. (It6, 1974), (Reuter, 1956), (Berg, 1980), that
l/
f(s) is a
Stieltjes transform. Since
f
is an increasing function mapping
]
-
1,
oo[
onto the real line with
f
(0)
=
0 and f'(0)
=
1/(1-
q) we get
in the vague topology.
or
x ~]q-(~-l),q-~[, n
=
l,2,.
. .
we find
=
(log
1
(x; q),
1
+
inn)-'
These expressions define in fact a continuous function on [I,
m[,
vanish-
ing at the points q-n, n
>
0, so the measure
p
has the density
cp
given
by
(2.7).
Using that the Stieltjes transformation is the second iteration
of the Laplace transformation, the assertion about the potential kernel
6,
follows.
0
Denoting by
I,,
a
>
0 the exponential distribution with density
aexp(-ax) on the positive half-line, we have
so we can write
I,
:=
I,,J
as the infinite convolution
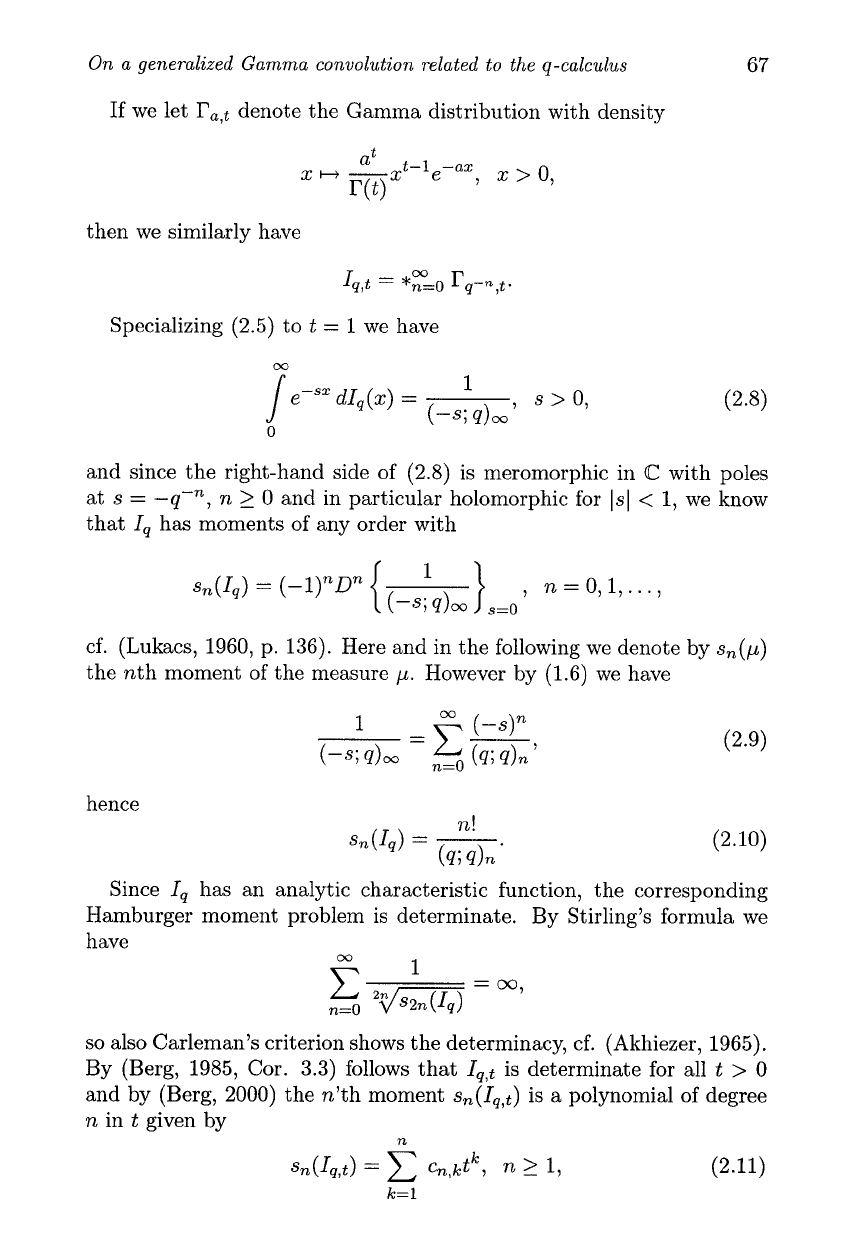
On a generalized Gamma convolution related to the q-calculus
67
If we let
ralt
denote the Gamma distribution with density
then we similarly have
Specializing (2.5) to
t
=
1
we have
and since the right-hand side of (2.8) is meromorphic in
C
with poles
at
s
=
-qWn, n
2
0
and in particular holomorphic for
1st
<
1,
we know
that
I,
has moments of any order with
cf. (Lukacs, 1960, p. 136). Here and in the following we denote by sn(p)
the nth moment of the measure p. However by (1.6) we have
hence
Since
Iq
has an analytic characteristic function, the corresponding
Hamburger moment problem is determinate. By Stirling's formula we
have
so also Carleman's criterion shows the determinacy, cf. (Akhiezer, 1965).
By (Berg, 1985, Cor. 3.3) follows that
Iq,t
is determinate for all
t
>
0
and by (Berg, 2000) the n'th moment
s,(I~,~)
is a polynomial of degree
n in
t
given by
n
