Keyfitz N., Caswell H. Applied Mathematical Demography

Подождите немного. Документ загружается.

16.6. Parental Control over Sex of Children 435

We could have anticipated this by noting that to move up one girl takes

on the average 1/g children; hence to move up two girls takes 2/g children.

To move up three girls takes an average of 3/g children, and similarly for

higher numbers. The cells for boys only, across the top of the diagram, are

the same but with b replacing g.

Hence the expectations shown on the right-hand points and across the

top of the diagram; having attached probabilities to these boundary points,

we now turn to the interior. Consider the interior point marked A in Figure

16.5, standing for a couple wishing one girl and one boy, either altogether

or in addition to the children they already have.

Suppose they try for a girl ﬁrst. Then the chance of moving up in the

diagram is g, and if they succeed they reach the point just above, from

which the expectation is 1/b. If they fail they reach the point at the right,

from which the expectation is 1/g. Hence the total expected number of

children from point A is

= g





+(1− g)





where 1 has been added within the parentheses to allow for the girl or boy

born in the move. On cancellation this reduces to

and with b =0.8andg =0.7equals0.7/0.8+1/0.7=2.30.

Using the opposite strategy of trying for a boy ﬁrst (Figure 16.5) would

give

= b





+(1− b)





or, with our numbers, 0.8/0.7+1/0.8=2.39. Evidently the right strategy

is to try for a girl ﬁrst. This suggests a general rule: parents should leave

the more controllable contingency to the last if they seek equal numbers of

boys and girls.

We want always to choose the strategy that gives the minimum number

of total children, for example, that corresponds to

Min





If b>g, that is, if we are surer to get a boy if we aim at a boy than a girl

if we aim at a girl, it is easily seen by algebra that b/g +1/b > g/b +1/g.

This is so because, if b>g>

,thenb −

>g−

and (b −

)

> (g −

)

or b

−b>g

−g; therefore b

+ g>g

+ b, and, on dividing both sides by

bg, b/g +1/b > g/b +1/g.Ifb>gwe should try for a girl ﬁrst to minimize

total children.

The ability of parents to inﬂuence the sex of their children will reduce the

average number of oﬀspring for parents targeting on a minimum number of

436 16. Microdemography

Want one

(more)

girl

Want one

(more)

boy Target

1/g

1/b

A = Min (A

, A

)

= b

(

1 +

)

+ (1 – b)

(

1 +

)

= +

= g

(

1 +

)

+ (1 – g)

(

1 +

)

= +

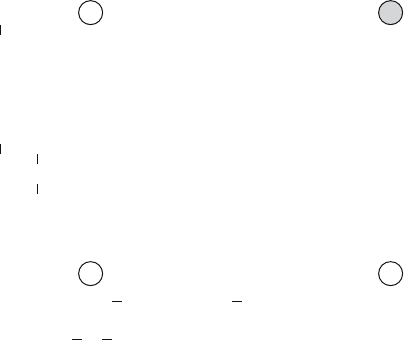
Figure 16.5. Enlargement of part of Figure 16.4, showing expected children for

couple wanting one boy and one girl (position A). Trying for a boy ﬁrst gives an

expected A

= b(1 + 1/g)+(1− b)(1 + 1/b)=b/g +1/b children. Trying for a

girl ﬁrst gives A

(1 + 1/b)+(1−g)(1 + 1/g)=g/b +1/g children, where b is the

chance of having a boy if the couple try for a boy, and g is the chance of having

a girl if they try for a girl.

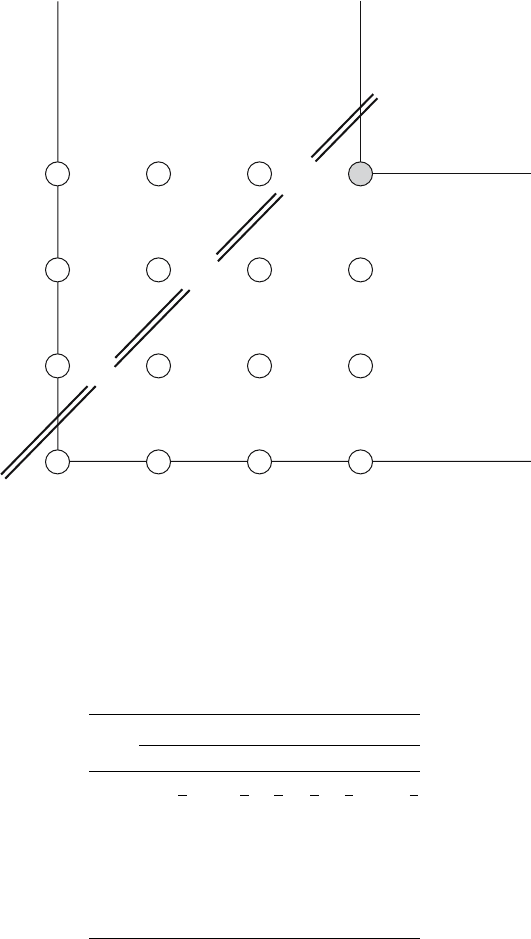
boys and of girls. Figure 16.6 shows expected numbers from various points

with b =0.8,g=0.7, and in parentheses with b =0.5,g=0.5.

Let us see how sensitive the result is to the degree of reliability with

which sex is determined. Table 16.4 shows the cases of parents aiming at

one boy, and at one boy and one girl. Making b = g =0.5, 0.6,..., we

see the average number of children they will have. If b = g =0.8 rather

than b = g =0.5, the expected total drops from 2.0 to 1.25 for couples

aiming at one boy, and from 3.0 to 2.25 for couples aiming at one boy and

one girl. In both cases the reliability of 0.8 in sex determination eliminates

three-quarters of the unwanted children.

A number of surveys have asked newly married couples how many boy

and how many girl children they want. One such survey in Hull, England,

showed 45.7 percent wanting one boy and one girl, 15.4 two boys and one

girl, and 12.6 two boys and two girls; the average was 2.55 children. Under

present circumstances, that is to say with b = g =0.5 approximately,

we ﬁnd by applying the numbers in parentheses in Figure 16.6 to these

percentages that the average attained would be 3.74 children, or 1.19 more

than wanted (Table 16.5).

16.6. Parental Control over Sex of Children 437

Boys

Girls

Try for boy first

Try for girl first

Absorbing barrier

3 2 1 0 boys

3210

girls

Try for boy first

Try for girl first

Aiming at

b = 0.8

g = 0.7

3.75

(6.00)

4.424

(6.25)

5.390

(6.88)

6.418

(7.88)

2.50

(4.00)

3.343

(4.50)

4.381

(5.50)

5.482

(6.88)

1.25

(2.00)

2.304

(3.00)

3.471

(4.50)

4.714

(6.25)

1.43

(2.00)

2.86

(4.00)

4.29

(6.00)

Figure 16.6. Expected number of children for various family objectives from zero

boys and zero girls up to three boys and three girls, when b =0.8,g=0.7, and

(in parentheses) when b = g =0.5.

Table 16.4. Expected number of children born to couples who stop after one boy,

and who stop after one boy and one girl

Expected total stopping after

One boy One boy and one girl

b = g

=1+

0.5 2.0 3.0

0.6 1.67 2.67

0.7 1.43 2.43

0.8 1.25 2.25

0.9 1.11 2.11

1.0 1.00 2.00

With b =0.8,g =0.7, numbers chosen because they are about halfway

to complete sex control represented by b = g =1.0, the average would be

2.88 children, or only 0.33 more than wanted.

438 16. Microdemography

A technology that goes halfway to sex control would eliminate nearly

three-quarters of the unwanted children born because of the sex preferences

of parents. Full sex control would reduce the birth rate for these parents

by 32 percent; half sex control would reduce their birth rate by 23 percent.

Five kinds of qualiﬁcation are required. The least important is that chil-

dren are born one at a time in the model, which has no easy way of taking

account of multiple births. Moreover, not all parents have preferences re-

garding the sex composition of their families, and the model deals only with

parents whose preferences are strong enough to be overriding. In fact, they

must be willing and able to have an inﬁnite number of children if necessary.

The model also supposes that the parental decision regarding composition

is made at the outset rather than in stages, or child by child. It supposes

homogeneity—that all parents have the same chances b and g.

The advent of partial or complete control of the sex of children would

have the eﬀect of changing the sex of the new generation, in some cultures

in favor of boys; in those cultures the advent of a high sex ratio in the new

generation would reduce the number of marriages; when the children came

to childbearing age marriages would be limited by the less numerous sex.

Unwed males would decrease the overall birth rate. But well before that

came about the culture would shift in favor of the initially less favored sex.

Within a decade or so of the birth of a disproportionate number of boys

couples would come to value girls more highly. Perhaps a series of waves

would ensue, not unlike those familiar in a market economy arising from

the interval of time between capital investments and the returns therefrom

(Westoﬀ and Rindfuss 1974). For an insightful analysis of some of the de-

mographic consequences of sex selection in recent China, see Tuljapurkar

et al. (1995).

Table 16.5. Application of average numbers of expected children to sex preferences

of parents in survey in Hull, England

Number of children

Average

Average born with

Parents’ born with b =0.8,

preferences Percent Wanted b = g =

g =0.7

One boy, one girl 45.7 2 3.0 2.304

Two boys, one girl 15.4 3 4.5 3.343

Two boys, two girls 12.6 4 5.5 4.381

Average of survey 2.55 3.74 2.88

Excess with b = g =

3.74 − 2.55 = 1.19

Excess with b =0.8,g =0.72.88 − 2.55 = 0.33

16.7. Mean Family Size from Order-of-Birth Distribution 439

16.7 Mean Family Size from Order-of-Birth

Distribution

Birth certiﬁcates almost invariably require that mothers report whether a

particular birth is their ﬁrst, second, third, and so on. The resultant order-

of-birth tabulations are available for many countries and regions, and one

would like to extract their implication for the mean number of children

born to mothers. The problem was considered by Burks (1933) and others.

The early writers assumed a stationary condition, in which the distribu-

tion of births by order in a calendar year or other period of observation is

the same as in the cohorts of mothers that are passing through the period

in question; the several cohorts are taken as of equal size. Suppose that

the observed number of ﬁrst births is N

, of second births is N

,andso

on, and that these numbers apply in all periods and for all cohorts. This is

to say that N

is the total of women who have any children at all, N

the

number who have two or more children, N

the number who have three or

more children, for all periods and all cohorts. If we call φ

the number who

stop with one child, φ

the number who stop with two children, and so on,

the N’s are the cumulative sums of the φ’s:

= φ

+ φ

+ ···

= φ

+ φ

+ ···

= φ

+ ···

and the φ’s are the diﬀerences of the N’s:

= N

− N

= N

− N

= N

− N

Themeannumberofchildreninafamilythathaschildrenis

+2φ

+3φ

+ ···

+ φ

+ ···

+ N

+ ···

Total births

First births

(16.7.1)

This highly simpliﬁed formula applied to United States data for 1933

requires only the fact that births in that year were 74 per thousand native

white women 15 to 44 years old, and ﬁrst births were 24, making a mean

family size of 3.1 children. For 1956 the corresponding ﬁgures were 115, 33,

and a mean family size of 3.5 children; for 1973 the mean was 2.40.

440 16. Microdemography

16.8 Parity Progression and Population Increase

How parents decide the size of their families has been the subject of much

investigation since eﬀective birth control has made that size subject to

deliberate decision. Some may have a concept at the beginning that they

retain throughout—they are ﬁxed on three children, say. The majority,

however, seem to make up their minds as they go along—they have one

child and then, depending on how things look, they decide whether to have

another. The following argument suggested by Norman Ryder formalizes

parental decision in terms of the probabilities of successively proceeding to

each further child.

Disregard time and consider the married couples that have children of

successive orders. Suppose that a certain fraction of women have a ﬁrst

child; of these a certain fraction have a second child; of those that have two

children a certain fraction go on to a third; and so on. We might take as

the cohort a group of couples that married at the same time, or a group of

women born at the same time. We could include a symbol for the fraction

of the cohort that survives, and another for the fraction that marries,

but simplicity is served by just supposing that r

of the girls born at a

given moment will grow up and have a ﬁrst child, of these the fraction r

will go on to a second child, and so on.

Then the probability that a girl child will have at least one child is r

that she will have two children is r

, and so on. Suppose that the ratio of

boys to girls among the births of any order is s, so the fraction 1/(1 + s)of

the births are girls. The net reproduction rate of the cohort of which the

girl in question is a member is

1+s

+ r

+ ···). (16.8.1)

Due allowance is implicitly made for mortality, nonmarriage, illegitimacy,

and voluntary or involuntary sterility within marriage.

We can enable the notion of parity progression to serve us better by

reducing the parameters in (16.8.1) from one for each child to just two in

all. Ryder found that r

≈ r

= h,say,andthatr

≈ r

≈···= k,

say. Then (16.8.1) becomes

1+s

(h + h

+ h

k + h

+ h

+ ···)

1+s



h +

1 − k



Table 16.6 shows values of R

for various combinations of h and k;the

combinations on the lower right give an increasing population.

With this result we can do experiments of various kinds. We can, in

particular, see how R

would be modiﬁed by lowering k,whichistheway

in which fertility change seems largely to have taken place in the United

16.9. Lower Mortality and Increase 441

Table 16.6. Net reproduction rate corresponding to mothers’ parity progression

ratio, h for ﬁrst and second child, and k for subsequent children, according to

(16.8.2)

0.40 0.45 0.50 0.55 0.60 0.65 0.70

0.60 0.59 0.61 0.64 0.68 0.73 0.79 0.88

0.65 0.66 0.69 0.73 0.78 0.83 0.91 1.00

0.70 0.74 0.78 0.82 0.87 0.94 1.02 1.14

0.75 0.82 0.86 0.91 0.98 1.05 1.15 1.28

0.80 0.91 0.96 1.01 1.08 1.17 1.28 1.43

0.85 1.00 1.06 1.12 1.20 1.30 1.42 1.59

States. Now, since

1+s



h +

1 − k



, (16.8.2)

we have

1+s



1 − k



Around s =1.05,h =0.80,k =0.65, we have dR

/dk =2.55, so that each

increase of 0.01 in k produces an increase of 0.0255 in R

,andsimilarlyfor

decreases.

To see the eﬀects of successive childbearing decisions on population

growth we need also to take account of timing. The age at which a woman

has her ﬁrst child, and the successive interbirth intervals, will evidently

make a diﬀerence in the rate at which the population grows. Timing is the

one element lacking in the present model. For the eﬀect of its omission,

consider h =0.80 and k =0.65, so that R

=2.63/2.05 = 1.28. If the

mean age of childbearing (strictly, the length of generation) is 25 years, the

intrinsic rate is 0.0099; if it is 30 years, the intrinsic rate is 0.0082.

16.9 For a Given Probability of Survivors, Lower

Mortality Lowers the Rate of Increase

When mortality is high, a man who wants to have a son who will see him

through his old age requires many children. This point has often been made

before, but we still need clariﬁcation of the relation between mortality and

the rate of population increase among people who want a certain assurance

of surviving children. With number of births given, the rate of increase r

goes up as mortality µ

goes down; we will see that the relation between

r and µ

is reversed if the birth rate is determined by the wish to have

surviving sons.

442 16. Microdemography

Table 16.7. Rate of increase r of populations with values of l

from 0.5 to 0.9

and probability p of at least one living son of 0.8 and 0.9; tabulation of (16.9.4)

p =0.8 p =0.9

0.5 0.0050 0.0169

0.6 0.0017 0.0137

0.7 −0.0022 0.0097

0.8 −0.0074 0.0045

0.9 −0.0155 −0.0035

Call the probability of at least one surviving son p; this must equal one

minus the probability that all sons will die. Consider survivorship to father’s

age 60; then the probability that not all sons will die is

1 − (1 − l

60−a

)(1 − l

60−a

) ···(1 − l

60−a

)=p (16.9.1)

if the ﬁrst son is born at age a

of the father, the second at age a

,...,and

the nth at age a

Now the rate of increase of the population for this particular family, still

on the one-sex model but for males, is the real root in r of the equation



i=1

−ra

=1. (16.9.2)

This discrete form of Lotka’s characteristic equation can be solved for r to

tell us what r would be in a population if the mortality and childbearing

patterns of a particular family were general.

Our problem is to ﬁnd r as a function of the life table l

from (16.9.2),

given that the number of children n will be determined by (16.9.1). Even

to deﬁne the solution of this would be awkward, so we approximate by

supposing all the children to be born at the same age of the father: a

= ···= 30, say. Then the ﬁrst equation, (16.9.1), is

1 − (1 − l

)

= p,

n =

log(1 − p)

log(1 − l

)

. (16.9.3)

The second equation, (16.9.2), is

−30r

=1;

and substituting n from (16.9.3) and taking logarithms gives

r =

log



log(1 − p)

log(1 − l

)



. (16.9.4)

Thevaluesofr are shown in Table 16.7, whose most important message

is that each rise in l

of 0.1 causes a decline in r of 0.003 to 0.008. The

16.9. Lower Mortality and Increase 443

point seems to be that the improvement in mortality permits a decline in

the number of children suﬃciently great that the births drop more than

enough to oﬀset the fall in mortality. Heer (1966) has applied simulation to

this problem, and his more complex model produces an inverted U -curve

in r.

Note that n, being the number of children, ought to be an integer, and if

p is to be assured it ought to be the next higher integer to the expression

on the right-hand side of (16.9.3). For some combinations of high p and low

the n implied in (16.9.3) will be an impossibly large number of children.

This does not apply, however, to the range shown in Table 16.7, for which

1to4malechildrensuﬃce.

Note that as l

moves toward unity a discontinuity exists, for r goes

steadily downward with 1 − l

for any ﬁxed value of p; but we know that

= 1 and r = 0 will provide with certainty the one child living at age 60

of the father. Evidently (16.9.4) is not to be taken seriously as mortality

falls very low, or when other than small positive values of n  1 are implied.

The Multi-State Model

The life table was in use for some centuries before anyone noticed that

the transition from life to death is an instance of much more general

transitions—from well to sick, from school to work, from single to mar-

ried, from working to unemployed, from living in Province A to living in

Province B, from fecund to pregnant. For each of these, and many other

transitions, a decrement table can be made, and the usefulness of doing so

has been recognized in ﬁeld after ﬁeld. To study schooling, one routinely

makes life tables of survival in school as against dropping out; to study the

eﬀectiveness of a contraceptive, one makes a life table of those remaining

fecund versus those falling into pregnancy.

The technique could well be applied more extensively. For unemploy-

ment, we ought to know not only the total number at a given moment and

the fraction they are of the labor force, but also the probability that an

unemployed individual will ﬁnd a job next month, the month after, or the

month after that. With ten percent unemployment in the country, it makes

a great deal of diﬀerence whether everyone in the labor force is out of a job

for 5 weeks a year, or one tenth of the labor force is out for 50 weeks. The

life table form would tell a person of certain characteristics who has just

lost his job the chance of being out of work for 1 month, 2 months, etc.

All these would be single decrement life tables, calculable by exactly the

same technique as has been used for the representation of mortality these

last 300 years. There is no need to adapt the life table method to them; the

method is immediately applicable. This chapter goes beyond them into the

realm of multiple contingencies. The person now out of work has a certain