Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


If
all
reactions
are
irreversible
(c'=
d'=
0),
a
condition
which
is
approximately
true
for
most
biochemical
and
biological
systems,
the
concentration
of
A
at
the
stationary
solution
P
1
and
the
critical
value
ccrit
coincide
and
they
are
independent
of
r.
The
irreversible
autocatalytic
processes
consume
the
reactant
A
to
the
ultimate
limit,
i.e.,
P
1
would
become
unstable
if
the
concentration
of
A
went
below
ccrit.
(iii)
Multispecies
-
competition
between
autocatalytic
reactions
Now
consider
a
network
of
2n+1
reactions:
n
first-order
autocatalytic
reactions
followed
by
n
degradation
processes
and
coupled
to
a common
irreversible
recycling
reaction,
which
is
again
controlled
from
the
outside,
which
allows
driving
the
system
far
from
equilibrium:
A+
X;
c.•
..
c;
2Xp
i =
1,
2,
•••
, n
I
d'
X;
d·'
..
•
B,
B . r<E>-+
A.
(7.5-9a)
(7.5-9b)
(7.
5-9
c)
One may
consider
this
reaction
scheme
as
competition
between
n
autocatalysts
for
the
common
source
of
material
A
for
synthesis.
For
the
reversible
reactions
(7.5-9a,b),
there
is
a
thermodynamic
restriction
of
the
choice
of
rate
constants
due
to
the
uniqueness
of
the
thermodynamic
equilibrium:
bfa
=
c;d;/C;
'd;
•
for
all
i =
1,
•••.
n,
and
a
and
b
are
equilibrium
concentrations.
The
dynamics
of
mechanism
(7.5-9)
again
can
be
described
by:
dajdt
=
rb
+
I:;
c;
'X;
2
-
I:;
ac;X;,
dbjdt
=
:Ei
d;X; -
(r
+
:Ei
di
1
)b,
dx;fdt
=
X;
(c;a
-
C;
'x;
-
d;)
+ d;
'b.
Of
course,
we
still
have
the
constant
of
motion,
a + b +
I:;
X;
= c
0
=
constant.
(7.5-10a)
(7.
S-lOb)
(7.5-10c)
The
fixed
points
are
readily
computed
for
the
case
of
irreversible
degradation,
i.e.,
d;'
= o.
There
are
2"
fixed
points
of
Eqs.(7.5-10).
A
convenient
notation
which
correspond
to
the
nonvanishing
components
is:
P;
is
the
fixed
point
with
X;
+ 0
and
xk
= 0
for
k +
i;
Pii
is
the
364
fixed
point
With
X;
=f
0,
Xj
=f
0,
and
Xk
0
fork
=f
i,
j;
etc.
See
Fig.7.5.2.
Fig.7.5.2
Without
a
loss
of
generality,
we
can
arrange
the
indices
in
such
a way
that
d
1
jc
1
< d
2
jc
2
< d:yfc
3
<
.•.
<d,,cn.
It
is
straightforward
to
show
from
Eqs.(7.5-10)
that
the
presence
of
species
X;
at
a
stable
stationary
point
implies
also
the
presence
of
species
Xi
with
j <
i.
Consequently,
all
fixed
points
different
from
P
0
,
P
1
,
•••
, Pn, P
12
, • •
·,
Pn-l
n'
P
123
,
•••
, P
12
___
n
are
unstable
irrespective
of
the
'values
of
the
external
parameters
r
and
c
0
•
Indeed,
only
one
of
the
fixed
points
listed
above
is
stable
depending
on
rand
c
0
:
if
c;'
>
o,
then
a
sequence
of
double
point
bifurcations
of
the
type
P
12
___
;
-+
P
12
___
i,i+l
with
increasing
value
of
c
0
•
At
the
point
of
coincidence,
a
change
in
stability
takes
place
and
a new
species
is
introduced
into
the
system
at
the
stable
stationary
state.
The
larger
the
environment,
which
can
sustain
a
larger
total
concentration
c
0
,
the
more
species
can
coexist.
A
similar
phenomenon
has
been
found
for
the
competition
of
self-replicating
macro-molecules
in
Lotka-Volterra
food
chain
(So
1979;
Gard
and
Hallman
1979],
or
based
on
Michaelis-Menten
type
kinetics
[Epstein
1979].
When
the
autocatalytic
reactions
are
also
irreversible,
i.e.,
c;'
= o, a
condition
usually
met
by
ecological
systems,
and
if
the
values
of
the
quotients
d;/C;
are
distinct,
then
only
P
0
and
the
one
species
fixed
points,
P
1
,
365

P
2
,
•••
,
Pn
exist
at
finite
values
of
c
0
•
P
1
,
the
steady
state
corresponding
to
the
lowest
value
of
d;/C;,
is
stable
for
c
0
> d
1
jc
1
•
In
this
case
we
observe
the
selection
of
the
fittest
species,
which
is
characterized
by
the
smallest
quotient
of
degradation
rate
over
the
replication
rate.
Theorem
7.5.1
For
an
irreversible
degradating
dynamical
system
(7.5-10)
(d;'=
0,
for
all
i E
[1,n]),
if
there
is
no
stationary
point
in
the
interior
of
the
state
space,
i.e.,
P
12
•••
n
does
not
exist,
then
at
least
one
species
dies
out.
Proof:
Suppose
the
system
of
linear
equations
X;·l
dX;/dt
=
C;a
-
C;
1
X;
-
d;
=
0,
i E
[1,n)
dbjdt
=
:E;
d;X; -
rb
= 0
1
has
no
solution
(a,
b,
x
1
,
•••
, xn)
with
positive
entries,
then
a
well-known
convexity
theorem
ensures
the
existence
of
real
numbers
q;
(i
e
[O,n]),
such
that
~(:E;
d;X; -
rb)
+
:E;
q;
(c;a
-
c;
'x;
-
d;)
>
o,
for
all
a,
b,
X;>
O, i E
[1,n].
A
Liapunov
function
V =
~b
+
:E;
q;
log
X;,
for
X;
>
0,
satisfies
dV/dt
=
~dbjdt
+
:E;
x;·l
q;
dx;/dt
> o.
Thus,
all
orbits
converge
to
points
where
at
least
one
of
the
concentrations
X;
vanishes.
May
and
Leonard
[1975)
have
shown
that
for
three
competitors,
the
classic
Lotka-Volterra
equations
possess
a
special
class
of
periodic
limit
cycle
solutions,
and
a
general
class
of
solutions
in
which
the
system
exhbits
nonperiodic
population
oscillations
of
bounded
amplitude
but
ever
increasing
cycle
time.
As
another
example
of
an
application
of
a
theorem
by
Sil'nikov
[1965],
a
criterion
is
obtained
which
allows
one
to
construct
one-parameter
families
of
generalized
Volterra
equations
displaying
chaos
[Arneodo,
Coullet
and
Tresser
1980].
Another
example
of
a
Lotka-Volterra
time
dependent
system
is
the
parametric
decay
instability
cascading
with
one
wave
heavily
damped
[Picard
and
Johnston
1982).
This
can
display
Hamiltonian
chaos
even
without
ensemble
averaging.
It
seems
likely
that
many
such
systems
can
be
nearly
ergodic
without
the
necessity
of
ensemble
averaging.
366

Recently,
Gardini
et
al
[1987]
have
studied
the
Hopf
bifurcation
of
the
three
population
equilibrium
point
and
the
related
dynamics
in
a
bounded
invariant
domain.
And
the
transitions
to
chaotic
attractors
via
sequences
of
Hopf
bifurcations
and
period
doublings
are
also
discussed.
Recently,
Beretta
and
Solimano
[1988]
have
introduced
a
generalization
of
Volterra
models
with
continuous
time
delay
by
adding
a
nonnegative
linear
vector
function
of
the
species
and
they
have
found
sufficient
conditions
for
boundedness
of
solutions
and
global
asymptotic
stability
of
an
equilibrium.
They
have
considered
a
predator-prey
Volterra
model
with
prey-refuges
and
a
continuous
time
delay
and
also
a
Volterra
system
with
currents
of
immigration
for
some
species.
Furthermore,
a
simple
model
in
which
two
predators
are
competing
for
one
prey
which
can
take
shelter
is
also
presented.
Goel
et
al
[1971]
studied
many
species
systems
and
considered
the
population
growth
of
a
species
by
assuming
that
the
effect
of
other
species
is
to
introduce
a
random
function
of
time
in
the
growth
equation.
They
found
that
the
resulting
Fokker-Planck
equation
has
the
same
form
as
the
Schrodinger
and
Block
equations.
They
have
also
shown
that
for
a
large
number
of
species,
statistical
mechanical
treatment
of
the
population
growth
is
desirable.
They
have
developed
such
treatment.
(iv)
Permanence:
In
1975,
Gilpin
[1975]
showed
that
competitive
systems
with
three
or
more
species
may
have
stable
limit
cycles
as
attractor
manifolds,
and
he
argued
that
it
would
be
reasonable
to
expect
to
find
this
in
nature.
The
question
of
whether
all
species
in
a
multispecies
community
governed
by
differential
equations
can
persist
for
all
time
is
one
of
the
fundamental
ones
in
theoretical
ecology.
Nonetheless,
various
criteria
for
this
property
vary
widely,
where
asymptotic
stabilty
and
global
asympototic
stability
are
two
of
the
criteria
most
widely
367

used.
But
neither
of
these
criteria
appears
to
reflect
intuitive
concepts
of
persistence
in
a
satisfactory
manner.
The
asymptotic
stability
is
only
a
local
condition,
while
the
global
asympototic
stability
rules
out
cyclic
behavior.
Hutson
and
Vickers
[1983]
argued
that
a
more
realistic
criterion
is
that
of
permanent
coexistence,
which
essentially
requires
that
there
should
be
a
region
separated
from
the
boundary
(corresponding
to
a
zero
value
of
the
population
of
at
least
one
species)
which
all
orbits
enter
and
remain
within.
Putting
it
differently,
an
interesting
population
dynamical
system
is
called
permanent
if
all
species
survive,
provided,
of
course,
they
are
present
initially.
More
precisely,
a
system
is
permanent
if
there
exists
some
level
k > 0
such
that
if
X;(O) > 0
for
all
i =
l,
.•
,n,
then
X;(t)
> k
for
all
t > T >
o.
This
property
was
called
cooperativity
[e.g.,
Schuster,
Sigmund,
and
R.
Wolff
1979].
Schuster
et
al
[1980]
has
also
discussed
self
replication
and
cooperation
in
autocatalytic
systems.
It
was
used
in
the
context
of
molecular
evolution
where
polynucleotides
effectively
helped
each
other
through
catalytic
interactions
as
we
have
just
discussed.
The
notation
applies
equally
well
in
ecology,
but
semantically
it
is
awkward
to
speak
of
cooperative
predator-prey
communities.
Moreover,
Hirsch
[1982]
has
defined
a
dynamical
system
dxjdt
=
f(x)
as
cooperative
if
af;/axi
> o
for
all
j +
i,
which
seems
to
be
more
an
appropriate
usage
of
this
term.
Another
equivalent
way
of
defining
a
permanent
system
is
to
postulate
the
existence
of
compact
set
c
in
the
interior
of
the
state
space
such
that
all
orbits
in
the
interior
end
up
in
c.
Since
the
distance
from
c
to
the
boundary
of
the
state
space
is
strictly
positive,
this
implies
that
the
system
is
stable
against
fluctuations,
provided
that
these
fluctuations
are
sufficiently
small
and
rare;
indeed,
the
effect
of
a
small
fluctuation
upon
a
state
in
c
would
not
be
enough
to
send
it
all
the
way
to
the
boundary
and
would
be
compensated
by
the
dynamics
which
would
lead
the
orbit
of
368

the
perturbed
state
back
into
c.
The
details
of
what
happens
in
C
is
of
no
relevance
in
the
context
of
permanence.
In
a
more
mathematical
term,
one
can
also
define
a
permanent
system
as
the
one
admitting
a
compact
set
c
in
the
interior
of
the
state
space
such
that
c
is
a
globally
stable
attractor.
In
ecology
or
biology,
there
is
a
difference
between
the
following
two
terms.
Roughly
speaking,
a
community
of
interacting
populations
is
permanent
if
internal
strife
cannot
destroy
it,
while
it
is
uninvadable
if
it
is
protected
against
disturbance
from
without.
Certainly,
uninvadability
is
not
a
property
per
se,
one
has
to
specify
which
invaders
the
community
is
protected
against.
Let
x
1
,
•••
,
xm
be
the
densities
of
established
populations
and
x~
1
,
•••
, xn
be
the
densities
of
potential
invaders.
Then
the
established
community
is
uninvadable
if
(a)
it
is
permanent,
and
(b)
small
invading
populations
will
also
persist.
Mathematically,
the
(x
1
,
•••
,xm)
community
is
uninvadable
inthe
(x
1
,
•••
,xn)
state
space
(where
n >
m),
if
there
exists
a
compact
set
C
in
the
interior
of
the
(x
1
,
•••
,xm)
subspace,
which
is
a
stable
attractor
in
the
(x
1
,
•••
,xn)
space,
and
even
a
globally
stable
attractor
in
the
(x
1
,
•••
,xm)
subspace.
There
are
several
results
on
permanence.
Theorem
7.5.2
A
necessary
and
sufficient
condition
for
an
interacting
dynamical
system
to
be
permanent
is
the
existence
of
a
Liapunov
function
[Gregorius
1979].
The
above
theorem
was
obtained
by
introducing
the
concept
of
repulsivity
of
certain
sets
with
respect
to
dynamical
systems
defined
on
metric
spaces.
An
important
necessary
condition
for
permanence
is
the
existence
of
an
equilibrium
in
the
interior
of
the
state
space.
This
is
just
a
consequence
of
the
Brouwer's
fixed
point
theorem
(Corollary
2.4.18).
Hutson
and
Moran
[1982)
have
proved
the
necessary
conditions
for
the
discrete
systems,
and
Sieveking
has
proved
for
the
continuous
systems.
369

Another
useful
condition
of
the
permanence
of
continuous
systems
of
the
type
dxijdt
=
xiFi(x)
was
given
by
Hofbauer
[1981].
If
there
exists
a
function
Lon
the
state
spaceS
which
vanishes
on
the
boundary
as
and
is
strictly
positive
in
the
interior,
and
if
the
time
derivative
of
L
along
the
orbits
satisfies
dL/dt
=
Lf,
where
f
is
a
continuous
function
with
the
property
that
for
any
X
on
as,
there
is
T
> 1
with
1/TJ:
f(x(t))dt
> o,
(7.5-11)
then
the
system
is
permanent.
Note
that,
iff
in
Eq.(7.5-11)
is
strictly
positive
on
as,
then
Eq.(7.5-11)
is
always
satisfied
and
L
is
just
a
Liapunov
function.
Then
Eq.(7.5-11)
means
that
Lis
an
"average
Liapunov
function".
Hutson
and
Moran
[1982]
showed
that
a
discrete
system
(Tx)i
=
xiFi(x)
is
permanent
if
there
exists
a
non-negative
function
P
on
s
which
vanishes
exactly
on
as
and
satisfies
supk>O
lillly..x
v•f
inf
P (Tky)
/P
(y)
> 1
for
all
X E as.
Hutson
and
Moran
[1982]
have
studied
the
discrete
equations
given
by
an
analogue
of
the
Lotka-Volterra
model,
(Tx)
1
= x
1
exp
(b
1
-
a
11
x
1
-
a
12
x
2
) ,
(Tx)
2
= x
2
exp(-b
2
+ a
21
x
1
-
a
22
x
2
),
with
all
bi
and
aii
>
o.
They
have
shown
that
the
system
is
permanent
iff
it
admits
a
fixed
point
in
the
interior
of
s.
This
is
similar
to
the
continuous
Lotka-Volterra
case.
Nonetheless,
for
the
continuous
case,
permanence
implies
global
stability,
but
the
discrete
case
allows
for
more
complicated
asymptotic
behavior.
Sigmund
and
Schuster
[1984]
offers
an
exposition
of
some
general
results
on
permanence
and
uninvadability
for
deterministic
population
models.
In
a
closely
related
discussion,
Gard
and
Hallam
[1979]
have
discussed
the
persistence-extinction
phenomena
and
have
shown
that
the
existence
of
persistence
or
extinction
functions
for
Lotka-Volterra
systems,
which
are
Liapunov
functions,
then
the
systems
are
persistent
or
extinct
respectively.
370

Hofbauer
and
Schuster
(1984],
Hofbauer
et
al
(1979,1981]
have
shown
that
in
a
flow
reactor,
hypercyclic
coupling
of
self-
reproducing
species
leads
to
cooperation,
i.e.,
none
of
the
concentrations
will
vanish.
Yet
autocatalytic
self-reproducing
macromolecules
usually
compete,
and
the
number
of
surving
species
increases
with
the
total
concentration.
Before
we
end
this
subsection
and
move
on
to
higher
order
autocatalytic
systems,
let
us
briefly
mention
the
exclusion
principle,
which
states
roughly
that
n
species
can
not
coexist
on
m
resources
if
m <
n.
This
can
easily
be
proved
for
the
case
that
the
growth
rates
are
linearly
dependent
on
the
resources.
But
for
more
general
types
of
dependence,
this
principle
is
not
valid.
As
an
exercise,
prove
this
statement.
For
example,
Koch
(1974]
presented
a
computer
simulation
showing
that
two
predator
species
can
coexist
on
a
single
prey
species
in
a
spatially
homogeneous
and
temporally
invariant
environment.
This
result
was
then
interpreted
in
the
context
of
Levin's
extended
exclusion
principle
(Levin
1970].
Armstrong
and
McGehee
(1976]
provided
a
different
interpretation
of
the
relation
of
Koch's
work
to
Levin's.
(v)
Second-order
autocatalysis:
In
order
to
demonstrate
the
enormous
richness
of
the
dynamics
of
higher
order
catalytic
systems,
we
now
study
the
trimolecular
reaction
coupled
to
degradation
and
recycling
in
analogy
to
Eqs.(7.5-J):
A +
2X
c••c
JX
X
d'•d
B
B r
-+
A.
The
corresponding
differential
equations
are:
dajdt
rb
+
c•x3
-
cax•,
db/dt
=
dx
-
(d'
+
r)b,
dx/dt
=
cax•
+
d'b
-
c•x3
-
dx,
and
together
with
the
conservation
equation:
a + b + x = c
0
=
constant.
371
(7.
5-12a)
(7.5-12b)
(7.5-12c)
(7.
5-lJa)
(7.
5-lJb)
(7.
5-13c)
For
simplicity,
we
only
consider
the
irreversible
degradation
case,
i.e.,
d'
= o,
nonetheless,
the
results
obtained
are
also
valid
for
d'
> o.
Note
that
the
fixed
point
P
0
(a
= c
0
,
E = x =
0)
is
always
stable.
The
two
other
fixed
points
P
and
Q
are
positioned
at:
(p,q)
=
(c
0
c ±
[c
0
2
c•
-
4d(c
+
c'
+
cdjr)
)y,}/2
(c
+
c'+
cdjr).
Note
that
p >
q,
as
p
grows
and
q
shrinks
with
increasing
c
0
•
It
is
easy
to
show
that
Q
is
always
a
saddle
point,
and
P
either
a
sink
or
a
source.
So,
for
c
0
< csad =
[4d(c
+
c'
+
cdjr)
]Yzjc, P
0
is
the
only
fixed
point,
and
as
c
0
crosses
csad'
by
a
saddle-node
bifurcation,
P
and
Q
emerge
into
the
interior
of
s.
To
determine
the
stability
of
P,
we
evaluate
the
trace
of
the
Jacobian,
A,
at
P:
trA(x=p)
=
d-
r-
p•
(c
+
c'),
(7.5-14)
which
is
a
decreasing
function
of
C
0
•
If
at
C
0
= csad'
Eq.(7.5-
14)
is
negative,
then
Pis
stable
for
all
C
0
> csad·
This
corresponds
to
a
direct
transition
from
region
I
to
region
V
and
it
always
happens
if
d S
r.
1
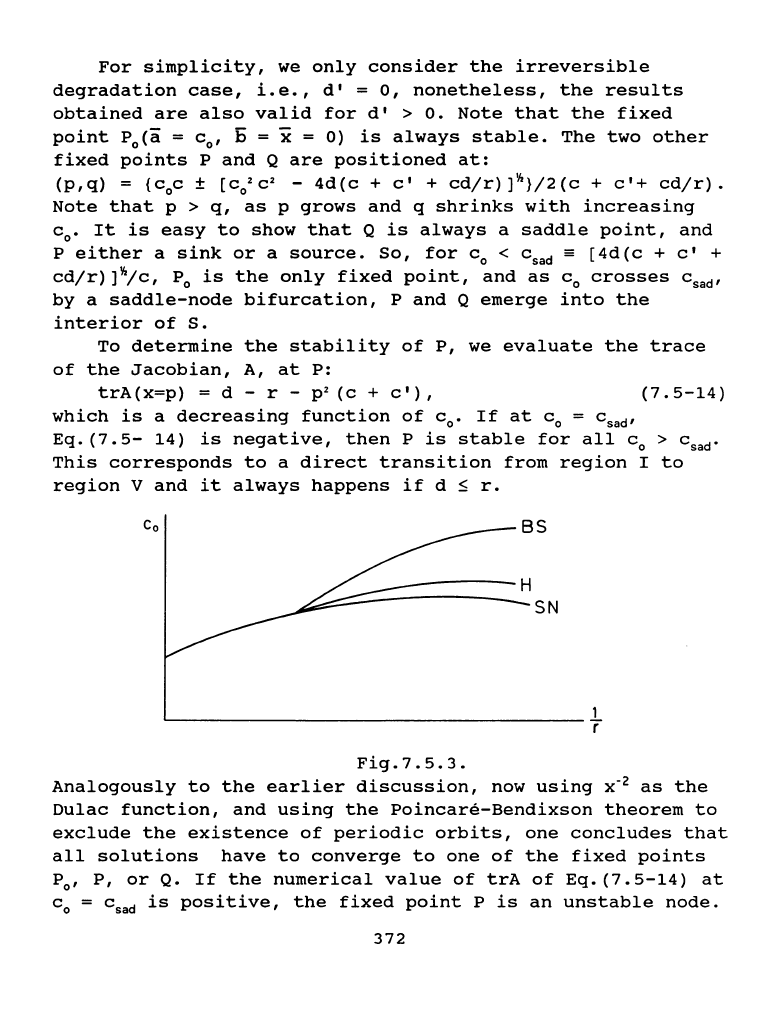
~--------------------------------------,
Fig.7.5.3.
Analogously
to
the
earlier
discussion,
now
using
x-
2
as
the
Dulac
function,
and
using
the
Poincare-Bendixson
theorem
to
exclude
the
existence
of
periodic
orbits,
one
concludes
that
all
solutions
have
to
converge
to
one
of
the
fixed
points
P
0
,
P,
or
Q.
If
the
numerical
value
of
trA
of
Eq.(7.5-14)
at
c
0
= csad
is
positive,
the
fixed
point
P
is
an
unstable
node.
372

With
increasing
c
0
,
the
eigenvalues
of
A
become
complex.
But
since
trace
A
is
decreasing,
the
eigenvalues
have
to
cross
the
imaginary
axis
at
c
0
=
cH
where
a
Hopf
bifurcation
takes
place.
The
value
of
cH
can
be
obtained
from
Eq.(7.5-14)
by
setting
trace
A(x
=
p)
=
0.
Marsden
and
McCracken
(1976]
showed
that
this
Hopf
bifurcation
is
always
subcritical.
This
means
that
the
bifurcating
periodic
orbit
is
always
unstable
and
occurs
for
c
0
> cH,
at
such
value
of
c
0
,
P
is
stable.
A
further
increase
of
c
0
leads
to
growth
of
the
periodic
orbit.
At
c
0
= c
8
s,
the
periodic
orbit
includes
the
saddle
point
Q.
Thus,
the
periodic
orbit
changes
to
a
homoclinic
orbit
and
disappears
for
c
0
> Cas·
This
phenomenon
is
called
"blue
sky
bifurcation"
[Abraham
and
Marsden
1978].
When c
0
> c
8
s,
orbits
starting
from
the
boundary
of
S
have
a
chance
to
converge
to
P.
At
values
close
to
c
8
s,
but
larger
than
c
8
s,
the
admissible
range
of
initial
concentration
x(O)
for
final
survival
of
the
autocatalyst
is
very
small.
Indeed,
if
x(O)
is
too
small
or
too
large
,
the
orbit
will
converge
to
P
0
and
the
autocatalyst
will
die
out.
For
much
larger
values
of
c
0
,
the
basin
of
attraction
of
P
becomes
almost
the
entire
s
because
the
saddle
Q
tends
toward
P
0
for
c
0
-+
oo.
Recently,
Kay
and
Scott
(1988)
have
studied
the
behavior
of
a
third
order
autocatalytic
reaction
diffusion
system.
They
have
found
that
for
indefinitely
stable
catalysts,
the
model
exhibits
ignition,
extinction
and
hysteresis,
and
the
range
of
conditions
over
which
multiple
stationary
states
are
found
to
decrease
as
the
concentration
of
the
autocatalyst
in
the
reservoir
increases.
They
have
also
found
that
the
final
ignition
and
extinction
points
merge
in
a
cusp
catastrophe
with
the
consequent
loss
of
multiplicity.
On
the
other
hand,
with
a
finite
catalyst
lifetime,
the
dependence
of
the
stationary
state
composition
on
the
diffusion
rate
or
the
size
of
the
reaction
zone
shows
more
complex
patterns.
The
stationary
state
profile
for
the
distribution
of
the
autocatalytic
species
now
allows
multiple
internal
extrema,
that
is
the
onset
of
dissipative
373
