Martienssen W., Warlimont H. (Eds.). Handbook of Condensed Matter and Materials Data

Подождите немного. Документ загружается.

702 Part 4 Functional Materials

Table 4.2-5 Critical temperature T

of superconductivity of V compounds [2.1], cont.

Compound Crystal T

structure (K) structure (K)

VNi

Rhomb. <4.2 V

Cub. A15

<4.2

VNi

Tetr. DO

<4.2 V

Tetr. <4.2

Tetr. <4.2 VZn

Cub. L1

<4.2

Hex. <4.2 VZn

− −

Cub. B1 <0.07 V

Cub. C15 8.8–6.5

Rhomb. <1.28 Rhomb.

8.5

Compound with a range of homogeneity

Metastable phase

“<” means that no superconductivity was found above the temperature indicated

Supposed compound

Superconducting properties not investigated

Unstable in the absence of carbon

Non-stoichiometric composition

Low-temperature modiﬁcation

High-temperature modiﬁcation

Formed by martensitic transformation

51015

(K)

C (at.%)C (at.%)

51015

Os, Ru

Al Au

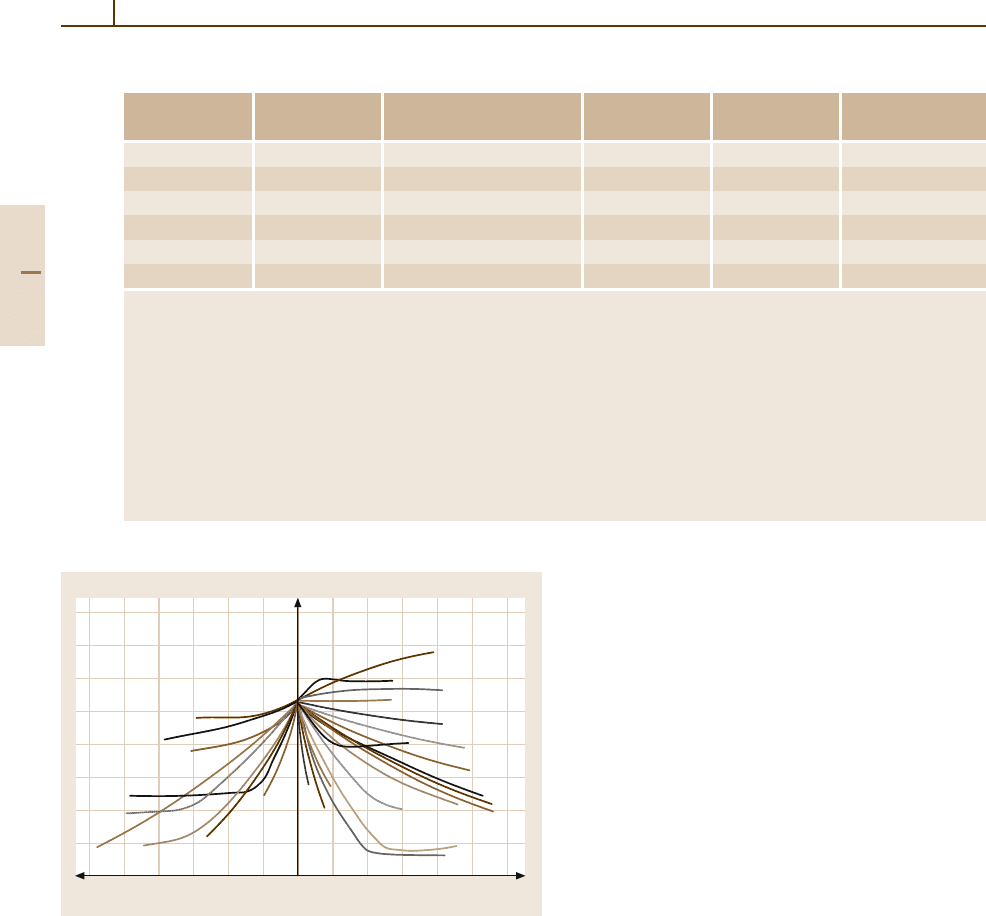
Fig. 4.2-3 Composition dependence of T

of superconductivity in

V solid solution alloys [2.1]

Nb Alloys

Based on its highest T

among all elements Nb has been

considered the most likely base metal for superconduct-

ing materials from the beginning and its alloys and

intermetallic compounds have been investigated most

extensively. Table 4.2-6 shows T

values for binary Nb

compounds, where Nb

Al, Nb

Ga, Nb

Ge, and Nb

with the cubic A15 type crystal structure stand out for

their particularly high T

.Ofthese,Nb

Sn has been de-

veloped into a high-ﬁeld superconducting material (see

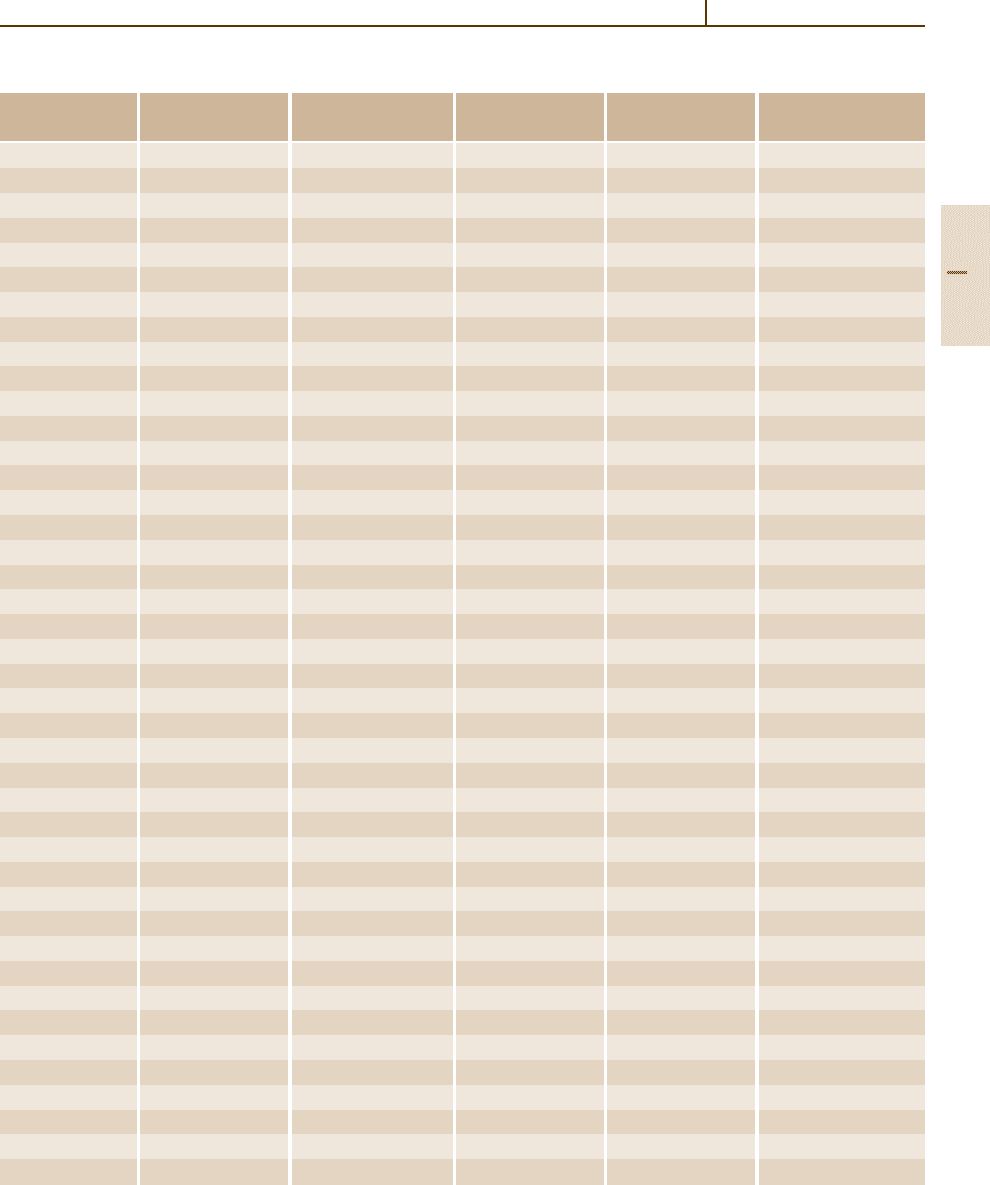
Sect. 4.2.1.2). Figure 4.2-4 shows the effect of alloying

additions on T

in Nb solid solutions. The Nb

−

Ti alloys

have, ﬁnally, been selected to form the most versatile

and widely applied high-ﬁeld superconducting material

for present applications (see Sect. 4.2.1.2).

Part 4 2.1

Superconductors 2.1 Metallic Superconductors 703

Table 4.2-6 Superconductivity of Nb compounds [2.1]

Compound Crystal T

structure (K) structure (K)

Al Cub. A15 18.8–15.0 Nb

Os Cub. A15 1.5–0.5

NbOAl Tetr. D8

0.74 Nb

Tetr. D8

1.86–1.40

NbAl

Tetr. D0

0.64 NbOs

Cub. A12 2.86

–2.52

As Tetr. Ti

P 0.31 Nb

P Tetr. Ti

P 2–1.83

(7.5–6.0)

NbAs

Monocl. <0.012

Cub. A15 9.6<1.5

Au Cub. A15 11.5–8.99 Nb

Cub. A12 2.47–1.7

Tetr. <1.0 NbPd Tetr. D8

2.0

NbB

Rhomb. B

8.25 Nb

Pt Cub. A15 10.9–6.0

NbB

− <0.05 Nb

Pt Tetr. D8

4.2–3.73

Rhomb. D7

<1.28 NbPt(α) Rhomb. B19 2.4–<1.39

NbB

Hex. C32 6.4

–<1.0 NbPt

Rhomb. <1.46

Tetr. 2.3 Nb

Tetr. D8

3.8–2.5

NbBe

Cub. A15 2.15 NbRe

Cub. A12 2.45–9.7

NbBe

Rhomb. <1.15 Nb

Rh Cub. A15 2.64–2.4

Rhomb. 1.47–<1.38 Nb

Tetr. D8

4.1–4.04

10.2

NbBe

Tetr. <1.38 NbRh(γ) Tetr. L1

3.76

Cub. A15 3.05 (4.2–2) NbRh(γ) Rhomb. 3.07

C Hex. 9.11– < 1.98 NbRh(ε) Rhomb. B19 3.00

NbC Cub. B1 11.7– < 1.15 (14

) Nb

(ξ) Monocl. Sm 2.7

Ga Cub. A15 16.8–14.1 (20.7)

NbRh

Cub. L1

<1.43

Tetr. 1.35 Nb

Tetr. 1.2

Tetr. <2 Nb

1.2

Hex. <2 NbS Hex. B8

<1.28 (3.3–3.8)

NbGa

− <2 NbS

Hex. C7 6.15–5.4

− <2 Nb

Sb Cub. A15 1.95–0.2

Rhomb. <2 Nb

<1.02

NbGa

Rhomb. <2 NbSb Hex. B8

<4.2

Ge Cub. A15 6.91 (23.2)

Tetr. 4.2–<1.15

Ge Tetr. Ti

P <0.2 NbSb

Monocl. 4.2–<1.15

Ge Hex. D8

1.90 Nb

Hex. 1.61

Tetr. <1.02 NbSe

Hex. C7 7.5–5.4(8.5–9)

NbGe

Hex. C40 2.23–2.09 NbSe

Monocl. <1.0

NbH Cub. <1.30 Nb

Hex. ε −Fe

N <1.5

NbH

Cub. <0.47 Nb

Si Tetr. 0.29(5.45)

In Cub. A15 9.2–4 Nb

Cub. A15 19–13

Ir Cub. A15 1.76–1.63 Nb

(α) Tetr.

<1.02

Tetr. D8

9.8

–2.4

Hex. D8

<1.02

NbIr

1+x

Tetr. L1

4.75 NbSi

Hex. C40 <1.20

Ir(α

) Rhomb. 4.6 Nb

Sn Cub. A15 18.5–4.0

NbIr

Cub. L1

<1.2 Nb

Rhomb. 2.07

Part 4 2.1

704 Part 4 Functional Materials

Table 4.2-6 Superconductivity of Nb compounds [2.1], cont.

Compound Crystal T

structure (K) structure (K)

N(β) Hex. 9.5–<1.2 NbSn

Rhomb. 2.68

(γ ) Tetr. 12.2–7.8 NbTc

Cub. A12 12.9–10.5

NbN(δ



) Tetr. 7.2 Nb

Cub. A15 <2.5

NbN(δ) Cub. B1 16.5–9.7 Nb

− <1.1

NbN(ε) Hex. <1.20 Nb

Hex. 1.49

Hex. <1.77 NbTe

Hex. C7 0.74–0.5

Tetr. 8.0–8.5 NbTe

− <0.025

NbO Cub. B1 1.61–1.38 Nb

Cub. A15 9.0

NbO

Tetr. <1.2 NbZn

Cub. L1

<1.02

“<” means that no superconductivity was found above the temperature indicated

Low-temperature modiﬁcation

High-temperature modiﬁcation

Non-stoichiometric composition

Metastable phase

Extrapolated maximum T

Under extreme conditions

Stabilized by adding interstitial elements

Phase is stable at >850

◦

C only

Fe,Ni

MaMa

Fig. 4.2-4 Composition dependence of T

of superconduc-

tivity in Nb solid solution alloys [2.1]

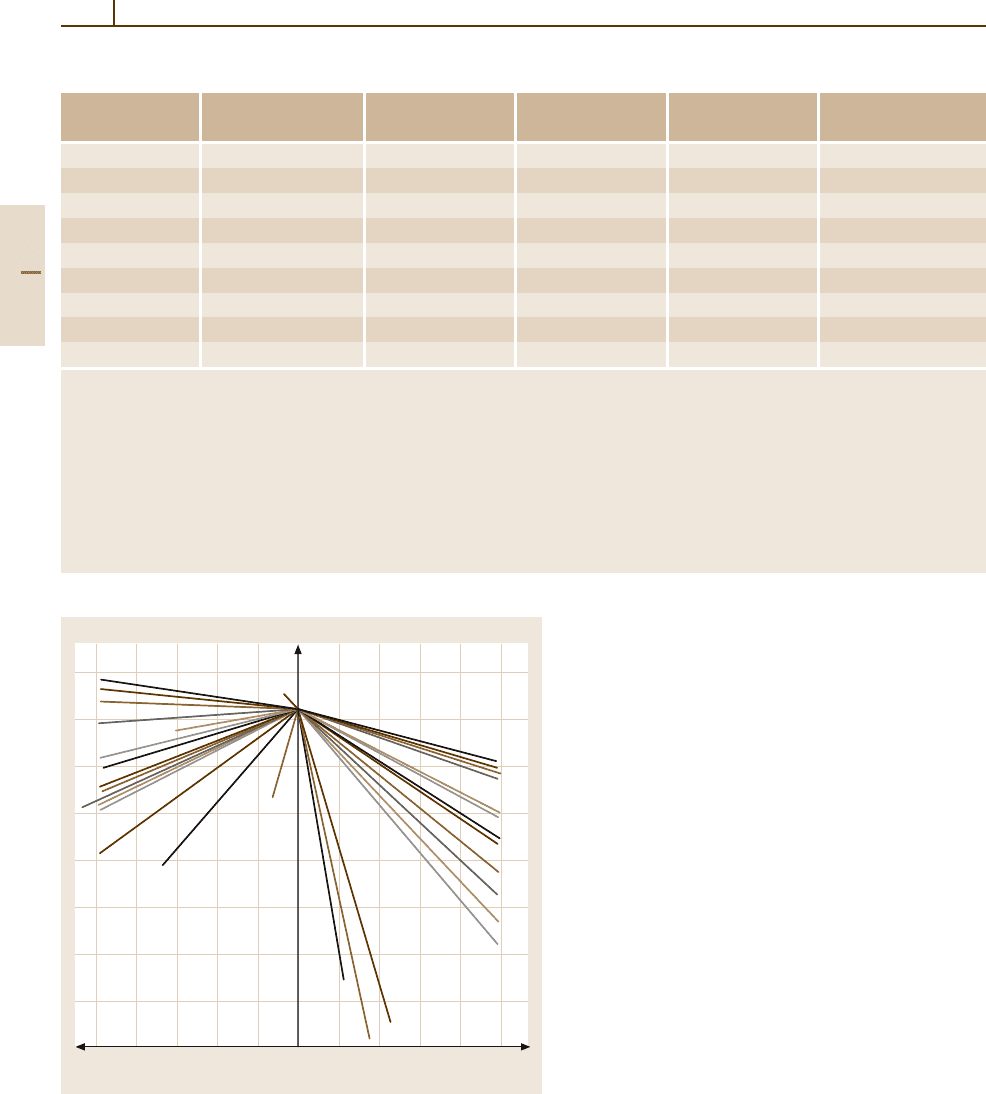
Tc Alloys

Since Tc is rare and difﬁcult to prepare in the alloy

form, its superconducting properties have been studied

to a limited extent only (see Table 4.2-7 and Fig. 4.2-5).

4.2.1.2 Practical Metallic Superconductors

Practical metallic superconductors for DC or AC

applications are invariably composite wires with super-

conducting ﬁlaments which are embedded in a normal

conducting matrix, usually Cu. They have a high longitu-

dinal conductivity and may contain further components

such as low conductivity barriers consisting of a Cu

−

alloy, or diffusion barriers of Nb or Ta. Accounts of

combining the aspects of the physics of superconductiv-

ity, materials science and technology, and electrical and

mechanical performance criteria which have to be taken

into account and are mastered in producing supercon-

ducting wires are given in [2.4–8].

Part 4 2.1

Superconductors 2.1 Metallic Superconductors 705

Table 4.2-7 T

of superconductivity of Tc compounds [2.1]

Compound Crystal T

structure (K) structure (K)

Cub. D8

<0.3

Cub. A12 8.10–7.73

TcBe Cub. 5.21 TcTi Cub. B2 <1.7

TcBe

Cub. ZrZn

5.25 Tc

Th Hex. C14 5.3

TcC Cub. 3.85 Tc

V(δ) Cub. 7.8–4.0

Hf Hex. C14 5.6 TcV(ε) Cub. B2 <1.39

Tetr. D8

15.8–14.7 Tc

Tetr. D8

7.88–8.35

Cub. A15 14–12 Tc

Zr Cub. A12 9.7

Nb Cub. A12 12.9–10.5 Tc

Zr Hex. C14 7.6

Hex. 5.92

“<” means that no superconductivity was found above the temperature indicated

Formed as an ordered phase by extended annealing of the solid solution phase

Non-stoichiometric composition

Compound not determinded deﬁnitely; T

may be related to the solid solution

Only two superconducting metallic phases are used

routinely in superconducting wires for applications: the

−

Ti solid solution phase and the Nb

Sn intermetallic

phase. Due to the difference of their intrinsic supercon-

ducting properties T

and B

, they have different ranges

of application as indicated in Table 4.2-8.

The critical current density J

(T, B) obtained by ap-

plying optimal microstructural pinning of the ﬂux lines

in conductors of different composition and at different

temperatures is shown in Fig. 4.2-6. Two factors of inﬂu-

ence may be applied to obtain a higher critical current

density J

(T, B) through effects of the intrinsic prop-

erties: either a decrease in temperature of application,

e.g., from 4.2 to 1.8 K, or an increase of B

by alloying

as shown for (Nb, Ta, Ti)

Sn in both Table 4.2-8 and

Fig. 4.2-6. It should be noted that the intrinsic properties

are affected only marginally by differences in processing

of the conductors.

Table 4.2-8 Characteristic properties of practical metallic superconductors (After [2.8])

Superconducting phase Composition T

(K) B

(T) Magnetic ﬁeld in application B(T)

−

Ti 46–52 wt% Ti, ≈ 10 ≈ 10.5(4.2K) ≤9(4.2K)

≈ 47 wt% Ti optimal > 9(1.8K)

Sn 25 at.% Sn, ≈ 18 ≈ 23 (4.2K): ≤ 20 T (4.2K)

(Nb, Ta, Ti)

Sn ≤ 7.5wt%Ta, ≈ 26–29 (4.2K) several T (< 4.2K)

≤ 0.2wt%Ti

(K)

C (at.%)C (at.%)

01234512345

Ru,Os,Rh

Fig. 4.2-5 Composition dependence of T

of superconductivity in

Tc solid solution alloys [2.1]

Part 4 2.1

706 Part 4 Functional Materials

0 4 8 12 16 20 24

B (T)

non-Cu

(A/cm

–2

)

0.1 µV/cm

NbTi

(NbTa)

(NbTaTi)

4.2K

2KNbTi

(NbTa)

(NbTaTi)

4.2K

Fig. 4.2-6 Non-Cu J

versus B characteristics of Nb

−

Ti, binary

Sn, alloyed (Nb, Ta)

Sn, and alloyed (Nb, Ta, Ti)

Sn multiﬁl-

amentary superconductors [2.8]

Nb–Ti Superconductors

Extensive accounts are given in [2.4, 6, 7]. The

−

Ti alloy phase diagram combined with the low-

temperature T

) and B

) relations of the

0 20406080100

Ti content (at.%)

1000

800

600

400

200

12.5

10.0

7.5

5.0

2.5

12.5

10.0

7.5

5.0

2.5

0 20406080100

Ti content (at.%)

Temperature (C°)

a) b)

(K) B

(T)

β+α

(4K)

Fig. 4.2-7a,b Phase relations and superconducting properties in the

−

Ti alloy system.

(a) Nb

−

Ti phase diagram in the solid state.

(b) T

and B

(4 K) of the metastable β phase

superconducting β phase (Fig. 4.2-7a and b), provide

the basic features of both the intrinsic superconducting

properties of the β phase and of the potential for ﬂux pin-

ning by precipitation of the α phase induced by suitable

heat treatment in the α +β two-phase range.

The subsequent characteristic data are taken from

systematic treatments and measurements of a Cu sta-

bilized Nb–46.5 wt% Ti superconductor [2.9] which is

in the range of optimum composition according to

Table 4.2-8 and Fig. 4.2-7b. The processing of the com-

posite consists essentially of a succession of deformation

treatments, usually by wire drawing to different strains

(true strain ε

= 2ln(d

/d), where d

is the initial rod

diameter and d is the ﬁlament diameter at the draw-

ing strain considered), followed by heat treatments for

20 h to 40 h, mainly at temperatures in the range 360 to

380

◦

C, but also up to 420

◦

C. The ﬁrst stage of defor-

mation of the homogeneous metastable β phase leads

to the formation of a high density of dislocations, thus

providing a high density of heterogeneous nucleation

sites for the precipitation of a ﬁne dispersion of α phase

particles during the ﬁrst heat treatment. During the sub-

sequent cycles of deformation and heat treatment the

α particles previously formed are elongated and partially

broken up. Additional particles may be newly formed

along dislocation cell walls and subgrain boundaries.

The particle spacing transverse to the drawing direc-

tion is reduced. These variations of the dispersion are

strongly affecting the pinning behavior and, thus, the J

values obtained, referring to a composite resistivity of

−14

Ω m.

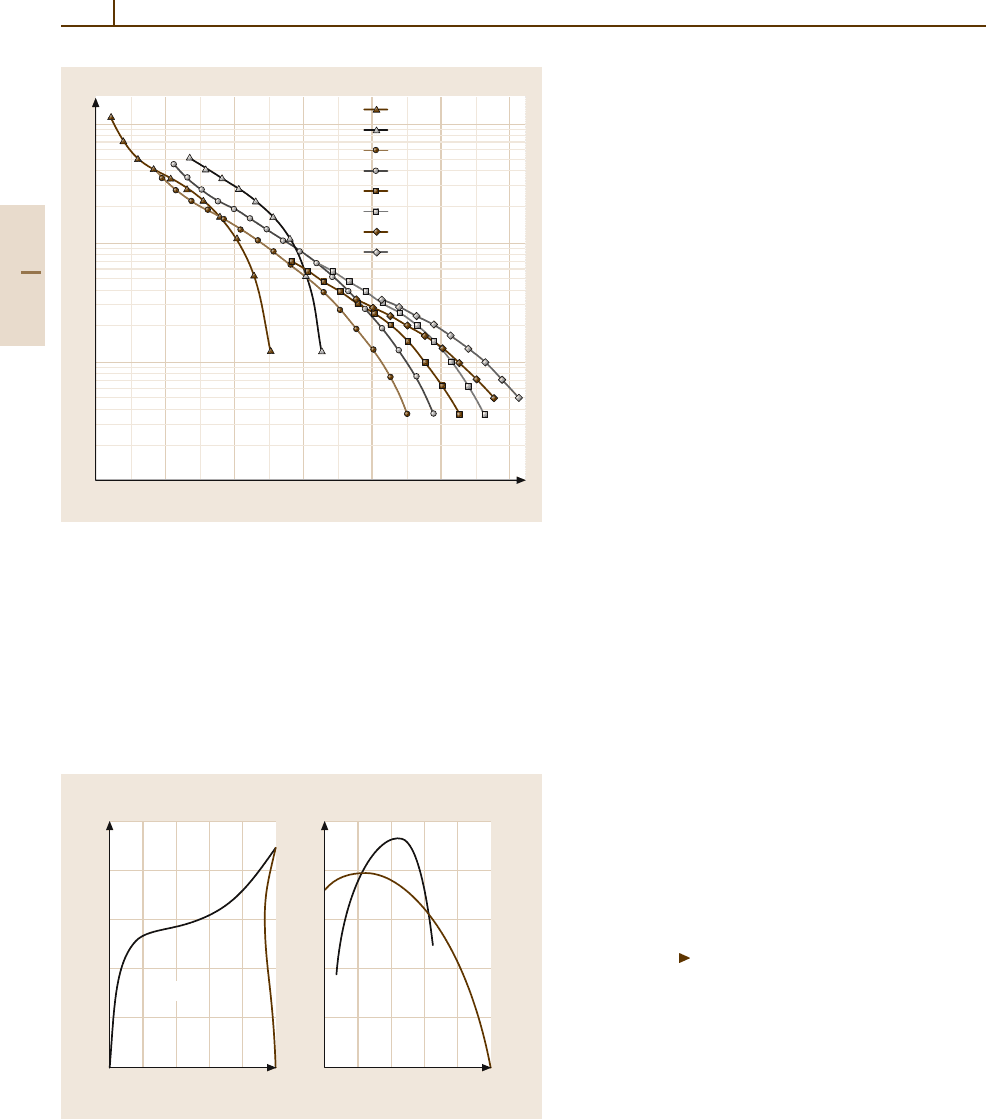
The diagrams of Fig. 4.2-8a–e show J

as a function

of the true drawing strain ε

. The parameters are different

thermal treatments, and different conditions (magnetic

ﬁeld, temperature) of measurement.

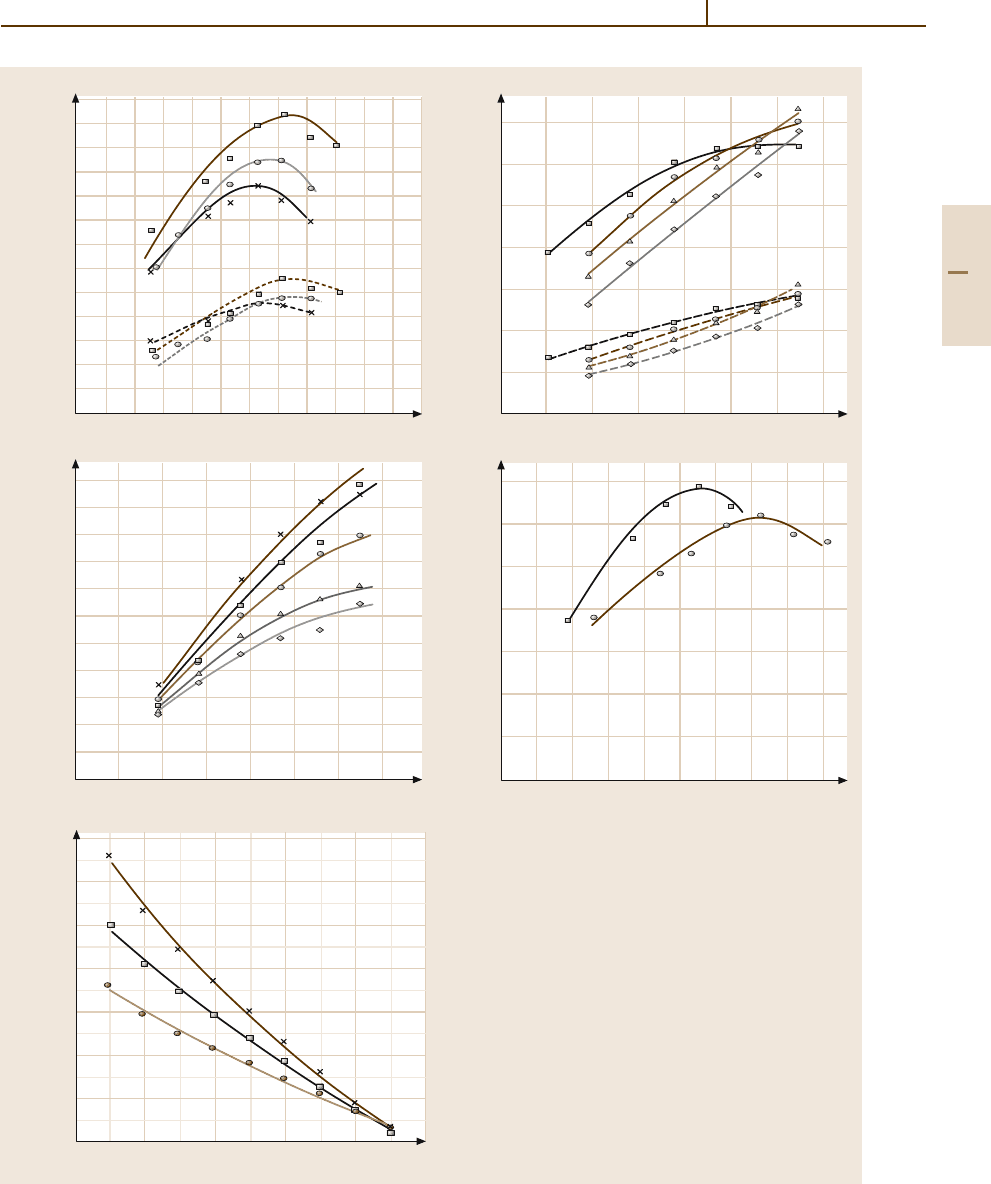
From measurements such as those shown in

Fig. 4.2-8e, the effective bulk pinning force F

can be

evaluated as shown in Fig. 4.2-9.

Fig. 4.2-8a–e Critical current variations as a function of

different thermal treatments, ﬁnal drawing strain and ﬁeld

of measurement, respectively, of an Nb–46.5 wt%Ti super-

conductor at 4.2 K [2.9].

(a)  160 h at 405

◦

C, ◦ 80 h at

405

◦

C; ×40 h at 375

◦

C. — B = 5T, --- B = 8T.(b) All

treatments for 80 h: 375

◦

C, ◦ 405

◦

C,  420

◦

C, ♦435

◦

— B = 5T, --- B = 8T.

◦

×160 h, 80 h, ◦ 40 h,  10 h, ♦5h. B = 5T.

(d) 160 h

at 420

◦

C: ◦ two heat treatments, three heat treatments.

B = 5T.

(e) Full ﬁeld optimum: ×80 h at 420

◦

C, 40 h at

375

◦

C, ◦ 5 h at 405

◦

Part 4 2.1

Superconductors 2.1 Metallic Superconductors 707

3000

2500

2000

1500

1000

500

8234567

3000

2000

1000

23 4 5

3500

3000

2500

2000

1500

1000

2345

3000

2000

1000

23456

9111357

(A/mm

–2

)

7000

6000

5000

4000

3000

2000

1000

Field (T)

(A/mm

–2

)

Strain ε

(A/mm

–2

)

(A/mm

–2

)

Strain ε

(A/mm

–2

)

Strain ε

a) b)

Strain ε

c) d)

Part 4 2.1

708 Part 4 Functional Materials

9111357

(GN/m

–3

)

B (T)

Fig. 4.2-9 Bulk pinning force F

versus magnetic ﬁeld,

corresponding to the J

data shown in Fig. 4.2-8e. Typical

particle spacings for the optimum J

is d ≈ 20 nm and d ≈

3–5 nm, respectively [2.9]

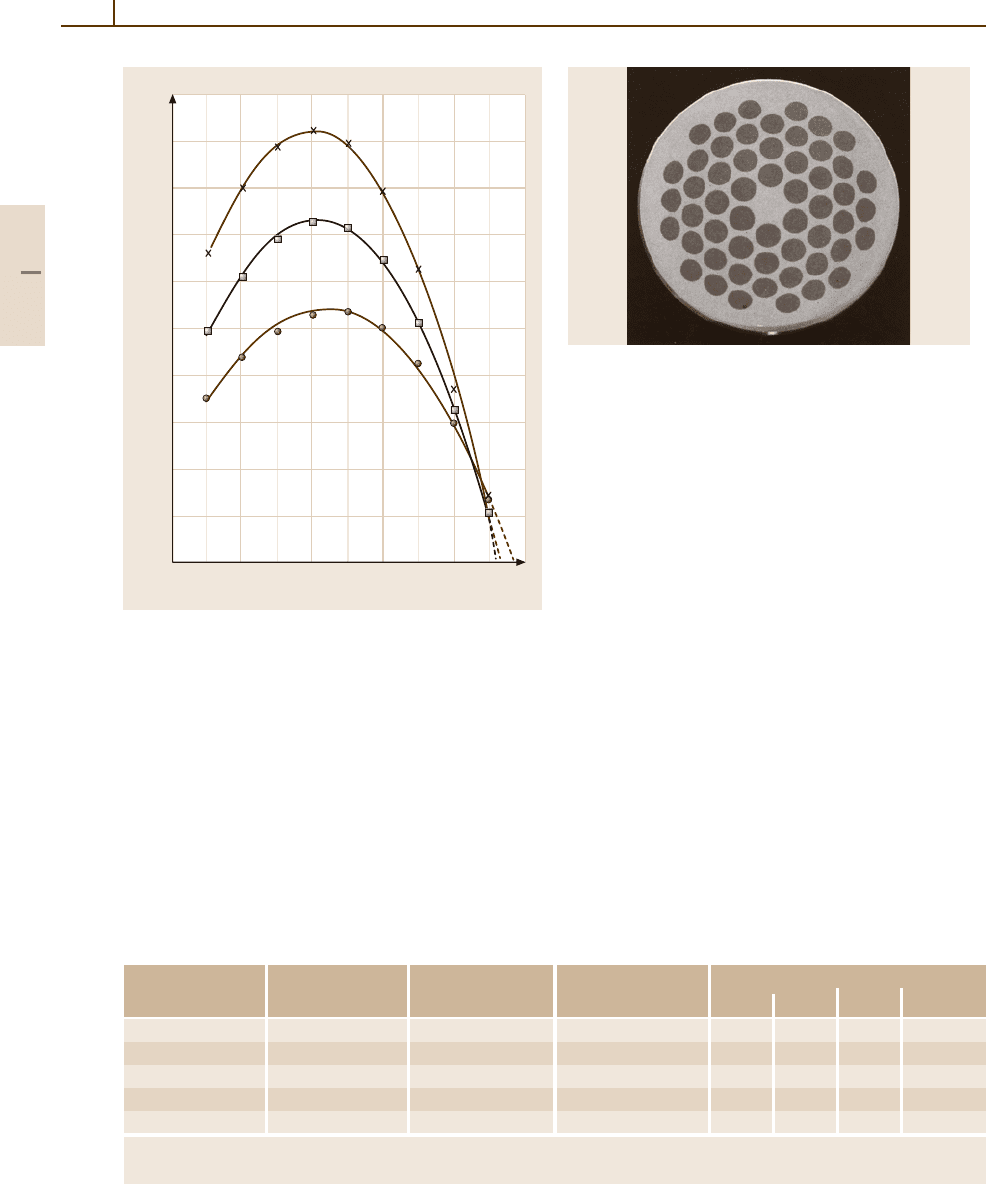
Based on systematic variations and optimizations

such as those shown in Fig. 4.2-8, commercial Nb

−

superconducting composite wires are provided for

a wide range of speciﬁcations. Figure 4.2-10 shows

a typical wire cross section and Table 4.2-9 lists some

characteristic data.

Table 4.2-9 Characteristic data at 4.2 K of commercial Nb

−

Ti superconductors, round wires

Filaments A

NbTi

Wire diameter Filament diameter Critical current J

,(A)atµ

H (T)

(mm) (µm) 3T 5T 7T 9T

54 1.35 0.3 27 100 70 45 −

54 1.35 0.6 53 380 265 170 −

54 1.35 0.85 75 − 480 310 140

45 1.8 0.4 36 150 105 70 −

45 1.8 0.7 62 420 295 185 −

Ratio of cross sectional area

Data extracted from a commercial brochure of Vacuumschmelze GmbH (9/1990)

Fig. 4.2-10 Typical cross section of a Cu-stabilized Nb

−

superconducting wire

Sn Superconductors

The production of superconductors based on Nb

Sn as

the superconducting phase [2.7, 8] is hampered by the

brittle behavior of this intermetallic phase which cannot

be deformed by wire drawing. Consequently the con-

ductors are produced by composing ductile components

ﬁrst, reducing the cross section of the composite into the

ﬁnal wire form by extrusion and drawing processes, and

ﬁnally forming the Nb

Sn phase by a diffusion–reaction

treatment after the speciﬁed wire diameter has been

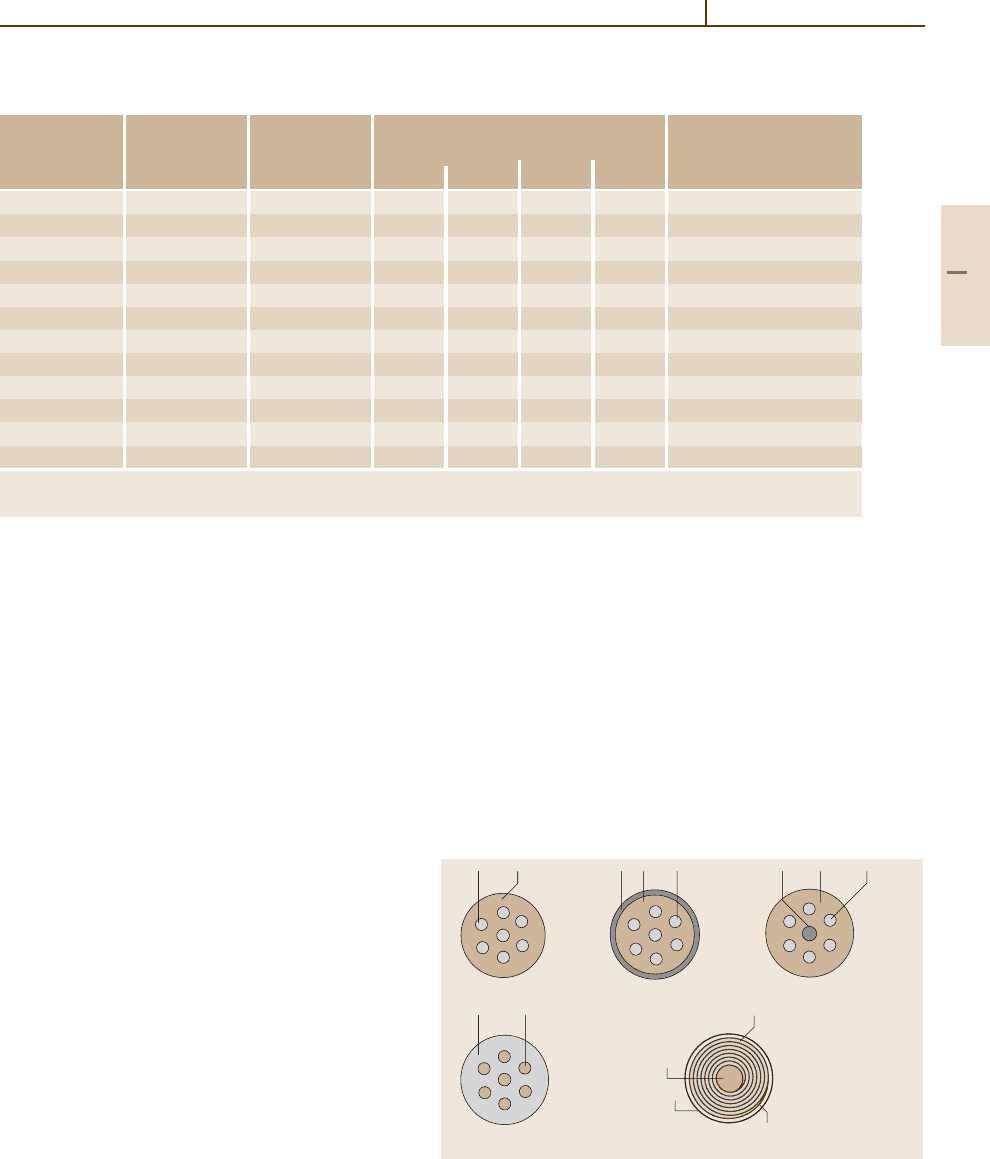
obtained. Fiveroutes of processing havebeen developed:

1. Bronze process: The initial rod composite con-

tains Nb rods surrounded by Cu

−

Sn alloy rods

(13–15 wt% Sn) in a suitable arrangement such

that after processing into wire form the diffusion–

reaction treatment leads to the formation of Nb

at the interface of Nb and Cu

−

Sn. The initial com-

posite, if composed in a suitable conﬁguration, has

excellent deformation properties such that a geo-

Part 4 2.1

Superconductors 2.1 Metallic Superconductors 709

Table 4.2-10 Characteristic data at 4.2 K of commercial Nb

Sn superconductors produced according to the bronze

process; ﬁlament diameters 4 to 6 µm

Stabilization Filament Wire diameter Critical current density I

(A) Code

material (mm) at µ

H =

8T 10T 12 T 14 T

None Nb 0.5 202 136 78 − NS 4500 (0.5)

None Nb 0.7 374 252 144 − NS 6000 (0.7)

None Nb 1.0 753 504 289 − NS 10 000 (1.0)

None Nb

−

Ta 0.5 171 123 86 58 HNST 4500 (0.5)

None Nb

−

Ta 0.7 316 228 159 107 HNST 6000 (0.7)

None Nb

−

Ta 1.0 636 456 321 215 HNST 10 000 (1.0)

Cu/Ta core Nb 0.7 305 204 117 − NS 6000 (0.7) Ta I

Cu/Ta core Nb 1.0 623 417 239 − NS 10 000 (1.0) Ta I

Cu/Ta core Nb 1.25 973 652 373 − NS 13 000 (1.25) Ta I

Cu/Ta core Nb

−

Ta 0.7 258 185 130 87 HNST 6000 (0.7) Ta I

Cu/Ta core Nb

−

Ta 1.0 526 378 266 178 HNST 10 000 (1.0) Ta I

Cu/Ta core Nb

−

Ta 1.25 822 591 416 279 HNST 13 000 (1.25) Ta I

According to the VACRYFLUX



designations of Vacuumschmelze GmbH (1990); the ﬁgures without parentheses indicate the

approximate number of ﬁlaments

metrically well controlled wire results, in which

decoupling of the ﬁlaments can be optimized and

no bridging of ﬁlaments occurs. Table 4.2-10 shows

the characteristic data of commercial Nb

Sn su-

perconductors produced according to the bronze

process.

2. External Sn diffusion process: Nb ﬁlaments in a Cu

matrix are produced from a mechanical compos-

ite of rods by processing into wire form; at its

ﬁnal diameter the wire is coated with Sn; during

a series of heat treatments with increasing tem-

perature, Sn and Cu react to form a Cu

−

Sn alloy

and this alloy reacts with the Nb ﬁlaments to form

Sn. This processis limited to wire with diameters

≤ 0.2mm.

3. Internal Sn diffusion process: Pure Sn or dilute Sn

alloys with Cu or Mg are incorporated in the ini-

tial rod composite: after processing into wire form

the Nb

Sn phase is formed by a diffusion–reaction

process with the Nb ﬁlaments. However, due to the

low melting point of Sn and its low yield stress, the

processing cannot use hot extrusion and the draw-

ing processes can readily lead to inhomogeneous

deformation and mechanical instabilities.

4. Jelly roll process: Nb foil or mesh and Cu foil are

wound into a roll as a starting rod which yields an Nb

ﬁlament bundle after processing into wire form. In

combination with the bronze or internal-Sn process

this results in Nb

Sn ﬁlaments with extremely low

diameter. It is difﬁcult to scale the process to large

quantities and wire cross-sections.

The four processes mentioned require a ﬁnal

diffusion–reaction treatment at 650 to 700

◦

C for 50 to

200 h. Since the stabilizing Cu constituent in the com-

posite must not be exposed to Sn interdiffusion in order

to maintain its high conductivity, a diffusion barrier is

incorporated in the composite which usually consists of

Ta in the form of a tube.

Nb Cu-Sn

Bronze process

Sn Cu Nb

External diffusion Internal diffusion

Solid–liquid diffusion Modified jelly roll

Sn Cu Nb

Nb Sn-Cu Nb foil

Cu-Sn foil

Sheath

Fig. 4.2-11 Processing routes of Nb

Sn superconductors [2.7]

Part 4 2.1

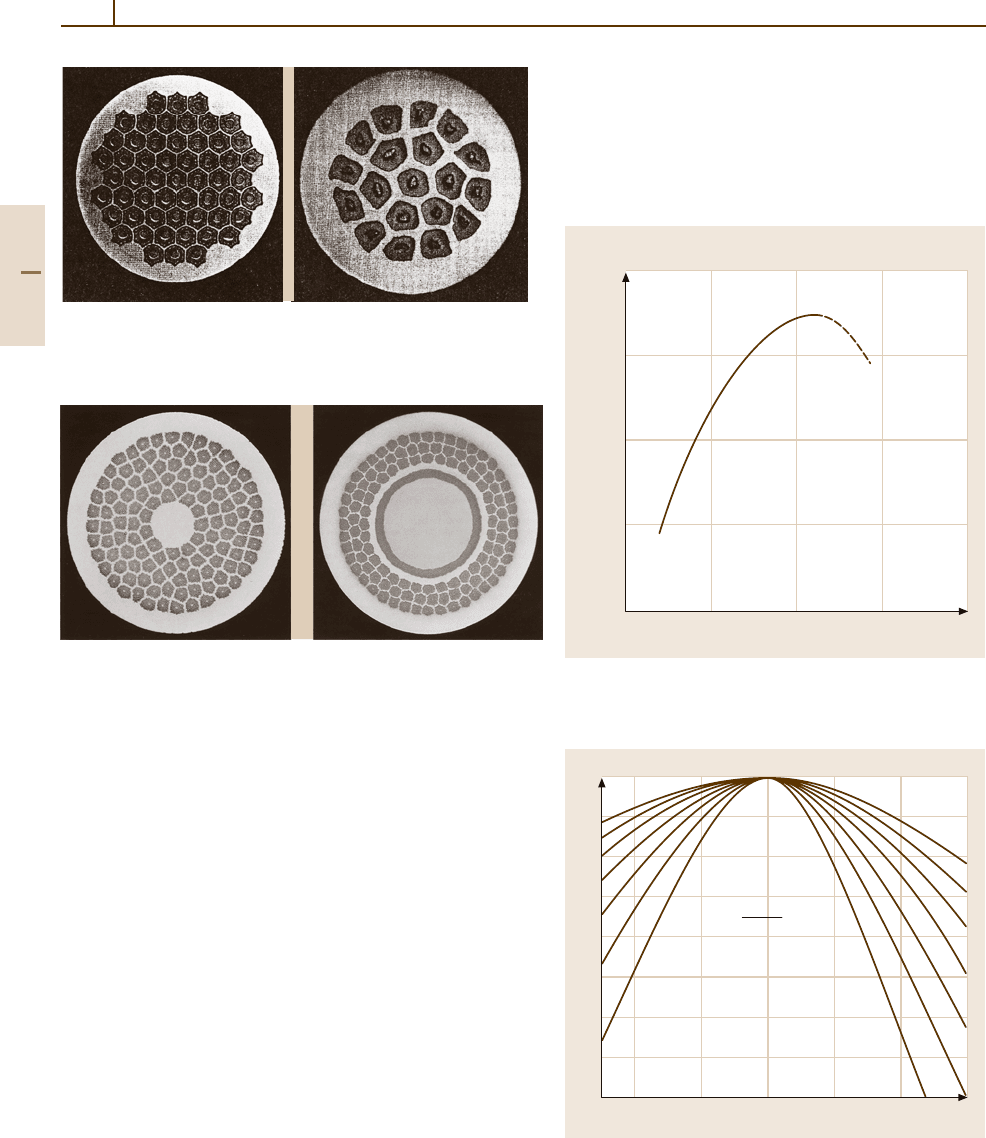
710 Part 4 Functional Materials

a) b)

Fig. 4.2-12a,b Examples of cross sections of superconductors pro-

duced by the internal-Sn (

(a) 0.8 mm diameter) and Nb-tube

(

(b) 0.5 mm diameter) processes [2.8]

a) b)

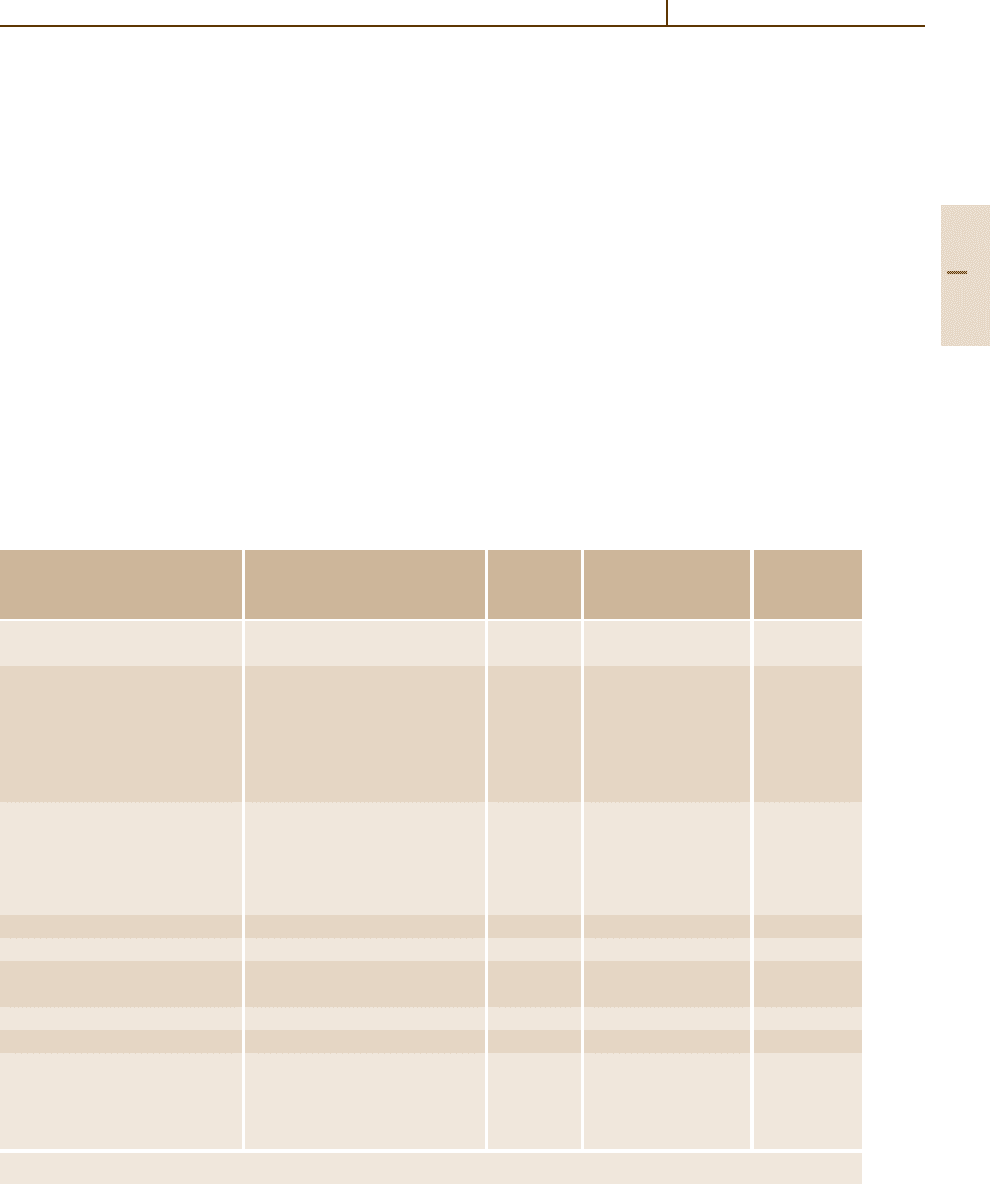
Fig. 4.2-13a,b Superconductors produced according to the bronze

process (Vacuumschmelze).

(a) Non-stabilized, 10 080 Nb ﬁlaments

inaCu

−

Sn matrix.

(b) Internally stabilized by Cu in a Ta tube, 6156

Nb ﬁlaments in a Cu

−

Sn matrix

5. Nb-tube process: The need of an extra barrier elem-

ent can be avoided by using Nb tubes, ﬁlled with Sn

or with a Sn compound such as NbSn

, embedded in

a Cu matrix. During heat treatment, a Nb

Sn layer

is formed inside the tube while its outer part acts

as a diffusion barrier. This processing route leads

to wires with high critical current densities but is

difﬁcult to scale up for industrial production.

Schematic drawings of the composites according to

these ﬁve processing routes are shown in Fig. 4.2-11.

Examples of cross sections of superconductors pro-

duced by the internal-Sn, bronze, and Nb-tube processes

are shown in Figs. 4.2-12 and 4.2-13.

In Nb

Sn superconductors the ﬂux lines are pinned

by the grain boundaries in the Nb

Sn ﬁlaments. The de-

pendence of the effective volume pinning force F

on the

grain size is shown in Fig. 4.2-14. This leads to the re-

quirement that the reaction temperature is optimized not

only regarding the rate of the diffusion–reaction process

but also regarding the resulting grain size.

Moreover, the critical current density depends

strongly on the state of strain in Nb

Sn, in particular

close to B

. Figure 4.2-15 shows the strain sensitiv-

(N/m

×10

)

Grain size (nm)

100 50 33 25∞

0 0.01 0.02 0.03 0.04

1/Grain size (nm

–1

)

Fig. 4.2-14 Dependence of the volume pinning force F

Sn on the grain size

Intrinsic strain (%)

= J

1.0

0.8

0.6

0.4

0.2

0 0.2 0.4 0.6– 0.4 – 0.2

B=4T

Temperature = 4.2 K

Fig. 4.2-15 Strain sensitivity J

(ε) of Nb

Sn superconduc-

tors [2.10]

Part 4 2.1

Superconductors 2.2 Non-Metallic Superconductors 711

ity J

(ε) for binary Nb

Sn under uniaxial longitudinal

strain. In its state of formation, i. e., in the absence of

an externally applied strain, the Nb

Sn phase as formed

has an intrinsic compressive strain of ε

= 0.2–0.3%,

depending on the conductor design and processing pa-

rameters. Thus, applying a tensile strain will ﬁrst lead

to an increase in J

. However, degradation sets in irre-

versibly if strains of a few tenths of one per cent are

exceeded due to crack formation in the brittle Nb

phase.

Table 4.2-10 lists characteristic data of commercial

Sn superconductors.

4.2.2 Non-Metallic Superconductors

4.2.2.1 Oxide Superconductors

Low-T

Superconducting Oxides

Only a few oxides with critical temperatures above

the boiling temperature of liquid helium (4.2 K) were

known up to 1986. Their T

did not exceed 14 K.

The stoichiometric composition of nearly all of these

compounds (T

> 4.2 K) is related to one of the for-

Table 4.2-11 Electrical and structural data of selected low-T

oxide superconductors

Compound Structure T

(K) µ

(T) µ

(mT)

Lattice parameters

a,b, c (nm)

BaPb

1−x

8–12

x = 0.2–0.3

Polycrystalline Orthorhombic (x =0.30) For x = 0.25: For x = 0.25:

a =0.6075, b = 0.610, 5.4 (1.8K) 23 (4.2K)

c =0.857 2.3 (8 K)

Tetragonal (x =0.275) For x =0.2:

a =0.6055, c = 0.8633 7.0 (1.15 K)

2.3 (6 K)

Single crystal Orthorhombic (x ≈0.25) For x =0.27:

a =0.6058, b = 0.6081, 3.8 (1.5K)

c =0.8552

Tetragonal (x ≈0.25) For x = 0.2:

a =0.6045, b = 0.8613 1.75 (3.5K)

0.9

0.1

0.75

−

0.25

11.5

BaSn

0.01

0.74–0.69

−

0.25–0.3

10–11

1...1.3

2–1.67

Cubic 9.6–12 3.3 (0 K)

a =0.841–0.836

2.6

1.5–2.7

Cubic 10.9–11.4 9.6–16.2(4.2K)

0.3

Hexagonal 7.5–0.6

0.1

2.9

0.1

Hexagonal ≈ 4.9 0.13–0.36

(Single crystal) a = 0.74;c =0.76 (dependent on

temperature and

crystal orientation

relative to H)

The electrical and structural data of BaPb

1−x

are values of different authors published in [2.2]

mulas:

BaPb

1−x

, Li

1+x

2−x

, or

(y < 0.5;A =Cs;Rb).

Relatively high T

valuesof the Ba

−

Ocom-

pounds were also observed after substitution of small

amounts of K and Sr for Ba, respectively, and after

substitution of Sn for Pb (Table 4.2-11).

Part 4 2.2