Stewart J. Calculus
Подождите немного. Документ загружается.


EXAMPLE 2 Evaluate , where consists of the arc of the parabola
from to followed by the vertical line segment from to .
SOLUTION The curve is shown in Figure 5. is the graph of a function of , so we can
choose as the parameter and the equations for become
Therefore
On we choose as the parameter, so the equations of are
and
Thus
M
Any physical interpretation of a line integral depends on the physical inter-
pretation of the function . Suppose that represents the linear density at a point
of a thin wire shaped like a curve . Then the mass of the part of the wire from
to in Figure 1 is approximately and so the total mass of the wire is approx-
imately . By taking more and more points on the curve, we obtain the mass
of the wire as the limiting value of these approximations:
[For example, if represents the density of a semicircular wire, then the
integral in Example 1 would represent the mass of the wire.] The center of mass of the
wire with density function is located at the point , where
Other physical interpretations of line integrals will be discussed later in this chapter.
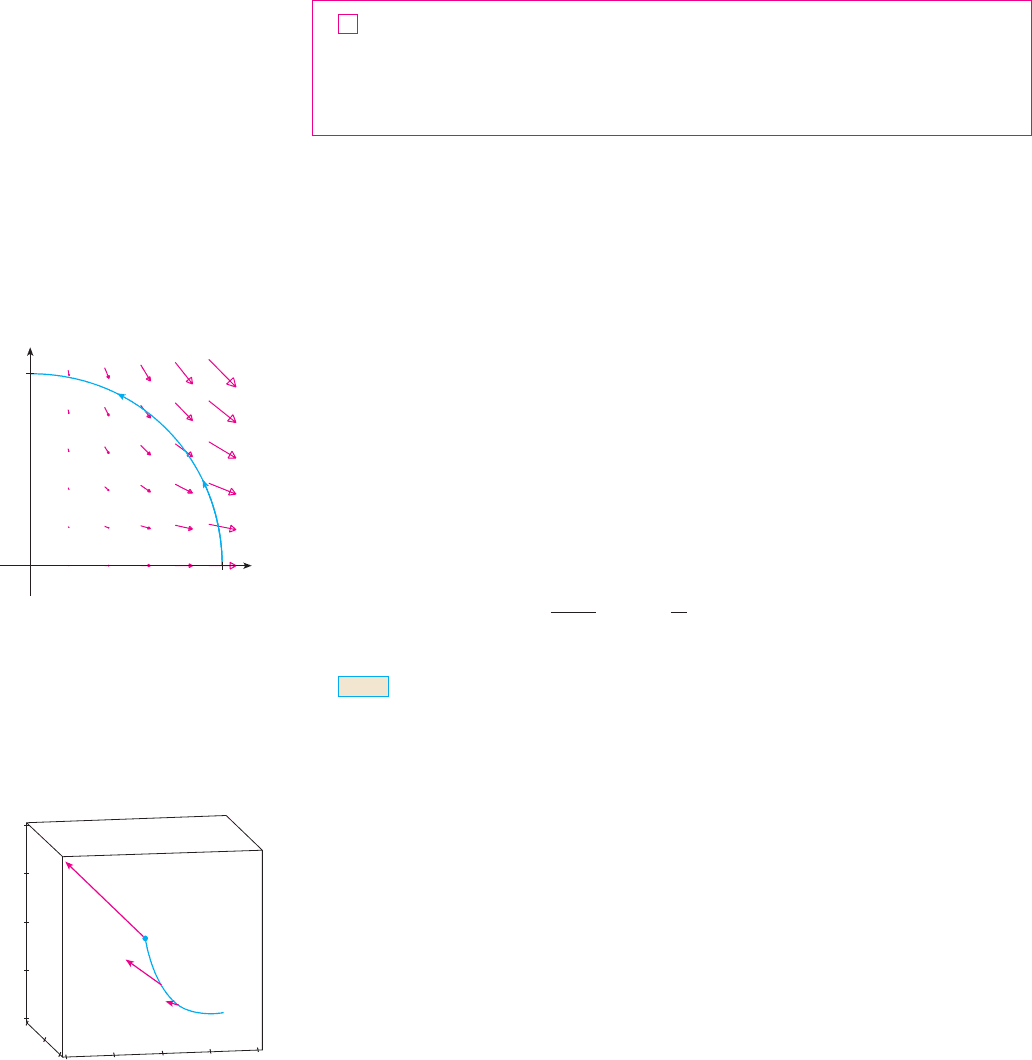
EXAMPLE 3 A wire takes the shape of the semicircle , , and is
thicker near its base than near the top. Find the center of mass of the wire if the linear
density at any point is proportional to its distance from the line .
SOLUTION As in Example 1 we use the parametrization , , ,
and find that . The linear density is
共x, y兲 苷 k共1 ⫺ y兲
ds 苷 dt
0 艋 t 艋
y 苷 sin tx 苷 cos t
y 苷 1
y 艌 0x
2
⫹ y
2
苷 1
V
y 苷
1
m
y
C
y
共x, y兲 dsx 苷
1
m
y
C
x
共x, y兲 ds
4
共x, y兲
f 共x, y兲 苷 2 ⫹ x
2
y
m 苷 lim
n
l
⬁
兺
n
i苷1
共x
i
*
, y
i
*
兲 ⌬s
i
苷
y
C
共x, y兲 ds
m
冘
共x
i
*
, y
i
*
兲 ⌬s
i
共x
i
*
, y
i
*
兲 ⌬s
i
P
i
P
i⫺1
C共x, y兲
共x, y兲f
x
C
f 共x, y兲 ds
y
C
2x ds 苷
y
C
1
2x ds ⫹
y
C
2
2x ds 苷
5
s
5
⫺ 1
6
⫹ 2
y
C
2
2x ds 苷
y
2
1
2共1兲
冑
冉
dx
dy
冊
2
⫹
冉
dy
dy
冊
2
dy 苷
y
2
1
2 dy 苷 2
1 艋 y 艋 2y 苷 yx 苷 1
C
2
yC
2
苷
1
4
ⴢ
2
3
共1 ⫹ 4x
2
兲
3兾2
]
0
1
苷
5
s
5
⫺ 1
6
苷
y
1
0
2x
s
1 ⫹ 4x
2
dx
y
C
1
2x ds 苷
y
1
0
2x
冑
冉
dx
dx
冊
2
⫹
冉
dy
dx
冊
2
dx
0 艋 x 艋 1y 苷 x
2
x 苷 x
C
1
x
xC
1
C
共1, 2兲共1, 1兲C
2
共1, 1兲共0, 0兲
y 苷 x
2
C
1
Cx
C
2x ds
1072
||||
CHAPTER 17 VECTOR CALCULUS
FIGURE 5
C=C¡ 傼 C™
(0,0)
(1,1)
(1,2)
C¡
C™
x
y
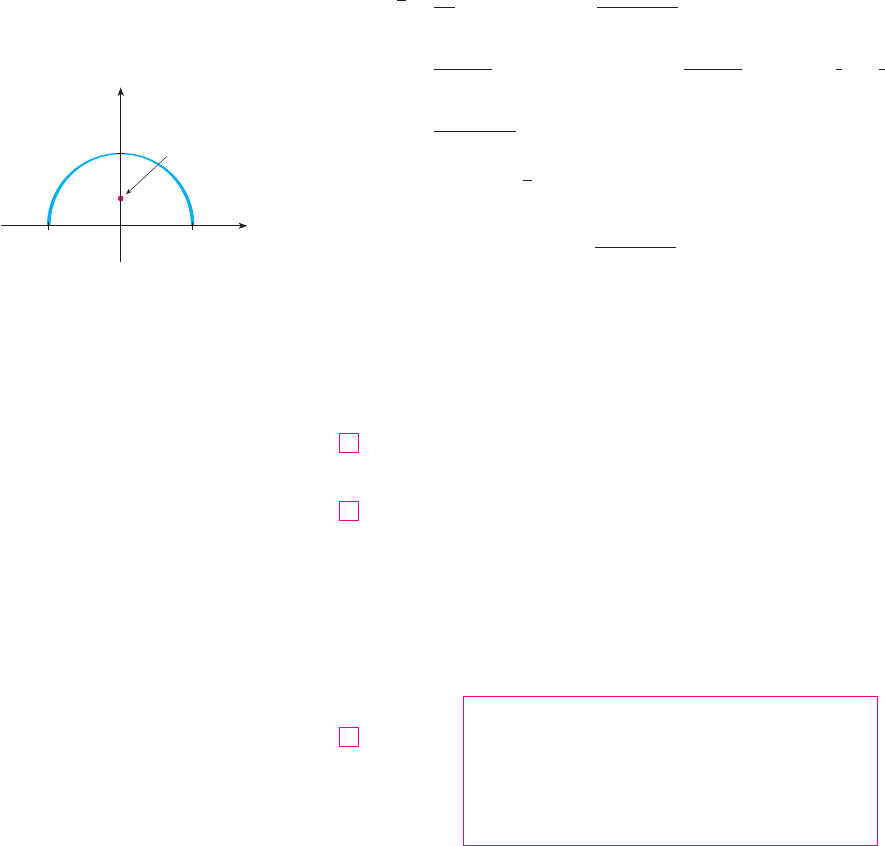
where is a constant, and so the mass of the wire is
From Equations 4 we have
By symmetry we see that , so the center of mass is
See Figure 6.
M
Two other line integrals are obtained by replacing by either or
in Definition 2. They are called the line integrals of along with
respect to x and y:
When we want to distinguish the original line integral from those in Equa-
tions 5 and 6, we call it the line integral with respect to arc length.
The following formulas say that line integrals with respect to and can also be
evaluated by expressing everything in terms of : , , ,
.
It frequently happens that line integrals with respect to and occur together. When
this happens, it’s customary to abbreviate by writing
When we are setting up a line integral, sometimes the most difficult thing is to think of
a parametric representation for a curve whose geometric description is given. In particular,
we often need to parametrize a line segment, so it’s useful to remember that a vector rep-
y
C
P共x, y兲 dx ⫹
y
C
Q共x, y兲 dy 苷
y
C
P共x, y兲 dx ⫹ Q共x, y兲 dy
yx
y
C
f 共x, y兲 dy 苷
y
b
a
f
(
x共t兲, y共t兲
)
y⬘共t兲 dt
y
C
f 共x, y兲 dx 苷
y
b
a
f
(
x共t兲, y共t兲
)
x⬘共t兲 dt
7
dy 苷 y⬘共t兲 dt
dx 苷 x⬘共t兲 dty 苷 y共t兲x 苷 x共t兲t
yx
x
C
f 共x, y兲 ds
y
C
f 共x, y兲 dy 苷 lim
n
l
⬁
兺
n
i苷1
f 共x
i
*
, y
i
*
兲 ⌬y
i
6
y
C
f 共x, y兲 dx 苷 lim
n
l
⬁
兺
n
i苷1
f 共x
i
*
, y
i
*
兲 ⌬x
i
5
Cf⌬y
i
苷 y
i
⫺ y
i⫺1
⌬x
i
苷 x
i
⫺ x
i⫺1
⌬s
i
冉
0,
4 ⫺
2共
⫺ 2兲
冊
⬇共0, 0.38兲
x 苷 0
苷
4 ⫺
2共
⫺ 2兲
苷
1
⫺ 2
y
0
共sin t ⫺ sin
2
t兲 dt 苷
1
⫺ 2
[
⫺cos t ⫺
1
2
t ⫹
1
4
sin 2t
]
0
y 苷
1
m
y
C
y
共x, y兲 ds 苷
1
k共
⫺ 2兲
y
C
yk共1 ⫺ y兲 ds
苷 k
[
t ⫹ cos t
]
0
苷 k共
⫺ 2兲 m 苷
y
C
k共1 ⫺ y兲 ds 苷
y
0
k共1 ⫺ sin t兲 dt
k
SECTION 17.2 LINE INTEGRALS
||||
1073
FIGURE 6
0
1_1
1
center of
mass
x
y

resentation of the line segment that starts at and ends at is given by
(See Equation 13.5.4.)
EXAMPLE 4 Evaluate , where (a) is the line segment from
to and (b) is the arc of the parabola from
to . (See Figure 7.)
SOLUTION
(a) A parametric representation for the line segment is
(Use Equation 8 with and .) Then , , and
Formulas 7 give
(b) Since the parabola is given as a function of , let’s take as the parameter and write
as
Then and by Formulas 7 we have
M
Notice that we got different answers in parts (a) and (b) of Example 4 even though the
two curves had the same endpoints. Thus, in general, the value of a line integral depends
not just on the endpoints of the curve but also on the path. (But see Section 17.3 for con-
ditions under which the integral is independent of the path.)
Notice also that the answers in Example 4 depend on the direction, or orientation, of the
curve. If denotes the line segment from to , you can verify, using the
parametrization
that
y
⫺C
1
y
2
dx ⫹ x dy 苷
5
6
0 艋 t 艋 1y 苷 2 ⫺ 5tx 苷 ⫺5t
共⫺5, ⫺3兲共0, 2兲⫺C
1
苷
冋
⫺
y
4
2
⫺
y
3
3
⫹ 4y
册
⫺3
2
苷 40
5
6
苷
y
2
⫺3
共⫺2y
3
⫺ y
2
⫹ 4兲 dy
y
C
2
y
2
dx ⫹ x dy 苷
y
2
⫺3
y
2
共⫺2y兲 dy ⫹ 共4 ⫺ y
2
兲
dy
dx 苷 ⫺2y dy
⫺3 艋 y 艋 2y 苷 yx 苷 4 ⫺ y
2
C
2
yy
苷 5
冋
25t
3
3
⫺
25t
2
2
⫹ 4t
册
0
1
苷 ⫺
5
6
苷 5
y
1
0
共25t
2
⫺ 25t ⫹ 4兲 dt
y
C
1
y
2
dx ⫹ x dy 苷
y
1
0
共5t ⫺ 3兲
2
共5 dt兲 ⫹ 共5t ⫺ 5兲共5 dt兲
dy 苷 5 dtdx 苷 5 dtr
1
苷 具0, 2典r
0
苷 具⫺5, ⫺3典
0 艋 t 艋 1y 苷 5t ⫺ 3x 苷 5t ⫺ 5
共0, 2兲
共⫺5, ⫺3兲x 苷 4 ⫺ y
2
C 苷 C
2
共0, 2兲共⫺5, ⫺3兲
C 苷 C
1
x
C
y
2
dx ⫹ x dy
V
0 艋 t 艋 1r共t兲 苷 共1 ⫺ t兲r
0
⫹ t r
1
8
r
1
r
0
1074
||||
CHAPTER 17 VECTOR CALCULUS
FIGURE 7
0
4
(_5,_3)
(0,2)
C¡
C™
x=4-¥
x
y
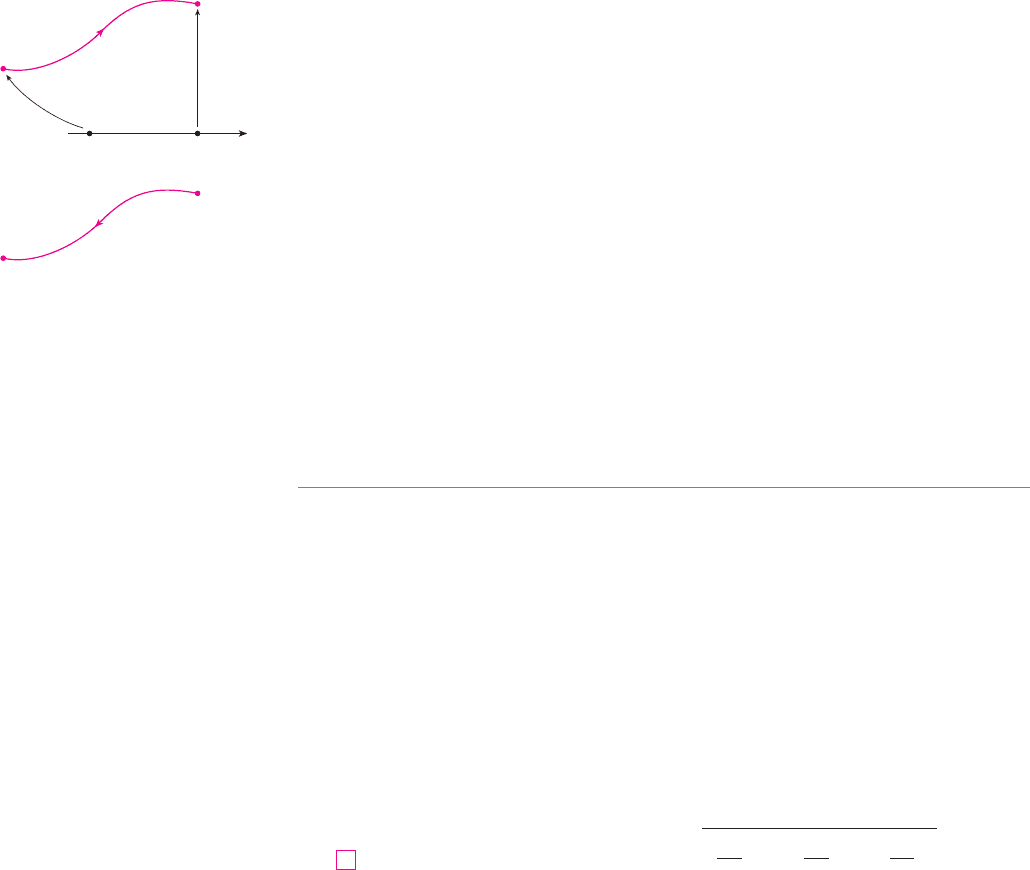
In general, a given parametrization , , , determines an orien-
tation of a curve , with the positive direction corresponding to increasing values of the
parameter (See Figure 8, where the initial point corresponds to the parameter value
and the terminal point corresponds to .)
If denotes the curve consisting of the same points as but with the opposite ori-
entation (from initial point to terminal point in Figure 8), then we have
But if we integrate with respect to arc length, the value of the line integral does not change
when we reverse the orientation of the curve:
This is because is always positive, whereas and change sign when we reverse
the orientation of .
LINE INTEGRALS IN SPACE
We now suppose that is a smooth space curve given by the parametric equations
or by a vector equation . If is a function of three variables
that is continuous on some region containing , then we define the line integral of
along (with respect to arc length) in a manner similar to that for plane curves:
We evaluate it using a formula similar to Formula 3:
Observe that the integrals in both Formulas 3 and 9 can be written in the more compact
vector notation
For the special case , we get
where is the length of the curve (see Formula 14.3.3).CL
y
C
ds 苷
y
b
a
ⱍ
r⬘共t兲
ⱍ
dt 苷 L
f 共x, y, z兲 苷 1
y
b
a
f 共r共t兲兲
ⱍ
r⬘共t兲
ⱍ
dt
y
C
f 共x, y, z兲 ds 苷
y
b
a
f
(
x共t兲, y共t兲, z共t兲
)
冑
冉
dx
dt
冊
2
⫹
冉
dy
dt
冊
2
⫹
冉
dz
dt
冊
2
dt
9
y
C
f 共x, y, z兲 ds 苷 lim
n
l
⬁
兺
n
i苷1
f 共x
i
*
, y
i
*
, z
i
*
兲 ⌬s
i
C
fC
fr共t兲 苷 x共t兲 i ⫹ y共t兲 j ⫹ z共t兲 k
a 艋 t 艋 bz 苷 z共t兲y 苷 y共t兲x 苷 x共t兲
C
C
⌬y
i
⌬x
i
⌬s
i
y
⫺C
f 共x, y兲 ds 苷
y
C
f 共x, y兲 ds
y
⫺C
f 共x, y兲 dy 苷 ⫺
y
C
f 共x, y兲 dy
y
⫺C
f 共x, y兲 dx 苷 ⫺
y
C
f 共x, y兲 dx
AB
C⫺C
t 苷 bB
aAt.
C
a 艋 t 艋 by 苷 y共t兲x 苷 x共t兲
SECTION 17.2 LINE INTEGRALS
||||
1075
FIGURE 8
B
A
t
ab
C
_C
A
B
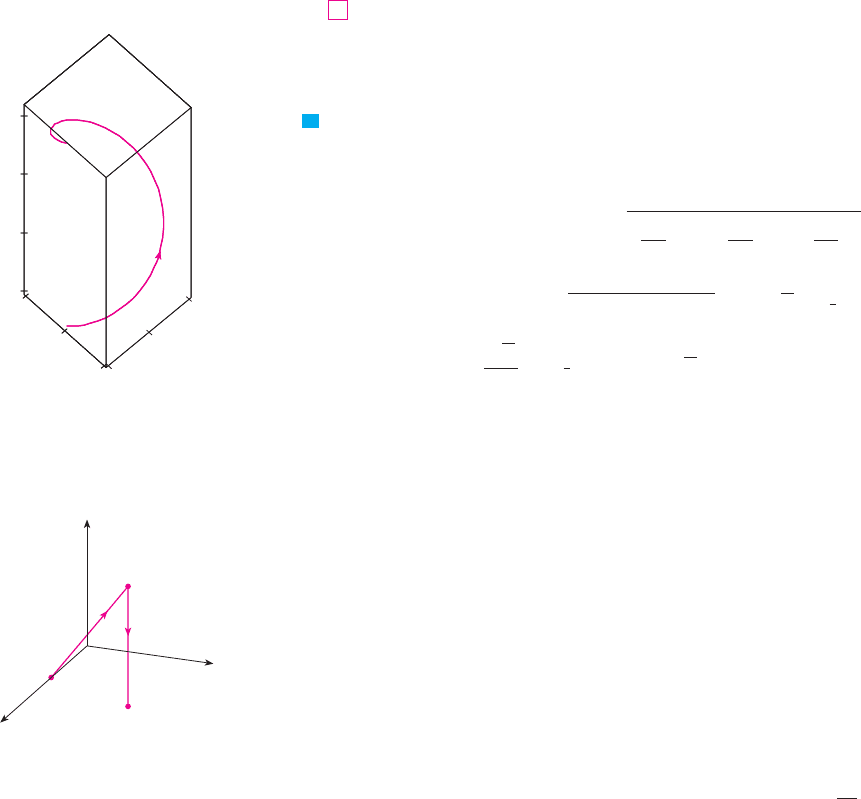
Line integrals along with respect to , , and can also be defined. For example,
Therefore, as with line integrals in the plane, we evaluate integrals of the form
by expressing everything , , , , , in terms of the parameter
EXAMPLE 5 Evaluate , where is the circular helix given by the equations
, , , . (See Figure 9.)
SOLUTION Formula 9 gives
M
EXAMPLE 6 Evaluate , where consists of the line segment
from to , followed by the vertical line segment from to
.
SOLUTION The curve is shown in Figure 10. Using Equation 8, we write as
or, in parametric form, as
Thus
Likewise, can be written in the form
or 0 艋 t 艋 1z 苷 5 ⫺ 5ty 苷 4x 苷 3
r共t兲 苷 共1 ⫺ t兲具3, 4, 5典 ⫹ t具3, 4, 0典 苷 具3, 4, 5 ⫺ 5t典
C
2
苷
y
1
0
共10 ⫹ 29t兲 dt 苷 10t ⫹ 29
t
2
2
册
0
1
苷 24.5
y
C
1
y dx ⫹ z dy ⫹ x dz 苷
y
1
0
共4t兲 dt ⫹ 共5t兲4 dt ⫹ 共2 ⫹ t兲5 dt
0 艋 t 艋 1z 苷 5ty 苷 4tx 苷 2 ⫹ t
r共t兲 苷 共1 ⫺ t兲具2, 0, 0典 ⫹ t 具3, 4, 5典 苷 具2 ⫹ t, 4t, 5t典
C
1
C
共3, 4, 0兲
共3, 4, 5兲C
2
共3, 4, 5兲共2, 0, 0兲
C
1
Cx
C
y dx ⫹ z dy ⫹ x dz
苷
s
2
2
[
t ⫺
1
2
sin 2t
]
0
2
苷
s
2
苷
s
2
y
2
0
1
2
共1 ⫺ cos 2t兲 dt苷
y
2
0
sin
2
t
s
sin
2
t ⫹ cos
2
t ⫹ 1 dt
y
C
y sin z ds 苷
y
2
0
共sin t兲 sin t
冑
冉
dx
dt
冊
2
⫹
冉
dy
dt
冊
2
⫹
冉
dz
dt
冊
2
dt
0 艋 t 艋 2
z 苷 ty 苷 sin tx 苷 cos t
Cx
C
y sin z ds
V
t.dz兲dydxzy共x
y
C
P共x, y, z兲 dx ⫹ Q共x, y, z兲 dy ⫹ R共x, y, z兲 dz
10
苷
y
b
a
f
(
x共t兲, y共t兲, z共t兲
)
z⬘共t兲 dt
y
C
f 共x, y, z兲 dz 苷 lim
n
l
⬁
兺
n
i苷1
f 共x
i
*
, y
i
*
, z
i
*
兲 ⌬z
i
zyxC
1076
||||
CHAPTER 17 VECTOR CALCULUS
FIGURE 9
1
x
z
y
C
1
0
_1
0
_1
0
2
4
6
1
x
z
y
C
1
0
_1
0
_1
0
2
4
6
FIGURE 10
y
z
x
0
(3,4,5)
(3,4,0)
(2,0,0)
C¡
C™
Then , so
Adding the values of these integrals, we obtain
M
LINE INTEGRALS OF VECTOR FIELDS
Recall from Section 6.4 that the work done by a variable force in moving a particle
from to along the -axis is . Then in Section 13.3 we found that the
work done by a constant force in moving an object from a point to another point in
space is , where
l
is the displacement vector.
Now suppose that is a continuous force field on , such as the
gravitational field of Example 4 in Section 17.1 or the electric force field of Example 5 in
Section 17.1. (A force field on could be regarded as a special case where and
and depend only on and .) We wish to compute the work done by this force in mov-
ing a particle along a smooth curve .
We divide into subarcs with lengths by dividing the parameter interval
into subintervals of equal width. (See Figure 1 for the two-dimensional case or
Figure 11 for the three-dimensional case.) Choose a point on the th subarc
corresponding to the parameter value . If is small, then as the particle moves from
to along the curve, it proceeds approximately in the direction of , the unit tan-
gent vector at . Thus the work done by the force in moving the particle from to
is approximately
and the total work done in moving the particle along is approximately
where is the unit tangent vector at the point on . Intuitively, we see that
these approximations ought to become better as becomes larger. Therefore we define the
work done by the force field as the limit of the Riemann sums in (11), namely,
Equation 12 says that work is the line integral with respect to arc length of the tangential
component of the force.
If the curve is given by the vector equation , then
, so using Equation 9 we can rewrite Equation 12 in the form
苷
y
b
a
F共r共t兲兲 ⴢ r⬘共t兲 dt W 苷
y
b
a
冋
F共r共t兲兲 ⴢ
r⬘共t兲
ⱍ
r⬘共t兲
ⱍ
册
ⱍ
r⬘共t兲
ⱍ
dt
T共t兲 苷 r⬘共t兲兾
ⱍ
r⬘共t兲
ⱍ
r共t兲 苷 x共t兲 i ⫹ y共t兲 j ⫹ z共t兲 kC
W 苷
y
C
F共x, y, z兲 ⴢ T共x, y, z兲 ds 苷
y
C
F ⴢ T ds
12
FW
n
C共x, y, z兲T共x, y, z兲
兺
n
i苷1
关F共x
i
*
, y
i
*
, z
i
*
兲 ⴢ T共x
i
*
, y
i
*
, z
i
*
兲兴 ⌬s
i
11
C
F
共
x
i
*
,
y
i
*
, z
i
*
兲 ⴢ 关⌬s
i
T共t
i
*
兲兴 苷 关F共x
i
*
, y
i
*
, z
i
*
兲 ⴢ T共t
i
*
兲兴 ⌬s
i
P
i
P
i⫺1
FP
i
*
T共t
i
*
兲P
i
P
i⫺1
⌬s
i
t
i
*
iP
i
*
共x
i
*
, y
i
*
, z
i
*
兲
关a, b兴
⌬s
i
P
i⫺1
P
i
C
C
yxQ
PR 苷 0⺢
2
⺢
3
F 苷 P i ⫹ Q j ⫹ R k
D 苷 PQW 苷 F ⴢ D
QPF
W 苷 x
b
a
f 共x兲 dxxba
f 共x兲
y
C
y dx ⫹ z dy ⫹ x dz 苷 24.5 ⫺ 15 苷 9.5
y
C
2
y dx ⫹ z dy ⫹ x dz 苷
y
1
0
3共⫺5兲 dt 苷 ⫺15
dx 苷 0 苷 dy
SECTION 17.2 LINE INTEGRALS
||||
1077
FIGURE 11
0
F(x
*
i
,y
*
i
,z
*
i
)
T(t
*
i
)
P
i
P¸
P
i-1
P
*
i
(x
*
i
,y
*
i
,z
*
i
)
y
z
x
P
n
This integral is often abbreviated as and occurs in other areas of physics as well.
Therefore we make the following definition for the line integral of any continuous vector
field.
DEFINITION Let be a continuous vector field defined on a smooth curve
given by a vector function , . Then the line integral of along C is
When using Definition 13, remember that is just an abbreviation for
, so we evaluate simply by putting , , and
in the expression for . Notice also that we can formally write .
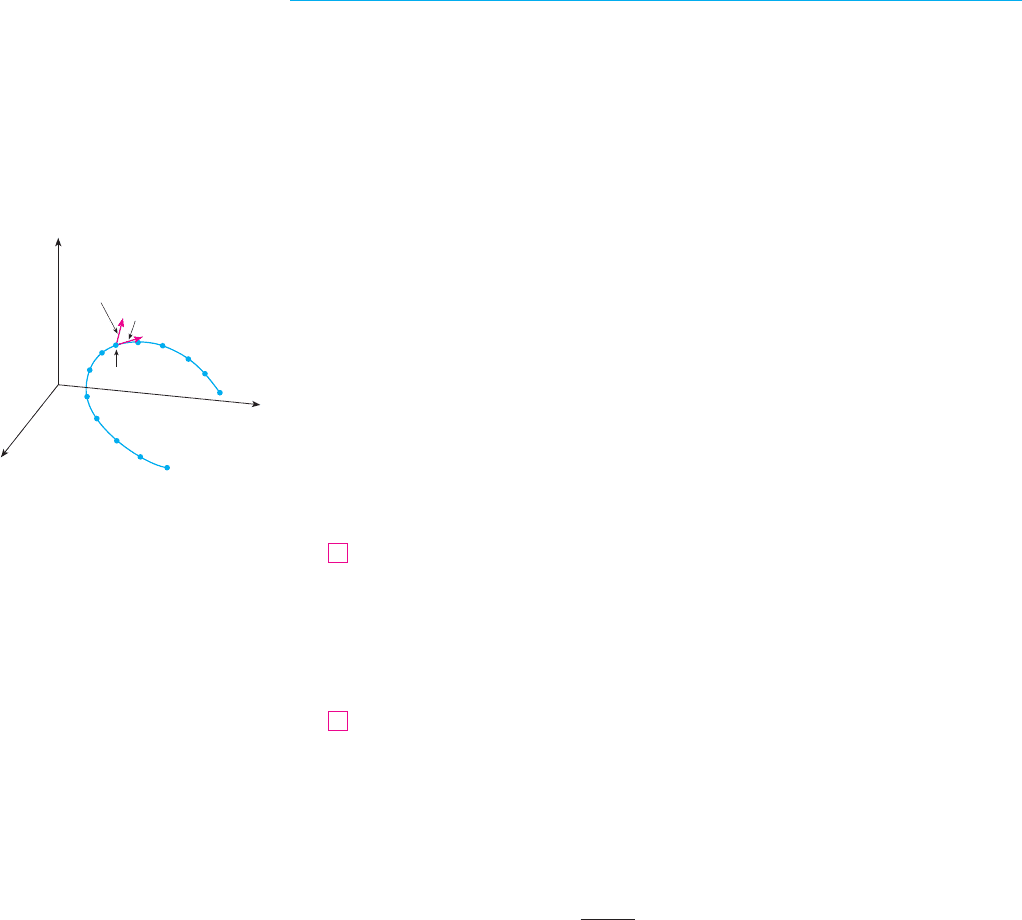
EXAMPLE 7 Find the work done by the force field in moving a
particle along the quarter-circle , .
SOLUTION Since and , we have
and
Therefore the work done is
M
Even though and integrals with respect to arc length are
unchanged when orientation is reversed, it is still true that
because the unit tangent vector is replaced by its negative when is replaced by
EXAMPLE 8 Evaluate , where and is the
twisted cubic given by
SOLUTION We have
F共r共t兲兲 苷 t
3
i ⫹ t
5
j ⫹ t
4
k
r⬘共t兲 苷 i ⫹ 2t j ⫹ 3t
2
k
r共t兲 苷 t i ⫹ t
2
j ⫹ t
3
k
0 艋 t 艋 1z 苷 t
3
y 苷 t
2
x 苷 t
CF共x, y, z兲 苷 xy i ⫹ yz j ⫹ z x kx
C
F ⴢ dr
⫺C.CT
y
⫺C
F ⴢ dr 苷 ⫺
y
C
F ⴢ dr
x
C
F ⴢ dr 苷 x
C
F ⴢ T ds
NOTE
苷 2
cos
3
t
3
册
0
兾2
苷 ⫺
2
3
y
C
F ⴢ dr 苷
y
兾2
0
F共r共t兲兲 ⴢ r⬘共t兲 dt 苷
y
兾2
0
共⫺2 cos
2
t sin t兲 dt
r⬘共t兲 苷 ⫺sin t i ⫹ cos t j
F共r共t兲兲 苷 cos
2
t i ⫺ cos t sin t j
y 苷 sin tx 苷 cos t
0 艋 t 艋
兾2r共t兲 苷 cos t i ⫹ sin t j
F共x, y兲 苷 x
2
i ⫺ xy j
dr 苷 r⬘共t兲 dtF共x, y, z兲
z 苷 z 共t兲y 苷 y共t兲x 苷 x共t兲F共r共t兲兲F共x共t兲, y共t兲, z共t兲兲
F共r共t兲兲
y
C
F ⴢ dr 苷
y
b
a
F共r共t兲兲 ⴢ r⬘共t兲 dt 苷
y
C
F ⴢ T ds
Fa 艋 t 艋 br共t兲
CF
13
x
C
F ⴢ dr
1078
||||
CHAPTER 17 VECTOR CALCULUS
N Figure 12 shows the force field and the curve
in Example 7. The work done is negative because
the field impedes movement along the curve.
FIGURE 12
0
1
1
y
x
N Figure 13 shows the twisted cubic in
Example 8 and some typical vectors acting at
three points on .C
C
FIGURE 13
y
z
x
0
0.5
1
1.5
2
2
1
0
1
2
0
F
{
r(1)
}
F
{
r(3/4)
}
F
{
r(1/2)
}
(1,1,1)
C
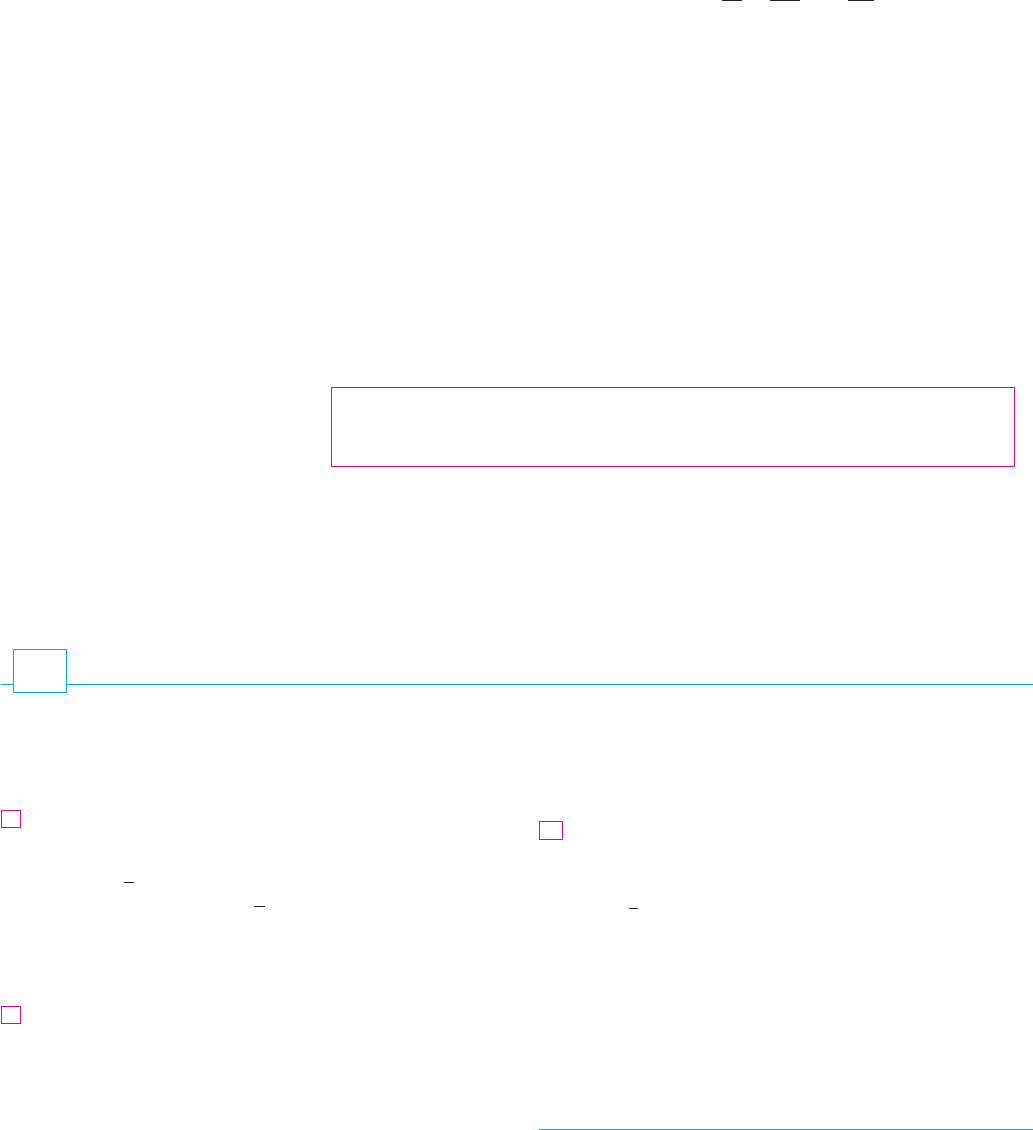
Thus
M
Finally, we note the connection between line integrals of vector fields and line integrals
of scalar fields. Suppose the vector field on is given in component form by the equa-
tion . We use Definition 13 to compute its line integral along :
But this last integral is precisely the line integral in (10). Therefore we have
For example, the integral in Example 6 could be expressed as
where
F共x, y, z兲 苷 y i ⫹ z j ⫹ x k
x
C
F ⴢ dr
x
C
y dx ⫹ z dy ⫹ x dz
where F 苷 P i ⫹ Q j ⫹ R k
y
C
F ⴢ dr 苷
y
C
P dx ⫹ Q dy ⫹ R dz
苷
y
b
a
[
P
(
x共t兲, y共t兲, z共t兲
)
x⬘共t兲 ⫹ Q
(
x共t兲, y共t兲, z共t兲
)
y⬘共t兲 ⫹ R
(
x共t兲, y共t兲, z共t兲
)
z⬘共t兲
]
dt
苷
y
b
a
共P i ⫹ Q j ⫹ R k兲 ⴢ
(
x⬘共t兲 i ⫹ y⬘共t兲 j ⫹ z⬘共t兲 k
)
dt
y
C
F ⴢ dr 苷
y
b
a
F共r共t兲兲 ⴢ r⬘共t兲 dt
CF 苷 P i ⫹ Q j ⫹ R k
⺢
3
F
苷
y
1
0
共t
3
⫹ 5t
6
兲
dt 苷
t
4
4
⫹
5t
7
7
册
0
1
苷
27
28
y
C
F ⴢ dr 苷
y
1
0
F共r共t兲兲 ⴢ r⬘共t兲 dt
SECTION 17.2 LINE INTEGRALS
||||
1079
9. ,
10. ,
is the line segment from to
,
is the line segment from (0, 0, 0) to (1, 2, 3)
12. ,: , , ,
13. ,: , , ,
14. ,
: , , ,
15. , consists of line
segments from to and from to
16. , consists of line segments from
to and from to
共3, 2, 0兲共1, 2, ⫺1兲共1, 2, ⫺1兲共0, 0, 0兲
C
x
C
x
2
dx ⫹ y
2
dy ⫹ z
2
dz
共2, 5, 2兲
共2, 3, 1兲共2, 3, 1兲共1, 0, 1兲
C
x
C
共x ⫹ yz兲 dx ⫹ 2x dy ⫹ xyz dz
0 艋 t 艋 1z 苷 t
2
y 苷 t
3
x 苷 t
2
C
x
C
z dx ⫹ x dy ⫹ y dz
0 艋 t 艋 1z 苷 t
2
y 苷 tx 苷 t
3
Cx
C
x
2
y
s
z
dz
0 艋 t 艋 1z 苷 t
3
y 苷 t
2
x 苷 tCx
C
共2x ⫹ 9z兲 ds
C
x
C
xe
yz
ds
11.
共1, 6, 4兲共⫺1, 5, 0兲C
x
C
xyz
2
ds
C: x 苷 2 sin t, y 苷 t, z 苷 ⫺2 cos t,0艋 t 艋
x
C
xyz ds
1–16 Evaluate the line integral, where is the given curve.
1. ,
2. ,
, is the right half of the circle
4. , is the line segment from to
5. ,
is the arc of the curve from to
6. ,
C is the arc of the curve from (1, 0) to
, consists of line segments from
to and from to
8. , consists of the top half of the circle
from to and the line segment from
to 共⫺2, 3兲共⫺1, 0兲
共⫺1, 0兲共1, 0兲x
2
⫹ y
2
苷 1
Cx
C
sin x dx ⫹ cos y dy
共3, 2兲共2, 0兲共2, 0兲共0, 0兲
C
x
C
xy dx ⫹ 共x ⫺ y兲 dy
7.
共e, 1兲x 苷 e
y
x
C
xe
y
dx
共4, 2兲共1, 1兲y 苷
s
x
C
x
C
(
x
2
y
3
⫺
s
x
)
dy
共4, 6兲共0, 3兲C
x
C
x sin y ds
x
2
⫹ y
2
苷 16Cx
C
xy
4
ds
3.
C: x 苷 t
2
, y 苷 2t,0艋 t 艋 1x
C
xy ds
C: x 苷 t
3
, y 苷 t,0艋 t 艋 2x
C
y
3
ds
C
EXERCISES
17.2
24. , where
and ,
25. , where has parametric equations ,
, ,
26. , where has parametric equations , ,
,
27–28 Use a graph of the vector field F and the curve C to guess
whether the line integral of F over C is positive, negative, or zero.
Then evaluate the line integral.
27. ,
is the arc of the circle traversed counter-
clockwise from (2, 0) to
28. ,
is the parabola from to (1, 2)
29. (a) Evaluate the line integral , where
and is given by
, .
;
(b) Illustrate part (a) by using a graphing calculator or com-
puter to graph and the vectors from the vector field
corresponding to , , and 1 (as in Figure 13).
30. (a) Evaluate the line integral , where
and is given by
, .
;
(b) Illustrate part (a) by using a computer to graph and
the vectors from the vector field corresponding to
and (as in Figure 13).
31. Find the exact value of , where is the curve with
parametric equations , , ,
.
32. (a) Find the work done by the force field
on a particle that moves once around the circle
oriented in the counterclockwise direction.
(b) Use a computer algebra system to graph the force field and
circle on the same screen. Use the graph to explain your
answer to part (a).
A thin wire is bent into the shape of a semicircle ,
. If the linear density is a constant , find the mass and
center of mass of the wire.
34. A thin wire has the shape of the first-quadrant part of the circle
with center the origin and radius . If the density function is
, find the mass and center of mass of the wire.
35. (a) Write the formulas similar to Equations 4 for the center of
mass of a thin wire in the shape of a space curve
if the wire has density function .
共x, y, z兲
C共x
, y, z 兲
共x, y兲 苷 kxy
a
kx 艌 0
x
2
⫹ y
2
苷 4
33.
CAS
x
2
⫹ y
2
苷 4
F共x, y兲 苷 x
2
i ⫹ xy j
0 艋 t 艋 2
z 苷 e
⫺t
y 苷 e
⫺t
sin 4tx 苷 e
⫺t
cos 4t
C
x
C
x
3
y
2
z
ds
CAS
⫾
1
2
t 苷 ⫾1
C
⫺1 艋 t 艋 1r共t兲 苷 2t i ⫹ 3t j ⫺ t
2
k
CF共x, y, z兲 苷 x i ⫺ z j ⫹ y k
x
C
F ⴢ dr
1兾
s
2
t 苷 0
C
0 艋 t 艋 1r共t兲 苷 t
2
i ⫹ t
3
j
CF共x, y兲 苷 e
x⫺1
i ⫹ xy j
x
C
F ⴢ dr
共⫺1, 2兲y 苷 1 ⫹ x
2
C
F共x, y兲 苷
x
s
x
2
⫹ y
2
i ⫹
y
s
x
2
⫹ y
2
j
共0, ⫺2兲
x
2
⫹ y
2
苷 4C
F共x, y兲 苷 共x ⫺ y兲 i ⫹ xy j
CAS
0 艋 t 艋 1z 苷 e
⫺t
y 苷 t
2
x 苷 tCx
C
ze
⫺xy
ds
0 艋 t 艋 5z 苷 t
4
y 苷 t
3
x 苷 t
2
Cx
C
x sin共y ⫹ z兲 ds
0 艋 t 艋
r共t兲 苷 cos t i ⫹ sin t j ⫹ sin 5t k
F共x, y, z兲 苷 y sin z i ⫹ z sin x j ⫹ x sin y k
x
C
F ⴢ dr
Let be the vector field shown in the figure.
(a) If is the vertical line segment from to ,
determine whether is positive, negative, or zero.
(b) If is the counterclockwise-oriented circle with radius 3
and center the origin, determine whether is posi-
tive, negative, or zero.
18. The figure shows a vector field and two curves and .
Are the line integrals of over and positive, negative,
or zero? Explain.
19–22 Evaluate the line integral , where is given by the
vector function .
19. ,
,
20. ,
,
,
,
22. ,
,
23–26 Use a calculator or CAS to evaluate the line integral correct
to four decimal places.
23. , where and
,1艋 t 艋 2r共t兲 苷 e
t
i ⫹ e
⫺t
2
j
F共x, y兲 苷 xy i ⫹ sin y j
x
C
F ⴢ dr
0 艋 t 艋
r共t兲 苷 t i ⫹ sin t j ⫹ cos t
k
F共x, y, z兲 苷 z
i ⫹ y j ⫺ x
k
0 艋 t 艋 1r共t兲 苷 t
3
i ⫺ t
2
j ⫹ t k
F共x, y, z兲 苷 sin x i ⫹ cos y j ⫹ xz k
21.
0 艋 t 艋 1r共t兲 苷 t
2
i ⫹ t
3
j ⫹ t
2
k
F共x, y, z兲 苷 共x ⫹ y兲
i ⫹ 共y ⫺ z兲 j ⫹ z
2
k
0 艋 t 艋 1r共t兲 苷 11t
4
i ⫹ t
3
j
F共x, y兲 苷 xy
i ⫹ 3y
2
j
r共t兲
C
x
C
F ⴢ dr
y
x
C¡
C™
C
2
C
1
F
C
2
C
1
F
y
x
0
1
1
2 3
2
3
_3 _2 _1
_3
_2
_1
x
C
2
F ⴢ dr
C
2
x
C
1
F ⴢ dr
共⫺3, 3兲共⫺3, ⫺3兲C
1
F
17.
1080
||||
CHAPTER 17 VECTOR CALCULUS

SECTION 17.2 LINE INTEGRALS
||||
1081
(b) Is this also true for a force field , where is a
constant and ?
46. The base of a circular fence with radius 10 m is given by
. The height of the fence at position
is given by the function , so
the height varies from 3 m to 5 m. Suppose that 1 L of paint
covers . Sketch the fence and determine how much paint
you will need if you paint both sides of the fence.
47. An object moves along the curve shown in the figure from
(1, 2) to (9, 8). The lengths of the vectors in the force field
are measured in newtons by the scales on the axes. Estimate
the work done by on the object.
48. Experiments show that a steady current in a long wire pro-
duces a magnetic field that is tangent to any circle that lies in
the plane perpendicular to the wire and whose center is the axis
of the wire (as in the figure). Ampère’s Law relates the electric
current to its magnetic effects and states that
where is the net current that passes through any surface
bounded by a closed curve , and is a constant called the
permeability of free space. By taking to be a circle with
radius , show that the magnitude of the magnetic
field at a distance from the center of the wire is
B
I
B 苷
0
I
2
r
r
B 苷
ⱍ
B
ⱍ
r
C
0
C
I
y
C
B ⴢ dr 苷
0
I
B
I
0
1
1
y
(meters)
x
(meters)
C
C
F
F
C
100 m
2
h共x, y兲 苷 4 ⫹ 0.01共x
2
⫺ y
2
兲共x, y兲
x 苷 10 cos t, y 苷 10 sin t
x 苷 具x, y 典
kF共x兲 苷 k x
(b) Find the center of mass of a wire in the shape of the helix
, , , , if the density
is a constant .
36. Find the mass and center of mass of a wire in the shape of the
helix , , , , if the density at
any point is equal to the square of the distance from the origin.
37. If a wire with linear density lies along a plane curve
its moments of inertia about the - and -axes are defined as
Find the moments of inertia for the wire in Example 3.
38. If a wire with linear density lies along a space curve
, its moments of inertia about the -, -, and -axes are
defined as
Find the moments of inertia for the wire in Exercise 35.
Find the work done by the force field
in moving an object along an arch of the cycloid
, .
40. Find the work done by the force field
on a particle that moves along the parabola from
to .
41. Find the work done by the force field
on a particle that moves
along the line segment from to .
42. The force exerted by an electric charge at the origin on a
charged particle at a point with position vector
is where is a constant. (See
Example 5 in Section 17.1.) Find the work done as the particle
moves along a straight line from to .
A 160-lb man carries a 25-lb can of paint up a helical staircase
that encircles a silo with a radius of 20 ft. If the silo is 90 ft
high and the man makes exactly three complete revolutions,
how much work is done by the man against gravity in climbing
to the top?
44. Suppose there is a hole in the can of paint in Exercise 43 and
9 lb of paint leaks steadily out of the can during the man’s
ascent. How much work is done?
45. (a) Show that a constant force field does zero work on a
particle that moves once uniformly around the circle
.x
2
⫹ y
2
苷 1
43.
共2, 1, 5兲共2, 0, 0兲
KF共r兲 苷 Kr兾
ⱍ
r
ⱍ
3
r 苷 具x, y, z 典
共x, y, z兲
共3, 4, 2兲共1, 0, 0兲
F共x, y, z兲 苷 具 y ⫹ z, x ⫹ z, x ⫹ y典
共2, 4兲共⫺1, 1兲
y 苷 x
2
F共x, y兲 苷 x sin y i ⫹ y j
0 艋 t 艋 2
r共t兲 苷 共t ⫺ sin t兲 i ⫹ 共1 ⫺ cos t兲 j
F共x, y兲 苷 x i ⫹ 共 y ⫹ 2兲 j
39.
I
z
苷
y
C
共x
2
⫹ y
2
兲
共x, y, z兲 ds
I
y
苷
y
C
共x
2
⫹ z
2
兲
共x, y, z兲 ds
I
x
苷
y
C
共 y
2
⫹ z
2
兲
共x, y, z兲 ds
zyxC
共x, y, z兲
I
y
苷
y
C
x
2
共x, y兲 dsI
x
苷
y
C
y
2
共x, y兲 ds
yx
C,
共x, y兲
0 艋 t 艋 2
z 苷 sin ty 苷 cos tx 苷 t
k
0 艋 t 艋 2
z 苷 3ty 苷 2 cos tx 苷 2 sin t
