Stewart J. College Algebra: Concepts and Contexts
Подождите немного. Документ загружается.

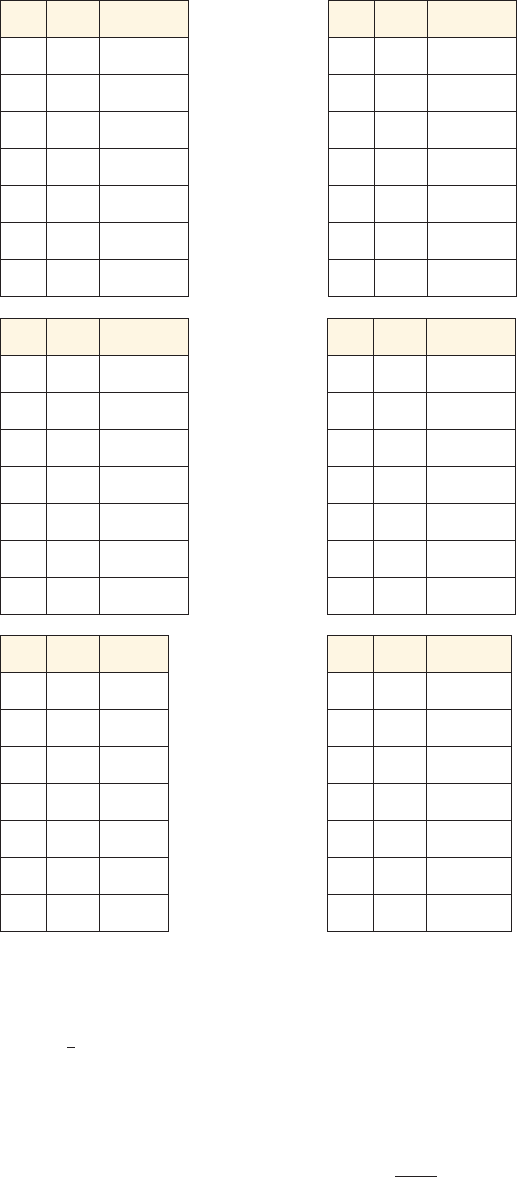
x
f 1x 2 f 1x ⴙ 22
- 6
105
99
- 4
99
- 2
82
0 53
2 20
4 6
6 2 —
x
f 1x 2 f 1x2ⴙ 1
- 3
20
21
- 2
27
- 1
53
0 42
1 39
2 70
3 21
424 CHAPTER 5
■
Quadratic Functions and Models
19–24
■ A function f is given by the table. Complete the table for the given transformation.
19. 20.
21. 22.
x
f 1x 2 f 1x2ⴚ 1
- 3
9
8
- 2
28
- 1
15
0 20
1 31
2 27
3 19
x
f 1x 2 f 1x ⴚ 22
- 6
13 —
- 4
29
13
- 2
38
0 49
2 55
4 62
6 75
x
f 1x 2 f 1ⴚx2
- 6
105
2
- 4
99
- 2
82
0 53
2 20
4 6
6 2
x
f 1x 2 1 ⴚ f 1x2
- 6
105
- 104
- 4
99
- 2
82
0 53
2 20
4 6
6 2
25–28 ■ Sketch the graph of the function, not by plotting points, but by starting with the
graph of a basic function and applying a vertical shift.
25. 26.
27. 28.
29–32
■ Sketch the graph of the function, not by plotting points, but by starting with the
graph of a basic function and applying a horizontal shift.
29. 30.
31. 32. f 1x 2= 1x + 4
f 1x 2= 0x - 3 0
f 1x 2= 1x + 12
2
f 1x 2= 1x - 52
2
f 1x 2= 0x 0- 1f 1x2= 1x + 1
f 1x 2= x
3
+ 5f 1x 2= x
2
- 1
23. 24.

SECTION 5.1
■
Working with Functions: Shifting and Stretching 425
33–36 ■ Sketch the graph of the function, not by plotting points, but by starting
with the graph of a basic function and applying vertical stretching or
shrinking.
33. 34.
35. 36.
37–52
■ Sketch the graph of the function, not by plotting points, but by starting with the
graph of a basic function and applying transformations.
37. 38.
39. 40.
41. 42.
43. 44.
45. 46.
47. 48.
49. 50.
51. 52.
53–60
■ A function f is given, and the indicated transformations are applied to its graph (in
the given order). Write the equation for the final transformed graph.
53. ; shift upward 3 units
54. ; shift downward 1 unit
55. ; shift 2 units to the left
56. ; shift 1 unit to the right
57. ; shift 3 units to the right and shift upward 1 unit
58. ; shift 2 units to the left and reflect in the x-axis
59. ; shift downward 2 units
60. ; shift 2 units to the right and reflect in the y-axis
61–64
■ The graphs of f and are given. Find a formula for the function .
61. 62.
gg
f 1x 2= log
3
x
f 1x 2= 2
x
f 1x 2= x
2
f 1x 2= 0 x 0
f 1x 2= 1x
f 1x 2= 1x
f 1x 2= x
2
f 1x 2= x
2
f 1x 2=-log
10
1x + 22f 1x 2=-log
5
1- x2
f 1x 2= log
2
1x + 22f 1x 2= log
2
1x - 42
f 1x 2= 1 + 2
-x
f 1x 2=-3
x
f 1x 2= 3
x
+ 5f 1x 2= 2
x
- 3
f 1x 2= 3 - 0
x - 2 0f 1x2= 6 - 1x + 4
f 1x 2=-1x + 42
2
- 3f 1x 2=-1x - 32
2
+ 5
f 1x 2=-1x
f 1x 2=-0 x 0
f 1x 2=-2 - x
2
f 1x 2= 1 - x
2
f 1x 2=
1
5
x
3
f 1x 2= 2x
4
f 1x 2= 3 0 x 0f 1x2=
1
4
x
2
x
y
g
0
f(x) = x
2
1
1
x
y
g
f(x) =
log¤ x
1
1
0
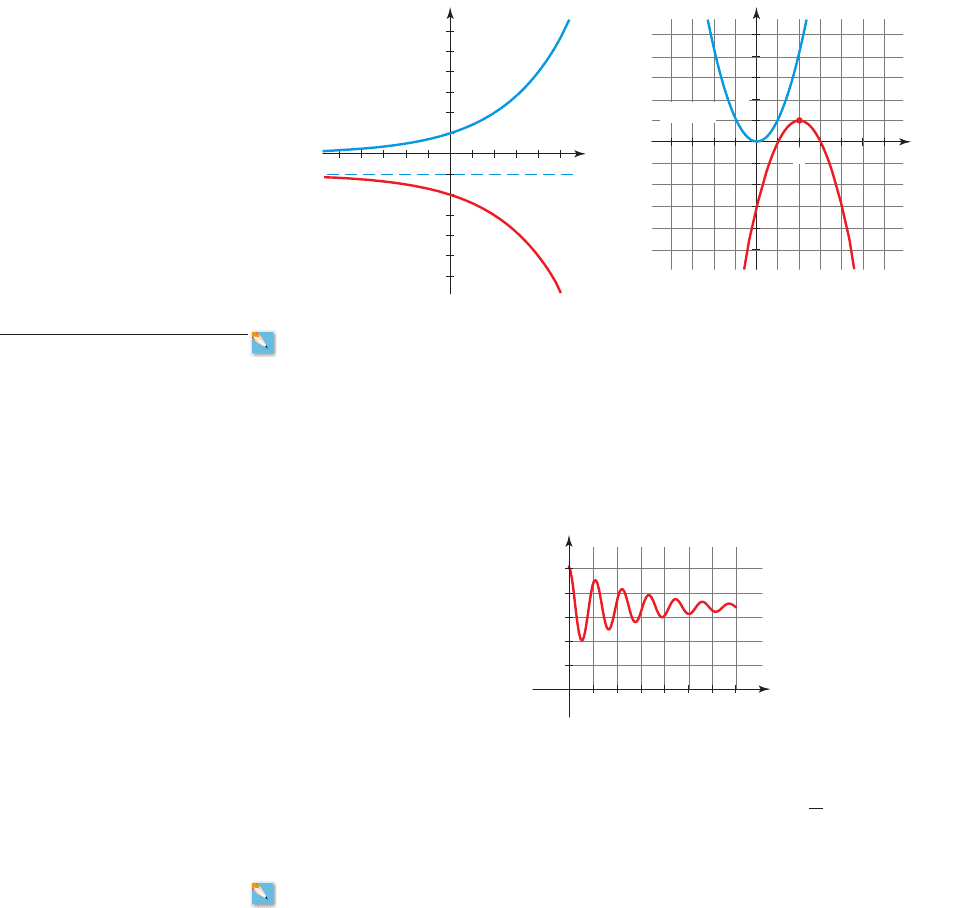
426 CHAPTER 5
■
Quadratic Functions and Models
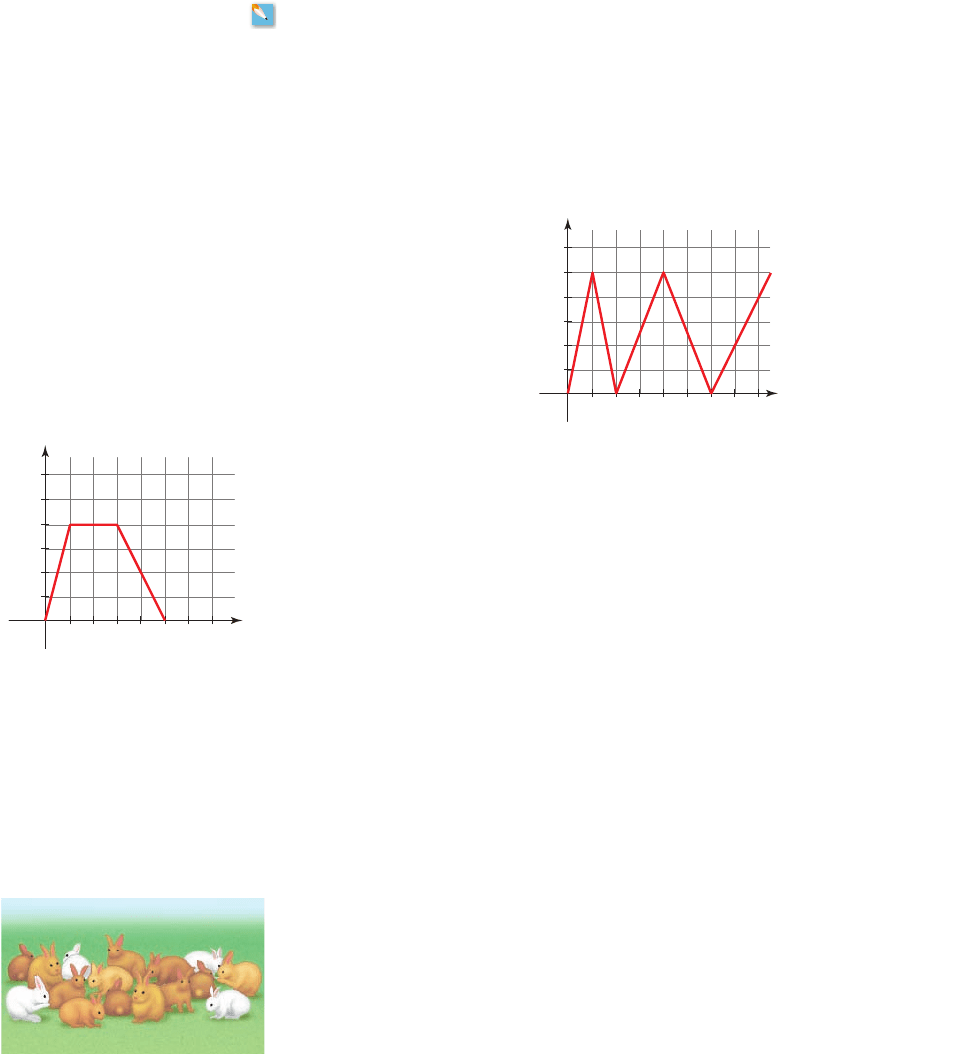
65. Bungee Jumping Luisa goes bungee jumping from a 500-foot-high bridge. The
graph shows Luisa’s height (in feet) after t seconds.
(a) Describe in words what the graph indicates about Luisa’s bungee jump.
(b) Suppose Luisa goes bungee jumping from a 400-foot-high bridge. Sketch a new
graph that shows Luisa’s height after t seconds.
(c) What transformation must be performed on the function h to obtain the function H?
Express the function H in terms of h.
H1t 2
h1t 2
CONTEXTS
t (s)
y
(ft)
500
4
0
x
y
g
f(x) = 2
x
2
_2
_4
_6
4
6
12_2 _1
0
x
y
g
0
f(x) = x
2
2
2
63. 64.
66. Train Ride Liam is riding a train that is traveling at a constant speed of 20 m/s. Liam
is inside the train walking in the direction opposite to that in which the train is moving.
Liam’s speed relative to the train at time t (in seconds) is /s. Find a
function that gives Liam’s speed relative to the ground. How are the graphs of and
related?
67. Distance Alessandra leaves home 30 minutes after her brother Alberto and drives at a
constant speed of 90 km/h on a straight road.
(a) Find a function d that models Alessandra’s distance from home t hours after she
started her trip.
(b) Find a function D that models Alessandra’s distance from home t hours after
Alberto started his trip.
(c) Graph the functions d and D. How are the graphs related?
68. Sales Growth The annual sales of a certain company can be modeled by the function
, where t represents years since 1990 and is measured in millions
of dollars.
(a) What shifting and shrinking operations must be performed on the function to
obtain the function ?
(b) Suppose you want t to represent years since 2000 instead of 1990. What
transformation would you have to apply to the function to accomplish
this? Write the new function that results from this transformation.y = g1t2
y = f 1t 2
y = f 1t 2
y = t
2
f 1t 2f 1t 2= 4 + 0.01t
2
V√V
√1t 2= 415t
m
SECTION 5.1
■
Working with Functions: Shifting and Stretching 427
69. Swimming Laps Miyuki practices swimming laps with her team. The function
graphed below gives her distance (in meters) from the starting edge of the pool
t seconds after she starts her laps.
(a) Describe in words Miyuki’s swim practice. What is her average speed for the first
30 seconds?
(b) Graph the function . How is the graph of the new function related to the
graph of the original function?
(c) What is Miyuki’s new average speed for the first 30 seconds?
y = 1.2f 1t 2
y = f 1t 2
t (s)
d (m)
50
30
0
t (min
)
d (ft)
200
10
0
70. Field Trip A class of fourth graders walks to a park on a field trip. The function
graphed in the margin gives their distance from school (in feet) t minutes after
they left school.
(a) What is the average speed going to the park? How long was the class at the park?
How far away is the park?
(b) Graph the function . How is the graph of the new function related to
the graph of the original function? What is the average speed going to the new
park? How far away is the new park?
(c) Graph the function . How is the graph of the new function related to
the graph of the original function? How does the field trip described by this
function differ from the original trip?
71. Bacterial Infection A culture of the bacteria Streptococcus pneumoniae
(S. pneumoniae) initially has 10 bacteria and increases at a rate of 5% per minute.
(a) Find a function that represents the number of bacteria in the culture after
t minutes.
(b) Suppose the culture initially has 50 bacteria and is the number of bacteria after
t minutes. Describe the stretching or shrinking operations that must be performed
on the function to obtain the function . Write the new function
that results from this transformation.
72. Rabbit Population A population of 20 rabbits is introduced to a small island. The
population increases at a rate of 60% per year.
(a) Find a function that represents the number of rabbits on the island after t years.
(b) Suppose 100 rabbits were introduced to the island and is the number of rabbits
after t years. Describe the stretching or shrinking operations that must be performed
on the function to obtain the function . Write the new function
that results from this transformation.
73. Newton’s Law of Cooling A cup of coffee has a temperature of and is placed
in a room that has a temperature of . The temperature of the coffee after t minutes
is modeled by the function
(a) Describe the transformations you would apply to the function to obtain
the function .
(b) Graph the function T by applying transformations to the graph of .y = 10.95 2
t
y = T1t2
y = 10.95 2
t
T1t 2= 75 + 11510.95 2
t
75°F
190°F
y = g1t2
y = g1t2y = f 1t 2
g1t 2
f 1t 2
y = g1t2
y = g1t2y = f 1t 2
g1t 2
f 1t 2
y = f 1t - 102
y = 0.50f 1t 2
y = f 1t 2

428 CHAPTER 5
■
Quadratic Functions and Models
2
■ The Squaring Function
A squaring function is a function of the form
where C is a number different from zero. We’ll see in this section that the squaring
function is the prototype quadratic function.
We all know that when we drop an object from a high building, the object falls
faster and faster. In fact, the distance d (in feet) it falls in t seconds is given by the
function . But suppose that you make a mistake in your physics class and
you write this function as (not squared). This apparently small error
makes a huge difference in reality. If objects fell according to this rule, we would
have quite a different world. For one thing, we would be able to leap over tall trees
with ease, and we wouldn’t need a parachute to go skydiving. We compare these two
different functions in the next example.
d1t 2= 16t
d1t 2= 16t
2
f 1x2= Cx
2
Every time you throw a ball, it
travels according to a quadratic
function.
example
1
Comparing Linear and Squaring Functions
Dave and his friend Harriet (who has superpowers) go skydiving together. In t sec-
onds Dave falls , but Harriet falls feet.
(a) Make a table of values for the functions D and H, and find their average rate
of change on intervals of length 1 second, for t between 0 and 5 seconds.
(b) What do the tables in part (a) indicate about how fast Dave and Harriet are
falling? Which skydiver do you think will have the softer landing?
H1t 2= 16tD1t 2= 16t
2
2
5.2 Quadratic Functions and Their Graphs
■
The Squaring Function
■
Quadratic Functions in General Form
■
Quadratic Functions in Standard Form
■
Graphing Using the Standard Form
IN THIS SECTION… we study quadratic functions and compare them to linear
functions. We learn how to express quadratic functions in standard form and then use the
standard form to graph such functions.
GET READY… by reviewing how to expand expressions and how to complete the square
in Algebra Toolkits B.1 and B.2. Test your understanding by doing the Algebra
Checkpoint at the end of this section.
Some of the most important functions in modeling the real world are functions that
involve squaring a quantity. Such functions are called quadratic functions. For ex-
ample, you are familiar with the function that gives the area of a square, ,
or the area of a circle, . One of the most fundamental functions in our
everyday experience is the quadratic function . This function gives the
distance d that a falling object travels in t seconds under the force of gravity. Every
time you throw a ball, drop your keys, or ride a roller coaster, you are experiencing
this function in action. In this section we begin our study of this important family of
functions.
d1t 2= 16t
2
A1r 2= pr
2
A1x 2= x
2
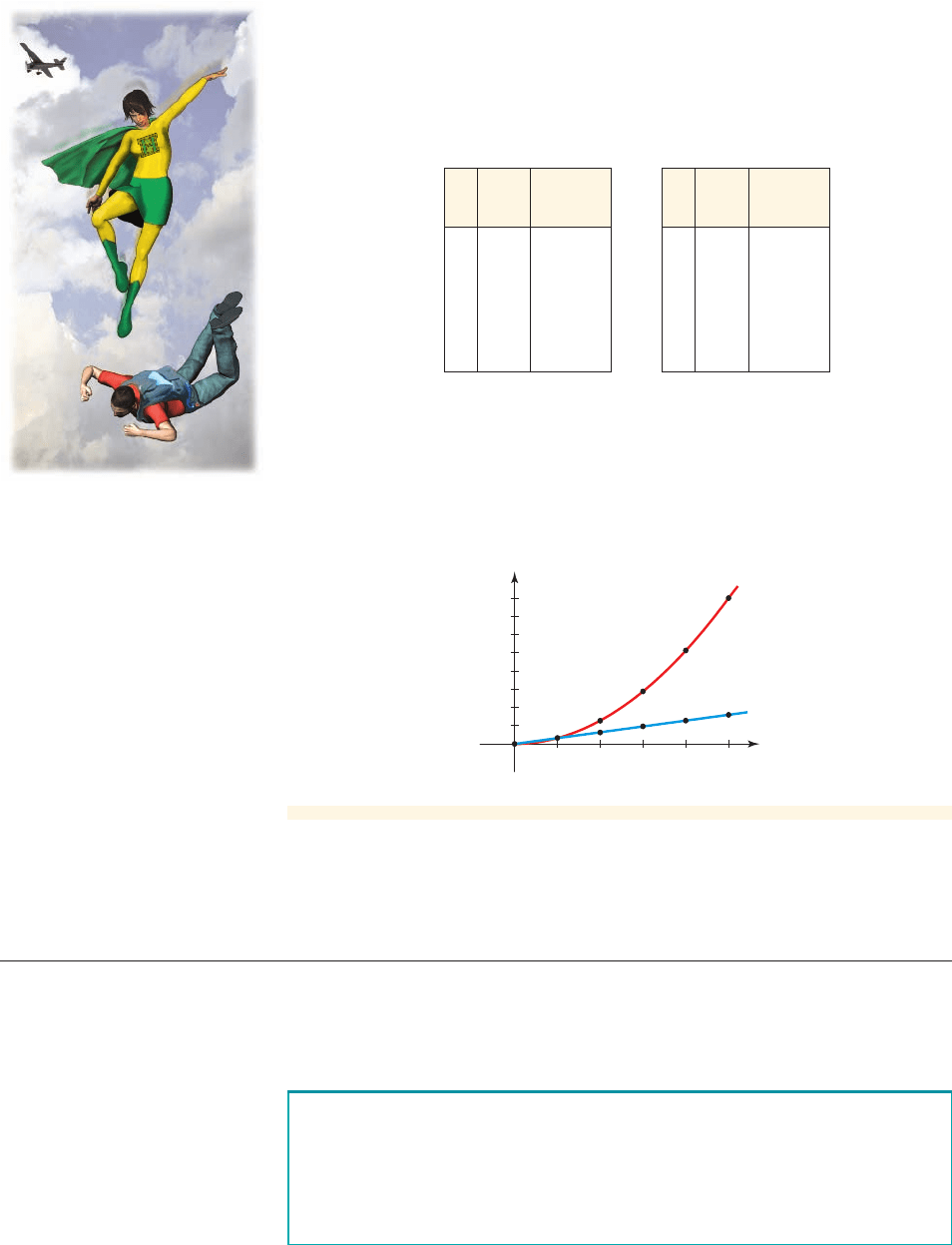
SECTION 5.2
■
Quadratic Functions and Their Graphs 429
H(t) 16 t Harriet
DaveD(t) 16 t
2
The average rate of change of
distance with respect to time is the
average speed. Average speed is
studied in Section 2.1.
t
D1t 2
Rate of
change
0 0 —
1 16 16
2 64 48
3 144 96
4 256 128
5 400 160
t
H1t 2
Rate of
change
0 0 —
1 16 16
2 32 16
3 48 16
4 64 16
5 80 16
(c) Sketch a graph of the functions D and H. What information can we get from
these graphs?
Solution
(a) The tables below give the values and average rates of change of the functions
D and H.
d (ft)
t (s)
H
D
0
12345
100
200
300
400
figure 1 Graphs of D and H
(b) Since H is a linear function, its rate of change is constant (Section 2.2). So
Harriet falls at a constant speed of 16 ft/s. Dave falls a lot faster; from the
table we see that his average speed increases on each succeeding one-second
interval. Clearly, Harriet will have the softer landing.
(c) The graphs in Figure 1 indicate that the gap between how far Dave has fallen
and how far Harriet has fallen keeps widening with passing time.
■ NOW TRY EXERCISE 43 ■
Example 1 shows that a squaring function grows much faster than a linear function.
On the other hand, exponential functions grow much faster than squaring functions, as
we will see in Example 1 of Section 6.2, page 494.
2
■ Quadratic Functions in General Form
A quadratic function is a combination of a squaring function and a linear function.
Quadratic Functions
A quadratic function is a function of the form
where a, b, c are real numbers and . This form of a quadratic function
is called the general form.
a 0
f 1x2= ax
2
+ bx + c

430 CHAPTER 5
■
Quadratic Functions and Models
example
2
Identifying Quadratic Functions
Determine whether the given function is a quadratic function.
(a) (b) (c)
Solution
(a) Notice that , so f is a quadratic function with ,
, and .
(b) The function is not a quadratic function, since has a term involving .
(c) The function h is not a quadratic function, since h does not have a nonzero
term involving .
■ NOW TRY EXERCISE 7 ■
x
2
x
3
gg
c = 3b = 0
a = 0.1f 1x2= 0.1x
2
+ 0
#
x + 3
h1x 2= 3
2
x + 1g1x 2= x
3
- 2x + 1f 1x2= 0.1x
2
+ 3
example
3
Expressing a Quadratic Function in General Form
Express the function in the general form of a quadratic
function.
Solution
We use the distributive property to multiply the factors of f:
Given function
Distributive property
Combine like terms
The general form is .
■ NOW TRY EXERCISE 9 ■
f 1x2= 2x
2
- 16x - 96
= 2x
2
- 16x - 96
= 2x
2
+ 8x - 24x - 96
f 1x 2= 12x + 8 21x - 122
f 1x2= 12x + 821x - 122
We study how to multiply
algebraic expressions using the
distributive property in Algebra
Toolkit B.1, page T25.
example
4
Expressing a Quadratic Function in General Form
Express the function in the general form of a quadratic
function.
Solution
We multiply out the squared term of f using Special Product Formula 1 (see
Algebra
Toolkit B.1
):
Given function
Special Product Formula 1
Multiply
Combine constant terms
The general form is .
■ NOW TRY EXERCISE 13 ■
f 1x2= 3x
2
- 24x + 49
= 3x
2
- 24x + 49
= 3x
2
- 24x + 48 + 1
= 31x
2
- 8x + 162+ 1
f 1x 2= 31x - 42
2
+ 1
f 1x2= 31x - 4 2
2
+ 1

SECTION 5.2
■
Quadratic Functions and Their Graphs 431
2
■ Quadratic Functions in Standard Form
In Example 4 we saw that the function can be expressed in the
general form of a quadratic function. This is an example of a quadratic function in
standard form according to the following definition.
Standard Form of a Quadratic Function
f 1x2= 31x - 4 2
2
- 1
example
5
Expressing Quadratic Functions in Standard Form
Express the quadratic function in standard form.
Solution
To get f in standard form we “complete the square” by first taking half of the coeffi-
cient of x and squaring it: . We then add and subtract the result.
The standard form is .
■ NOW TRY EXERCISE 21 ■
f 1x2= 1x + 82
2
- 40
= 1x + 82
2
- 40
= 1x
2
+ 16x + 642- 64 + 24
perfect square
f 1x 2= x
2
+ 16x + 24
A
16
2
B
2
= 64
f 1x2= x
2
+ 16x + 24
A quadratic function can be expressed in the standard
form
by completing the square.
f 1x2= a1x - h 2
2
+ k
f 1x2= ax
2
+ bx + c
The next example shows how “completing the square” allows us to express a
quadratic function in standard form.
We study how to factor perfect
squares in Algebra Toolkit B.2,
page T33.
Given function
Complete the square: Add 64 inside
parentheses, and subtract 64 outside
Factor and simplify
e
e
example
6
Expressing a Quadratic Function in Standard Form
Express the quadratic function in standard form.
Solution
Since the coefficient of is not 1, we must factor this coefficient from the terms in-
volving x before completing the square.
The standard form is .
■ NOW TRY EXERCISE 23 ■
f 1x2= 21x - 3 2
2
+ 5
= 21x - 32
2
+ 5
= 21x
2
- 6x + 92- 2
#
9 + 23
perfect square
= 21x
2
- 6x2+ 23
f 1x 2= 2x
2
- 12x + 23
x
2
f 1x2= 2x
2
- 12x + 23
Given function
Factor 2 from x-terms
Complete the square: Add 9 inside
parentheses, and subtract outside
Factor and simplify
2
#
9
432 CHAPTER 5
■
Quadratic Functions and Models
2
■ Graphing Using the Standard Form
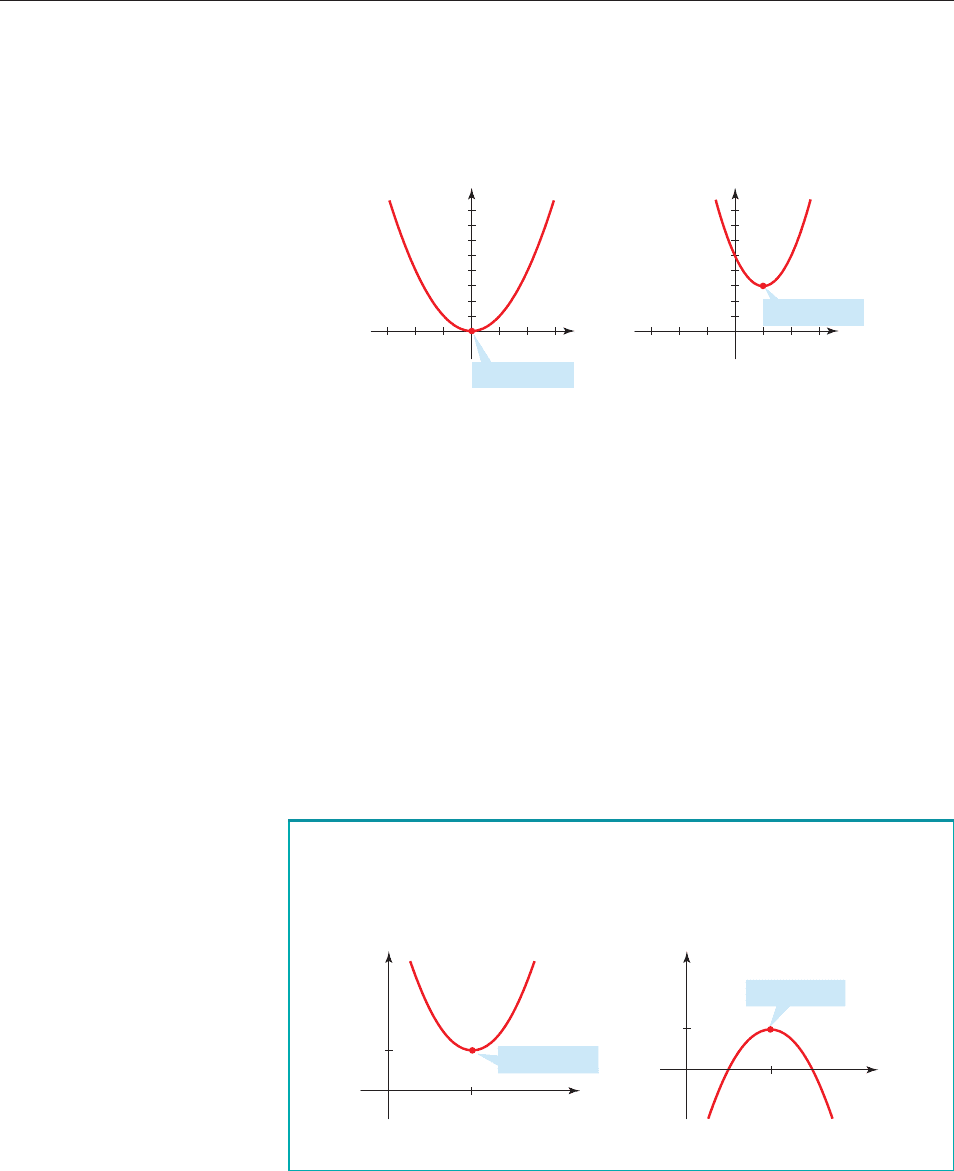
Recall that the graph of the squaring function is a U-shaped curve called
a parabola. The vertex of this parabola is at the point (0, 0) (see Example 3 in
Section 1.6). The graph of f is shown in Figure 2(a).
f 1x2= x
2
1
Vertex (0, 0)
x
y
2
0
1
(a) Graph of f(x)=x
2
(b) Graph of f(x)=2(x-1)
2
+3
x
y
2
0
Vertex (1, 3)
figure 2
If we transform f by shifting 1 unit to the right, stretching by a factor of 2, and
then shifting upward 3 units, we get the function
We recognize this as a quadratic function in standard form (see Figure 2(b)). Since
any quadratic function can be put into standard form, we see that the graph of any
quadratic function is a parabola obtained by transforming the basic squaring func-
tion . Notice that the standard form of a quadratic function allows us to
quickly identify the location of the vertex. In this example the vertex is at (1, 3)
because the original vertex (0, 0) has been shifted 1 unit to the right and 3 units
upward.
Graphs of Quadratic Functions
f 1x2= x
2
f 1x2= 21x - 1 2
2
+ 3
To graph a quadratic function, we first express it in standard form.
The graph of the quadratic function in standard form
is a parabola with vertex ; the parabola opens upward if or
downward if .a 6 0
a 7 01h, k 2
f 1x2= a1x - h 2
2
+ k
y
x
0
Ï=a(x-h)™+k, a>0
y
x
0
Ï=a(x-h)™+k, a<0
h
k
h
Vertex (h, k)
Vertex (h, k)
k
SECTION 5.2
■
Quadratic Functions and Their Graphs 433
example
7
Graphing a Quadratic Function
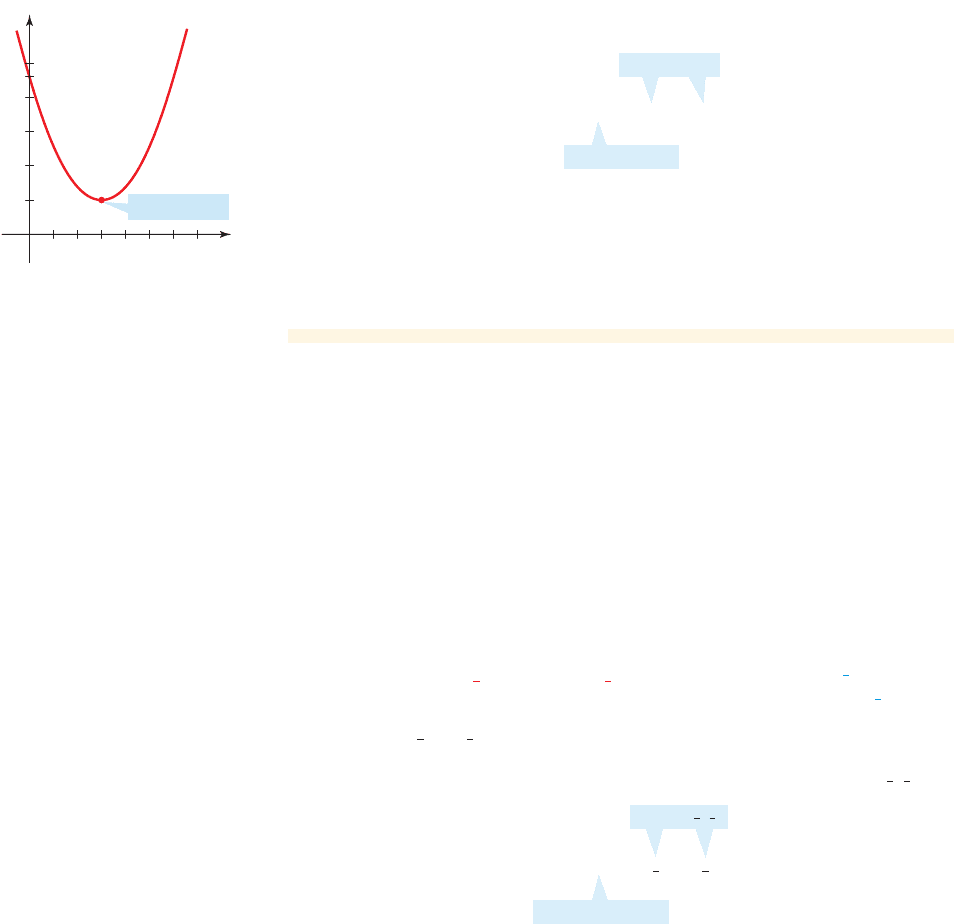
Let .
(a) Express f in standard form.
(b) Sketch a graph of f.
Solution
(a) In Example 6 we found that the standard form of f is
(b) The standard form tells us that we get the graph of f by taking the parabola
and performing the following transformations: Shift to the right 3 units,
stretch by a factor of 2, then shift upward 5 units. The vertex of the parabola is at
(3, 5). The parabola opens upward because the coefficient 2 is positive.
Vertex (3, 5)
Opens upward
The y-intercept is obtained by evaluating f at 0. This is more easily done by
using the general form of a quadratic function. In this case
So the y-intercept is 23. The graph is shown in Figure 3.
■ NOW TRY EXERCISE 29 ■
f 102= 210 2
2
- 1210 2+ 23 = 23
f 1x2= 21x - 3 2
2
+ 5
y = x
2
f 1x2= 21x - 3 2
2
+ 5
f 1x2= 2x
2
- 12x + 23
y
x
25
Ï=2(x-3)™+5
23
15
5
3
0
Vertex (3, 5)
figure 3
example
8
Graphing a Quadratic Function
Let .
(a) Express f in standard form.
(b) Sketch the graph of f.
Solution
(a) To express this quadratic function in standard form, we complete the square.
(b) From the standard form we see that the graph is a parabola with vertex .
Vertex
Opens downward
f 1x2=-Ax -
1
2
B
2
+
9
4
A
1
2
,
9
4
B
A
1
2
,
9
4
B
=-Ax -
1
2
B
2
+
9
4
=-Ax
2
- x +
1
4
B+ 2 - 1- 12
#
1
4
=-1x
2
- x2+ 2
f 1x 2=-x
2
+ x + 2
f 1x2=-x
2
+ x + 2
5
perfect square
Given function
Factor from the x-terms
Complete the square: Add inside
parentheses, and subtract outside
Factor and simplify
A- 1
#
1
4
B
1
4
- 1
