Weingast B.R., Wittman D. The Oxford Handbook of Political Economy
Подождите немного. Документ загружается.


812 european integration
setting and pursuing personal agendas. This is an avenue through which the values
of the elite, which seem to have played an important role in the history of European
integration, can enter the process. Similarly, the increasingly prominent institutional
dimension of the integration process works to condition the effects of these interest
group politics and intergovernmental negotiations. These observations help to ex-
plain why the progress of European integration has taken its particular form, one
which has not always been fully anticipated by the member states.
In recent historical-institutionalist accounts, the point has been put more strongly:
institutions “as the carriers of history” (in the words of David 1994)aresaidtobe
responsible for the path-dependent character of European integration. But, as eval-
uated in this chapter, this effort to rehabilitate early interpretations of the dynamics
of European integration as a process characterized by path dependence, spillovers,
and positive feedbacks has not been entirely successful. The preconditions for the
existence of a non-ergodic process have not been identified satisfactorily. Testable
implications of this view, which can be used to distinguish it from the alternatives,
have not been adequately specified.
Much of the literature on the political economy of European integration seeks to
establish the incompatibility of these views instead of exploring how they fit together.
Methodologically, these different strands of work approach the problem in very
different ways—some rely on narrative, others on econometrics—which may help
to explain the inability of their advocates to appreciate one another’s contributions.
More effectively bringing together these different strands of work, analytically and
empirically, will help to push this research agenda forward.
References
Arthur,B.1988. Self-reinforcing mechanisms in economics. Pp. 9–32 in The Economy as an
Evolving Complex System, ed. P. W. Anderson, K. J. Arrow, and D. Pines. Reading, Mass.:
Addison Wesley.
1989. Competing technologies, increasing returns and lock-in by historical events.
Economic Journal, 99: 116–32.
Berger,H.,andRitschl,A.1995. Germany and the political economy of the Marshall Plan,
1947–52: a re-revisionist view. Pp. 199–245 in Europe’s Postwar Recovery, ed. B. Eichengreen.
Cambridge: Cambridge University Press.
David,P.A.1985. Clio and the economics of qwerty. American Economic Review: Papers and
Proceedings, 75: 332–7.
1994. Why are institutions the “carriers of history?” Path-dependence and the evolution
of conventions, organizations and institutions. Structural Change and Economic Dynamics,
5: 205–20.
Dehousse,R.1994. Community competences: are there limits to growth? Pp. 103–25 in Europe
after Maastricht: An Ever Closer Union, ed. R. Dehousse. Munich: Beck.
Frieden,J.A.2002. Real sources of european currency policy: sectoral interests and European
monetary integration. International Organization, 56: 831–60.

barry eichengreen 813
Gabel,M.2001. Divided opinion, common currency: the political economy of public sup-
port for EMU. Pp. 49–76 in The Political Economy of European Monetary Unification,ed.
B. Eichengreen and J. Frieden. Boulder, Colo.: Westview Press.
Garrett,G.1992. International cooperation and institutional choice: the European Commu-
nity’s internal market. International Organization, 46: 533–58.
2001. The politics of Maastricht. Pp. 111–30 in The Political Economy of European Monetary
Unification, ed. B. Eichengreen and J. Frieden. Boulder, Colo.: Westview Press.
Haas,E.1958. The Uniting of Europe. Stanford, Calif.: Stanford University Press.
Keohane,R.1984. After Hegemony. Princeton, NJ: Princeton University Press.
and Hoffmann,S.1991. Institutional change in Europe in the 1980s. Pp. 1–39 in The
New European Community: Decisionmaking and Institutional Change, ed. R. Keohane and
S. Hoffmann. Boulder, Colo.: Westview Press.
Krasner,S.D.1989. Sovereignty: an institutional perspective. Pp. 69–96 in The Elusive State:
International and Comparative Perspectives, ed. J. A. Caporaso. Newbury Park, Calif.: Sage.
Lange,P.1993. The Maastricht social protocol: why did they do it? Politics and Society, 21: 5–36.
Leibowitz,S.,andMargolis,S.1990. The fable of the keys. Journal of Law and Economics,
33: 1–26.
Majone,G.1992. Regulatory federalism in the European Community. Environment and Plan-
ning C: Government and Policy, 10: 299–316.
Martin,L.1993. International and domestic institutions in the EMU process. Economics and
Politics, 5: 125–44.
2001. International and domestic institutions in the EMU process and beyond.
Pp. 131–55 in The Political Economy of European Monetary Unification,ed.B.Eichengreen
and J. Frieden. Boulder, Colo.: Westview Press.
Moravcsik,A.1993. Preferences and power in the European Community: a liberal intergov-
ernmentalist approach. Journal of Common Market Studies, 31: 473–524.
1998. The Choice for Europe: Social Purpose and State Power from Messina to Maastricht.
Ithaca, NY: Cornell University Press.
Pierson,P.1996. The path to European integration: a historical institutionalist analysis.
Comparative Political Studies, 29: 123–63.
and Leibfried,S.1995. Multi-tiered institutions and the making of social policy.
Pp. 1–40 in European Social Policy, ed. S. Leibfried and P. Pierson. Washington, DC: Brook-
ings Institution.
Pollack,M.A.1997. Obedient servant or runaway Eurocracy? Delegation, agency and agenda
setting in the European Community. International Organization, 51: 99–134.
Rose,A.2000. One money, one market: estimating the effect of common currencies on trade.
Economic Policy, 15: 8–45.
Ross,G.1994. Jacques Delors and European Integration. Oxford: Polity Press.
Sandholtz,W.1993. Choosing union: monetary politics and Maastricht. International Orga-
nization, 47: 1–39.
chapter 45
..............................................................
TRADE,
IMMIGRATION,
AND
CROSS-BORDER
INVESTMENT
..............................................................
ronald rogowski
Political economists have understood since the famous Stolper–Samuelson essay of
1941 that increasing international trade—whether in products (e.g. grain, steel) or in
factors of production (labor, capital)—can have strong distributional consequences,
benefiting some groups domestically and internationally but harming others. By
now four distinct models in international political economy offer specific predictions
about the distributional consequences, between and among nations, of cross-border
exchange.
1
While virtually all models conclude that trade improves world welfare,
2
some allow that trade can diminish welfare in particular countries, chiefly the ad-
vanced industrial ones. One of the models predicts factoral within-country effects
¹ The between-nation impact is usually called a welfare effect, and trade in factors of production is
commonly labeled migration or foreign investment. I argue below that the full social and political impact
of lowered barriers to trade—what is commonly called “globalization”—can be understood only if we
look at these effects as a whole, and if we consider trade both in products and in factors.
I omit any discussion of the familiar Ricardo (or, as some would have it, Ricardo–Pangloss)
single-factor, single-good model, since it predicts no distributional consequences: every group in every
country gains from trade.
² I note one small, but potentially important, exception below.

ronald rogowski 815
(e.g. workers win, capital loses), the other three mainly sectoral effects (computers
win, textiles lose).
By now most political scientists, but not all, will be familiar with (if not totally
sympathetic to) the approach that this chapter embodies, namely deriving explicit
political implications from what were originally devised as economic models. In this
case, economic models yield definite predictions about who will win and who will
lose from increasing trade in goods or factors; the political side of the task is to
figure out, at a minimum, (a) the political pressures that are likely to arise from these
distributional consequences, and (b) the kinds of coalitions that form, or that can be
formed by smart political entrepreneurs, on behalf of particular trade-related policies.
In what follows, I will discuss the implications of the four models, the empirical
evidence for and against them, and what all this suggests for future research. I
will focus particularly on the anomalies—i.e. the seeming violations of theoretical
prediction—that have arisen in recent work, and on the efforts to modify theory in
response to those anomalies.
I shall label the four main models (1) Heckscher–Ohlin (HO),(2) specific factors,
including Samuelson–Jones (SJ) and Ricardo–Viner (RV),(3) neo-Ricardian,and(4)
Economies of Scale (EOS). As the name given to the last model implies, the first three
all assume constant returns to scale (doubling all inputs exactly doubles output). All
but the neo-Ricardian model normally assume, in addition, that the same production
technology is available in all countries, i.e. all countries have identical production
functions; but the models differ (obviously) in their assumption about whether
factors are mobile among sectors, an issue that also becomes important within the
neo-Ricardian model. All models make the conventional assumption of diminishing
marginal returns to any individual factor.
We consider these models in turn, focusing in each case first on trade in products
and then on cross-border migration and investment.
1 Heckscher–Ohlin
.............................................................................
(a) Trade in products. In the simplest two-factor, two-good variant of this workhorse
model, trade in goods and services benefits every country as a whole—to put it
concretely, trade raises GDP per capita. Hence, obviously, trade also improves world
welfare; but it harms, in every country, owners of locally scarce resources.
3
The
well-known Mayda–Rodrik paper (1999) accurately applies this model to a world
³ The classic example asks us to imagine Japan (densely populated) and Australia (thinly populated)
in autarky: in Japan, both land and grain (a land-intensive product) will be expensive, i.e. will trade for
many hours of labor, or of labor-intensive products (e.g. cloth). In Australia, land and grain will be
cheaper in isolation, i.e. will require fewer hours of labor, or of labor-intensive products, to buy. If trade
now opens between Japan and Australia, Japan will export steel and import grain; Australia will export
grain and import steel. Trade will harm Japanese landowners and benefit Japanese workers; but it will
benefit Australian landowners and harm Australian workers.
816 trade, immigration, and cross-border investment
in which the only factors are human capital and labor: in the developed countries,
abundant in human capital, trade would harm (and be opposed by) the unskilled; in
developing countries, abundant in unskilled labor, opposition to trade would come
from the owners of (scarce) human capital. Since the model assumes costless mobility
of factors among sectors (e.g. workers displaced in apparel or automobiles can with
no difficulty go into computers or financial services) sectoral employment does not
matter: in developed countries all unskilled, in developing ones all skilled, workers
are harmed. Again following the basic model, in developed economies globalization
would increase the returns to human capital, the incentives to acquire it, and the
extent of economic inequality; while in developing economies all of these would
diminish. Since in each country the gains to the abundant factor outweigh the losses
to the scarce one (otherwise trade could not be welfare improving for the country as a
whole), smart politicians and credible institutions can shape compensation schemes
that sustain free trade.
(b) Migration and investment. Imagine, however, a Heckscher–Ohlin world in
which no products, but only factors of production, can move. Locally abundant
factors will seek the higher returns of areas in which they are scarce; and migration
and investment, just like trade in products, will benefit locally abundant factors,
harm locally scarce ones, and improve world welfare. The classical illustration of
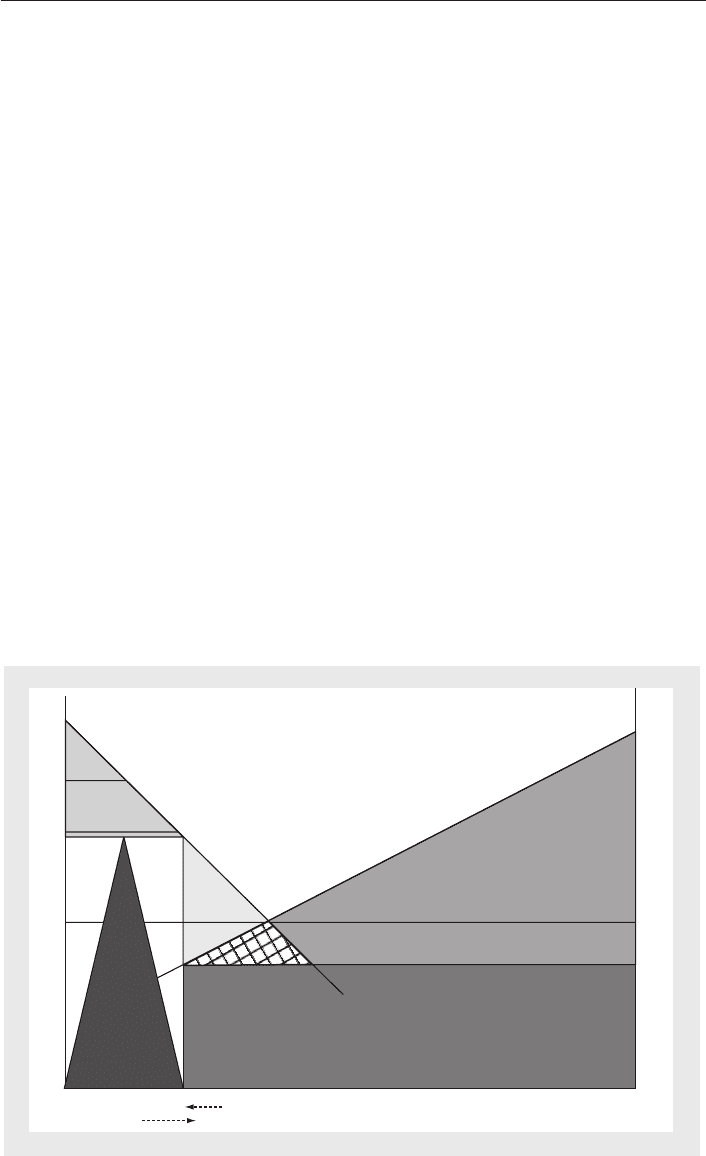
these points is the simple two-country, two-factor model presented in Figure 45.1.
We imagine that the “world” population is divided between countries A and B, with
everybody to the left of the vertical line residing in A, everybody to the right in
B. Pre-migration, the wage (equivalent, in any competitive market, to the marginal
productivity of labor, or MPL) in country A, w
A
,ishigherthanincountryB,w
B
.
per capita product before
migration (country A)
per capita product after
migration (country A)
B
w
B
A
w
A
w'
w'
population of
country A
population of country B
Fig. 45.1 Marginal productivity of labor in a two-country model of migration

ronald rogowski 817
Under the general principle of declining marginal productivity of each factor, MPL in
each country decreases as the country’s population increases. Again before migration,
the total product in country A is just the sum of all the marginal products, or the
total area under the MPL curve; labor in country A receives its wage times its total
labor, or the area of the darkly shaded left-side rectangle; owners of capital receive
the remainder, or the area of the lightly shaded left-side triangle. Similarly labor in
country B receives the area of the darkly shaded right-side rectangle, capital the area
of the medium-shaded right-hand triangle.
If labor can migrate freely between the two countries (and assuming that no capital
moves with it), labor will flow from B to A (shifting the vertical line of division
between the two countries to the right) until the wage is equalized in the two countries
at w
. The wage in country A will fall, that in country B will rise; country A capitalists
will benefit, country B capitalists will be harmed. But world production will increase,
since after migration world product will include the diagonally striped center triangle
that, before migration, was forgone. Hence migration, for the world as a whole, is un-
ambiguously welfare improving. Exactly the same analysis would hold for movements
of capital, save that ex ante capital would have the higher return in the less capital-
abundant (i.e. poorer) country and would migrate from rich countries to poor ones.
Less often noted, however, is that in advanced economies not just the wages of
the unskilled, but per capita income, can fall as a result of migration and foreign
investment; that is, the losses to the scarce factor can actually outweigh the gains to
the abundant one(s). Again, this can readily be seen in the simple terms of Figure 45.1.
Recall that total product in each country is the area under the MPL (marginal product
of labor) line: the MPL tells us how much additional product we get from each new
unit of labor, and the sum of all those marginal products is the total product.
4
Then
per capita total product in each country is just that area under the MPL curve divided
by the population segment (represented, remember, by the x-axis). This is the same
thing as the average height of the trapezoid composed of (for our high-wage country
in Figure 45.1) the medium-shaded triangle and the dark-shaded rectangle on the left-
hand side. As population moves from country B to country A, per capita product—
the average height of this trapezoid—must fall in A and rise in B. The point can be
generalized: in the simplest Cobb–Douglas production function, with only capital
and labor as inputs, per capita output y(= Y/L) is always increasing in the capital–
labor ratio k(= K /L ).
5
As trade in factors opens, capital-abundant countries export
capital and import labor, decreasing k. Since, in the model, the marginal gain in
productivity in the capital-scarce countries outweighs the marginal loss in the capital-
abundant ones, world welfare improves;
6
but, in theory, developed-country welfare
can easily decline.
⁴ Similarly, if we graphed each student’s weight against her or his height, we would have a “marginal”
curve; if we take the sum of all those weights—i.e. the area under the weight–height curve, or the
cumulative distribution—we will know the total poundage of all students below a specific height.
⁵ In the standard Cobb–Douglas notation, Y = AK
·
L
1−·
; · O (0, 1); hence, where y = Y/L and
k = K /L, y = Ak
·
. Whatever decreases the capital–labor ratio must decrease per capita output.
⁶ In the notation used immediately above, dy/dk =
A·
k
1−·
; i.e. the marginal per capita gain in output
from any given increase in the capital–labor ratio will be higher, the lower is the initial capital–labor
ratio.

818 trade, immigration, and cross-border investment
Add to this what is suggested by the impressive historical work of O’Rourke and
Williamson (2001), namely that migration has equalized factor prices more quickly
and thoroughly than either investment or trade, and we perhaps begin to see why: (a)
developed-country politicians have found it far harder to construct pro-immigration
than pro-free trade coalitions; and (b) only highly undemocratic regimes (think the
Berlin Wall) have been able to restrict emigration.
But the pattern oftrade,whetherinproductsorfactors,predictedbytheHOtheory
has increasingly seemed at odds with reality. This is the first major anomaly we must
face. Even in the 1980s, HO would have predicted capital to have earned about 60
times as much in India as in the USA, making it something of a marvel that, even
allowing for a lot of political risk, capital did not flood from the rich countries to
the poor ones (Lucas 1990). Today, HO predicts with equal clarity that skilled labor
should be migrating in droves from the rich countries (where skilled workers are
abundant) to the poor ones (where they are scarce: cf. Davis and Weinstein 2005,
discussed at greater length below).
That important issue to one side, the HO model predicts the same factoral coali-
tionsonmigrationandinvestmentasitdoesontrade:indevelopedcountries,allun-
skilled workers should oppose immigration and outward foreign investment; in poor
countries, all skilled workers and capitalists should oppose emigration and inward
foreign investment. As a first approximation of the alignments that have emerged on
the issue of the new, and now seemingly stillborn, European Constitution, this does
not do badly.
7
2 Specific Factors
.............................................................................
As was emphasized above, the Heckscher–Ohlin model, like Ricardo’s classical pic-
ture, assumes that factors of production (labor, capital, land) can redeploy costlessly
between sectors. Workers, or machinery or land, that are displaced by import compe-
tition move frictionlessly into exporting sectors. What happens if, somewhat more
realistically, we replace this assumption of “mobile factors” with one in which at
least some factors are “specific,” i.e. can be used only in a particular sector, or can
be adapted to others only at considerable cost?
(a) Trade in products.TheRicardo–Viner (RV) specific-factors model is straightfor-
ward, indeed obvious: factors specific to import-competing sectors will embrace pro-
tection, those specific to exporting sectors will favor free trade. Thus, while American
capitalists generally should favor free trade (because capital is abundant in the USA),
owners of textile factories, swamped by Third-World imports and unable to convert
their factories to other uses, will (and do) advocate protection. A more interesting
⁷ In Paris, wealthy districts voted overwhelmingly yes, poor ones no. In France as a whole, farmers,
blue-collar workers, public sector employees, and the unemployed all voted no by more than 60%. A
major issue was the likelihood of increasing immigration from the new member countries. See the New
York Times, 30 May 2005.

ronald rogowski 819
prediction of this model, emphasized for example in Hiscox (2002), is that, as factors
become more specific—i.e. less able to move between sectors—politics will divide less
along factoral lines (workers vs. capitalists) and more along sectoral ones (textiles vs.
computers).
In sharp contrast, however, the Samuelson–Jones (SJ) specific-factors model, which
is the more widely accepted modern treatment,
8
emphasizes the ambiguous distri-
butional consequences for the mobile factor (depending, in essence, on its own-
ers’ consumption preferences). In the SJ model, effects on specific factors, but not
necessarily mobile ones, continue to be governed by conventional factor-abundance
considerations.
Imagine for example an advanced economy with abundant physical capital, above-
average human capital, but scarce unskilled labor, producing two kinds of goods: high
tech and low tech. The physical capital can be used only in the high-tech sector, the
unskilled labor only in low-tech production; skilled labor is used in both sectors and
is fully mobile between them. Then freeing up trade will benefit owners of physical
capital and harm unskilled workers; but it will have ambiguous effects on skilled
workers (i.e. owners of human capital), depending chiefly on their relative propensity
to consume high-tech products (which will rise in price as trade increases) vs. low-
tech ones (which will become cheaper). Certainly one possible political scenario, in
such an SJ world, is a protectionist coalition of unskilled and (at least some) skilled
workers; and the current outsourcing anxieties of developed-country skilled workers,
seemingly one of the advanced economies’ most mobile groups, make this version of
the specific-factors model seem quite relevant.
More generally, where the RV model predicts that increasing specificity of factors
will lead to sectoral coalitions, the SJ one predicts increasingly anomalous factoral
coalitions, e.g. the possibility that skilled labor in a developed economy, despite being
abundant, may wind up advocating protection—or, equally, that scarce skilled labor
in a developing economy could favor free trade. Exactly as in the HO model, however,
trade improves all countries’ (and hence world) welfare.
As an aside, it seems that human capital is inherently more specific than physical
capital. Smart owners of physical capital can nowadays diversify their portfolios with
a few mouse-clicks, but investment in human capital is arduous, focused, and long
term, be it the learning of a specific skill or the attainment of a university degree.
Moreover, Estevez-Abe, Iversen, and Soskice (2001) have emphasized that decisions
about specificity of human capital are to a large extent endogenous, conditioned on
subsidies to training and social insurance mechanisms: an eighteen-year-old, forced
to choose between a general and easily redeployable form of human capital (e.g. a
university education) and a much more specific one (e.g. mastering the production
processes of a particular firm), is likelier to choose the latter if she knows that
generous unemployment and retraining benefits will kick in if the firm or sector
subsequently fails.
⁸ See Samuelson 1971;Jones1971; or any conventional textbook treatment, e.g. Krugman and Obstfeld
1994,ch.3.

820 t rade, immigration, and cross-border investment
(b) Migration and investment. Here, too, the SJ and the RV variants of specific
factors come to interestingly opposed conclusions. In SJ, group preferences regarding
immigration and foreign investment will remain exactly as in HO: scarce factors op-
posed, abundant ones in favor, whether they are mobile or specific. Coalitions on this
issue will remain wholly factoral and wholly predicted by relative factor abundance.
Under the RV model, however, preferences on immigration will be uniform (and
pro-immigration) in exporting sectors, but divided along factoral linesinimport-
competing sectors; or, to put it another way, all owners of abundant factors will favor
immigration, while some owners of scarce (but export-specific) factors will also be
pro-immigrant.
For simplicity, suppose that a developed-country economy consists of only two
sectors: textiles, heavily pressured by import competition, and software, characterized
by booming exports. Each is produced by only two factors, each specific to its sector:
textile-specific capital and labor; software-specific capital and labor. Assuming that
immigrants can no more easily master the skills of the software sector than can do-
mestic labor, software workers, despite the relative scarcity of labor in their economy
overall, will favor immigration as much as they do free trade: if anything immigration
will drive down the costs of their textiles.
9
By the same token, textile workers will
hate immigration even more than they do free trade. But textile owners, pressured
already by low-wage competition from abroad, will favor immigration as a way of
lowering their costs. Hence in the RV picture of the world, we should expect to see:
(a) some groups where preferences over trade and immigration diverge (e.g. favoring
both protection and immigration, or free trade and restricted immigration); and (b)
uniformly sectoral coalitions on trade, but some factoral ones on migration.
In the specific-factors model, as in the HO one, trade in factors improves world and
poor-country welfare; it may, however, not raise per capita real income in developed
countries.
3 The Neo-Ricardian Model
.............................................................................
In an important recent paper, Davis and Weinstein (2005) stress the peculiar impli-
cations of the HO model noted earlier in this chapter. Since the return to each factor
must equal its marginal product (e.g. the wage is just the marginal product of labor),
and since the marginal product should always diminish with relative abundance
of that factor, returns to factors should always be lower where the factor is most
abundant.
10
Thus skilled labor, clearly abundant in the USA, should earn a higher
⁹ If, that is, we consider only the factor-returns story. In a recent paper, Hanson, Scheve, and
Slaughter (2005) emphasize that, at least in tolerably generous welfare states, the post-redistribution
implications of migration may depart considerably from those of free trade.
¹⁰ In the simple two-factor Cobb–Douglas production function discussed earlier (n. 5),
Y = AK
·
L
1−·
implies that the wage will equal the marginal productivity of labor, i.e.
w = ∂Y/∂ L = A(1 − ·)k
·
, where (as before) k = K /L. Hence, assuming identical production

ronald rogowski 821
real wage where skills are scarce (e.g. India); and American skilled workers should
be emigrating to India. And the fact of skilled migration into the USA and other
advanced economies is a second major anomaly that we must address.
In fact, Davis and Weinstein (DW) argue persuasively, the USA may well offer
higher returns than the rest of the world to virtually all factors, including many
that it holds abundantly; and they suggest that the only explanation must lie in
US technological and legal-system superiority. In short, they advocate (as, to be sure,
have many students before them) abandonment of the traditional HO assumption
of identical production functions in all countries and an admission that total factor
productivity, the A term in most production functions (e.g. Cobb–Douglas: above,
n. 5), is simply higher in advanced economies.
As Davis and Weinstein note, however, the distributional, welfare, and pattern of
trade implications of assuming between-nation differences in technology are often at
least as strange as those of the HO model, and sometimes are downright alarming.
Trade in products continues to be welfare improving for all countries, just as in the
original Ricardo model (although of course, exactly as in that model, factor prices
no longer equalize between countries); but migration, while it improves world and
sending-country welfare, may (and, in their empirical estimation, does) harm all
sectors in the receiving country, chiefly by eroding the more productive country’s
terms of trade advantage.
(a) Trade in products. Since in the DW model there is only one factor, labor, which
can move costlessly among sectors, distributional effects within countries disappear:
everybody will favor free trade. If (as DW do not do), one assumed instead that labor
was differentiated and specific to sectors, the neo-Ricardian model predicts an ordinal
relationship: the more productive the given sector, the likelier it would be to benefit
from, and to favor, free trade. This is not an altogether empty prediction, but it is
less informative than we might wish, both because the source of the productivity
advantage remains a black box, and because productivity must to some extent be
endogenous.
(b) Migration and investment.
11
Here the DW model has its real bite. Because
trade can no longer equalize wages between countries, migration will continue (if
allowed) even under costless trade in products, until wages equalize; and production
will then locate according to absolute, not comparative, advantage. Migration is
unambiguously welfare lowering for the more productive country, but welfare im-
proving for the less productive one and for the world as a whole. If labor is freely
mobile between sectors, everybody in the advanced country will oppose immigration
(even as all favored free trade); to the extent that labor is immobile among sectors,
presumably immigration will be most opposed by those sectors in which the country’s
functions across countries, the wage will (in isolation) be higher where labor is scarcer. The same will
hold true for any factor: the relatively scarcer (more abundant) it is, the higher (lower) will be its return
in the given economy.
¹¹ In the strict DW model, there is no capital, hence no cross-border investment; but obviously if K
were the only factor instead of L, the same logic would apply: all owners of capital (which is to say,
everybody) within the advanced economy would oppose cross-border investment.
