Boudet R. Quantum Mechanics in the Geometry of Space-Time: Elementary Theory
Подождите немного. Документ загружается.

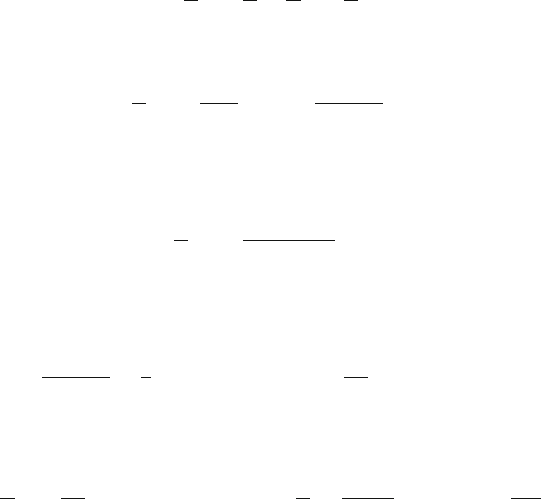
128 19 About the Quantum Fields Theory
The only explanation of the presence of c in the right hand part of Eq. 19.3 is
the following.
This presence corresponds to the construction.
1
R
=
π
2
×
1
R
×
2
π
(19.5)
then
π
2
=
∞
0
sin x
x
dx =
∞
0
sin(k
R)
k
dk
Denoting k
= k/c
π
2
=
∞
0
sin(kR/c)
k
dk
(19.6)
and using
sin(k
R)
k
R
=
1
2
π
0
e
ik
R cos θ
sin θdθ =
1
4π
π
0
2π
e
i(k
.R)
d
one deduces
1
R
=
⎡
⎣
1
4π
∞
0
π
0
2π
0
e
i(k
.R)
dk
d
⎤
⎦
×
2
π
=
1
2π
2
c
e
i((k.R)/c)
d
3
k
k
2
that is the formula Eq. 19.3.
One sees on Eqs. 19.5, 19.6 that the Planck constant is introduced inside π/2
(not inside 2/π ), an indisputable nonsense!
Nevertheless the use of such a device does not alter the validity of the calculation
of the Lamb shift which remains one of the most admirable work in the theory of the
electron.
References
1. W. Heitler, The Quantum Theory of Radiation (Clarendon Press, Oxford, 1964)
2. D. Hestenes, J. Math. Phys. 8, 798 (1967)
3. J. Seke, Mod. Phys. Lett. B 7, 1287 (1993)
4. G. Jakobi, G. Lochak, C.R. Ac. Sc. (Paris) 243, 234 (1956)
5. R. Boudet, New Frontiers in Quantum Electrodynamics and Quantum, ed. by A. O. Barut
(Plenum, New York, 1990), p. 443
6. M. Kroll, W. Lamb, Phys. Rev. 75, 388 (1949)
Index
B
Biquaternion, 10, 11
Bivector spin, 25
Bosons
A, 25
W, B, Z, 65
G, 75
C
Charge current, 86
Clifford algebras, 7
Contributions
charged, 69
neutral, 69
Coulomb laws, 89
D
Darwin solutions, 83
Dirac
matrices, 111
spinor, 15
Dirac equation
complex language, 29
real language, 29
Doublets, 54
E
Einstein formula, 39
Electric field, 90
Electromagnetism
Principles, 84
Potential, 84
Electron, 29
Electron charge, 29
Energy-momentum tensor
electron, 31
Yang-Mills, 48
F
Free Dirac electron, 37
G
Gauge U(1)
complex language, 21
real language, 23
Gauge U(1) invariance
complex language, 21
real language, 23
Gauge U(1) theories
electron, 23
electroweaks, 71
Gauge SU(2)
complex language, 45
real language, 47
Gauge SU(2) invariance
complex language, 46
real language, 47
Gauge SU(2) theories
electroweaks, 64
Yang–Mills, 48
Gauge SU(2)XU(1), 53, 70
Gauge SU(3), 77
Gell-Mann matrices,
75
Glashow, Weinberg,
Salam theory, 61
Gluon, 79
Grassmann algebra, 7
129
H
Hestenes
group, 15
spinor, 14
I
Idempotents, 54
Incident wave, 97
Inner products, 8
Isospin matrices, 46
Isotropic vectors, 54
L
Lagrangian
Electron, 33
Yang-Mills field, 65
Lamb shift, 83, 101
Lorentz
force, 40, 89
retarded potential, 87
Louis de Broglie
clock, 38
frequency, 39
M
Magnetic field, 90
Momentum-energy tensors, 115
Moving frame , 16, 115
P
Plane wave, 96
Pauli
Matrices, 111
spinor, 111
Positron, 36
Poynting vector, 93
Probability current, 14
Q
Quaternion, 10, 113
T
Tetrode
tensor, 31
theorem, 33, 123
Total angular momentum, 31
Transition current, 95
W
Weinberg angle, 65
Y
Yang–Mills theory, 45
Yvon–Takabayasi angle, 14
Z
Zitterbewegung, 71
130 Index
