Everitt B.S. The Cambridge Dictionary of Statistics
Подождите немного. Документ загружается.


The estimator does not have finite expectation since x can take the value zero. A modified
version, Chapman’s estimator, adds one to the frequency of animals caught in both samples
(x) with the resulting population size estimator
^
N ¼
y þ1
x þ1
ðX þ 1Þ1
[KA1 Chapter 10.]
CAR: Abbreviation for coarsening at random.
Cardano , Gi ro lamo ( 1501^1 576): Born in Milan, Italy Cardano studied medicine in Padua and
for a time practised as a country doctor. But he was better known as a gambler particularly
with dice. His writings about gambling with dice contain the first expression of the
mathematical concept of probability and Cardano clearly states that the six sides of an
honest die are equally likely and introduces chance as the ratio of the number of favourable
cases to the number of equally possible cases. Girolamo Cardano, physician, mathematician
and eccentric died insane at the age of 75.
Cardiord distribution: A probability distribution, f (θ), for a
circular random variable
, θ, given by
f ðÞ¼
1
2p
f1 þ2 cosð Þg; 0 2p; 0
1
2
[Statistical Analysis of Circular Data, 1995, N. I. Fisher, Cambridge University Press,
Cambridge.]
Carryover effects: See crossover design.
CART: Abbreviation for classification and regression tree technique.
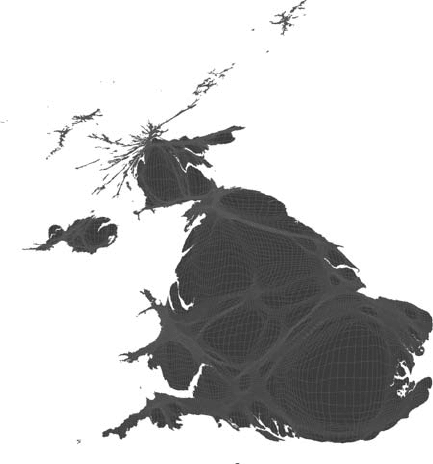
Cartogram: A diagram in which descriptive statistical information is displayed on a geographical
map by means of shading, different symbols or in some other perhaps more subtle way. An
example of the latter is given in Fig. 26. See also disease mapping.[Statistics in Medicine,
1988, 7, 491–506.]
Cascadedparameters: A group of parameters that are interlinked and where selecting a value for
the first parameter affects the choice available in the subsequent parameters. This approach
brings important advantages to parameter estimation in the presence of
nuisance parameters
.
[Computational Statistics, 2007, 22, 335– 351.]
Case-cohort study: A research design in epidemiology that involves sampling of controls at the
outset of the study who are to be compared with cases from the cohort. The design is
generally used when the cohort can be followed for disease outcome, but it is too expensive
to collect and process information on all study subjects. See also case-control study and
nested case-control study.[Environmental Health Perspectives, 1994, 102,53–56.]
Case-control study: A traditional case-control study is a common research design in
epidemiology
where the exposures to risk factors for cases (individuals getting a disease) are compared to
exposures for controls (individuals not getting a disease). The design is retrospective in the
sense that a sample of controls is sampled from subjects who are disease free at the end of the
observation period. The primary advantage of this design is that exposure information needs to
be gathered for only a small proportion of cohort members, thereby considerably reducing the
data collection costs. A disadvantage is the potential for
recall bias
. See also retrospective
design, nested case-control study and case-cohort study. [Case-Control Studies: Design,
Conduct, Analysis, 1982, J.J. Schlesselman, Oxford University Press, Oxford.]
69
Case series: A series of reports on the condition of individual patients made by the treating physician.
Such reports may be helpful and informative for rare diseases, for example, in the early days
of the occurrence of AIDS, but it has to be remembered that in essence they are nothing more
than anecdotal evidence dignified by the case series label. [Clinical Trials in Psychiatry,2
nd
edition, 2008, B. S. Everitt and S.Wessely, Wiley, Chichester.]
Catast r ophe theo ry: A theory of how small, continuous changes in independent variables can
have sudden, discontinuous effects on dependent variables. Examples include the sudden
collapse of a bridge under slowly mounting pressure, and the freezing of water when
temperature is gradually decreased. Developed and popularized in the 1970s, catastrophe
theory has, after a period of criticism, now become well established in physics, chemistry
and biology. [ Physica Series A, 2000, 285, 199–210.]
Categorical variable: A variable that gives the appropriate label of an observation after allocation to
one of several possible categories, for example, respiratory status: terrible, poor, fair, good,
excellent, or blood group: A, B, AB or O. Respiratory status is an example of an ordered
categorical variable or
ordinal variable
whereas blood type is an example of an unordered
categorical variable. See also binary variable and continuous variable.
Catego r izi ng continous vari ab l es: A practice that involves the conversion of continuous
variables into a series of categories, that is common in medical research. The rationale is
partly statistical (avoidance of certain assumptions about the nature of the data) and partly
that clinicians are often happier when categorizing individuals. In general there are no
statistical advantages in such a procedure. [ British Journal of Cancer, 1991, 64, 975.]
Cauchy, Augusti n-Louis (1789^1 857): Born in Paris, Cauchy studied to become an Engineer but
ill health forced him to retire and teach mathematics at the École Polytechnique. Founder of the
theory of functions of a complex variable, Cauchy also made considerable contributions to the
theory of estimation and the classical linear model. He died on 22 May 1857 in Sceaux, France.
Cauchy distribution: The probability distribution, f (x), given by
Fig. 26 A cartogram
showing population density in
the UK (used with permission
of Dr. B. Hennig).
70
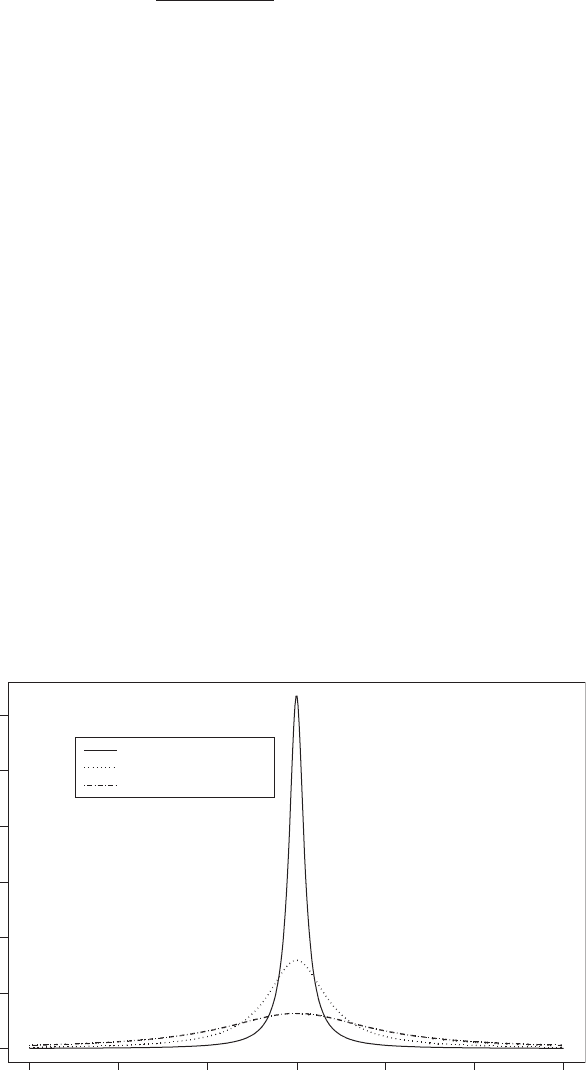
f ðxÞ¼
β
p½β
2
þðx αÞ
2
1x 1; β
4
0
where α is a
location parameter
(median) and beta β a
scale parameter
.
Moments
and
cumulants
of the distribution do not exist. The distribution is unimodal and symmetric
about α with much heavier tails than the normal distribution (see Fig. 27). The upper and
lower quartiles of the distribution are α ± β. Named after
Augustin-Louis Cauchy
(1789–
1857). [STD Chapter 7.]
Cau c hy int eg r al: The integral of a function, f (x), from a to b defined in terms of the sum
S
n
¼ f ðaÞðx
1
aÞþf ðx
1
Þðx
2
x
1
Þþþf ðx
n
Þðb x
n
Þ
where the range of the integral (a, b) is divided at points x
1
; x
2
; ...; x
n
. It may be shown that
under certain conditions (such as the continuity of f (x) in the range) the sum tends to a limit
as the length of the intervals tends to zero, independently of where the dividing points are
drawn or the way in which the tendency to zero proceeds. This limit is the required integral.
See also Riemann–Stieltjes integral. [KA1 Chapter 1.]
Cauchy^Schwarz inequality: The following inequality for the integrals of functions, f (x), and
g(x), whose squares are integral
Z
½f ðxÞgðxÞdx
2
Z
½f ðxÞ
2
dx
Z
½gðxÞ
2
dx
In statistics this leads to the following inequality for expected values of two random
variables x and y with finite second moments
½EðxyÞ
2
Eðx
2
ÞEðy
2
Þ
The result can be used to show that the correlation coefficient, ρ, satisfies the inequality
2
1. [KA1 Chapter 2.]
x
f(x)
–15 –10
–5
0 5 10 15
0.0 0.1 0.2 0.3 0.4 0.5 0.6
alpha = 0, beta = 0.5
alpha = 0, beta = 2
alpha= 0, beta = 5
Fig. 27 Cauchy distributions for various parameter values.
71
Causal i ty: The relating of causes to the effects they produce. Many investigations in medicine seek to
establish causal links between events, for example, that receiving treatment A causes
patients to live longer than taking treatment B. In general the strongest claims to have
established causality come from data collected in experimental studies. Relationships
established in
observational studies
may be very suggestive of a causal link but are always
open to alternative explanations. [SMR Chapter 5.]
Causespecific deathrate: A death rate calculated for people dying from a particular disease. For
example, the following are the rates per 1000 people for three disease classes for developed
and developing countries in 1985.
C1 C2 C3
Developed 0.5 4.5 2.0
Developing 4.5 1.5 0.6
C1 = Infectious and parasitic diseases
C2 = Circulatory diseases
C3 = Cancer
See also crude annual death rate and age-specific death rate.
CC F: Abbreviation for common cause failure.
Ceiling effect: A term used to describe what happens when many subjects in a study have scores on a
variable that are at or near the possible upper limit (‘ceiling’). Such an effect may cause
problems for some types of analysis because it reduces the possible amount of variation in
the variable. The converse, or floor effect, causes similar problems. [Arthritis and
Rheumatism, 1995, 38, 1055.]
Cellular proliferation models: Models used to describe the growth of cell populations. One
example is the
deterministic model
NðtÞ¼N ðt
0
Þe
υðtt
0
Þ
where N(t) is the number of cells in the population at time t, t
0
is an initial time and υ
represents the difference between a constant birth rate and a constant death rate. Often also
viewed as a
stochastic process
in which N(t) is considered to be a random variable.
[Investigative Ophthalmology and Visual Science, 1986, 27, 1085–94.]
Censored observations: An observation x
i
on some variable of interest is said to be censored if it
is known only that x
i
L
i
(left-censored)orx
i
U
i
(right-censored) where L
i
and U
i
are
fixed values. Such observations arise most frequently in studies where the main response
variable is time until a particular event occurs (for example, time to death) when at the
completion of the study, the event of interest has not happened to a number of subjects. See
also interval censored data, singly censored data, doubly censored data and non-
informative censoring. [SMR Chapter 2.]
Censored regression models: A general class of models for analysing truncated and censored
data where the range of the dependent variable is constrained. A typical case of censoring
occurs when the dependent variable has a number of its values concentrated at a limiting
value, say zero. [Statistics in Medicine, 1984, 3, 143–52.]
Census: A study that aims to observe every member of a population. The fundamental purpose of the
population census is to provide the facts essential to government policy-making, planning
and administration. [SMP Chapter 5.]
72
Cen tering: A procedure used in an effort to avoid
multicollinearity
problems when both linear,
and higher order terms are included in a model. In
multilevel models
covariates are often
cluster-mean centred to facilitate interpretation of corresponding regression parameters.
[Applied Longitudinal Analysis, 2004, G. M Fitzmaurice, N. M. Laird and J. H. Ware,
Wiley, New York.]
Cen tile: Synonym for percentile.
Centilereferencecharts: Charts used in medicine to observe clinical measurements on individual
patients in the context of population values. If the population centile corresponding to the
subject’s value is atypical (i.e. far from the 50% value) this may indicate an underlying
pathological condition. The chart can also provide a background with which to compare the
measurement as it changes over time. An example is given in Fig. 28.[Statistics in Medicine,
1996, 15, 2657–68.]
Centralized database: A
database
held and maintained in a central location, particularly in a
multicentre study
.
Central limit theorem: If a random variable Y has population mean µ and population variance σ
2
,
then the sample mean,
y, based on n observations, has an approximate normal distribution
with mean µ and variance σ
2
/n, for sufficiently large n. The theorem occupies an important
place in statistical theory. [KA1 Chapter 8.]
Cen tral range: The range within which the central 90% of values of a set of observations lie. [SMR
Chapter 3.]
Centraltendency: A property of the distribution of a variable usually measured by statistics such as
the mean, median and mode.
Centro id meth od: A method of
factor analysis
widely used before the advent of high-speed
computers but now only of historical interest. [Psychological Review, 1931, 38, 406–27.]
CEP: Abbreviation for circular error probable.
CER: Abbreviation for cost-effectiveness ratio.
CERES plot: Abbreviation for combining conditional expectations and residuals plot.
CFA: Abbreviation for confirmatory factor analysis.
CHAID: Abbreviation for chi-squared automated interaction detector.
Chain-binomial models: Models arising in the mathematical theory of infectious diseases, that
postulate that at any stage in an
epidemic
there are a certain number of infected and
susceptibles, and that it is reasonable to suppose that the latter will yield a fresh crop of
cases at the next stage, the number of new cases having a
binomial distribution
. This results
in a ‘chain’ of binomial distributions, the actual probability of a new infection at any stage
depending on the numbers of infectives and susceptibles at the previous stage. [Stochastic
Analysis and Applications, 1995, 13, 355–60.]
Chaini ng: A phenomenon often encountered in the application of
single linkage clustering
which
relates to the tendency of the method to incorporate intermediate points between distinct
clusters into an existing cluster rather than initiate a new one. [MV2 Chapter 10.]
Chain-of-events data: Data on a succession of events that can only occur in a prescribed order.
One goal in the analysis of this type of data is to determine the distribution of times between
successive events. [Biometrics, 1999, 55, 179–87.]
73

Weight
Age in weeks/ months
BOYS WEIGHT (kg)
0–1 year
Weight (kg)
Some degree of weight
loss is common after birth.
Calculating the percentage
weight loss is a useful way
to identify babies who
need extra support.
14
345 6 78 9
10 11
99.6th
98th
91st
75th
50th
25th
9th
2nd
0.4th
16 18 20 22 24 26 28 30 32 34 36 38 40 42 44 46 48 50 52
13.5
13.5
13
12.5
12
11.5
11
10.5
10
9.5
9
8.5
8
7.5
7
6.5
6
5.5
5
99.6th
99.6th
98th
98th
91st
91st
75th
75th
50th
50th
25th
25th
9th
9th
2nd
2nd
0.4th
0.4th
4.5
4
3.5
3
2.5
2
1.5
1
0.5
13
12.5
12
11.5
11
10.5
10
9.5
9
8.5
8
7.5
7
6.5
6
5.5
5
4.5
4
3.5
3
2.5
2
1.5
1
0.5
525048464442403836343230282624
22
201816141210864
1
2
3
4
5
6
7
8
9
10
11
20
Age in weeks/ months
Fig. 28 Centile chart of birthweight for gestational age.
Chains of infection: A description of the course of an infection among a set of individuals. The
susceptibles infected by direct contact with the introductory cases are said to make up the
first generation of cases; the susceptibles infected by direct contact with the first generation
are said to make up the second generation and so on. The enumeration of the number of cases
in each generation is called an epidemic chain. Thus the sequence 1–2–1–0 denotes a chain
consisting of one introductory case, two first generation cases, one second generation case
and no cases in later generations. [Occupational and Environmental Medicine, 2004, 61,
96–102.]
Ch a l mers,Tho mas Cl a rk ( 1917^1995) : Born in Forest Hills, New York, Chalmers graduated
from Columbia University College of Physicians and Surgeons in 1943. After entering
private practice he became concerned over the lack of knowledge on the efficacy of accepted
medical therapies, and eventually became a leading advocate for
clinical trials
, and later for
meta-analysis
setting up a meta-analysis consultancy company at the age of 75. In a
distinguished research and teaching career, Chalmers was President and Dean of the
Mount Sinai Medical Center and School of Medicine in New York City from 1973 to
1983. He died on 27 December 1995, in Hanover, New Hampshire.
Champernowne, David Gawen (1912^2000): Born in Oxford, Champernowne studied
mathematics at King’s College, Cambridge, later switching to economics, and gaining first
class honours in both. Before World War II he worked at the London School of Economics
and then at Cambridge where he demonstrated that the evolution of an income and wealth
distribution could be represented by a Markovian model of income mobility. During the war
he worked at the Ministry of Aircraft Production, and at the end of the war became Director
of the Oxford Institute of Statistics. In 1948 he was made Professor of Statistics at Oxford,
and carried out work on the application of
Bayesian analysis
to
autoregressive series
.In
1958 Champernowne moved to Cambridge and continued research into the theory of capital
and the measurement of economic inequality. He died on 22 August 2000.
Chance events: According to Cicero these are events that occurred or will occur in ways that are
uncertain-events that may happen, may not happen, or may happen in some other way.
Cicero’s characterization of such events is close to one dictionary definition of chance
namely, ‘the incalculable element in existence that renders events unpredictable’. But for
Leucippus (circa 450 B.C.) the operation of chance was associated with some hidden cause
and such a view was held widely in the middle ages where chance had no place in the
universe, rather all events were though of as predetermined by God or by extrinsic causes
determined by God. But in the 20
th
century the deterministic view of the universe was
overthrown by the successful development of quantum mechanics with its central tenet
being that it is impossible to predict exactly the outcome of an atomic (or molecular) system
and where this uncertainty is not due to measurement error or experimental clumsiness but is
a fundamental aspect of the basic physical laws themselves. [Chance Rules, 2nd edition,
2008, B. S. Everitt, Springer, New York.]
Change point problems: Problems with chronologically ordered data collected over a period of
time during which there is known (or suspected) to have been a change in the underlying
data generation process. Interest then lies in, retrospectively, making inferences about the
time or position in the sequence that the change occurred. A famous example is the
Lindisfarne scribes data in which a count is made of the occurrences of a particular type
of pronoun ending observed in 13 chronologically ordered medieval manuscripts believed to
be the work of more than one author. A plot of the data (see Fig. 29) shows strong evidence
of a change point. A simple example of a possible model for such a problem is the following;
75

y
i
¼ α
1
þ β
1
x
i
þ e
i
; i ¼ 1; ...; τ
y
i
¼ α
2
þ β
2
x
i
þ e
i
; i ¼ τ þ 1; ...; T
Interest would centre on estimating the parameters in the model particularly the change
point, τ.[Statistics in Medicine, 1983, 2, 141–6.]
Change scores: The change in an outcome of interest from pre-treatment to post-treatment. Often
used as a response variable in
longitudinal studies
. See Lord’s paradox.[Sociological
Methodology, 1990, 20,93–114.]
Chaos: Apparently random behaviour exhibited by a
deterministic model
.[Chaos, 1987, J. Gleick,
Sphere Books Ltd, London.]
Chapman ^ Kol mogoroff equation: See Markov chain.
Chapman’s estimator: See capture–recapture sampling.
Characteristic function: A function, (t), derived from a probability distribution, f (x), as
ðtÞ¼
Z
1
1
e
itx
f ðxÞdx
where i
2
= −1 and t is real. The function is of great theoretical importance and under certain
general conditions determines and is completely determined by the probability distribution.
If (t) is expanded in powers of t then the rth central
moment
,
0
r
, is equal to the coefficient of
ðitÞ
r
=r! in the expansion. Thus the characteristic function is also a
moment generating
function
. [KA1 Chapter 4.]
Characteristic root: Synonym for eigenvalue.
Characteristic vector: Synonym for eigenvector.
Occurrences of pronoun
60
50
4030
20
10
231456897101211 13
Manuscript Number
Fig. 29 A plot of the Lindisfarne scribes data indicating a clear change point.
76

Chebyshev ^ Hermite polynomial: A function, H
r
(x)defined by the identity
ðDÞ
r
αðxÞ¼H
r
ðxÞαðxÞ
where αðxÞ¼ð1=
ffiffiffiffiffiffi
2p
p
Þe
1
2
x
2
and D = d/dx. It is easy to show that H
r
(x) is the coefficient of
t
r
/r! in the expansion of expðtx
1
2
t
2
Þ and is given explicitly by
H
r
ðxÞ¼x
r
r
½2
2:1!
x
r2
þ
r
½4
2
2
:2!
x
r4
r
½6
2
3
:3!
x
r6
þ
where r
½i
¼ rðr 1Þ...ðr i þ 1Þ. For example, H
6
(x)is
H
6
ðxÞ¼x
6
15x
4
þ 45x
2
15
The polynomials have an important orthogonal property namely that
Z
1
1
H
m
ðxÞH
n
ðxÞαðxÞdx ¼ 0; m 6¼ n
¼ n!; m ¼ n
Used in the
Gram–Charlier Type A series
. [KA1 Chapter 6.]
Chebyshev , P afnuty Lvovich (1 821^1 894): Born in Okatovo, Russia, Chebyshev studied at
Moscow University. He became professor at St Petersburg in 1860, where he founded the
Petersburg mathematical school which influenced Russian mathematics for the rest of the
century. Made important contributions to the theory of the distribution of prime numbers, but
most remembered for his work in probability theory where he proved a number of funda-
mental limit theorems. Chebyshev died on 8 December 1894 in St Petersburg, Russia.
Chebyshev’sinequality: A statement about the proportion of observations that fall within some
number of standard deviations of the mean for any probability distribution. One version is
that for a random variable, X
Prob k
x
k
hi
1
1
k
2
where k is the number of standard deviations, σ, from the mean, µ. For example, the
inequality states that at least 75% of the observations fall within two standard deviations
of the mean. If the variable X can take on only positive values then the following, known as
the
Markov inequality
, holds;
Prob½X x1
x
[KA1 Chapter 3.]
Chemometrics: The application of mathematical and statistical methods to problems in Chemistry.
[Chemometrics: A Textbook, 1988, D. L. Massart, B. G. M. Vandeginste, S. N. Deming,
Y. Michotte and L. Kaufman, Elsevier, Amsterdam.]
Chernoff’s faces: A technique for representing
multivariate data
graphically. Each observation is
represented by a computer-generated face, the features of which are controlled by an
observation’s variable values. The collection of faces representing the set of observations
may be useful in identifying groups of similar individuals,
outliers
, etc. See Fig. 30. See also
Andrews’ plots and glyphs. [MV1 Chapter 3.]
77

Chi nese restaurant process (C R P): A discrete-time
stochastic process
that at time n produces
a partition of the integers f1; 2; ...; ngand can be described in terms of a scheme by which n
customers sit down in a Chinese restaurant with an infinite number of tables. (The terminol-
ogy is inspired by the Chinese restaurants in San Francisco which appear to have an infinite
seating capacity.) Customers walk in and sit down at some table, with the tables being
chosen as follows;
1. The first customer always chooses the first table.
2. The mth customer chooses either the first unoccupied table with probability
γ
m 1 þ γ
or occupied table i with probability
m
i
m 1 þ γ
where m
i
is the number of customers
already seated at table i and γ is a parameter of the process.
After n customers have sat down the seating plan gives a partition of the integers f1; 2; ...; ng.
As an example consider the following possible arrangement of 10 customers;
Table 1: (1,3,8), Table 2: (2,5,9,10), Table 3: (4,6,7)
The probability of this arrangement is given by;
1
γ
1 þγ
1
2 þγ
γ
3 þγ
1
4 þ γ
1
5 þγ
2
6 þγ
2
7 þ γ
2
8 þγ
3
9 þγ
The CRP has been used to represent uncertainty in the number of components in a
finite
mixture distribution
. The distribution induced by a
Dirichlet process
can be generated
incrementally using the Chinese restaurant process. [Statistica Sinica, 2003, 13, 1211–
1235.]
1
2
3
4
5
6
7
8
9
10
Fig. 30 Chernoff’s faces
representing ten multivariate
observations.
78
