Kopnin N.B. Theory of Nonequilibrium Superconductivity

Подождите немного. Документ загружается.

end p.130

Top

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第7页共7页 2010-8-8 15:35

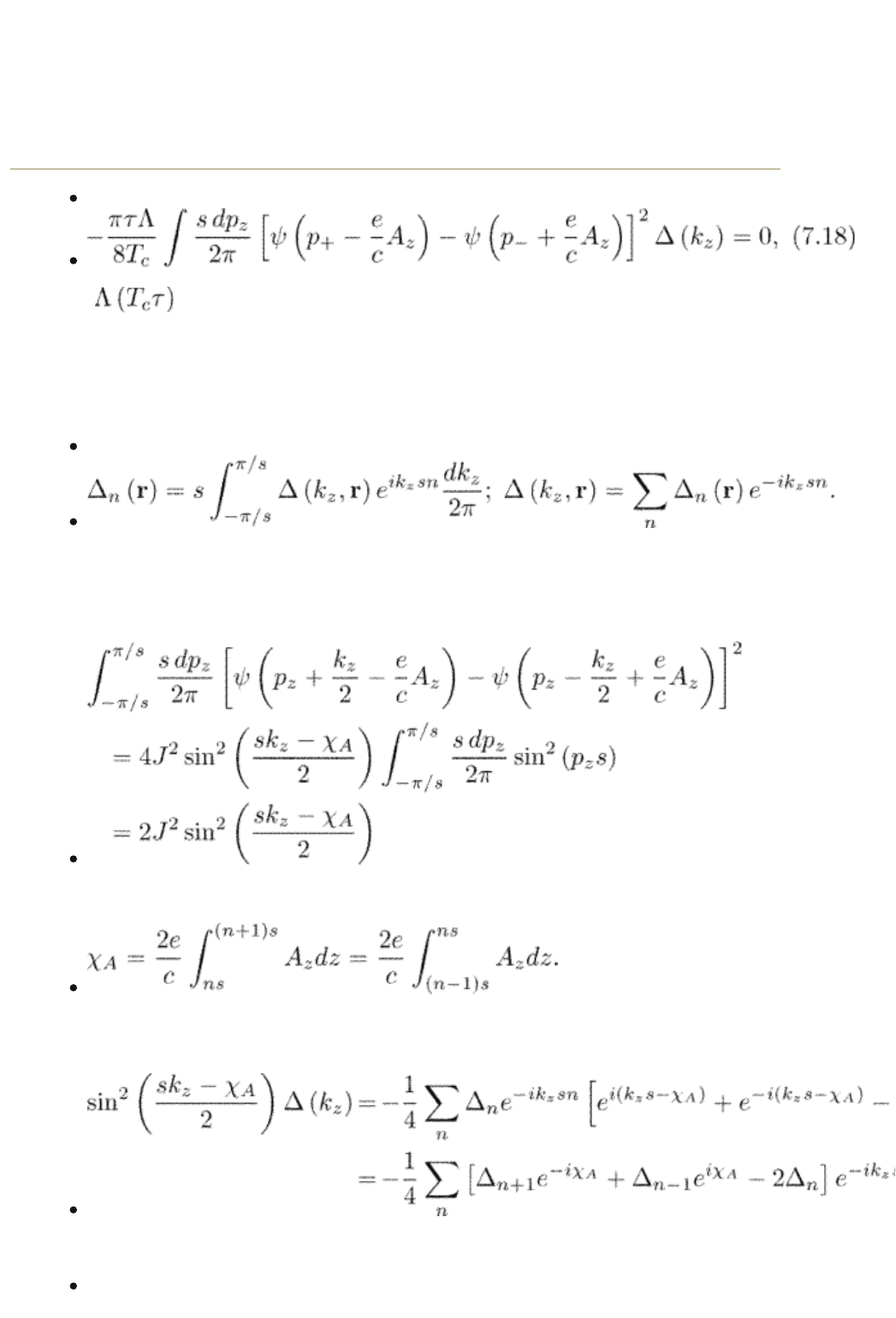
(7.18)

where is defined by eqn (6.22). The first line is a Fourier transform at

the momentum k

In the z-coordinate representation, the order parameter is determined only on

the conducting layers. We specify the layers with the number n such that z

ns. We have

(7.19)

Equations (7.18) and (7.19) completely determine the order parameter in a

layered superconductor. However, eqn (7.18) can be simplified further using eqn

(7.2). We have

where

We use the fact that the vector potential varies slowly at distances of the order of

the interlayer distance. One has

Equation (7.18) in the coordinate representation becomes

(7.20)

Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of

Technology, and L.D. Landau Institute for Theoretical Physics, Moscow

Theory of Nonequilibrium Superconductivity

Print ISBN 9780198507888, 2001

pp. [131]-[135]

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第1页共6页 2010-8-8 15:37

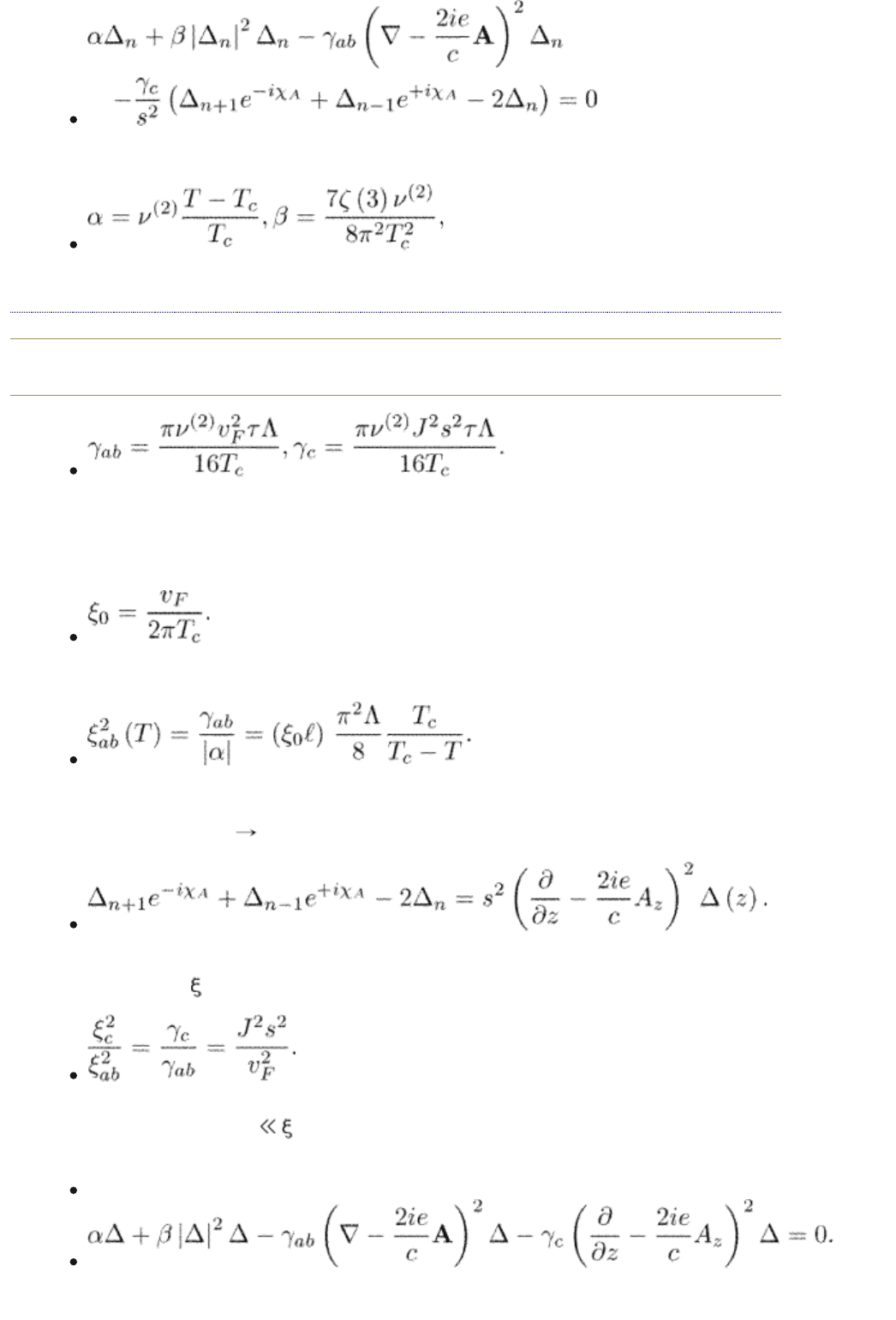
where

end p.131

Equation (7.20) is the Lawrence–Doniach equation, for layered superconductors.

It has been derived microscopically by Klemm et al. (1975).

As in the three-dimensional case, we put

The temperature-dependent coherence length in the ab plane is

The last line of eqn (7.20) is the discrete analogue of the second derivative. In

the continuous limit, s

0, we can write

Comparing this with the first term in the square brackets, we can define the

coherence length

through the ratio

In the continuous limit, s

(7.20) transforms into the Ginzburg–Landau

equation for anisotropic uniaxial superconductor

(7.21)

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第2页共6页 2010-8-8 15:37

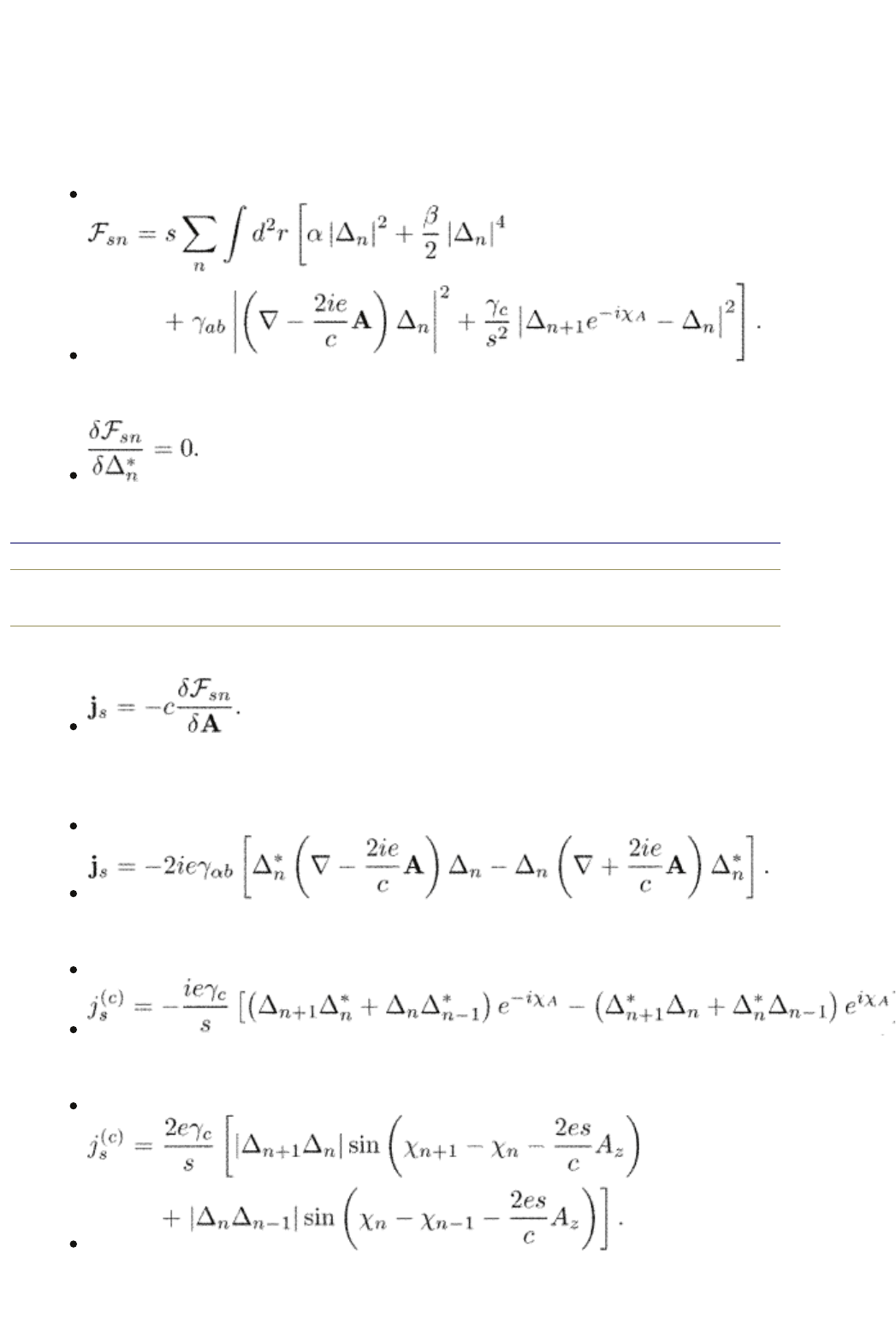
It has exactly the same form as eqn (6.68) for an anisotropic d-wave

superconductor.

7.3.2 Free energy and the supercurrent

Equations (7.13) and (7.16) allow us to reconstruct the Lawrence–Doniach free

energy. Using eqn (3.71) or (5.30) with the definition eqn (7.7) we find

(7.22)

Equation (7.20) is reproduced when one calculates the variation

end p.132

The supercurrent can be found using the definition

The supercurrent density in the ab direction at the position z

= ns of the n-th

layer has the usual form

(7.23)

The current density in the c direction at the n-th layer becomes

(7.24)

(7.25)

This expression corresponds to the Josephson current which is produced by

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第3页共6页 2010-8-8 15:37

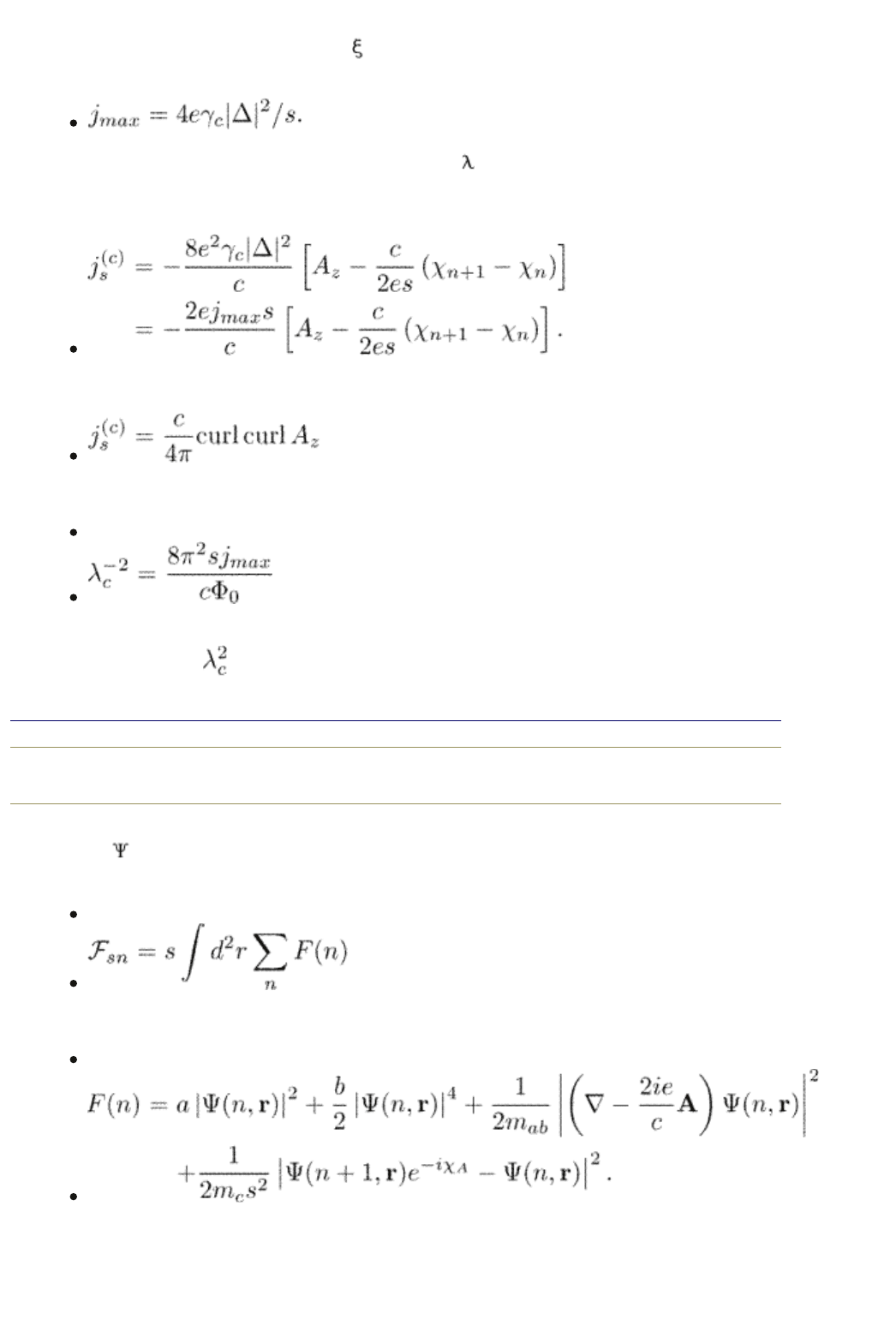
Cooper pair tunneling between the layers.

For a highly layered case, when s ~

, the maximum Josephson current in eqn

(7.25) is

It enters the definition of the penetration length

for currents flowing in the c

direction. Indeed, for small fields, one can expand eqn (7.25) in a small

argument to get

Together with the Maxwell equation

this determines

(7.26)

which is called the Josephson length. If the Josephson coupling is small the

penetration length

diverges.

end p.133

When the order parameter is normalized in such a way that it is the wave

function

of superconducting electrons, the Lawrence–Doniach free energy

equation (7.22) becomes

(7.27)

where the energy density at a layer n is

(7.28)

This is similar to eqn (1.27). The constants a and b satisfy

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第4页共6页 2010-8-8 15:37

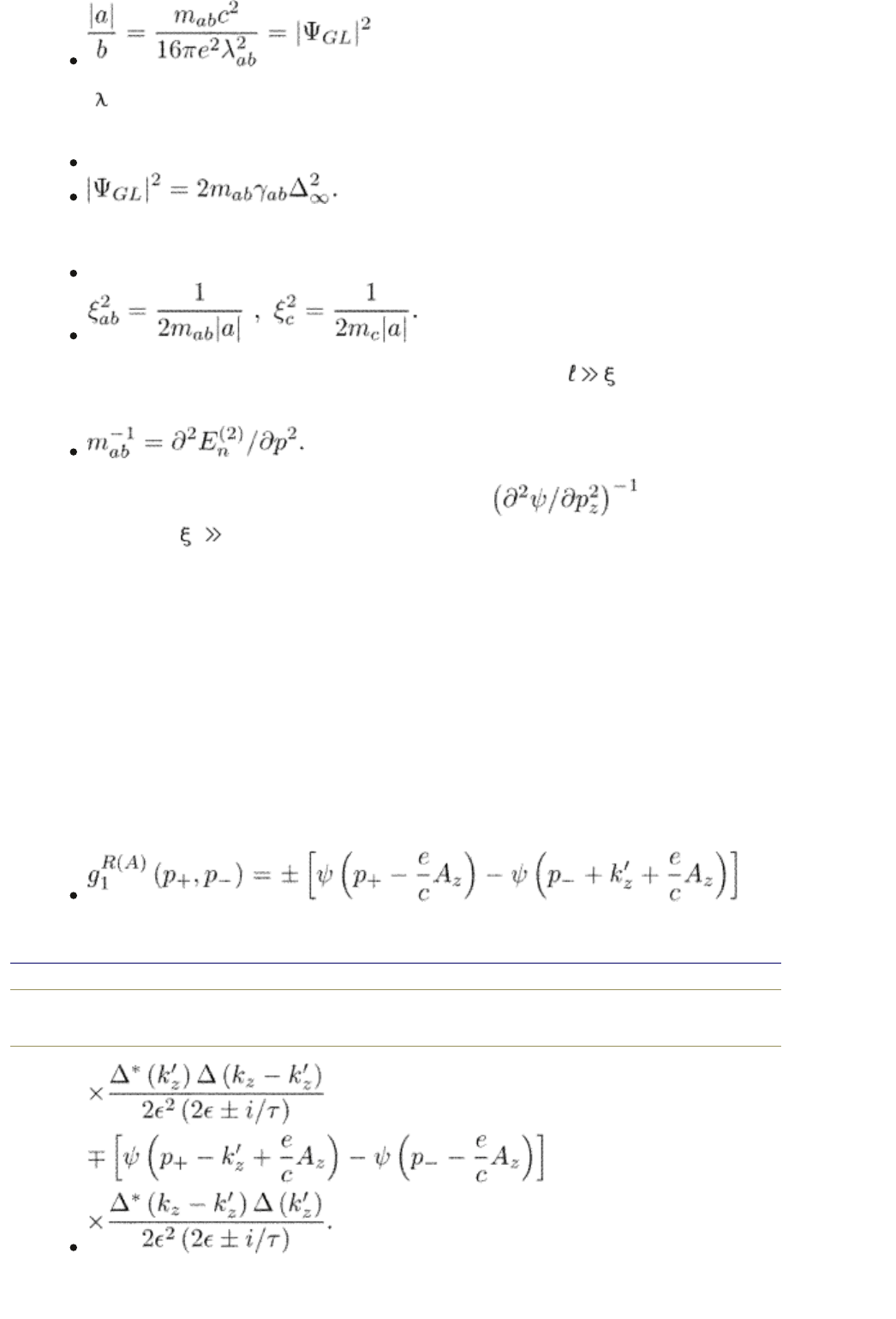
where

is the London penetration length for currents flowing in the ab plane.

The order parameter magnitude is

(7.29)

The coherence lengths are expressed through the “effective masses”

(7.30)

The effective mass in the ab plane for clean superconductors

has the

usual meaning

The “effective mass” m

, however, can be written as only in the

continuous limit

The Lawrence–Doniach equations can also be derived using the model by Graf al.

(1993) mentioned in the beginning of this section. In this case, the effective

mass m

is expressed through the interlayer diffusion.

7.3.3 Microscopic derivation of the supercurrent

The expression for the supercurrent can also be obtained microscopically using

our results for the Green functions eqn (7.13). The current along the ab plane

has a standard form and can be obtained exactly in the same way as it was done

for the usual Ginzburg–Landau equation in Section 6.1.2. The current along c

direction can be calculated from the corresponding terms in the Green function,

namely,

end p.134

We now insert this expression into the definition of current, eqn (7.6), and make

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第5页共6页 2010-8-8 15:37

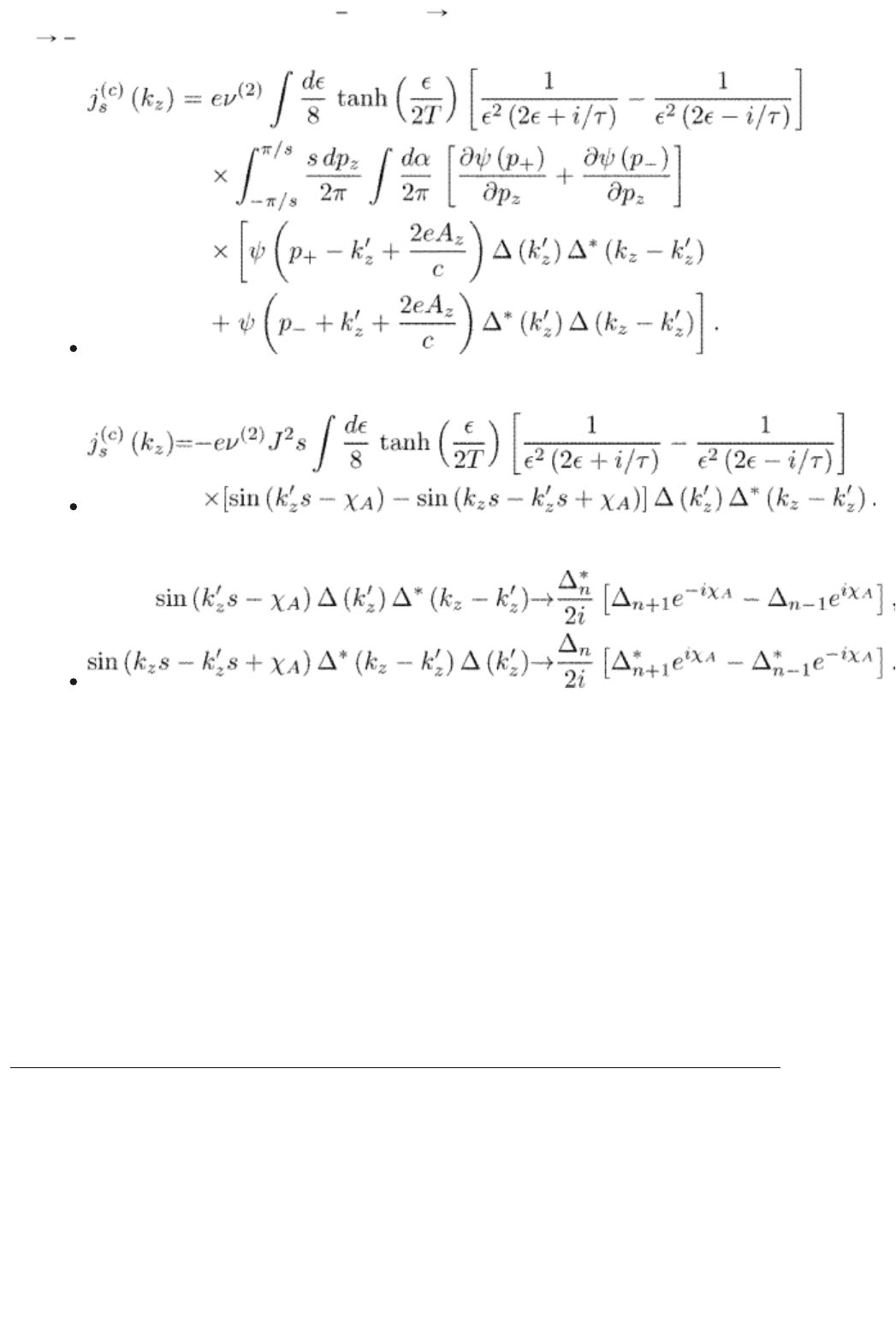
the shift of integration variable p

(e/c) A

. The terms which are odd in p

drop out after integration over dp

and we get

Using eqn (7.2) we obtain

In the Fourier representation of eqn (7.19),

Finally, we return to eqn (7.24).

7.4 Applications of the Lawrence–Doniach model

The Lawrence–Doniach model is widely used for layered superconductors; it is

simple and quite general at the same time. It covers the whole class of

anisotropic systems from three-dimensional weakly anisotropic to highly

anisotropic layered and even two-dimensional superconductors. It is able to

provide a reasonable description for many properties of the mixed state of

layered superconductors. For example, layered superconductors can have

two-dimensional analogues of the usual Abrikosov vortices, the so-called pancake

vortices connected with each other by strings of Josephson vortices. Pancake

vortices have been first considered by Artemenko and Kruglov (1990) and by

Clem (1991). Pancakes turned out

end p.135

Top

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第6页共6页 2010-8-8 15:37

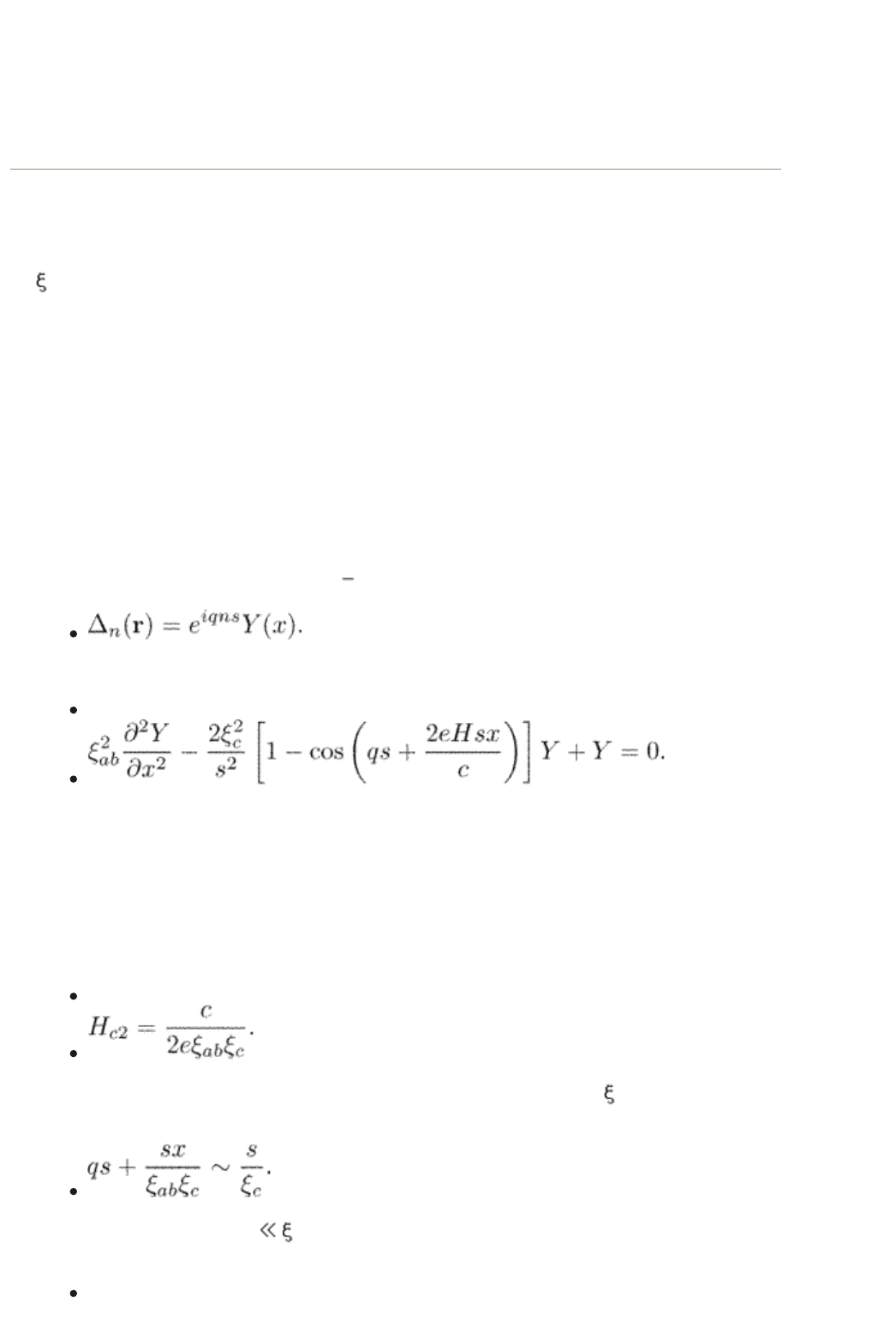
to be very important for high temperature superconductors most of which are

highly anisotropic or layered compounds. Another interesting feature of layered

superconductors is the unusual behavior of the upper critical magnetic field: For

orientation parallel to the layers, it grows rapidly as temperature decreases and

(T) approaches the interlayer distance s. Yet another important property is the

so-called intrinsic pinning considered first by Tachiki and Takahashi (1989) and

elaborated further by Ivlev and Kopnin (1989) and by Barone et al. (1990). Many

problems specific for layered superconductors arc discussed in the review by

Blatter et al. (1994). Here we briefly outline two mentioned examples: the upper

critical field and intrinsic pinning which will be used later when we consider the

vortex dynamics in anisotropic and layered superconductors.

7.4.1 Upper critical field

Let the magnetic field be parallel to the layers. As in Section 1.1.2 we look for a

second-order phase transition from the normal into the superconducting state

which occurs with lowering the field. We choose the y-axis along the direction of

the field with the gauge A = (0. 0,

Hx) and assume a solution in the form

The linearized equation (7.20) for Y (x) becomes the Mathieu equation:

(7.31)

There are two limiting cases: (i) Weakly layered (nearly continuous) limit, and

(ii) highly layered case. Consider them separately.

7.4.1.1 Weakly layered case

In the continuous anisotropic case the upper critical field is known to be (Kats

1969, 1970, Lawrence and Doniach 1971):

(7.32)

This equation can be reproduced also from eqn (7.31). Since x ~

we find that

the argument of the cosine function in eqn (7.31) is

In the continuous limit, s

, the cosine function in eqn (7.31) can be

expanded in a small argument. We obtain

(7.33)

Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of

Technology, and L.D. Landau Institute for Theoretical Physics, Moscow

Theory of Nonequilibrium Superconductivity

Print ISBN 9780198507888, 2001

pp. [136]-[140]

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第1页共6页 2010-8-8 15:39

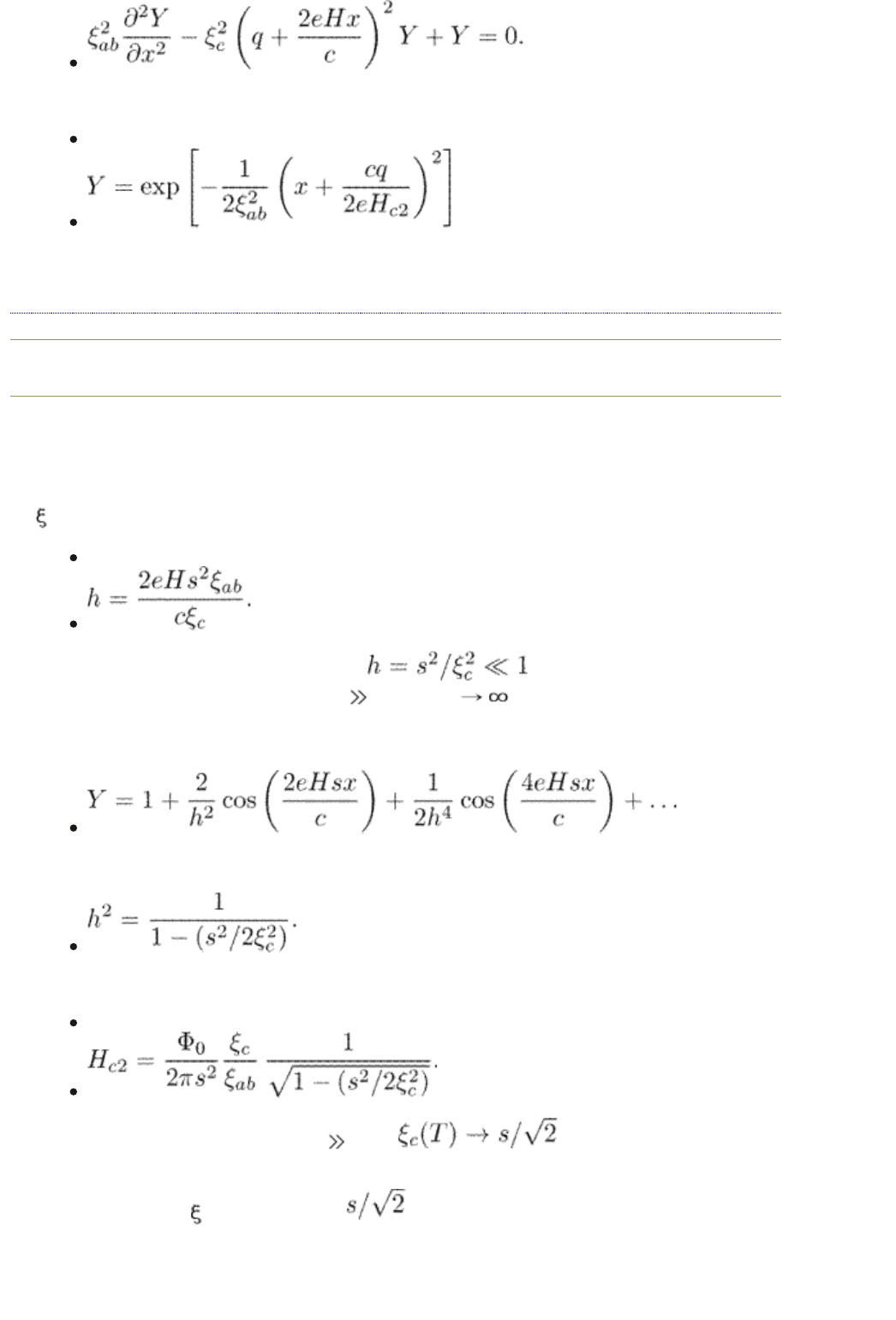
This is the oscillator equation (1.33). Again, the lowest-level solution is

(7.34)

where H

is determined by eqn (7.32).

end p.136

7.4.1.2 Highly layered case

As we shall see, this limit corresponds to a high upper critical field and is realized

for a certain relation between the interlayer distance s and the coherence length

We introduce the parameter

(7.35)

For a weakly layered superconductor, . On the contrary, a

highly layered case is realized for h

1 when H . For this limit, a solution

of eqn (7.31) can be obtained as an expansion in powers of a small 1/h. The

lowest-level periodic solution corresponds to an even Mathieu function

provided

This relation determines the upper critical field

(7.36)

This solution is valid as long as h 1, or .

We observe that the upper critical field diverges as the temperature-dependent

coherence length

c(T) approaches from above with lowering the

temperature (Klemm et al. 1975). In this limit, vortex cores fit in-between the

superconducting layers, and the supercurrents do not destroy superconductivity.

Divergence of the upper critical field is similar to the Little and Parks effect for a

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第2页共6页 2010-8-8 15:39

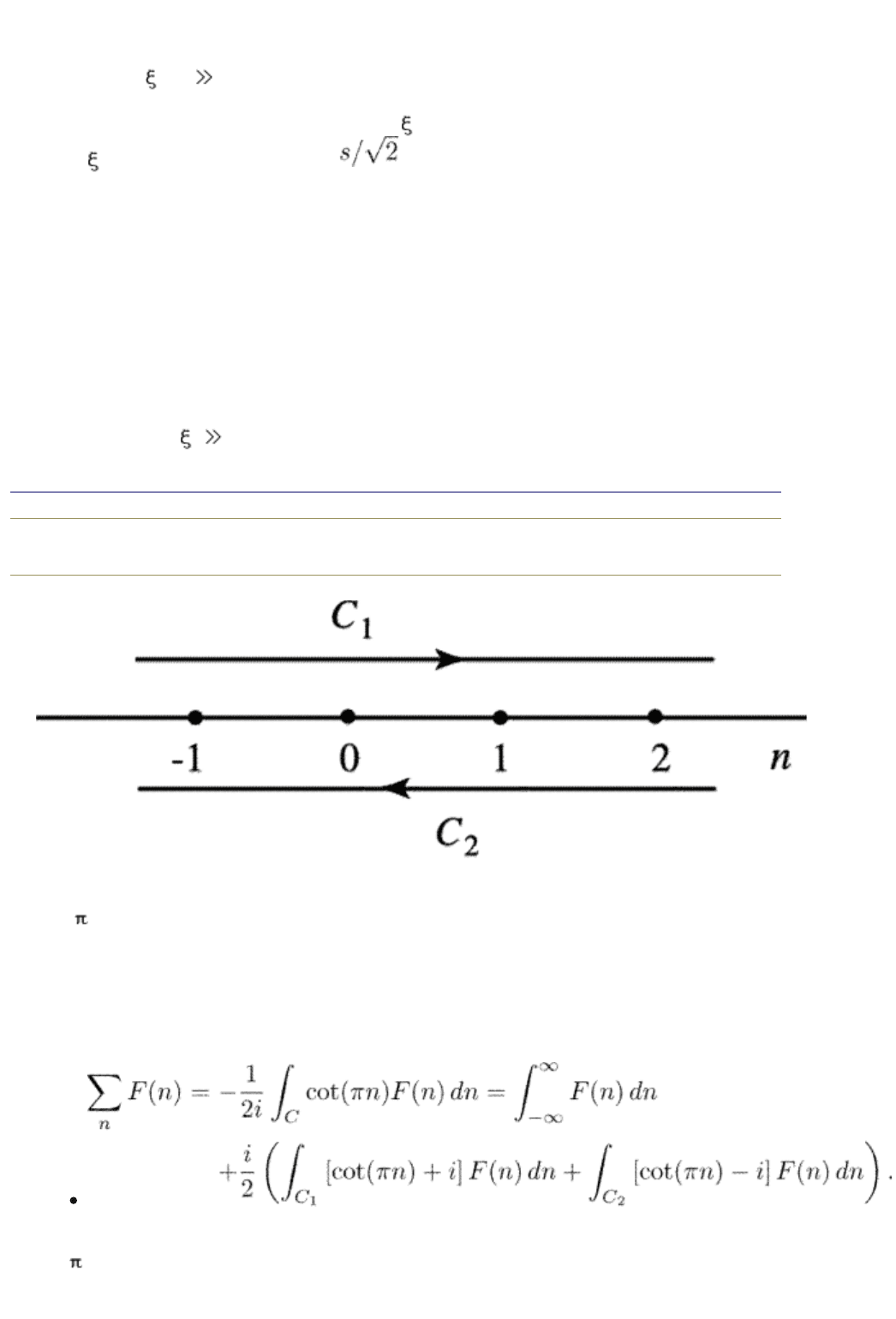
non-singly-connected superconductor where the critical temperature is a periodic

function of the magnetic field instead of vanishing with an Increasing field.

Therefore, an anisotropic superconductor that is essentially a three-dimensional

system with

(T) s at a higher temperature, can undergo a transition into

effectively a two-dimensional superconductor with a lowering of the temperature

if the zero-temperature coherence length

(0) is short enough,. This occurs

when

(T) decreases and reaches .

7.4.2 Intrinsic pinning: Single vortex in a weak potential

In this section we consider an important effect specific for layered systems,

namely, an interact ion of the order parameter with the underlying crystalline

structure. More particularly, we discuss an example of vortices which are aligned

parallel to the superconducting planes. The interaction with the inhomogeneous

layered environment gives rise to the “intrinsic pinning”: The vortex energy

becomes dependent on the vortex position with respect to the planes and

produces a pinning force which tries to keep vortices in between the

superconducting layers (Tachiki and Takahashi 1989). We consider a nearly

continuous limit

end p.137

FIG. 7.1. Integration, contours in the summation formula. The poles of

cot(

n) are shown by dots.

Assume that the magnetic field is parallel to the layers and find the energy of a

single vortex in a layered structure. We use the approach developed by Barone

et al. (1990). To calculate the sum in eqn (7.27) we employ the summation

formula

The integration contour C goes above and, below the poles n = 0, ±1, ... of

cot(

n) encircling them clockwise, while the contours C

and C

go above and

below the poles, respectively, so that C = C

+ C

(see Fig. 7.1). The total free

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第3页共6页 2010-8-8 15:39