R?ssler U. Solid State Theory: An Introduction

Подождите немного. Документ загружается.

320 10 Light–Matter Interaction

the Bloch equations

(k,t)=−

(k,t)

− ν

(k,t)

(k,t)=−

(k,t)

+ ν

(k,t)+ω

(k,t)

(k,t)=−

(k,t)+1

− ω

(k,t) . (10.108)

The two time constants can be distinguished by their geometrical relation to

the components of the Bloch vector: the longitudinal relaxation time T

(for

) is related with the diagonal elements of the density matrix, while the

transverse relaxation time T

(for the components U

and U

) refers to its oﬀ-

diagonal elements. Their physical content discloses by looking at the meaning

of the components of the Bloch vector: U

describes the po pu lation inversion

of the two-level system, which decays with T

,thepopulation lifetime, while

1,2

, describing the phase-coherent polarization of the two-level system, decay

with the phase relaxation, dephasing or decoherence time T

. Dephasing takes

place not only by scattering processes with phonons, lattice defects, and o t her

electronic excitations, but also by electron–hole recombination. If, besides

the latter, which is the only process contributing to T

, no oth er processes

contribute to T

,thenT

=2T

, because the number of electron–hole pairs is

proportional to P

∼ exp(−2t/T

). Including all other processes leads to the

relation T

≤ 2T

10.7 Semiconductor Bloch Equations

In order to include the particle interaction H

Coul

it is convenient to intro-

duce the electron–hole picture by replacing the Fermion operators according

to c

→ α

and c

→ β

†

−k

. With these modiﬁcations, making use of

(k)=E

(−k) and replacing under the sum k by −k, the terms of the

system Hamiltonian can be written as





(k)α

†

+ E

(k)β

†



(10.109)

Coul



k,k

′

;q=0



†

k+q

†

′

−q

′

+ β

k−q

′

†

′

†

+2α

†

k+q

′

†

′



(10.110)

= −E(t)





†

−k

+ h.c.



. (10.111)

10.7 Semiconductor Bloch Equations 321

Bringing the hole operator terms into normal order one ﬁnds with



k,k

′

;q=0

k−q

′

†

′

†



k,k

′

,q=0

†

k+q

†

′

−q

′



q=0



†

−



k;q=0

(10.112)

and single-particle energies E

(k)=E

(k) for electrons an d E

(k)=

−E

(k)+



q=0

for holes

+ H

Coul





(k)α

†

+ E

(k)β

†

−



q=0





k,k

′

;q=0



†

k+q

†

′

−q

′

+ β

†

k+q

†

′

−q

′

−2α

†

k+q

†

′

−q

′



. (10.113)

In the ﬁrst part of this section, we have investigated the dynamics of

the two-level system with an electron in the conduction band and a hole in

the valence band by taking into account only the single-particle terms in the

equation-of-motion of the density matrix. Now, we also consider the contribu-

tion of the particle interactions H

′

Coul

represented by the four-operator terms

in (

10.113). This is done in three steps. The ﬁrst step is the calculation of the

commutators b etween H

′

Coul

and the operators α

†

,β

†

,andα

†

−k

(Prob-

lem 10.8). In the second step, we replace the operator terms by their thermal

expectation values. Thetwo-operatortermsα

†

 = f

and β

†

 = f

have the obvious meaning of electron and hole populations at wave vector

k, while α

†

−k

 = P

∗

is the macroscopic polarization due to the applied

external ﬁeld. With the results of Problem 10.8, the equations of motion of

these quantities take the following form [

284]:

∂

∂t

∗

(t)=i



(k)+E

(k)



¯h

∗

(t)+id

∗

(t)



(t)+f

(t) − 1





′

;q=0



α

†

k−q

†

′

†

−k

−α

†

′

†

−k−q



+ α

†

−k−q

†

′

−α

†

k+q

†

′

−q

′

†

−k





, (10.114)

∂

∂t

(t)=−2Im



E(t)P

∗

(t)





′

;q=0



α

†

′

−q

k−q

′

−α

†

k+q

†

′

−q

′



+ α

†

k−q

†

′

−q

′

−α

†

k+q

†

′

−q

′





, (10.115)

322 10 Light–Matter Interaction

∂

∂t

(t)=−2Im



E(t)P

∗

(t)





′

;q=0



β

†

−k

†

′

−q

−k−q

′

−β

†

−k+q

†

′

−q

−k

′



+ α

†

′

†

′

†

−k

−k+q

−α

†

′

†

−k−q

−k





. (10.116)

In the last step we factorize the expectation values of the four-operator terms

into expectation values of two-operator terms, following the concept of the

Hartree–Fock approximation (see Sect.

4.4). Only those products are consid-

ered whose factors are diagonal in the wave vector indices and thus, represent

macroscopic expectation values. Let us take as an example:

α

†

k−q

†

′

−q

′

†

−k

 = −α

†

k−q

′

†

′

−q

†

−k



+ α

†

′

−q

′

†

k−q

†

−k

 (10.117)

The two terms can b e factorized into products of macroscopic expectation

values appearing in the equations of motion to give

α

†

k−q

†

′

−q

′

†

−k

≃ −δ

k−q,k

′

α

†

k−q





 

ek−q

α

†

−k





 

∗

+ δ

q,0

α

†

′

α

†

−k

, (10.118)

where the last term does not contribute under the sum over q because q =0

is excluded. In the same way, one ﬁnds

α

†

′

−q

k−q

′

≃



k,k

′

− δ

q,0



(t)f

′

(t) , (10.119)

where the second term does not contribute under the sum over q =0.The

neglected terms represent collision terms, while the remaining ones give the

following set of equations

∂

∂t

(t)=−i



+ ǫ



(t)

− i

(t)+f

(t) − 1

¯h

E(t)+



q=0

k−q

(t)

(10.120)

∂

∂t

(t)=−

¯h

E(t)+



q=0

k−q

(t)

∗

(t)

(10.121)

∂

∂t

(t) . (10.122)

Here ¯hǫ

= E

(k)+Σ

(k),i = e, h are the renormalized single-particle

energies with the self-energy

(k)=−



|k−q|

. (10.123)

Problems 323

We introduce the general i zed Rabi frequency

R,k

(t)=

¯h



E(t)+



q=k

|k−q|

(t)



, (10.124)

which combines the applied ﬁeld with the dipole ﬁeld of the generated

electron–hole pairs, and arrive at the semiconductor Bloch equations:

∂

∂t

(t)=−i(ǫ

+ ǫ

) P

(t) − i(f

(t)+f

(t) − 1) ω

R,k

(t) (10.125)

∂

∂t

(t)=−2Im (ω

R,k

(t)P

∗

(t)) =

∂

∂t

(t). (10.126)

For v

→ 0, i.e., when switching oﬀ the Coulomb interaction, these equations

reduce to the optical Bloch equations.

In spite of their simple form, these equations describe the rather complex

dynamics of electron–hole pair excitations in that they include many-body

eﬀects within the Hartree–Fock approximaton. This results in the renormal-

ization of the single-particle energies and of the Rabi frequency, but (with the

population inversion factor) takes into account also the ﬁlling of the phase

space by the occupation of the single-particle states due to the light–matter

interaction. The occupied states (electrons in the conduction band and holes

in the valence band) reduce the available phase space for further excitations

because of the Pauli principle. This phase space ﬁlling is known as the Pauli

blocking. The semiconductor Bloch equations and their extensions, includ-

ing collision terms, are prerequisite in modelling nonlinear susceptibilities to

describe da ta from time-reso lved spectroscopy or in designing semiconductor

lasers. Damping terms with longitudinal and transverse relax ation times T

1,2

can be added as in the optical Bloch equations and pa rt of their microscopic

origin can be calculated by considering the collision terms.

Problems

10.1 Show with the help of p/m = −i/¯h[r,H

] that, in matrix representa-

tion with eigenstates of H

,theAp -coupling term can be expressed

(for resonant excitation) as d

· E with the dipole matrix element

= −ec0|r|v0  between band edge Bloch functions.

10.2 Make use of the Wannier representation (see Problem 6 .2) to show that

the momentum matrix element between Bloch functions is diagonal in

the wave vector.

10.3 Make use of the solution of the hydrogen problem to express |φ

(0)|

terms of a conﬂuent hypergeometric function for positive and negative

energies. Find the dep e n dence of this expression for the bound states

324 10 Light–Matter Interaction

in dependence on the quantum number n and calculate the absorption

coeﬃcient for the electron–hole continuum.

10.4 Formulate the exciton op erators B

ν,Q

†

ν,Q

in terms of products of

fermion operators for electrons in the conduction and valence band. Cal-

culate the commutator between the exciton operators and deﬁne the

condition under which excitons can be considered as bosons.

10.5 Start with the phenomenological result of (

10.42) close to an excitonic

resonance at E

ν0

=¯hω

ν0

and express the LT-splitting of this exciton in

terms of its oscillator strength! Consider the ﬁr st term of the exchange

interaction in (

10.62) to calculate the LT-splitting as a perturbation cor-

rection to the (tran sverse) exciton energy E

ν0

and verify by comparison

with the phenomenological result the expression for the exciton oscillator

strength given in (

10.43).

10.6 Make use of the expansion

A(r,t)=



λ,κ

¯h

2ε

ω(κ)V

1/2

λκ



λκ

(t)+a

†

λκ

(t)



iκ·r

(10.127)

of the vector potential in terms of Boson operators a

λκ

(t), where

λκ

,λ =1, 2 are transverse unit vectors with e

λκ

· κ =0,toshow

that the Hamiltonian of the radiation ﬁeld H

rad

takes the form

rad



λ,κ

¯hω(κ)

†

λκ

. (10.128)

10.7 Make use of the equation of motion for the oﬀ-diagonal element of the

density matrix under quasi-equilibrium condition to calculate the dielec-

tric polarization P (t)=Tr(ρ

(k,t)d

) and the dielectric susceptibility

χ(ω). Recover the result for the imaginary part of the dielectric function

(ω) obtained in Sect.

10.2.

10.8 Calculate the commutators of H

′

Coul

with the operators α

†

,β

†

and

†

−k

to verify (

10.114 ...116).

Appendices

A.1 Elements of Group Theory

Geometrical operations (translation, rotation, inversion), which leave a geo-

metrical object (here, the crystal lattice) invariant, are symmetry operations.

Mathematically, they form a group, the symmetry group of the crystal: for the

translations it is the translation group, for the r otations, inversion, and their

combinations it is the point group.Thenumberg of elements in the group

is its or der. The symmetry of a system implies the invariance of the system

Hamiltonian H (be it for phonons, electrons, or magnons) under unitary oper-

ations corresponding to the geometrical operations of the symmetry group.

These unitary operations form a group which is isomorphic to the symmetry

group. When applied to a set of eigenfunctions of H, this set is transformed

into another set of eigenfunctions, which can be represented as a linear com-

bination of the former ones. The eigenfunctions of a degenerate eigenvalue

transform among each other and form an invariant subspace in the Hilbert

space of H. In a chosen basis, these unitary operations can be formulated as

matrices which deﬁne another group isomorphic to the symmetry grou p. For

a proper choice of the basis, all matrices of the matrix representation have

block-diagonal form with the dimension of the block matrices indicating the

degeneracy of the invariant subspaces. These subspaces, spanned by the set

of degenerate eigenfunctions, can be classiﬁed by characteristic properties of

the corresponding block matrices using the character tables of the symmetry

group and the concept of irreducible representations [

47–50].

The symmetry classiﬁcation of eigenstates is the simplest for the transla-

tion group and ﬁnds its expression in Bloch’s theorem. A translation operator

applied to a Bloch function ψ

(r) yields

(r)=ψ

(r + R)=e

−ik·R

(r)(A.1)

i.e., it multiplies the Bloch function by a phase factor depending on the

wave vector k and the translation R. Owing to the fact that translations

326 A Appendices

commute with each other (the translation group is Abelian), there are only

one-dimensional repre sentations, namely the phase factors.

The spherical symmetry of the Coulomb potential leads to the angular

momentum classiﬁcation of the eigenstates of an atom. For the hydrogen

problem, we have the (2l + 1)-fold states with angular momentum l.They

transform under rotations with the corresponding (2l +1)× (2l +1) matri-

ces D

(α, β, γ), which form a (2l + 1)-dimensional irreducible representation

of the full rotation group. The group elements depend continuously on the

parameters α, β,andγ which deﬁne the g roup element by the three Euler

angles.

In contrast with the full rotation group (which is inﬁnite and continuous)

the point groups of crystal lattices are ﬁnite and discrete. For example, the

symmetry group of a c ube, O

, is the same as that of the sc, b cc, and fcc

lattices. It consists of 48 elements: the identity (E), three axes with four-

fold rotations (C

), four axes with threefold rotations (C

), six axes with

twofold rotations (C

), and all these operations combined with the inversion

(J). In general, the elements of the point group do not commute (the group

in non-Abelian). However, the point group falls into disjunct classes of conju-

gated elements, where group elements A and B are called conjugated to each

other if the relation A = XBX

−1

holds for all X of the group. For the cubic

point group there are 10 classes:

E,3C

, 6C

, 8C

, 6C

,J,3JC

, 6JC

, 8JC

, 6JC

(A.2)

where the numbers in front of the symbols for the symmetry operations give

the number of group elements belonging to the class.

Consider now the block matrices that transform the degenerate invariant

subspaces. They are d dimensional irreducible representations of the symme-

try group. Diﬀerent irreducible representations with the same dimension d,

D(X),D

′

(X), are equivalent if there is a d dimensional matrix M with

M =0andD(X)=MD

′

(X)M

−1

for all elements X of the group. Note,

that with respect to this operation with M, the coeﬃcients of the characteris-

tic polynomial of D(X), especially the trace of D(X)orthechar acter,donot

change. Thus, inequivalent irreducible representations can be distinguished

by looking at their characters. Similarly for the op eration of conjugation:

all matrices of an irreducible representation belonging to a class have the

same character. This leads to the character table listing the characters of the

inequivalent irreducible representations for the diﬀerent classes of conjugated

elements. These irreducible representations play the same role in classifying

the eigenstates of H with respect to the point group as the crystal momentum

k does for the translation group and the angular momentum l for the rota-

tion group. Their meaning is that of quantum numbers due to the underlying

symmetry. Already knowing the classes, it remains now to sp ecify the number

of the inequivalent irreducible representations and their dimensions.

According to the theorems of the theory of ﬁnite groups, the number of

classes equals the number of irreducible representations, i.e., the character

table has the same number of rows and columns. Moreover, the sum over the

A.1 Elements of Group Theory 327

squared dimensions of the irreducible representations (which is the sum of the

characters for the class containing the identity E, because it is represented by

d-dimensional unit matrices) must be equal to the order g of the group. As it

turns out, the order of the cubic symmetry group can be decomposed only in

one way into 10 squared integers:

48 = 2(1

), (A.3)

i.e., the group has four one-dimensional, two two-dimensional, and four three-

dimensional irreducible representations. As the inversion J is an element of

the group, the eigenstates can be classiﬁed as having even or odd parity. This

is considered in the notation of the irreducible representations by a ± or g, u

(for gerade or ungerade). Another remarkable property of the character table

is that the rows and columns understood as vectors are orthogonal to each

other when properly weighted with the number of elements in a class. These

are the famous orthogonality relations of the characters.

Diﬀerent notations are in use for the irreducible representations of the

cubic point group O

(see the character table). The notation with the symbols

A, E, and T for on e-, two-, and three-dimensional representations, respec-

tively, is applied to characterize localized (e.g., impurity) states of the given

point symmetry, while the notation with the symbol Γ refers to the Bloch

states a t the center of the Br i llouin zone with k =(0, 0, 0). (Note, that this

wave vector does not change under the symmetry operations and therefore,

the group of this wave vector is the point group of the crystal.) Of the two dif-

ferent notations with the symbol Γ the one with double indices is the older one

and indicates the removal of the level degeneracy for ﬁnite k. These symbols

are found e.g., in some ﬁgures of Chaps.

5 and 9.

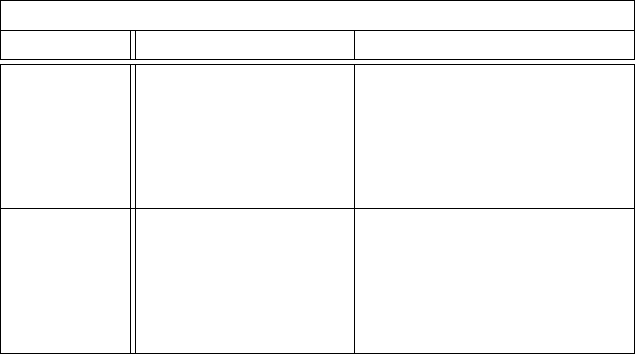
Character table of the point group O

E 3C

J 3JC

8JC

6JC

1111111111

111−1 −1 11 1−1 −1

22−10022−100

3 −101−1 3 −101−1

3 −10−113 −10−11

−

11111−1 −1 −1 −1 −1

−

111−1 −1 −1 −1 −111

−

22−100−2 −2100

−

3 −101−1 −31 0−11

−

3 −10−11−31 0 1−1

It is quite instructive to sp ecify objects (wave functions, operators) that

transform according to these irreducible representations. Using the fact, that

328 A Appendices

the crystal point groups are subgroups of the full rotation group, this can be

done by formulating the spherical harmonics in Cartesian coordinates to ﬁnd

the so-called cubic harmonics, which for l =0, 1, 2, 3aregivenby

l =0→ 1(Γ

)

l =1→ x, y, z (Γ

−

)

l =2→ z

−

+ y

),x

− y

(Γ

); yz, zx, xy (Γ

)

l =3→ xyz (Γ

−

); z(x

− y

),x(y

− z

),y(z

− x

)(Γ

−

Thus, the ﬁvefold degeneracy of the l = 2 spherical har monics splits under

the r educed symmetry o f the cubic point gr oup into a twofold (Γ

)anda

threefold level (Γ

), which is the crystal ﬁeld splitting discussed in Sect.

5.5

and Problem 5.8. Of the seven spherical harmonics with l = 3 only four appear

in this list, while the remaining three are cubic harmonics which transform as

−

, i.e., the cubic cry stal ﬁeld causes a mixing of angular momentum states

with l =1and3.

The point group T

of the zinc blende lattice is a subgroup of O

and con-

tains the classes E, 3C

, 8C

, 6JC

,and6JC

. Consequently, the number

of irreducible representations is reduced to ﬁve. Considering only the corre-

sponding columns in the character table of O

, we ﬁnd identical rows for pairs

of representations which merge into one irreducible representation of T

.Thus,

the character table of T

is obtained from that of O

.NotethatT

does not

contain the inversion J and eigenstates cannot be classiﬁed by parity.

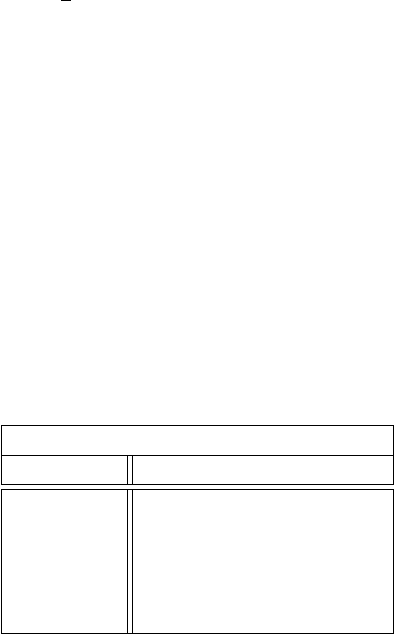
Character table of the point group T

E 3C

6JC

111 1 1

111−1 −1

E Γ

22−10 0

3 −10 1−1

3 −10−11

The crystal momentum k is a good quantum number. Thus, for ﬁnite

k the eigenstates have to be classiﬁed by the irreducible representations of

the g roup of the wave vector, consisting of all elements of the crystal point

group which do not change k.Fork  (1, 0, 0) or along the ∆-axis o f the

Brillouin zone the cub ic point group O

reduces to C

with eight elements in

ﬁve classes, and T

to C

with four elements each in one class. Decomposing

8 = 1+1+1+1+2

gives the dimensions of the ﬁve irreducible r epresentations

of C

, while (obviously) C

has four one-dimensional representations. The

notation of the irreducible representations reminds of the ∆-axis. The group of

the wave vector along (1,0,0) keeps x unchanged while y and z change. Thus, x

transforms as ∆

, while for C

y and z transform into each other like ∆

and

A.2 Fourier Series and Fourier Transforms 329

the threefold states of symmetry Γ

−

split into ∆

+ ∆

(which is considered

the notation Γ

−

). This explains the splitting of the phonon dispersion curves

(Chap.

3) and of the energy bands (Chap. 5) away from the Γ point. For C

y and z transform according to diﬀerent irreducible representations ∆

, ∆

but

due to time invariance these states are degenerate. Similar considerations hold

for the other directions in k space.

2JC

∆

111 1 1

∆

111−1 −1

∆

11−11−1

∆

11−1 −11

∆

2 −20 0 0

′

∆

111 1

∆

1 −11−1

∆

11−1 −1

∆

1 −1 −11

A.2 Fourier Series and Fourier Transforms

Consider a function f(x) deﬁned in the interval −L/2 ≤ x ≤ +L/2ora

periodic function f (x + L)=f(x). It can be represented by the Fourier series

f(x)=

+∞



n=−∞

, with k

2nπ

,ninteger. (A.4)

The Fourier coeﬃcients are given by



+L/2

−L/2

f(x)e

−ik

dx. (A.5)

If the length L is taken as the linear extension of a solid and f (x)asawave

function describing some state of the solid, the periodicity of f(x) reﬂects the

reasonable assumption that the physical properties connected with this state

repeat with the period L or, what is equivalent, that t hey do not depend on L.

This is the concept of periodic boundary conditions.

The Fourier series expansion makes us e of the fact that complex exp onen-

tials are normalized and orthogonal, i.e.,



+L/2

−L/2

−ik

dx = δ

, (A.6)

and that they form a complete set on the interval of length L:



(x−x

′

)

= δ(x − x

′

). (A.7)