Schutz B. A first course in general relativity

Подождите немного. Документ загружается.

23 1.11 Paradoxes and physical intuition

Suppose a particle has speed W in the ¯x direction of

O, i.e. ¯x/

t = W. In another

frame O, its velocity will be W



= x/t, and we can deduce x and t from the Lorentz

transformation. If

O moves with velocity v with respect to O, then Eq. (1.12) implies

x = (¯x +v

t)/(1 −v

)

1/2

and

t = (

t +v¯x)/(1 −v

)

1/2

Then we have



x

t

(¯x + v

t)/(1 −v

)

1/2

(

t +v¯x)/(1 −v

)

1/2

¯x/

t +v

1 + v¯x/

W + v

1 + Wv

. (1.13)

This is the Einstein law of composition of velocities. The important point is that |W



| never

exceeds 1 if |W| and |v| are both smaller than one. To see this, set W



= 1. Then Eq. (1.13)

implies

(1 − v)(1 −W) = 0,

that is that either v or W must also equal 1. Therefore, two ‘subluminal’ velocities produce

another subluminal one. Moreover, if W = 1, then W



= 1 independently of v: this is the

universality of the speed of light. What is more, if |W|1 and |v|1, then, to ﬁrst order,

Eq. (1.13)gives



= W + v.

This is the Galilean law of velocity addition, which we know to be valid for small velocities.

This was true for our previous formulae in § 1.8: the relativistic ‘corrections’ to the Galilean

expressions are of order v

, and so are negligible for small v.

1.11 Pa ra d oxe s a nd p h ys i ca l i nt u it i o n

Elementary introductions to SR often try to illustrate the physical differences between

Galilean relativity and SR by posing certain problems called ‘paradoxes’. The commonest

ones include the ‘twin paradox’, the ‘pole-in-the-barn paradox’, and the ‘space-war para-

dox’. The idea is to pose these problems in language that makes predictions of SR seem

inconsistent or paradoxical, and then to resolve them by showing that a careful application

of the fundamental principles of SR leads to no inconsistencies at all: the paradoxes are

apparent, not real, and result invariably from mixing Galilean concepts with modern ones.

Unfortunately, the careless student (or the attentive student of a careless teacher) often

comes away with the idea that SR does in fact lead to paradoxes. This is pure nonsense.

Students should realize that all ‘paradoxes’ are really mathematically ill-posed problems,

that SR is a perfectly consistent picture of spacetime, which has been experimentally veri-

ﬁed countless times in situations where gravitational effects can be neglected, and that SR

24 Special relativity

‘Its top speed is 186 mph – that’s 1/3 600 000 the speed of light.’

Figure 1.15

The speed of light is rather far from our usual experience! (With kind permission of S. Harris.)

forms the framework in which every modern physicist must construct his theories. (For the

student who really wants to study a paradox in depth, see ‘The twin “paradox” dissected’

in this chapter.)

Psychologically, the reason that newcomers to SR have trouble and perhaps give ‘para-

doxes’ more weight than they deserve is that we have so little direct experience with

velocities comparable to that of light (see Fig. 1.15). The only remedy is to solve problems

in SR and to study carefully its ‘counter-intuitive’ predictions. One of the best methods for

developing a modern intuition is to be completely familiar with the geometrical picture of

SR: Minkowski space, the effect of Lorentz transformations on axes, and the ‘pictures’ of

such things as time dilation and Lorentz contraction. This geometrical picture should be in

the back of your mind as we go on from here to study vector and tensor calculus; we shall

bring it to the front again when we study GR.

1.12 F urt h er re ad i ng

There are many good introductions to SR, but a very readable one which has guided our

own treatment and is far more detailed is Taylor and Wheeler (1966). Another widely

admired elementary treatment is Mermin (1989). Another classic is French (1968). For

treatments that take a more thoughtful look at the fundamentals of the theory, consult

Arzeliès (1966), Bohm (2008), Dixon (1978), or Geroch (1978). Paradoxes are discussed

25 1.13 Appendix: The twin ‘paradox’ dissected

in some detail by Arzeliès (1966), Marder (1971), and Terletskii (1968). For a scientiﬁc

biography of Einstein, see Pais (1982).

Our interest in SR in this text is primarily because it is a simple special case of GR

in which it is possible to develop the mathematics we shall later need. But SR is itself

the underpinning of all the other fundamental theories of physics, such as electromag-

netism and quantum theory, and as such it rewards much more study than we shall give it.

See the classic discussions in Synge (1965), Schrödinger (1950), and Møller (1972), and

more modern treatments in Rindler (1991), Schwarz and Schwarz (2004), and Woodhouse

(2003).

The original papers on SR may be found in Kilmister (1970).

1.13 A p p en d ix : T h e t w in ‘ p ar ad ox ’ d i ss e ct ed

The problem

Diana leaves her twin Artemis behind on Earth and travels in her rocket for 2.2 ×10

(≈ 7yr)ofher time at 24/25 = 0.96 the speed of light. She then instantaneously reverses

her direction (fearlessly braving those gs) and returns to Earth in the same manner. Who is

older at the reunion of the twins? A spacetime diagram can be very helpful.

Brief solution

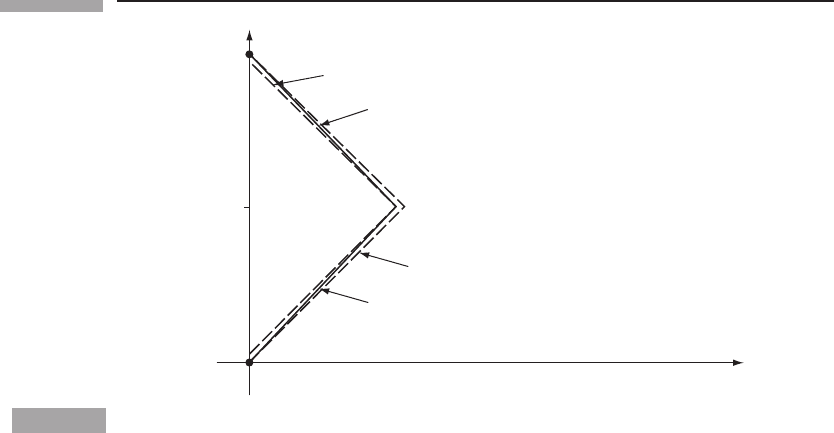
Refer to Fig. 1.16 on the next page. Diana travels out on line PB. In her frame, Artemis’

event A is simultaneous with event B, so Artemis is indeed ageing slowly. But, as soon as

Diana turns around, she changes inertial reference frames: now she regards B as simulta-

neous with Artemis’ event C! Effectively, Diana sees Artemis age incredibly quickly for

a moment. This one spurt more than makes up for the slowness Diana observed all along.

Numerically, Artemis ages 50 years for Diana’s 14.

Fuller discussion

For readers who are unsatisﬁed with the statement ‘Diana sees Artemis age incredibly

quickly for a moment’, or who wonder what physics lies underneath such a statement,

we will discuss this in more detail, bearing in mind that the statement ‘Diana sees’ really

means ‘Diana observes’, using the rods, clocks, and data bank that every good relativistic

observer has.

Diana might make her measurements in the following way. Blasting off from Earth, she

leaps on to an inertial frame called

O rushing away from the Earth at v = 0.96. As soon

as she gets settled in this new frame, she orders all clocks synchronized with hers, which

read

t = 0 upon leaving Earth. She further places a graduate student on every one of her

26 Special relativity

Departure Artemis’ line of simultaneity

Artemis’





Diana’s first time-axis

Diana’s first line of simultaneity

Reversal (Diana changes reference frames)

Diana’s second time-axis

Diana’s second line of simultaneity

Reunion

50 yr

25 yr





Time axis

Figure 1.16

The idealized twin ‘paradox’ in the spacetime diagram of the stay-at-home twin.

clocks and orders each of them who rides a clock that passes Earth to note the time on

Earth’s clock at the event of passage. After traveling seven years by her own watch, she

leaps off inertial frame

O and grabs hold of another one



that is ﬂying toward Earth at

v = 0.96 (measured in Earth’s frame, of course). When she settles into this frame, she again

distributes her graduate students on the clocks and orders all clocks to be synchronized with

hers, which read



= 7 yr at the changeover. (All clocks were already synchronized with

each other – she just adjusts only their zero of time.) She further orders that every graduate

student who passes Earth from



= 7 yr until she gets there herself should record the time

of passage and the reading of Earth’s clocks at that event.

Diana ﬁnally arrives home after ageing 14 years. Knowing a little about time dilation,

she expects Artemis to have aged much less, but to her surprise Artemis is a white-haired

grandmother, a full 50 years older! Diana keeps her surprise to herself and runs over to

the computer room to check out the data. She reads the dispatches from the graduate stu-

dents riding the clocks of the outgoing frame. Sure enough, Artemis seems to have aged

very slowly by their reports. At Diana’s time

t = 7yr, the graduate student passing Earth

recorded that Earth’s clocks read only slightly less than two years of elapsed time. But

then Diana checks the information from her graduate students riding the clocks of the

ingoing frame. She ﬁnds that at her time



= 7 yr, the graduate student reported a reading

of Earth’s clocks at more than 48 years of elapsed time! How could one student see Earth

to be at t = 2 yr, and another student, at the same time, see it at t = 48 yr? Diana leaves the

computer room muttering about the declining standards of undergraduate education today.

We know the mistake Diana made, however. Her two messengers did not pass Earth

at the same time. Their clocks read the same amount, but they encountered Earth at the

very different events A and C. Diana should have asked the ﬁrst frame students to continue

recording information until they saw the second frame’s



= 7 yr student pass Earth. What

27 1.13 Appendix: The twin ‘paradox’ dissected

(a)







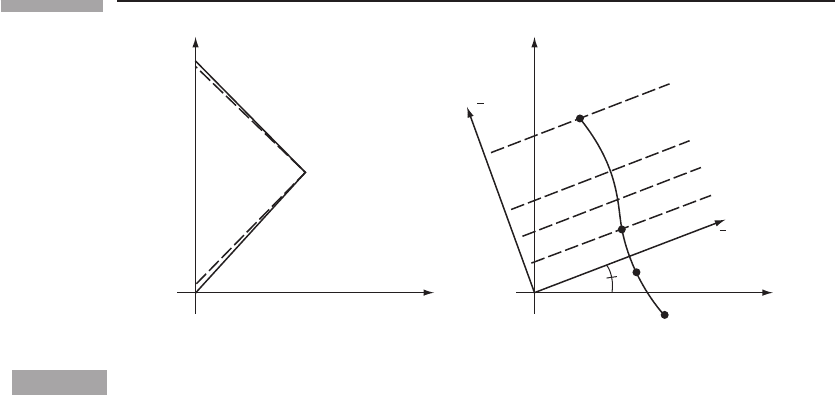
Diana assigns t

= 7 yr to

both of these dashed lines!

(b)

Figure 1.17

Diana’s change of frame is analogous to a rotation of coordinates in Euclidean geometry.

does it matter, after all, that they would have sent her dispatches dated

t = 171 yr? Time is

only a coordinate. We must be sure to catch all the events.

What Diana really did was use a bad coordinate system. By demanding information

only before

t = 7 yr in the outgoing frame and only after



= 7 yr in the ingoing frame,

she left the whole interior of the triangle ABC out of her coordinate patches (Fig. 1.17(a)).

Small wonder that a lot happened that she did not discover! Had she allowed the ﬁrst

frame’s students to gather data until

t = 171 yr, she could have covered the interior of that

triangle.

We can devise an analogy with rotations in the plane (Fig. 1.17(b)). Consider trying

to measure the length of the curve ABCD, but being forced to rotate coordinates in the

middle of the measurement, say after you have measured from A to B in the x −y system.

If you then rotate to ¯x −¯y, you must resume the measuring at B again, which might be at a

coordinate ¯y =−5, whereas originally B had coordinate y = 2. If you were to measure the

curve’s length starting at whatever point had ¯y = 2 (same ¯y as the y value you ended at in

the other frame), you would begin at C and get much too short a length for the curve.

Now, nobody would make that error in measurements in a plane. But lots of people

would if they were confronted by the twin paradox. This comes from our refusal to see time

as simply a coordinate. We are used to thinking of a universal time, the same everywhere to

everyone regardless of their motion. But it is not the same to everyone, and we must treat

it as a coordinate, and make sure that our coordinates cover all of spacetime.

Coordinates that do not cover all of spacetime have caused a lot of problems in GR.

When we study gravitational collapse and black holes we will see that the usual coordinates

for the spacetime outside the black hole do not reach inside the black hole. For this reason,

a particle falling into a black hole takes inﬁnite coordinate time to go a ﬁnite distance. This

is purely the fault of the coordinates: the particle falls in a ﬁnite proper time, into a region

not covered by the ‘outside’ coordinates. A coordinate system that covers both inside and

outside satisfactorily was not discovered until the mid-1950s.

28 Special relativity

1.14 E xe rc is e s

1 Convert the following to units in which c = 1, expressing everything in terms of m

and kg:

(a) Worked example: 10 J. In SI units, 10 J = 10 kg m

−2

. Since c = 1, we have

1s= 3 × 10

m, and so 1 s

−2

= (9 ×10

)

−1

−2

. Therefore we get 10 J =

10 kg m

(9 × 10

)

−1

−2

= 1.1 ×10

−16

kg. Alternatively, treat c as a conver-

sion factor:

1 = 3 ×10

−1

1 = (3 × 10

)

−1

10 J = 10 kg m

−2

= 10 kg m

−2

× (1)

= 10 kg m

−2

× (3 × 10

)

−2

= 1.1 ×10

−16

kg.

You are allowed to multiply or divide by as many factors of c as are necessary to

cancel out the seconds.

(b) The power output of 100 W.

−34

J s. (Note the deﬁnition of  in terms

of Planck’s constant h:  = h/2π .)

(d) Velocity of a car, v = 30 m s

−1

(e) Momentum of a car, 3 × 10

kg m s

−1

(f) Pressure of one atmosphere = 10

−2

(g) Density of water, 10

kg m

−3

(h) Luminosity ﬂux 10

−1

−2

2 Convert the following from natural units (c = 1) to SI units:

(a) A velocity v = 10

−2

(b) Pressure 10

kg m

−3

(d) Energy density u = 1kgm

−3

(e) Acceleration 10 m

−1

3 Draw the t and x axes of the spacetime coordinates of an observer O and then draw:

(a) The world line of O’s clock at x = 1m.

(b) The world line of a particle moving with velocity dx/dt = 0.1, and which is at

x = 0.5 m when t = 0.

t and ¯x axes of an observer

O who moves with velocity v = 0.5 in the positive

x direction relative to O and whose origin (¯x =

t = 0) coincides with that of O.

(d) The locus of events whose interval s

from the origin is −1m

(e) The locus of events whose interval s

from the origin is +1m

(f) The calibration ticks at one meter intervals along the ¯x and

t axes.

(g) The locus of events whose interval s

from the origin is 0.

(h) The locus of events, all of which occur at the time t = 2 m (simultaneous as seen

by O).

29 1.14 Exercises

(i) The locus of events, all of which occur at the time

t = 2 m (simultaneous as seen

O).

(j) The event which occurs at

t = 0 and ¯x = 0.5 m.

(k) The locus of events ¯x = 1m.

(l) The world line of a photon which is emitted from the event t =−1m,x = 0, trav-

els in the negative x direction, is reﬂected when it encounters a mirror located at

¯x =−1 m, and is absorbed when it encounters a detector located at x = 0.75 m.

4 Write out all the terms of the following sums, substituting the coordinate names

(t, x, y, z)for(x

, x

(a)



α=0

x

, where {V

, α = 0, ... ,3} is a collection of four arbitrary numbers.

(b)



i=1

(x

)

5 (a) Use the spacetime diagram of an observer O to describe the following experiment

performed by O. Two bursts of particles of speed v = 0.5 are emitted from x = 0

at t =−2 m, one traveling in the positive x direction and the other in the negative

x direction. These encounter detectors located at x =±2 m. After a delay of 0.5 m

of time, the detectors send signals back to x = 0 at speed v = 0.75.

(b) The signals arrive back at x = 0 at the same event. (Make sure your spacetime dia-

gram shows this!) From this the experimenter concludes that the particle detectors

did indeed send out their signals simultaneously, since he knows they are equal

distances from x = 0. Explain why this conclusion is valid.

O moves with speed v = 0.75 in the negative x direction rel-

ative to O. Draw the spacetime diagram of

O and in it depict the experiment

performed by O. Does

O conclude that particle detectors sent out their signals

simultaneously? If not, which signal was sent ﬁrst?

(d) Compute the interval s

between the events at which the detectors emitted their

signals, using both the coordinates of O and those of

6 Show that Eq. (1.2) contains only M

αβ

+ M

βα

when α = β, not M

αβ

and M

βα

independently. Argue that this enables us to set M

αβ

= M

βα

without loss of generality.

7 In the discussion leading up to Eq. (1.2), assume that the coordinates of

O are given as

the following linear combinations of those of O:

t = αt +βx,

¯x = μt + vx,

¯y = ay,

¯z = bz,

where α, β, μ, ν, a, and b may be functions of the velocity v of

O relative to O,but

they do not depend on the coordinates. Find the numbers {M

αβ

, α, b = 0, ... ,3} of

Eq. (1.2) in terms of α, β, μ, ν, a, and b.

8 (a) Derive Eq. (1.3)fromEq.(1.2), for general {M

αβ

, α, β = 0, ... ,3}.

(b) Since ¯s

= 0inEq.(1.3) for any {x

}, replace x

by −x

in Eq. (1.3) and

subtract the resulting equation from Eq. (1.3) to establish that M

= 0fori =

1, 2, 3.

30 Special relativity

= 0toestablishEq.(1.4b). (Hint: x, y, and z are

arbitrary.)

9 Explain why the line PQ in Fig. 1.7 is drawn in the manner described in the text.

10 For the pairs of events whose coordinates (t, x, y, z) in some frame are given below,

classify their separations as timelike, spacelike, or null.

(a) (0, 0, 0, 0) and (−1,1, 0, 0),

(b) (1, 1, −1, 0) and (−1, 1, 0, 2),

(d) (−1, 1, −1, 1) and (4, 1, −1, 6).

11 Show that the hyperbolae −t

+ x

= a

and −t

+ x

=−b

are asymptotic to the

lines t =±x, regardless of a and b.

12 (a) Use the fact that the tangent to the hyperbola DB in Fig. 1.14 is the line of simul-

taneity for

O to show that the time interval AE is shorter than the time recorded

O’s clock as it moved from A to B.

(b) Calculate that

(s

)

= (1 −v

)(s

)

O regards O’s clocks to be running slowly, at just the ‘right’

rate.

13 The half-life of the elementary particle called the pi meson (or pion) is 2.5 ×10

−8

when the pion is at rest relative to the observer measuring its decay time. Show, by the

principle of relativity, that pions moving at speed v = 0.999 must have a half-life of

5.6 × 10

−7

s, as measured by an observer at rest.

14 Suppose that the velocity v of

O relative to O is small, |v|1. Show that the time

dilation, Lorentz contraction, and velocity-addition formulae can be approximated by,

respectively:

(a) t ≈ (1 +

)

(b) x ≈ (1 −

)¯x,



≈ w + v −wv(w + v) (with |w|1 as well).

What are the relative errors in these approximations when |v|=w = 0.1?

15 Suppose that the velocity v of

O relative to O is nearly that of light, |v|=1 −ε,

0 <ε 1. Show that the same formulae of Exer. 14 become

(a) t ≈ 

√

(2ε),

(b) x ≈ ¯x/

√

(2ε),



≈ 1 − ε(1 − w)/(1 +w).

What are the relative errors on these approximations when ε = 0.1 and w = 0.9?

16 Use the Lorentz transformation, Eq. (1.12), to derive (a) the time dilation, and (b)

the Lorentz contraction formulae. Do this by identifying the pairs of events where

the separations (in time or space) are to be compared, and then using the Lorentz

transformation to accomplish the algebra that the invariant hyperbolae had been used

for in the text.

17 A lightweight pole 20 m long lies on the ground next to a barn 15 m long. An Olympic

athlete picks up the pole, carries it far away, and runs with it toward the end of the barn

31 1.14 Exercises

at a speed 0.8 c. His friend remains at rest, standing by the door of the barn. Attempt

all parts of this question, even if you can’t answer some.

(a) How long does the friend measure the pole to be, as it approaches the barn?

(b) The barn door is initially open and, immediately after the runner and pole are

entirely inside the barn, the friend shuts the door. How long after the door is shut

does the front of the pole hit the other end of the barn, as measured by the friend?

Compute the interval between the events of shutting the door and hitting the wall.

Is it spacelike, timelike, or null?

(d) Does the runner believe that the pole is entirely inside the barn when its front hits

the end of the barn? Can you explain why?

(e) After the collision, the pole and runner come to rest relative to the barn. From the

friend’s point of view, the 20 m pole is now inside a 15 m barn, since the barn door

was shut before the pole stopped. How is this possible? Alternatively, from the

runner’s point of view, the collision should have stopped the pole before the door

closed, so the door could not be closed at all. Was or was not the door closed with

the pole inside?

(f) Draw a spacetime diagram from the friend’s point of view and use it to illustrate

and justify all your conclusions.

18 (a) The Einstein velocity-addition law, Eq. (1.13), has a simpler form if we introduce

the concept of the velocity parameter u, deﬁned by the equation

v = tanh u.

Notice that for −∞< u < ∞, the velocity is conﬁned to the

acceptable limits −1 <v<1. Show that if

v = tanh u

and

w = tanh U,

then Eq. (1.13) implies



= tanh(u + U).

This means that velocity parameters add linearly.

(b) Use this to solve the following problem. A star measures a second star to be moving

away at speed v = 0.9 c. The second star measures a third to be receding in the

same direction at 0.9 c. Similarly, the third measures a fourth, and so on, up to

some large number N of stars. What is the velocity of the Nth star relative to the

ﬁrst? Give an exact answer and an approximation useful for large N.

19 (a) Using the velocity parameter introduced in Exer. 18, show that the Lorentz

transformation equations, Eq. (1.12), can be put in the form

t = t cosh u − x sinh u, ¯y = y,

¯x =−t sinh u + x cosh u, ¯z = z.

32 Special relativity

(b) Use the identity cosh

u − sinh

u = 1 to demonstrate the invariance of the

interval from these equations.

nary two-dimensional Euclidean geometry, where the coordinate transformation

analogous to the Lorentz transformation is

¯x = x cos θ +y sin θ,

¯y =−x sin θ + y cos θ.

What is the analog of the interval? Of the invariant hyperbolae?

20 Write the Lorentz transformation equations in matrix form.

21 (a) Show that if two events are timelike separated, there is a Lorentz frame in which

they occur at the same point, i.e. at the same spatial coordinate values.

(b) Similarly, show that if two events are spacelike separated, there is a Lorentz frame

in which they are simultaneous.