Urry D.W. (Ed.) What Sustains Life? : Consilient Mechanisms for Protein-Based Machines and Materials

Подождите немного. Документ загружается.

8.1 Thesis 345

in extension, is completely recovered on release

of the deforming force, that is, on relaxation.

On the other hand, for a nonideal elastomer, all

of the energy expended in deformation is not

recovered on relaxation. In this case, the

stress-strain curve is said to exhibit hysteresis.

8.1.9.3.3

Performance of Mechanical Work

with an Isometric Contraction Followed by

an Isotonic Contraction with Relaxation to

Initial Force

During an isometric contraction, for example,

due to hydrophobic association, force develops

by the damping of internal chain dynamics

of an interconnecting elastic chain segment

(see Figure 5.8). To the extent that the chain

segment exhibits hysteresis, on allowing the

decrease in chain length to ensue (as in an

isotonic contraction), only a part of the energy

of deformation is recovered. Such a

mechanochemical engine could be no more

efficient than allowed by the nonideal (hys-

teretic) elastic chain segment.

In our analysis of hysteresis, appreciation was

clear for an elastic segment that stores all of the

deforming energy in the chain backbone. Thus,

a mechanochemical motor, in which loss of

deformation energy into associated chains

occurred, would decrease efficiency for the con-

version of chemical energy into mechanical

work and vice versa. This begins to place con-

straints on the mechanism of efficient biologi-

cal motors, and it begins to limit the utilization

of levers, ratchets, and physical pushing derived

from mechanical interactions between chains

when searching for a more efficient mechanism

for the performance of mechanical, chemical, or

even electrical work.

8.1.10

The Electrostatic Argument

of a Low Dielectric Constant Versus

AGap to Explain pKa Shifts and

Ion-pair Formation

8J. 10.1 Why Ion Pairs Form in

Protein-based Polymers

Salts dissolve in water because the free energy

of the separated hydrated ion is lower than

when associated with other ions within the salt

crystal. Ionized side chains of proteins remain

in water as long as they retain adequate hydra-

tion. Therefore, any process that decreases

effective hydration of ionic side chains of pro-

teins becomes a driving force for ion pairing.

Commonly, hydration has been treated by

assuming a uniform medium of a given dielec-

tric constant, e, where the force, f, between two

charges, q and q', is given by the product, qq',

divided by the distance, r, between charges

squared times the dielectric constant, that is, f =

qqVer^. When the charges are on a pair of oppo-

sitely charged plates, with water between them,

the value of

for water is experimentally deter-

mined to be about 80 at room temperature.

Thus,

by this argument, and consistent with

limited water, ions pair only when 8 becomes

sufficiently small. In calculations of protein, an

8 as low as 5 or less is used, and in calculations

of small peptides where there are partial

charges on atoms instead of a charge of one or

more, 8 has been commonly taken as unity.^^'^^

Qualitatively, the argument for using a low

dielectric constant seems reasonable. The

low dielectric constant comes into question,

however, on closer scrutiny of experimental

results on model proteins for which control of

composition is possible, and it makes difficult

what must be subjective judgments of a correct

dielectric constant for water-filled clefts and

crevices of various dimensions found in many

protein-based machines.

8.1.10.2 The Usual Electrostatic Argument

of a Low Dielectric Constant as

Responsible for pKa Shifts

The usual electrostatic consideration of pKa

shifts has been to assume that the ionizable

function resides in a location within the protein

that is of low dielectric constant. As the rea-

soning goes, because of the low dielectric locale,

the carboxyl function does not ionize to form

carboxylate until the pH is much higher, that is,

the carboxyl exhibits a pKa shift. A simple

means of relating pKa shift to dielectric con-

stant uses the expression for solvation energy,

SE,

due to Born, SE = [(ze)^/2r](l - 1/8), where

z is the charge on the ion, e is the unit electron

charge, 8 is the dielectric constant of the sur-

rounding medium, and r now stands for the

radius of the ion. As treated in Chapter 5,

346

8. Consilient Mechanisms for Protein-based Machines of Biology

section 5.7.8.3, the interest is in fitting the

series of experimental data points due to pKa

shift as a function of mole fraction,

/K,

of the

lysine functional group in the polymer,

poly[/v(GVGIP),/x(GXGIP)] with X = Lys(K)

and /K varied from 1.0 to 0.06.^^ The data are

included in Figure 5.30 along with the data for

poly[/v(GVGIP),/x(GXGIP)] with X = Glu(E),

and /x again varied from 1.0 to 0.06.^^

Interest centers on the change in solvation

energy, ASE = Q[(£i-i - 8i)/8i_i8i], where Q is

substituted for the coefficient [(ze)V2r]. To

fit the data in Figure 5.30A,B, a dielectric

constant of 5 or less had to be used. Yet from

experimental determination of the polymer,

poly(GVGIP), at the phase-separated concen-

tration of 61% polymer and 39% water by

weight, the minimal value for the dielectric

constant was 65.^^ Because the polymer is a

repeating pentapeptide sequence, because the

barrier to backbone mobility is between 1 and

1.5kcal/mole, and because any introduction of

a charged functional group must raise the value

of the dielectric constant, there is no way that

the functional group can be held in a locale of

low dielectric constant. There must be another

physical basis for the hydrophobic-induced pKa

shifts in Figure 5.30. As developed in Chapter 5

and reviewed briefly above, the physical basis

is the competition for hydration between

hydrophobic and charged groups, called AGap,

the apolar-polar repulsive free energy of

hydration.

Accordingly, one should maintain a healthy

skepticism of the results of calculations of

protein structure and function where low

dielectric constants become the basis to drive

ion-pair formation. This is especially the case

when there are water-filled crevices and clefts

on the surface of proteins and indeed coursing

through the protein structure. The "waters of

Thales" are there as an integral part of protein

structure and function. To assume otherwise

has been a useful approximation in the past, but

it would seem no longer to be the most pro-

ductive approach.

Another limitation of the assumption of low

dielectric constants to explain pKa shifts is the

absence of an explanation for the obligatory

coupling of pKa shifts to positive cooperativity

shown in Figures 5.20,5.23,5.31, and 5.34. Also,

as argued above, positive cooperativity is a

central aspect of efficient energy conversion by

protein-based machines and naturally arises

out of a competition for hydration between

hydrophobic and charged groups.

Positive cooperativity arises from a competi-

tion for hydration between hydrophobic and

charged residues just as do pKa shifts. On the

other

hand,

the classic electrostatic argument

would appear to have no obvious way to become

integral with positive cooperativity: Further-

more, in Figure 1.4 a residual pKa shift of 1.7

pH units is found under circumstances where the

model protein is completely unfolded. Under

such circumstances one is compelled by the elec-

trostatic analysis to choose a dielectric constant

approaching 80 where there would be no sig-

nificant energy of interaction. In our view,

the classic electrostatic argument is simply left

wanting, unable to describe the extensive data on

elastic-contractile model proteins.

8.1.10.3 Electrostatics Become Significant

in Protein Structure and Function When

Charges Are Under the Influence of

Hydrophobic Domains

Electrostatic interactions, considered here

simply as the interactions of charged species,

become significant in proteins in aqueous media

when under the influence of hydrophobic

domains (see Figures 5.30A,B), that is, when

competing for adequate hydration with hydro-

phobic groups. In particular, pKa shifts and

changes in reduction potential do not necessar-

ily result from being buried in a localized region

(medium) of low dielectric constant within the

protein or from direct electrostatic interactions,

but rather result from competition for hydration

between hydrophobic and polar groups.

It is our behef that the presence of hydrated

hydrophobic side chains limits the available

hydration for ionic side chains and increases

the probability of ion pairing. Thus, while the

formation of ion pairs may be considered an

electrostatic event, it occurs in the aqueous

milieu of protein folding, assembly, and function

due to the dominance of the presence of, or

potential for, hydrophobic hydration.

8.1 Thesis

347

Above, in section 8.1.9.1, we considered a

purely electrostatic mechanism for chemo-

mechanical transduction. A high density of

charged groups dominated a relatively minimal

presence of hydrophobic groups in the cross-

linked polymer PMA. Its efficiency for chemo-

mechanical transduction was compared with

the inverse circumstance of limited charge

under the influence of a dominantly hydro-

phobic model protein. The latter proved to be

much more efficient even though the functional

group was the same, the -COOH/-COO"

chemical couple.

8.1.11

ATP: Biology's

Energy Currency

The apolar-polar repulsive free energy of

hydration, AGap, as reviewed above, results

from a competition for hydration between

charged species and hydrophobic groups. In

the most extreme case reported thus far, this

competition raised the pKa of a carboxyl from

about 4 to about 11.^^ This constitutes an

amount sufficient to raise the free energy of

carboxylates by 8 to lOkcal/mole. The basic

issue to be addressed here is whether there is

reason to believe that the free energy of the

phosphate group would also be subject to

competition for hydration with hydrophobic

groups.

Simply stated, is the free energy of hydroly-

sis of ATP and other related phosphoanhy-

drides sensitive to limitations in hydration? If

the answer is no, then the apolar-polar repul-

sive free energy of hydration, AGap, would not

be relevant to ATP's role in energy conversion.

If the answer is yes, then phosphates would

indeed be sensitive to the competition for

hydration with hydrophobic groups, as exten-

sively documented experimentally with the car-

boxylate group. Again, if the answer is yes, then

the development, within Chapter

most

specif-

ically section 5.7 and the associated data in

Figures 5.23 through 5.32, of the concept of an

apolar-polar repulsive free energy of hydration

would be fundamentally congruent with the

energizing and functioning of high-energy

phosphates in biology.

In the process of building the case for this

equivalence of energizing carboxylates and

phosphates, a number of quotations will be

drawn from the literature rather than relying in

all cases on our interpretation of the literature.

In doing so, the case for high-energy phos-

phates resulting from limited hydration will

become very evident, and the energizing of

phosphates, as with the energizing of carboxy-

lates as evidenced by hydrophobic induced pKa

shifts,

becomes another illustration of con-

silience, that is, of a "common groundwork of

explanation."

In terms of the molecular process whereby

ATP forms and functions as biology's energy

coin, there are two aspects—the protonation/

deprotonation of carboxylates due to a proton

concentration gradient that drives a rotor to

form ATP from ADP and Pi, as in the ATP syn-

thase, and the molecular process whereby the

breakdown of ATP to ADP with release of Pi

drives the protein-based machines of biology, as

in the myosin II motor of muscle contraction.

8,1.11,1 Standard Free Energies of

Hydrolysis of Phosphate Bonds

In this section the standard free energy

of hydrolysis of ATP (and its equivalent

nucleotide triphosphates, NTPs) to ADP and

AMP and related biologically phosphorylated

molecules are noted.^'^The standard free energy

of hydrolysis of ATP to form ADP and Pi can

be obtained from the equilibrium constant for

the reaction

ATP ^ ADP-h Pi

to give the equilibrium constant, Keq,

Ke,-([ADP][PJ)/[ATP]

(8.1)

(8.2)

The reference conditions for the reaction must

be carefully defined and maintained, especially

the availability of water and the presence of

salts,

when making comparisons of different

high-energy compounds. From equilibrium

theory, Keq can be written in terms of the

change in Gibbs free energy AG for the

reaction

_^-AG/RT

eq ~ ^

(8.3)

348

8. Consilient Mechanisms for Protein-based Machines of Biology

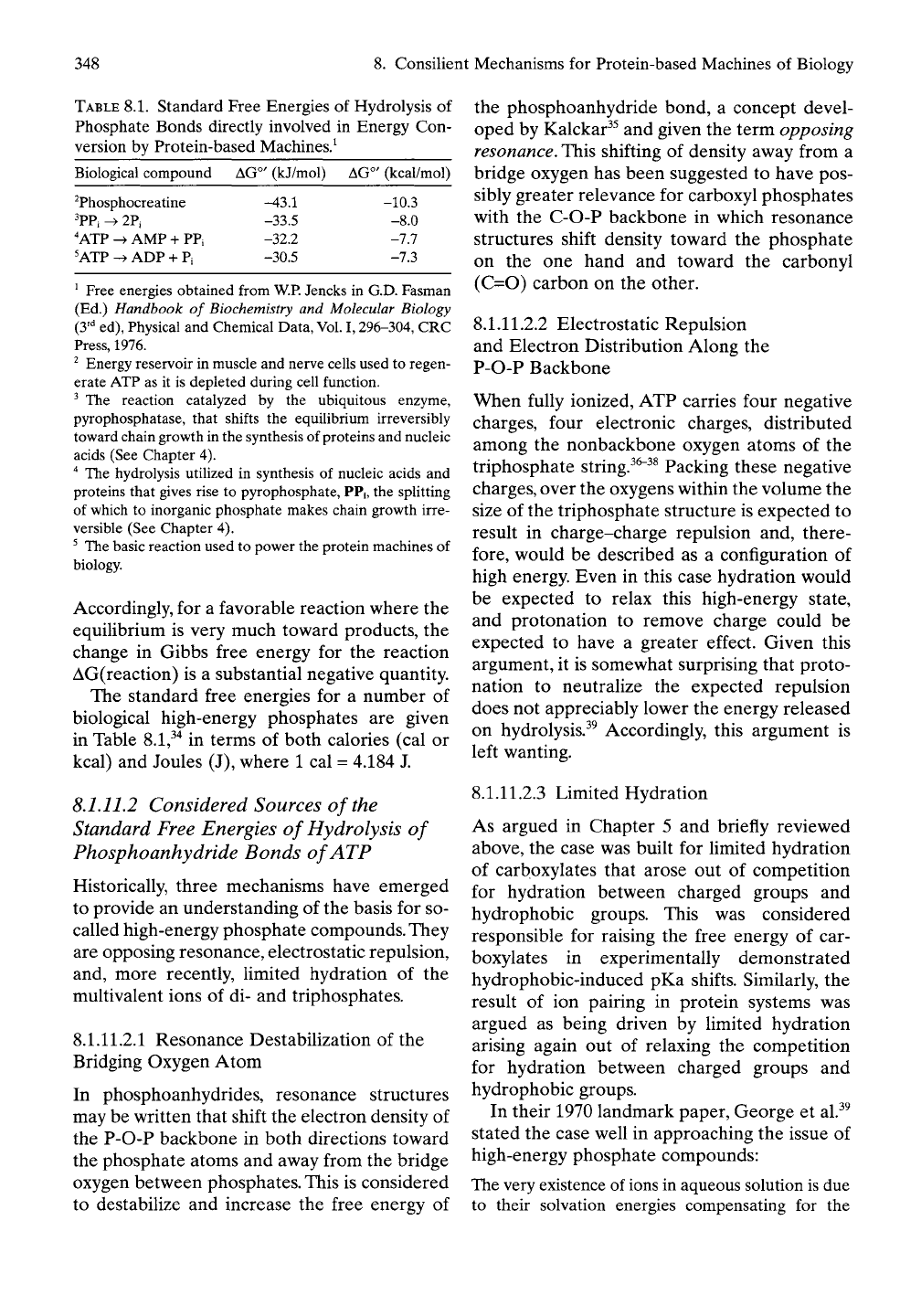
TABLE

8.1. Standard Free Energies of Hydrolysis of

Phosphate Bonds directly involved in Energy Con-

version by Protein-based Machines.^

Biological compound AG°' (kJ/mol) AG°' (kcal/mol)

^Phosphocreatine

^PPi ^ 2Pi

'ATP -^ AMP + PPi

'ATP -^ ADP + Pi

-43.1

-33.5

-32.2

-30.5

-10.3

-8.0

-7.7

-7.3

^ Free energies obtained from

W.P.

Jencks in G.D. Fasman

(Ed.) Handbook of

Biochemistry

and Molecular Biology

(y^ ed), Physical and Chemical Data,

Vol.

1,296-304, CRC

Press,

1976.

^ Energy reservoir in muscle and nerve cells used to regen-

erate ATP as it is depleted during cell function.

^ The reaction catalyzed by the ubiquitous enzyme,

pyrophosphatase, that shifts the equilibrium irreversibly

toward chain growth in the synthesis of proteins and nucleic

acids (See Chapter 4).

The hydrolysis utilized in synthesis of nucleic acids and

proteins that gives rise to pyrophosphate, PPi, the splitting

of which to inorganic phosphate makes chain growth irre-

versible (See Chapter 4).

' The basic reaction used to power the protein machines of

biology.

Accordingly, for a favorable reaction where the

equilibrium is very much toward products, the

change in Gibbs free energy for the reaction

AG(reaction) is a substantial negative quantity.

The standard free energies for a number of

biological high-energy phosphates are given

in Table

8.1,^"^

in terms of both calories (cal or

kcal) and Joules (J), where 1 cal = 4.184 J.

8J.11.2 Considered Sources of the

Standard Free Energies of Hydrolysis of

Phosphoanhydride Bonds of ATP

Historically, three mechanisms have emerged

to provide an understanding of the basis for so-

called high-energy phosphate

compounds.

They

are opposing resonance, electrostatic repulsion,

and, more recently, limited hydration of the

multivalent ions of di- and triphosphates.

8.1.11.2.1 Resonance Destabilization of the

Bridging Oxygen Atom

In phosphoanhydrides, resonance structures

may be written that shift the electron density of

the P-O-P backbone in both directions toward

the phosphate atoms and away from the bridge

oxygen between phosphates. This is considered

to destabilize and increase the free energy of

the phosphoanhydride bond, a concept devel-

oped by Kalckar^^ and given the term opposing

resonance.

This shifting of density away from a

bridge oxygen has been suggested to have pos-

sibly greater relevance for carboxyl phosphates

with the C-O-P backbone in which resonance

structures shift density toward the phosphate

on the one hand and toward the carbonyl

(C=0) carbon on the other.

8.1.11.2.2 Electrostatic Repulsion

and Electron Distribution Along the

P-O-P Backbone

When fully ionized, ATP carries four negative

charges, four electronic charges, distributed

among the nonbackbone oxygen atoms of the

triphosphate string.^^^^ Packing these negative

charges,

over the oxygens within the volume the

size of the triphosphate structure is expected to

result in charge-charge repulsion and, there-

fore,

would be described as a configuration of

high energy. Even in this case hydration would

be expected to relax this high-energy state,

and protonation to remove charge could be

expected to have a greater effect. Given this

argument, it is somewhat surprising that proto-

nation to neutralize the expected repulsion

does not appreciably lower the energy released

on hydrolysis.^^ Accordingly, this argument is

left wanting.

8.1.11.2.3 Limited Hydration

As argued in Chapter 5 and briefly reviewed

above, the case was built for limited hydration

of carboxylates that arose out of competition

for hydration between charged groups and

hydrophobic groups. This was considered

responsible for raising the free energy of car-

boxylates in experimentally demonstrated

hydrophobic-induced pKa shifts. Similarly, the

result of ion pairing in protein systems was

argued as being driven by Umited hydration

arising again out of relaxing the competition

for hydration between charged groups and

hydrophobic groups.

In their 1970 landmark paper, George et al.^^

stated the case well in approaching the issue of

high-energy phosphate compounds:

The very existence of ions in aqueous solution is due

to their solvation energies compensating for the

8.1 Thesis 349

large amount of energy required to disrupt the

crystal lattice in the dissolution of an ionic crystal, or

to break the covalent bond to hydrogen and bring

about electron transfer in the ionization of an acid.

... Hence even in the absence of values for the high

energy phosphate compounds the conclusion is

inescapable that at biological pH an important term

contributing to their thermodynamic reactivity will

be the difference between the solvation energies for

the multi-charged reactant and product ions.

Drawling from their thermodynamic data and

calculations on ATP, ADP and AMP hydroly-

ses,

George et al.^^ concluded that the source of

release of high energy on hydrolysis of ATP and

ADP is a Umitation of hydration in the reac-

tants,

for example, in ATP.

Almost two decades later Hayes et al."^^

concluded.

Although intramolecular (opposing resonance and

electrostatic) effects play an important role in deter-

mining the energy of hydrolysis in some of these

reactions, it is concluded that in those hydrolyses of

most importance in energy storage and transduction

(ATP -> ADP + orthophosphate, and phosphocrea-

tine + ADP -^ creatine + ATP), relative solvation

energies of reactant and products are by far the most

important factors in determining these energies.

From de Mies,^^

However, from the values of Table IV, it can be

inferred that a small change in the organization of

solvent around the molecules of reactants and prod-

ucts might easily lead to a significant change in the

thermodynamic parameters of a reaction.

This brings us directly to our argument that,

from these data Hayes et

al."^^

concluded that sol-

vation energies of reactants and products are by

far the most important factors in determining

the energies of hydrolysis of phosphate com-

pounds that are used for energy storage and

transfer in the living cell. The same conclusion

has been reached recently by Ewig and Van

Wazer"^^

w^ho

calculated the energy of hydrolysis

of pyrophosphate in the gas

phase.

Furthermore,

due to intramolecular competition for hydra-

tion, even greater effects of limited solvation

energy can be expected for the phosphoanhy-

drides of biology such as ATP and ADP that

also contain the adenine aromatic hydrophobic

group than for the inorganic phosphate prod-

ucts calculated by Ewig and Van Wazer."^^

8.1.11.3 Means of Energizing a Phosphate

Sufficient for Addition to ADP

8.1.11.3.1 Our View: Competition for

Hydration with Hydrophobic Moieties

Energizes Polar Species

From an analysis of the hydrophobic-induced

pKa and reduction potential shifts with the

associated destruction of hydrophobic hydra-

tion on formation of carboxylates (see Figure

5.25B), we argue that the solvation limitation

of phosphates can be made critical by the

competition for hydration between polar and

hydrophobic domains.

8.1.11.3.2 Analogy Between Formation

of High-energy Phosphate Bonds and

Hydrophobic-induced pKa Shifts:

The General Argument

The magnitude of this apolar-polar repulsive

free energy of hydration, when the polar

species is carboxylate, has been demonstrated

experimentally to approach the order of 40 kJ/

mole or 8 to lOkcal/mole. This would be suffi-

cient to raise the free energy of an inorganic

phosphate for addition to an ADP, and in

general to destructure hydrophobic hydration

and open hydrophobic folds.

8.1.11.3.3 Question: How is the Free Energy

of Phosphate Raised Sufficiently for Addition

to ADP with Formation of ATP?

The free energy of phosphate is raised by the

forced confrontation of the site containing

ADP and Pi (or R-Pi) with a hydrophobically

hydrated surface, as argued below for ATP

synthase.

8.1.11.3.4 Question: How Can the Binding of

ATP Drive a Conformational Change?

The binding of ATP to a site in sufficient prox-

imity to a spatially localized hydrophobic asso-

ciation can result in the separation of associated

hydrophobic domains. In the absence of ATP,

the site exhibits transient partial openings due

to normal thermal fluctuations. During such a

fluctuation, the build up of too much hydropho-

bic hydration causes the hydrophobic associa-

350

8. Consilient Mechanisms

for

Protein-based Machines

Biology

tion

re-form, because

the

Tt-divide

below

the operating temperature

for

that spatially

localized association.

during

the

fluctuation

ATP should become proximal,

the

incipient

hydrophobic hydration

destructured; there

no longer

driving force

for

closure,

and the

conformational change involving separation

associated hydrophobic domains occurs.

The

cross-bridge

of the

myosin

motor demon-

strates such features

its interaction with actin

and

in the

interaction

of the

head

of the

lever

arm with

the

amino-terminal domain

(see

Figures 2.17

and

8.53).

8.1.11.3.5 Question: Over What Distances

Can such Destruction

Hydrophobic

Hydration

Effective?

The destructuring

hydrophobic hydration

by polar species

is a

cooperative process. This

allows

the

opening

of a

hydrophobic associa-

tion that includes

ion pairing

proceed with

the result

adding

the

separated positive

and

negative charges

for

each

function

their

own right

polar species

for

disruption

further removed hydrophobic associations

in a

sort

propagating domino

unzipping effect.

Thus,

the

distance over which

the

destabilizing

of hydrophobic associations

can

occur depends

the

structure involved,

but

could readily

become several nanometers,

as is the

case

for

the GroEL/GroES chaperonin (see Chapter 7).

The specific distance would depend

on the

details

of the

distances between

the

newly

emerged charges

dissociation

of the ion-

pair-containing hydrophobic domains.

If we

consider

Mrad y-irradiation cross-linked

Model Protein

i of

Table

5.5,

(GVGVP

GVGVP GEGVP GVGVP GVGVP

GVGVP)39(GVGVP),

in the

contracted state,

the density

carboxyls

such that

the car-

boxyls

are

separated

more than 2nm.

By the

time 50% ionization

has

occurred,

the

volume

has increased 10-fold, the maximal allowed with

20 Mrad y-irradiation cross-linking. This occurs

with

mean distance between charges

than

4nm.

Contraction

due to

hydrophobic

association begins

carboxylates become less

than one every

nm. Thus the effect

a charged

carboxylate

can be

said

reach

out a

distance

of more than

2nm in its

capacity

destructure

hydrophobic hydration.

The

domino effect

separating ion pairs can

expected

increase

the distance further.

The multivalent phosphates, already signifi-

cantly limited

hydration,

can be

expected

reach

out

substantially further than carboxy-

lates

their search

for

adequate hydration.

This effect becomes enhanced when phosphate

access

water

limited. When

the

phosphate

occurs

at the

base

of a

cleft,

the

direction

for

access

water becomes severely limited

and

the thirst

for

hydration

can be

directed

by the

cleft

target sites

hydrophobic association.

In other words,

the

cleft functions

as a

conduit

to direct

the

thirst

for

hydration.

means

the cleft,

the

capacity

for

disrupting hydropho-

bic hydration

target sites

can be

boosted

effecting separation

ion pairs enroute, which

boost

the

polar species capacity

disrupt

hydrophobic hydration. Accordingly,

the use of

structure

direct

the

forces

apolar-polar

repulsion becomes

useful design feature

certain ATP-driven protein-based machines,

such

as the

myosin

motor.

8.1.11.3.6

The

Distance Issue

Can

Also

an Issue

Contact Area

In another context,

the

distance issue

can

become

matter

contact surface area

the hydrophobically associating hydrophobic

domains that

are

held together

by a

negative

AGHA- Even

the surface area

for

hydrophobic

contact were more than

nm^,

only sufficient

hydrophobic hydration would have

to be

destroyed during

transient fluctuation

cause

AGHA

become positive.

The

change from

negative to a positive AGHA

to shift from dom-

inantly associated

primarily dissociated.

8.1.11.3.7 Estimate

AGHA(P04

on Phosphorylation

Poly[30(GVGIP),(RGYSLG)]

As calculated

and

discussed

section 5.3.4.4.4,

the

AGHA(P04")

due to phosphorylation of

poly[30(GVGIP)(GRGDSP)]

at the Ser (S)

residue raises

the

value

of Tt to an

extent

that

the

calculated increase

free energy

for hydrophobic association becomes about

8.1 Thesis 351

^Moving the

Tt-divide

whatever means

AGHA (X) =

AHt (GVGIP) -

AHt

(GVGVP) (1)

where

is the interaction that changes AHt from that

the

reference polymer and

AGHA (%)

is the change

in Gibbs free energy for hydrophobic associatiob due

to the interaction.

K^l

for the following

phosphorylation

ATP

ATP +

poIy[30GVGIP),(RGYSLG)] =

ADP +

poly[30GVGIP)],(RGYS{PO4=} LG) (2)

Using the

slope,

ATVAGHA, defined by charged species

K +/OF-E-/OF of Figure 5-10 (6.8 cal/mol-pent/deg)

and ArKP04=) of 860°K allows an estimate of

AGHA

(P04=). Accordingly,

AGHA(P04=)

= 6.8x860 + 2.3 « 8

kcal/mol-pentamer

.*. Phosphorylation raised the free energy of the

insoluble (hydrophobically assocuated) state of the

model protein by

8 kcal/mol-P04~, i.e., polymer-

P04~

is in an energized state in the soluble, unfolded

model protein, as implied by K » 1.

FIGURE

8.3.

Independent estimates of

the

increase in

free energy on phosphorylation of poly[30(GVGIP),

(RGYSLG)].

8kcal/mole. Also, the equilibrium constant

for the enzymatic phosphorylation of

poly[30(GVGIP),(RGYSLG)] using ATP was

essentially one, which by Table 8.1 would be

essentially 7 to 8kcal/mole for hydrolysis of

the terminal phosphate of ATP under standard

conditions. This argument is laid out in

Figure 8.3. Accordingly, these experimental

results provided direct demonstration that the

serine-phosphate bond can be fully energized

by an increase in competition for hydration

with hydrophobic groups. From this, we con-

clude that the free energy of hydrolysis can be

a direct function of the presence of hydropho-

bic residues and that an increase in free energy

of phosphates can arise from a competition for

hydration.

8.1.11.4 Uses of ATP in Construction and

Maintenance of the Living Organism

The following is a partial list of the uses of ATP

or an energetically equivalent NTP: (1) to

produce the macromolecules nucleic acids, pro-

teins,

and polysaccharides; (2) to produce the

organelles and membranes (lipid biosynthesis);

(3) to pump ions across cell membranes against

concentration gradients to maintain the proper

electrochemical gradients across cell mem-

branes; (4) to transport nutrients into the cells

and remove waste; (5) to drive trafficking of

molecules, vesicles, and organelles (e.g., direc-

tional transport of Golgi, lysosomes, and

chloroplasts) within the cells; (6) to use molec-

ular chaperones to achieve proper protein

folding and proteasomes and cellusomes to

degrade spent protein; (7) to change supercoil-

ing (Type II topoisomerase) and correct errors

in nucleic acids; (8) to achieve motility as in cell

crawling, muscle contraction, the beating of

cilia and flagella, the actuation of hearing; (9)

to provide additional energy requirements that

include motor-dependent tasks in the cell such

as "mitosis and cytokinesis, the morphogenesis

of the endoplasmic reticulum, exo-, endo-, and

pinocytosis, the polarization of the egg, gastru-

lation, and even thought itself [neuronal

growth-cone motility and the axonal transport

of neurotransmitter-containing vesicles].'"^^

8.1.12

ATPases, Biology's Workhorse

Protein-based Machines, and AGap

8.1.12.1 The Disposition of ATP at Its

ATPase Binding Site

From the perspective of the consilient mecha-

nism and its functional component, AGap, the

most striking feature of ATPases derives from

the way in which ATP molecules attach to the

protein. ATP binds near an aqueous interface

of the protein. Even more telling is that the

ATP molecule orients such that the triphos-

phate tail resides at the aqueous interface. This

is where hydrolysis occurs. More revealing with

regard to mechanism, ATP often binds with its

phosphates at the edge of a water-filled cleft.

8.1.12.2 The Specific Case of the ATPase

of the Myosin II Motor

In particular, as a step in the functional cycle of

the myosin II motor, ATP binding occurs at a

water-filled cleft."^ In the scallop muscle, as

shown below, the water-filled cleft can be seen

to communicate in two directions. In one direc-

tion it communicates with the hydrophobic

352

8. Consilient Mechanisms for Protein-based Machines of Biology

association of an actin cross-bridge attachment

site.

In the other direction the water-filled cleft

communicates to the hydrophobic association

involving principally the head of the lever arm

and the amino-terminal domain of the cross-

bridge. In both cases the hydrophobic associa-

tions become disrupted on ATP binding. In the

first case binding results in detachment from

the actin filament. In the second case, by dis-

rupting the hydrophobic association between

the amino-terminal domain and the head of the

lever arm, ATP binding causes the cross-bridge

to reach toward its next actin site of attach-

ment. A credible mechanism requires that it

provide insight into these fundamental struc-

tural features of ATP binding to ATPases, in

general, and specifically to the ATPase that is

the myosin II motor.

By the hydrophobic consiUent mechanism

for the myosin II motor and specifically by

means of AGap, ATP binding effects both

hydrophobic dissociation from the actin

binding site and release of the hydrophobic

association at the head of the lever arm, allow-

ing the cross-bridge to move forward toward

the next attachment site. Of course, loss of

phosphate would reconstitute the hydrophobic

associations, that is, would effect hydrophobic

re-attachment to the actin binding site in

concert with re-association of the head of the

lever arm with the amino-terminal domain to

result in the powerstroke. Section 8.5.4 presents

crystal structure stereo views from which the

above-noted perspective derives.

8.1.12.3 Three Aspects of Force

Generation in ATPases

The concept of an apolar (hydrophobic)-polar

(e.g., charge) repulsive free energy of hydra-

tion, AGap, contributes to understanding the

mechanism whereby ATPases function in three

distinguishing respects. First and second, ATP

binding, and particularly on hydrolysis with for-

mation of ADP plus Pi, has the potential to

effect both "push" and "pull" components of

force. Third, release of

results in development

of an elastic "pull" component of force that is

most in evidence during isometric contractions.

These three elements of force development are

discussed immediately below.

8.1.12.4 Binding ATP Energizes a

Protein-based Machine by the Mechanical

Result of Hydrophobic Dissociation

Due to AGap

In reviewing their studies on the Fi motor of

ATP synthase, Oster and Wang"^"^ recently

stated, "In many motors, the force generating

step is associated with the binding of nucleotide

to the catalytic site. We propose that this is true

of all ATPases. In particular, for the Fi motor

this is the only way to reconcile all of the bio-

chemical and mechanical measurements with

its high mechanical efficiency." (The itaUcs are

the authors' own emphasis.) As presented here,

the hydrophobic consilient mechanism makes a

distinction between energizing and the use of

that energy in force generation. In our view, this

distinction is not so significant for the rotary

Fi motor of ATP synthase functioning as an

ATPase. In this case by the hydrophobic con-

siUent mechanism, ATP-driven rotation of the

rotor results from repulsion due to an increase

in AGap, the apolar-polar repulsive free energy

of hydration.

As discussed below in section 8.4.4.11, ATP

binding provides the major "push" component

of force, but we expect the peak in AGap to

occur at the moment of hydrolysis when the

charge concentration is greatest with the

momentary presence of both ADP and Pi. In

the synthesis function of the Fi motor of ATP

synthase, we expect that the maximum repul-

sion occurs between the most hydrophobic side

of the rotor and the ADP and Pi state and that

this maximal repulsion decreases on ATP for-

mation, which, in the consilient view, drives

ATP formation. Accordingly, because repulsion

is the force that drives the ATPase function of

the Fi motor and because repulsion drives rota-

tion, ATP binding would provide near-maximal

force generation, enhanced only at the moment

of hydrolysis to form ADP plus Pi.

8.1.12.5 Development of an Elastic "PuW

Component of Force Resulting from an

Apolar-Polar Repulsion on ATP Binding

On the basis of the consilient mechanism, and

AGap in particular, one can also consider the

development of elastic force on ATP binding to

8.1 Thesis 353

result from the damping of internal chain

dynamics by repulsion of otherwise more kinet-

ically free chains or loops. Insight into this

aspect of the consilient mechanism surfaces

with Model Protein v in Figures 1.4 and 5.31, as

considered in the associated discussions in

Chapters 1 and 5. Repulsion between the

negatively charged carboxylates and hydro-

phobic phenylalanine (Phe, F) residues results

in a repulsive free energy of 2.4kcal/mole-

carboxylate, as measured experimentally by the

residual pKa shift, after dissolution is complete.

In this case keeping the phenylalanine (Phe, F)

residues distant from the carboxylates of the

glutamate (Glu, E") limits torsion angles that

would otherwise be possible if the repulsion

were not present. This constitutes a damping of

internal chain dynamics. This repulsion limits

the freedom of backbone motion; it restricts

the backbone torsion angle combinations that

would bring carboxylates and phenylalanine

side chains too close. Such a restriction of acces-

sible torsion angles constitutes a decrease in

chain entropy that should be expressed as an

increase in elastic force between the beginning

and end points of the restricted chain segment.

If the 30mer chain segment were held at the

mean distance during repulsion, removal of the

repulsion, in this case protonation to form

-COOH, should relax the deforming force.

Based on the consilient interpretations of the

model protein data, the presence of a nega-

tively and multiply charged ATP separated by

water molecules from a nearby chain or loop

that contained hydrophobic residues would

repulse the hydrophobic groups, causing them

to reside at a greater distance and possibly in

the shadow of the backbone. This constitutes a

"deformation" that limits the freedom of

motion of the chain or loop.

Similarly, apolar-polar repulsion between a

highly charged group and very hydrophobic

side chains of a chain or loop of protein would

be expected to limit the freedom of rotation

about backbone bonds of sufficiently kineti-

cally free chain segment or loop. This increase

in AGap would decrease the number of states

accessible to the polymer and decrease the

entropy of the chain. The resulting develop-

ment of an elastic force in the chain segment

or loop of protein could be used to perform

mechanical work of changing protein structure.

The mechanism of elasticity would now take on

the description of force development due to "a

damping of internal dynamics by repulsion"

rather than by extension. In this specialized cir-

cumstance, ATP binding could provide a useful

force-generating step in an ATPase, also not

inconsistent with the suggestion of Oster and

Wang.''

Accordingly, reasoning from the consilient

mechanisms, release of phosphate has the

potential to relax the apolar-polar repulsion

and relax an entropic elastic deforming force.

Thus,

instead of an electrostatic repulsion, for

example, the less efficient charge-charge repul-

sion, there would be the apolar-polar repul-

sion, which is argued above in section

8.1.9.1

and shown in Figure 5.36 to be more than 10

times more efficient as a mechanism for chemo-

mechanical transduction. In our view, to be effi-

cient, a machine should avoid the frictional

losses inherent in latches, ratchets, and mechan-

ically driven camshafts. Entropic elastic chains

and through-solvent apolar-polar repulsive

forces provide for efficient interconversion of

chemical and mechanical energy. At present,

however, we have no example of this develop-

ment of elastic force in the myosin II motor.

Therefore, it stands as a potential source of

force development without example, as yet, in

the protein machines of biology.

8.1.12.6 Release of Pi Discharges on

Energized Protein-based Machine by the

Third Mechanical Result of a ''Puir

Component of Force Due to Re-

established Hydrophobic Association

When considering ATPases that function as

classic contractile linear motors, the distinction

between energizing and force generation

becomes much clearer. ATP binding energizes

by disrupting hydrophobic association, perhaps

usefully boosted at the moment of hydrolysis,

but then force generation does not occur until

release of Pi that allows hydrophobic associa-

tion of the contracted state to recur. This could

be thought of as a "pull" component of force

generation that under isometric conditions

expresses as the generation of an elastic force.

Thus,

ATP binding could be viewed as effecting

354

8. Consilient Mechanisms for Protein-based Machines of Biology

relaxation, whereas loss of ATP yields the ulti-

mate in contraction, approaching a rigor-like

state,

as noted in Chapter 7. Such would be the

case unless or until a sweUing pressure became

relevant.

Release of the y-phosphate from protein-

bound ATP, leaving bound ADP, restores much

hydrophobic association that existed in the

protein before ATP binding. Release of the y-

phosphate permits reconstitution of sufficient

hydrophobic hydration to drive hydrophobic

association. In the broad view of the consilient

mechanism, as regards ATPases, ATP binding

causes hydrophobic dissociation, and Pi and

ADP release re-establishes maximal hydro-

phobic association. In terms of the movable cusp

of insolubiUty, binding of the polar ATP mole-

cule raises the temperature of the movable cusp

of insolubility to give solubility, and the decrease

in polaritys on phosphate release lowers the

movable cusp of insolubility to re-establish the

insolubility of hydrophobic association

In the preceding discussion of ATPase types

of protein-based

machineSy

the hydrophobic and

elastic consilient mechanisms, by means of a

single operative component of the Gibbs free

energy of hydrophobic association, namely, that

of apolar-polar repulsion, have provided three

distinct contexts for force development. Thus, we

believe the consilient mechanisms will prove to

be fundamental to the function of the ATPases

of biology. As argued below, evidence emerges

for the underlying competition for hydration

between hydrophobic and polar (e.g., charged)

groups to be a basic element in the functioning

of key protein-based machines of biology.

8.2 Overview of Energy

Conversion in Biological

Systems

8.2.1 The Overall Energy

Conversions: Photosynthesis

and Respiration

The overall energy conversions of biology were

briefly considered in Chapter 2, the overview

chapter, and grossly represented in Figures 2.10

(for photosynthesis) and 2.11 (for respiration).

Here the statements of photosynthesis and res-

piration are given below for ready reference in

order to place in context the component energy

flows that sustain Life."*^"^^

Photosynthesis, in overview, uses light energy

from the sun to combine water and carbon

dioxide to produce carbohydrate and oxygen.

Respiration, in overview, is exactly the reverse

with the exception that, instead of an input of

light energy, the output is chemical energy, as

given in Figure 8.4 and considered below.

The light reactions themselves involve

energy steps almost 10 times greater than the

energy steps involved in the subsequent reac-

tions of photosynthesis and of respiration. In

general, there are many thousands of subse-

quent reactions, where the energy steps are 8 to

lOkcal/reaction or less. From this perspective,

the task of gaining a sense of what sustains Life

would seem to be overwhelming.

Fortunately, as will be discussed below for

respiration, there are only a few tens of reac-

tions in the oxidation of a glucose molecule to

produce 6(C02) and 6(H20). Most significantly,

there are but three products that constitute the

most immediate resulting chemical energy.

These are reduced nicotinamide adenine dinu-

cleotide (NADH, 10 molecules), reduced flavin

Photosynthesis:

The first step in biology's reversal of

the arrow of time

carbon dioxide + water

light energy

6{C02) 6(H20)

• carbohydrate + oxygen

(1) [C(H20)]6 6(02)

Respiration:

Animals and plants in the dark use the

chermical energy of respiration to create more diverse

and complex life forms.

carbohydrate + oxygen

[C(H20)]6 6(02)

* water

carbon dioxide + chemical energy (2)

6(H20) 6(C02)

But these remarkable chemical equations do not

explain how!

FIGURE 8.4. Photosynthesis and respiration repre-

sent very broad expressions of the energy conver-

sions that sustain Life.