Bhushan B. Handbook of Micro/Nano Tribology, Second Edition

Подождите немного. Документ загружается.

for some starting conﬁgurations, if the hydrogen atoms were held rigid as the upper surface slid over the

lower surface they would have passed directly above and below one another. Analysis of video sequences

of the dynamics revealed that as the hydrogen atoms in each surface approached or collided, they would

revolve around each other in the sliding plane. This motion resembled more of a “zigzag” pattern when

the opposing hydrogen atoms were not directly in line in the sliding direction at the start of the simulation.

This motion caused oscillations in the normal and frictional forces as a function of sliding distance (or,

alternatively, sliding time). The oscillations possessed the periodicity of the unit cell, and their magnitude

depended on the magnitude of the applied load (Figures 11.34a and b). Similar oscillations have been

observed experimentally using an AFM (Mate et al., 1987).

For all loads, the approach of opposing hydrogen atoms caused in increase in both the normal and

the frictional forces. As the hydrogen atoms moved past one another, both the normal and the frictional

forces decreased. At low loads, the hydrogen atoms passed by one another with ease. Thus, the frictional

force oscillated about zero, yielding values of average frictional force close to zero (Figure 11.34a). At

higher loads, it was more difﬁcult for the hydrogen atoms to pass by one another and they became “stuck.”

Once stuck, continued sliding of the upper lattice resulted in a linear increase in the frictional force as

a function of sliding distance until some critical force was reached. At the critical force the hydrogen

atoms “slipped” past one another and the frictional force dropped very sharply. This oscillatory, non-

symmetric shape of the frictional force curve has been observed both experimentally (Mate et al., 1987;

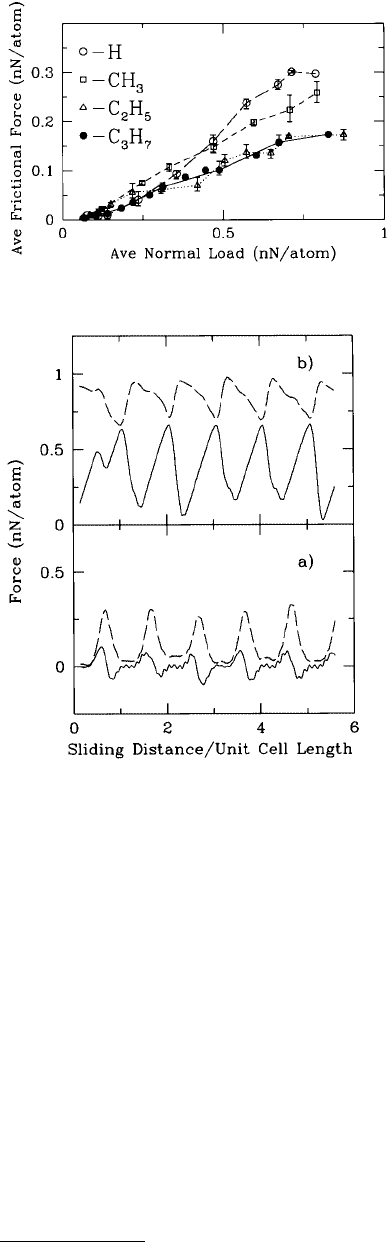
FIGURE 11.33 Average frictional force per rigid-

layer atom as a function of average normal load per

rigid-layer atom for sliding the upper surfaces shown

in Figure 11.32 in the [11

–

2] crystallographic direction

at 100 m/s and 300 K. The upper and lower lines drawn

from these points extend to the maximum and mini-

mum frictional force values obtained from indepen-

dent starting conﬁgurations. Thus, the lines represent

the range of frictional force values obtained at a given

load. Data points that do not have lines extending

above and below them were calculated from one sliding

simulation. (Data from Harrison et al., 1993c; Perry

and Harrison, 1997).

FIGURE 11.34 Frictional force (solid lines) and normal

force (dashed lines) per rigid-layer atom vs. sliding distance

when the system shown in Figure 11.32a is slid in the [11

–

crystallographic direction. The average normal and fric-

tional forces per rigid-layer atom are 0.11 and 0.0041

nN/atom in (a) (corresponding to a pressure of 1.4 GPa)

and 0.85 and 0.36 nN/atom (corresponding to a pressure

of 10.3 GPa) in (b). (Data from Harrison, J. A. et al. (1995),

Thin Solid Films 260, 205–211.)

German et al., 1993) and in other simulations (Glosli and McClelland, 1993; Landman et al., 1989a,b).

In all of these cases, this behavior was attributed to atomic-scale stick-slip or ratcheting.

The collisions suffered by the interface hydrogen atoms resulted in mechanical excitation of the

interface hydrogen atoms (and subsurface layer atoms) on opposing surfaces. Computation of the vibra-

tional energy between layers as a function of sliding distance conﬁrmed this behavior. The vibrational

energy between layers for the lower diamond lattice as a function of sliding distance typically shows

“ringing” of the surface bonds, followed by a propagation of this vibrational energy away from the surface,

until it is absorbed by the heat bath (Figure 11.35). This conversion of the work of sliding into heat

dissipated into the bulk was shown to be the mechanism of wearless atomic-scale friction.

When some of the hydrogen atoms on the upper surface were replaced with chemically bound methyl

groups, the average frictional force behaved in much the same way as for the hydrogen-terminated case.

In this case, the remaining hydrogen atoms, as well as the chemisorbed methyl groups, suffered collisions

with the hydrogen atoms on the lower surface. In addition to excitation of carbon–hydrogen bonds, the

methyl groups as a whole were able to rotate like turnstiles as they interacted with the hydrogen atoms

on the lower surface. This motion introduced a novel mechanism of energy dissipation, although one

that appeared to have little inﬂuence on the total amount of energy dissipated by the diamond lattices.

Extending the length of the chemisorbed hydrocarbon chains to ethyl and n-propyl caused a dramatic

reduction in the average frictional force at higher loads. The size and ﬂexibility of these larger hydrocarbon

chains, in conjunction with the conformation they adopted when sliding, were responsible for this

reduction. The carbon–carbon bond of a given ethyl group (or the chain backbone) was not typically

parallel to the sliding direction at the start of the simulation. However, under small applied loads sliding

usually induced alignment of the chain backbone with the sliding direction (Figure 11.36a). In this

conformation, both the hydrogen atoms and the ethyl groups underwent “collisions” with hydrogen

atoms on the lower surface. These collisions were similar to those that occurred in the hydrogen- and

methyl-terminated systems. Because atomic-scale friction is a direct result of the mechanical excitation

caused by these collisions, the friction was approximately the same as it was in the hydrogen-terminated

and methyl-terminated systems.

FIGURE 11.35 Average vibrational energy for each pair of oscillator atoms as a function of sliding distance. These

energies are derived from an MD simulation of two hydrogen-terminated, diamond (111) surfaces in sliding contact

(system shown in Figure 11.32a). The vibrational energy between the ﬁrst and second layers of the lower diamond

surface is shown in the lower panel, between the second and third layers in the middle panel, and between the third

and fourth layers in the upper panel. (From Harrison, J. A. et al. (1995), Thin Solid Films 260, 205–211. With

permission.)

In contrast, at higher applied loads the chains were not able to align when sliding, but rather were

forced to occupy potential energy valleys between the hydrogen atoms on the lower surface

(Figure 11.36b). This reduced the mechanical excitation of the system; thus, it reduced the friction force.

Recently, Sorensen et al. (1996) carried out simulation studies of atomic-scale friction for a number

of tip–surface and surface–surface contacts consisting of Cu atoms. One of the systems studied was

composed of a ﬂat tip of Cu atoms placed on a Cu(111) substrate. The tip consisted of stacked (111)

layers of Cu with 25 atoms (corresponding to a contact area of 140 Å

) touching the Cu substrate. The

interatomic potentials used to model the interactions between atoms were derived from effective medium

theory (EMT) (Jacobsen et al., 1987). Low-temperature (0 K) simulations were performed by moving

rigid atoms in the top of the tip 0.05 Å in the sliding direction, and then allowing the dynamic atoms

to relax by a local energy minimization procedure. MD simulations were used for 300 K investigations.

In these simulations, the rigid atoms were displaced in the sliding direction before each time step and

the temperature of the system was controlled by a Langevin thermostat.

When the Cu(111) tip was slid in the [

–

1] direction on the Cu(111) substrate at 0 K, the lateral force

on the tip exhibited the characteristic sawtooth pattern indicative of stick-slip motion. This behavior had

the periodicity of the substrate (2.6 Å), which has been observed in previous atomic-scale friction

simulations (Landman et al., 1989b; Harrison et al., 1992c, 1993a). There were two maxima in the friction

force per 2.6 Å unit cell, because the Cu tip atoms at the interface jumped discontinuously in a zigzag

pattern from fcc to hexagonal closest-packed positions on the Cu(111) substrate. At higher loads, the

slips occurred at larger displacements. As a result, the frictional force increased with increasing load.

Similar trends with increasing load have also been observed in other simulations (Harrison et al., 1992c).

Sorensen et al. (1996) also studied the effect of sliding velocity on the atomic-scale friction of contacting

Cu(111) surfaces. The time-dependent frictional force in this system was examined at temperatures of

both 12 and 300 K, and for sliding speeds of both 2 and 10 m/s. Superimposed on the sawtooth pattern

indicative of stick-slip motion were the random oscillations due to thermal ﬂuctuations of the system.

For both the low- and high-temperature 2-m/s sliding simulations, the ﬂuctuations in the frictional force

curve increased substantially after each slip event and decayed during the subsequent slick event. The

ﬂuctuation patterns were almost identical for each stick-slip event. These ﬂuctuations were too large to

be due entirely to thermal motion. Indeed, the authors attributed these ﬂuctuations to systematic motion,

FIGURE 11.36 The solid lines represent the center of mass trajecto-

ries of the –CH

portion of ethyl groups (Figure 11.32c) attached to a

hydrogen-terminated, diamond (111) surface as it slides over a hydro-

gen-terminated, diamond (111) surface. The trajectories are plotted

on a potential energy contour map of a hydrogen-terminated, dia-

mond (111) surface. Sliding corresponds to moving from the bottom

of the ﬁgure to the top of the ﬁgure. Trajectories that disappear from

the plots at large values of Y reappear at the bottom of the plot (near

Y = 0) due to periodic boundary conditions. Filled triangles represent

the starting points of individual trajectories and the dashed lines rep-

resent the path of the tethered portion of the ethyl group (–CH

–).

The closer the triangle is to the dashed line, the more aligned the

backbone of the ethyl group is in the sliding direction. The average

normal load per atom on the rigid-layer atoms of the upper surface

was 0.065 nN/atom in (a) and 0.42 nN/atom in (b). The contour values

are 0.271, 0.771, 1.271, 1.771, and 2.271 eV. (From Harrison, J. A. et al.

(1993), J. Phys. Chem. 97, 6573–6576. With permission.)

namely, the excitation of phonons. This observation is similar to the results found in the atomic-scale

friction of diamond (Harrison et al., 1995; Perry and Harrison, 1996b).

For the low-temperature 2 m/s sliding simulations, the authors concluded that almost all the energy

released after the slip event was dissipated by the thermostat during the long stick events. From the

magnitude of the ﬂuctuations in frictional force, it was clear that this was not the case when the sliding

velocity was increased to 10 m/s. In this case, the ﬂuctuations increased signiﬁcantly after the ﬁrst slip

and remained large for the remainder of the simulation. In this case, the stick event was shorter; therefore,

there was not sufﬁcient time for the energy to be dissipated by the thermostat. The result was that the

maximum values of frictional force were lower than they were at slower velocities. The implication was

that phonons excited at previous slips promoted subsequent slip events.

Sorensen et al. (1996) also investigated the effects of contact area, contact geometry (tip–surface system

vs. two contacting surfaces) and surface alignment on the atomic-scale friction.

Sliding friction at metallic interfaces has also been studied by Hammerberg et al. (1995) who investi-

gated Cu interfaces in sliding contact for pressures in the kilobar range. These two-dimensional MD

simulations contained 65,000 atoms, which are modeled with EAM potentials (Holian, 1991). The

simulations were carried out at a range of different densities and sliding velocities. The calculated

frictional force was found to be an increasing function of density, as expected from the adhesive model

of asperity interactions. In this model, microscopic asperities were in contact under the local load required

for mechanical stability at shear strains sufﬁcient to initiate plastic ﬂow. In addition, the simulations

showed that the coefﬁcient of friction was a weaker function of density in this pressure regime than the

adhesive model would predict. The friction coefﬁcient was also found to be a decreasing function of

velocity, and above a critical velocity the temporal behavior of the tangential force became oscillatory.

Above a critical velocity, the mechanical response of the underdamped stick-slip system scaled with time

and the structure of the interface changed. In this region, a band of nanocrystalline material (approxi-

mately 16 nm wide) formed that had a signiﬁcant dislocation density.

Boundary lubrication of close-packed hydrocarbon chains attached to rigid substrates has also been

studied using MD simulations (Figure 11.37) (Glosli and McClelland, 1993). The ﬁlms were modeled as

a periodic 6 × 6 array of monomer alkane chains with rigid-bond lengths. The CH

and CH

entities

were treated as united atoms (Ryckaert et al., 1978). The forces between pairs of united atoms on chains

more distant than third nearest neighbors and the forces for all interactions between chains were derived

from an LJ potential. Sliding was modeled by moving the substrate of the upper ﬁlms at a 2.2 m/s velocity

in the x-direction.

The frictional behavior of the ﬁlms as a function of interaction strength between chains on opposing

surfaces was investigated. The time average of the shear stresses τ/τ

, (where τ

= 15.6 MPa), given as the

FIGURE 11.37 Initial conﬁguration of the computational cell viewed from the top and from the side. The x-axis

is the sliding direction. Periodic boundary conditions are applied in the x- and y-directions. (From Glosli, J. N. and

McClelland, G. M. (1993), Phys. Rev. Lett. 70, 1960–1963. With permission.)

total frictional force divided by the area of the computational cell, was examined as a function of

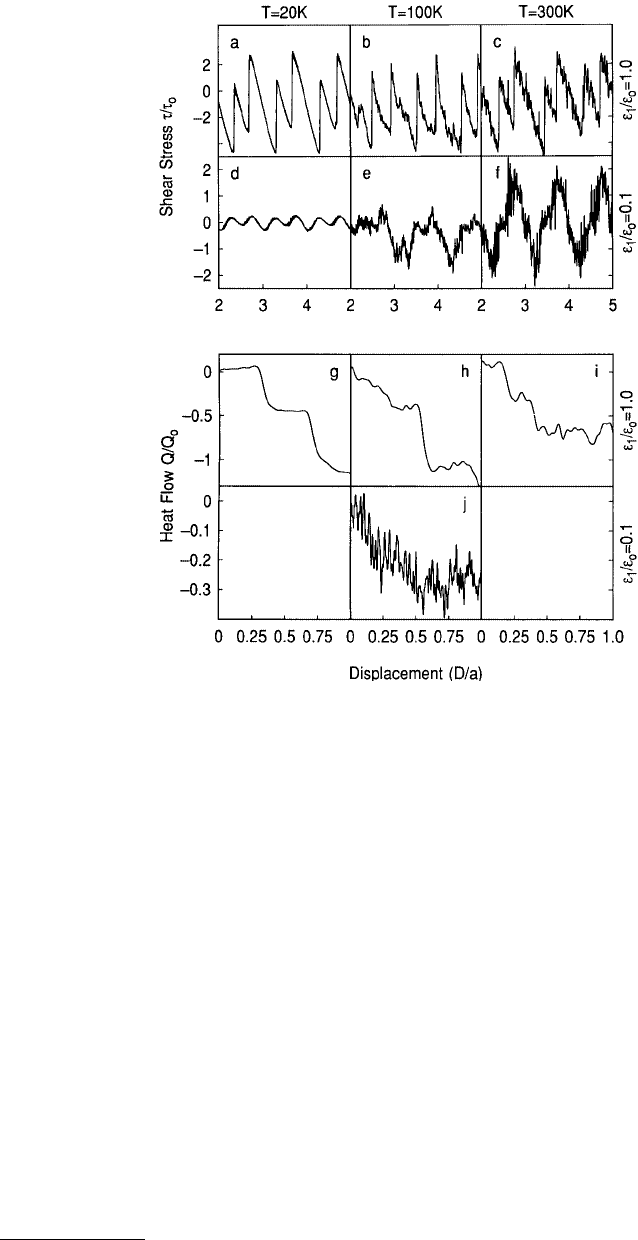
displacement at several temperatures (Figure 11.38). The shear stress possessed the characteristic saw-

tooth pattern associated with forces alternating between aiding and opposing the sliding motion. When

the force changed sign, the ends of the chains on each ﬁlm abruptly jumped 0.66 Å (a relative motion

of 1.11 Å). As a result, the two ﬁlms vibrated in synchronization in opposite lateral directions. The

vibrational excitation of the ﬁlms is apparent from a thickening of the line in Figure 11.38a. This effect

became more pronounced as the temperature of the system was increased (Figures 11.38b and c). The

heat ﬂow into the thermostat clearly showed that the pluck corresponded to the conversion of mechanical

energy, stored as strain, to thermal energy. The interaction strength had a profound effect on the dynamics

of these ﬁlms (Figure 11.38a and d). For small interaction strengths (Figure 11.38d), the lateral force

varied smoothly with position. Thus, a region of viscous dissipation existed. Indeed, at a simulation

temperature of 20 K, an interaction strength threshold to plucking of approximately 28 K was identiﬁed.

For larger interaction strengths and for temperatures below 70 K, friction resulted from the plucking

motion and showed little temperature dependence. Above 70 K the friction was strongly affected by

changes in the mechanical characteristics of the ﬁlm, examples of which include rotational melting and

FIGURE 11.38 Shear stress (a) to (f) and heat ﬂow (g) to (j) as a function of sliding displacement for normal

(ε

/ε

= 1.0) and reduced (ε

/ε

= 0.1) interfacial interaction strengths at three temperatures. In (a) to (f) the

horizontal scale extends over three lattice periods, in (g) to (j), over one period. In (h) and (i) the results for 10 and

20 cycles, respectively, have been averaged together to reduce thermal noise. The velocity v/v

equals 0.0112. Quantities

have been normalized by τ

= 15.6 MPa, a = 4.44 Å, and Q

= σ/a

= 6 mJ/m

. (From Glosli, J. N. and McClelland,

G. M. (1993), Phys. Rev. Lett. 70, 1960–1963. With permission.)

associated loss of the nearly herringbone structure near 118 K. The friction increased dramatically after

70 K, then declined steadily as the temperature was increased.

Molecular dynamics simulations have also been used to study the friction in monolayers of perﬂuo-

rocarboxylic acid and hydrocarboxylic acid on SiO

(Koike and Yoneya, 1996). This system was chosen

because AFM (Overney et al., 1994) and SFA (Briscoe and Evans, 1982) experiments have shown that

the friction force on ﬂuorocarbon-covered areas was three to ﬁve times larger than it was on hydrocarbon-

covered areas. In the simulations, surfaces of SiO

(001) were composed of 510 to 714 atoms and were

used both as the base and the slider. The monolayers were composed of 36 chains of C

COOH and

COOH adsorbed on the SiO

surface. A valence force ﬁeld was used to calculate the forces between

atoms (Dauber-Osguthorpe et al., 1988).

Computational cell sizes corresponded to areas of 18.4 and 22.1 Å

/molecule. These areas corresponded

to experimental surface pressures between 20 and 40 mN/m. The LB ﬁlms were close packed and

contained almost no gauche defects. The ﬁlms were equilibrated and then compressed at a constant

velocity of 100 m/s. The slider was then moved at a constant velocity of 100 m/s in the [010] direction

under a constant load of 0.60 nN.

Glosli and McClelland (1993) had previously observed two types of energy dissipation, resulting in

friction of bilayers, continuous or viscous dissipation and discontinuous or plucking dissipation. These

two types of dissipation were also observed in Koike and Yoneya’s 1996 simulations. For the sliding of a

gold surface on a long-chain thiol monolayer, Tupper and Brenner (1994b) found that the period of

oscillation in the frictional force corresponded to the distance between the gold atoms. In contrast,

because the SiO

slider was smooth compared with the in-plane interactions of the monolayer, Koike

and Yoneya (1996) found the period of the oscillations in the frictional force were consistent with the

periodicities of the tilt angles of the ﬁlms and potential energy changes in the two systems.

The average frictional force calculated for the C

COOH ﬁlm was approximately three times larger

than for the C

COOH. Thus, these simulations were in agreement with experimentally obtained

trends (Overney et al., 1994). The authors concluded that it is impossible to explain the differences in

frictional force using only the stiffness of the ﬁlms as has been suggested previously. Rather, the difference

in frictional force was largely due to differences in the intermolecular interactions. In addition, the

frictional force was found to be approximately proportional to the difference in the potential energy

ﬂuctuations between shear and equilibrium conditions.

Potential energy ﬂuctuations were also found to be important in earlier simulations of atomic-scale

friction (Harrison et al., 1995). These simulations showed that two hydrogen-terminated diamond sur-

faces in sliding contact were deformed in the stick phase of motion, and released this energy as heat after

the slip event. Thus, large amounts of energy were dissipated subsequent to sticking events, and the more

the surfaces deformed the more energy was dissipated.

Simulations of adsorbed monolayers of rare gas atoms are also found in the literature. These studies

typically make comparisons with quartz crystal microbalance (QCM) experiments. Early QCM experi-

ments by Krim and co-workers (Watts et al., 1990; Krim et al., 1991) examined the friction between

adsorbed gas atoms (Ar, Kr, Xe) and a (111)-oriented noble metal substrate (Au or Ag). Krypton adsorbed

on Au ﬁrst as islands of liquid. These islands grew in size until the gas atoms were adsorbed as an entire

monolayer. (This monolayer existed as a disordered, two-dimensional liquid at low doses.) For Kr, as

more atoms were adsorbed, the liquid became denser and eventually underwent a phase change into a

crystalline state that was incommensurate with the Au substrate. Krim and co-workers observed that

both the solid and liquid Kr monolayers exhibited a viscous force law, meaning that no threshold force

was needed to initiate the sliding and the friction was proportional to the relative velocity. This behavior

is more commonly associated with the macroscopic friction of liquids. In addition, Krim and co-workers

determined that the solid monolayers slid more easily than the liquid monolayers. That is, the frictional

force on the solid monolayer was less than the force on the liquid monolayer and the force on the liquid

monolayer was approximately three orders of magnitude weaker than that between Kr layers in a bulk

ﬂuid.

In an effort to understand these counterintuitive results, Robbins and co-workers (Cieplak et al., 1994;

Smith et al., 1996) performed MD simulations, in conjunction with perturbation theory, for a layer of

adsorbed molecules sliding over a substrate. The Au surface was modeled as a rigid substrate with atoms

“ﬁxed” in their ideal positions. This produced a ﬁxed periodic potential that acted on the adsorbate. The

interactions between the noble gas adsorbate atoms was modeled by an LJ potential with parameters, ε

and σ, appropriate for Kr. The potential proposed by Steele et al. (1973) was modiﬁed by addition of a

multiplicative factor f to model the adsorbate–substrate interactions. The multiplicative factor, f, allowed

the corrugation of the potential (its variation with the plane of the substrate) to be varied without

changing the energy of adsorption. Standard MD algorithms (Thompson and Robbins, 1990b) were used

to follow the position and velocity of all the atoms interacting with the aforementioned potentials.

These simulations showed that the form of the frictional law was dependent on the equilibrium phase

of the adsorbed layer (Figure 11.39). A static frictional force (i.e., nonzero frictional force when the

velocity equals zero) was only found when the adsorbed layer locked into a commensurate solid phase

and when f was sufﬁciently large. A viscous frictional law was found whenever the layer was in a ﬂuid

or incommensurate solid phase. (The crystalline monolayer was incommensurate due to the mismatch

between the atomic sizes of Kr and Au.) In addition, the friction was lower for the crystalline monolayer

in agreement with the ﬁndings of Krim and co-workers.

The slip time τ, which characterizes the rate of momentum transfer between the substrate and the

adsorbate, was also calculated in the MD simulations. (Lower values of τ imply larger friction.) Pertur-

bation theory was used to show that, for the symmetry of these simulations, τ was inversely proportional

both to the structure factor and to the phonon lifetime. The calculated values of τ increased with coverage

in the same way as the experimentally determined values. That is, τ had a small value in the ﬂuid phase

and a large value in the solid phase. In addition, the simulations showed that the order within the ﬁlm,

as measured by the structure factor, was responsible for the changes in τ with coverage. Thus, the

monolayer solid, because it is a rigid network of atoms that cannot deform itself readily to an external

potential, has a smaller degree of order and thus longer slip time (lower friction). Because a ﬂuid layer

could easily deform and lock itself to the substrate, inhibiting its motion, the degree of order is large,

and the slip time is small (larger friction).

The various examples discussed here — sliding diamond interfaces and self-assembled monolayers —

illustrate speciﬁc mechanisms where energy is dissipated at sliding interfaces resulting in a frictional

force. Furthermore, they show how variables such as temperature and interface structure can have a

profound effect on friction. As in the simulations of liquid lubrication discussed in the preceding section,

the insights being generated by these simulations will help to guide scientists and engineers interested in

designing materials with speciﬁc friction and wear properties. Clearly, though, much more can be done

to characterize and understand friction at nanometer-scale solid surfaces, and we expect simulations (in

conjugation with new experimental methods) to continue to play a leading role in developing this

understanding.

FIGURE 11.39 Variation of the steady-state velocity v with force

per atom F. Results for the incommensurate case that models Kr on

Au (ε' = 1.19ε, σ' = 1.19σ, N

= 49, and N

surf

= 0.068 Å

) at

T/ε = 0.385 (solid phase) and 0.8 (ﬂuid phase) are shown by open

and ﬁlled circles, respectively. Results for ε' = ε, σ' = σ = 2

1/6

T/ε = 0.385, and N

= 64 are shown by the squares. For f = 0.3

(ﬁlled squares) the adsorbate is a commensurate solid, and for f =

0.1 (open squares) the adsorbate is incommensurate. (From Smith,

E. D. et al. (1996), Phys. Rev. B 54, 8252–8260. With permission.)

11.5.2 Friction in the Presence of a Third Body

Free particles at an interface can have effects on friction that are just as remarkable as the effects of surface

roughness or of an adsorbed monolayer. This is exempliﬁed by the results of Perry and Harrison (1996a,

1997) who used MD simulations to investigate the atomic-scale friction and wear when small hydrocarbon

molecules are trapped between two (111) crystal faces of diamond. These trapped molecules, sometimes

referred to as third-body molecules, might model hydrocarbon contamination trapped between contact-

ing surfaces prior to a sliding experiment. Alternatively, these molecules might model hydrocarbon debris

formed when two diamond surfaces are in sliding contact.

In separate studies, three simple hydrocarbon molecules were trapped between the hydrogen-termi-

nated (111) faces of two diamond surfaces and atomic-scale friction was measured as a function of normal

load. The third-body molecules examined were methane (CH

), ethane (C

), and isobutane

((CH

)

CH) (Figure 11.40). The sliding simulations were carried out as described in Section 11.5.1.

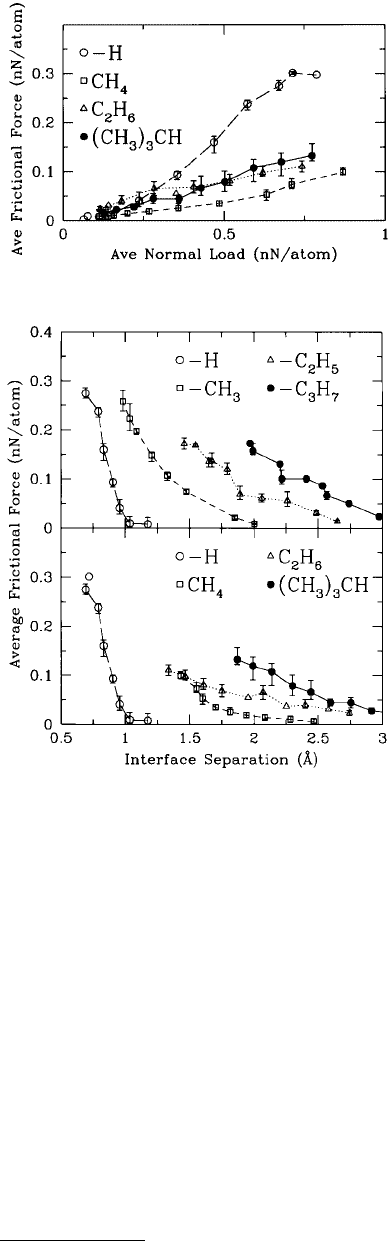
Examination of the average frictional force as a function of load revealed that for all three systems the

frictional force generally increased as a function of load (Figure 11.41). However, the presence of each

of the third-body molecules markedly reduced the average frictional force compared with the hydrogen-

terminated diamond (111) system in the absence of third-body molecules. The methane system exhibited

the lowest frictional force, while the ethane and isobutane systems had somewhat larger frictional force

values over the load range examined, although still lower than the bare surface.

The third-body molecules reduced the frictional force by acting as a boundary layer between the two

diamond surfaces (Figure 11.42). The interfacial separation, hence, average distance between hydrogen

atoms on opposing diamond surfaces, was always larger in the presence of the third-body molecules.

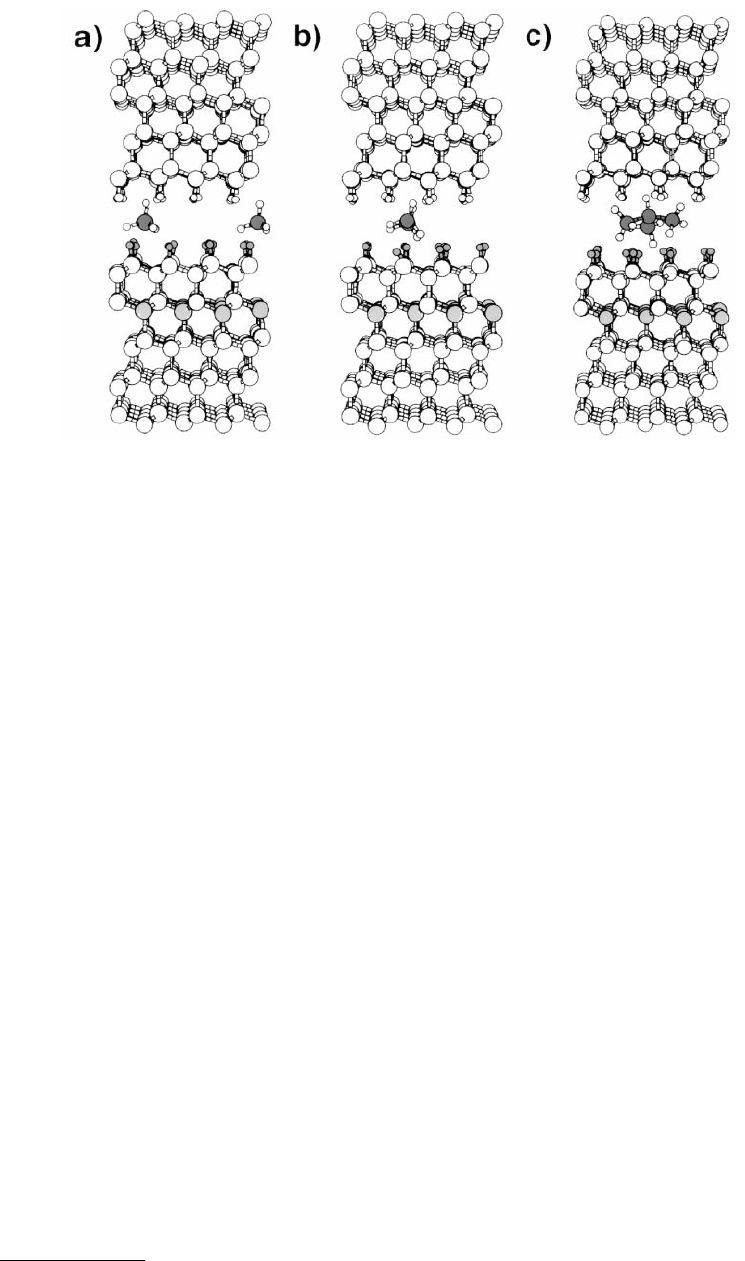
FIGURE 11.40 Initial conﬁgurations at low load for the diamond plus third-body molecule systems. These systems

are composed of two diamond surfaces, viewed along the [

–

110] direction, and two methane molecules in (a), one

ethane molecule in (b), and one isobutane molecule in (c). Large white and dark gray spheres represent carbon

atoms of the diamond surfaces and the third-body molecules, respectively. Small gray spheres represent hydrogen

atoms of the lower diamond surface. Hydrogen atoms of the upper diamond surface and the third-body molecules

are both represented by small white spheres. Large light gray spheres represent fourth-layer carbon atoms of the

lower diamond surface. Sliding is achieved by moving the rigid layers of the upper surface from left to right in the

ﬁgure. (From Perry, M. D. and Harrison, J. A. (1997), J. Phys. Chem. 101, 1364–1373. With permission.)

Thus, the third-body molecules caused a reduction in the interaction of hydrogen atoms on opposing

diamond surfaces. The principal mechanism of energy dissipation in the system was the interaction of

the third-body molecules directly with the diamond surfaces. Because the submonolayer coverage of

third-body molecules meant that they did not interact with all of the surface hydrogens, the total

vibrational excitation of the surfaces was less in the presence of these molecules. The larger surface area

of ethane and isobutane molecules caused them to collide with a greater number of surface hydrogens,

thereby increasing the friction.

A detailed description of the interaction of the third-body molecules with the diamond surfaces

depends on their size, their shape, and the alignment of the opposing diamond surfaces. The spherical

shape and small size of the methane molecules allowed them to tumble between the diamond surfaces

traversing regions of low potential energy between hydrogen atoms on opposing diamond surfaces (Perry

and Harrison, 1996a,b). This led to very little vibrational excitation of the hydrogen atoms terminating

the diamond surfaces (Figure 11.43). The methane molecules underwent this sort of motion regardless

of load or diamond–surface alignment. Thus, the frictional force was low for all loads examined and the

range of frictional force values obtained at a given load was small (Perry and Harrison, 1997).

In contrast, the larger ethane and isobutane molecules suffered frequent collisions as they slid between

hydrogen sites on the diamond surfaces. In addition, because these molecules had nonspherical shapes,

FIGURE 11.41 Average frictional force per rigid-

layer atom as a function of average normal load per

rigid-layer atom for the third-body systems shown in

Figure 11.40 while sliding the upper diamond surface

in the [11

–

2] crystallographic direction. Open squares,

open triangles, ﬁlled circles, and open circles represent

data for the methane (CH

) system, the ethane (C

)

system, the isobutane ((CH

)

CH) system, and dia-

mond surfaces in the absence of third-body molecules,

respectively. Lines have been drawn to aid the eye.

(Data from Perry, M. D. and Harrison, J. A. (1997), J.

Phys. Chem. 101, 1364–1373.)

FIGURE 11.42 Average frictional force per rigid-

layer atom as a function of interface separation for the

third-body systems shown in Figure 11.40 while slid-

ing the upper diamond surface in the [11

–

2] crystallo-

graphic direction. The symbols are the same as in

Figure 11.41. Lines have been drawn to aid the eye.

(Data from Perry, M. D. and Harrison, J. A. (1997), J.

Phys. Chem. 101, 1364–1373.)

several initial conﬁgurations of the molecules relative to the sliding direction were possible. The effects

of initial molecular orientation, size of molecule, and load on frictional force were ascertained by

examining frictional force as a function of sliding distance (a so-called friction trace) for individual

simulations (Figure 11.44).

Each maximum and minimum in the friction trace was assigned to speciﬁc atomic-scale motions. The

shapes of these extrema were indicative of the type of atomic-scale motion and thus the amount of

interaction with the diamond surfaces. At low loads, the ethane molecule typically began the simulations

with its C–C bond at a 45° angle to the sliding direction (Figure 11.44, bottom panel). In this conﬁgu-

ration, the ethane molecule “ﬁts” well between hydrogen atoms on the lower diamond surface and

therefore interacted weakly with the surface. During sliding, the ethane molecule was able to adopt

conﬁgurations similar to this throughout the course of the simulations. As a result, the friction trace was

periodic and regular and the average frictional force was low.

At high loads, however, it became more difﬁcult for the ethane molecule to orient its C–C bond at

45° to the sliding direction during sliding. Indeed, the friction trace became irregular, indicating the

presence of many different conﬁgurations during sliding. Analysis of animated sequences of the simula-

tions also conﬁrmed this conclusion. These conﬁgurations led to additional interactions with the diamond

surfaces and increased frictional force. The number of conﬁgurations adopted during sliding depended

on the relative alignment of the opposing diamond and the starting orientation of the molecule in addition

to the load. For example, when the ethane molecule began the simulation with its C–C bond parallel to

the sliding direction, its interactions with the diamond were much different than when the initial

orientation was 45° to the sliding direction (Figure 11.44, top panel).

Because the isobutane molecule is also nonspherical, it had a number of possible starting conﬁgurations

(Figure 11.45). These different starting conﬁgurations led to different interactions with the opposing

diamond surfaces during sliding. The different types of interactions experienced by the isobutane mol-

ecule in the arrow vs. the sled orientation (Figure 11.45) are apparent from examination of the friction

traces shown in Figure 11.46. In addition, for the same starting conﬁguration, the interaction with the

FIGURE 11.43 Average vibrational energy between the (C–H) bonds of the upper hydrogen-terminated, diamond

(111) surface as a function of sliding distance for the hydrogen-terminated (H) (Figure 11.32a) and methane (CH

)

(Figure 11.40a) systems. For the hydrogen-terminated system, the average normal load and average frictional force

were 0.79 nN/atom and 0.32 nN/atom, respectively. For the methane-debris system, the average normal load and

the average frictional force were 0.85 nN/atom and 0.094 nN/atom, respectively. (From Perry, M. D. and Harrison,

J. A. (1996), Thin Solid Films 290/291, 211–215. With permission.)