Blank B.E., Krantz S.G. Calculus: Single Variable

Подождите немного. Документ загружается.

GENESIS

DEVELOPMENT5

Archimedes

The Greek geometer Eudoxus (ca. 408ca. 355 BCE)

used the limiting process to rigorously demonstrate area

and volume formulas that Democritus (ca. 460ca. 370

BCE) had discovered earlier. The arguments that

Eudoxus introduced came to be known as the Method of

Exhaustion. In the hands of Archimedes, this method

became a powerful technique.

In one of his earliest works, Archimedes derived a

formula for the area of a parabolic sector. An arc

AVB

of a parabola is drawn in Figure 1. The point V is

known as the vertex of the segment and is character-

ized as follows: Among all line segments from the chord

AB to the parabolic arc

AB that are perpendicular to

chord

AB, the one terminating at V is longest. Archi-

medes demonstrated that the area A enclosed by

line segment

AB and parabolic arc

AVB is 2hb/3, or

(4/3) 3 jΔAVBj (using the notation |R|todenotethe

area of a planar region R). The introduction of V creates

two parabolic subarcs,

AV and

VB.Eachhasavertex;

these vertices are labelled V1andV2inFigure2a.

Archimedes proved from a few easily derived geometric

properties of the parabola that

jΔAV

Vj1 jΔVV

Bj5

jΔAVBj:

At the next iteration, illustrated in Figure 2b, Archi-

medes interposed four new vertices V

, V

,andV

and concluded that

jΔAV

j1 jΔV

Vj5 j

ΔAV

Vj and

jΔVV

j1 jΔV

Bj5

jΔVV

Bj:

Combining these two equalities yields

7ΔAV

7 1 7ΔV

V7 1 7ΔVV

7 1 7Δ V

ð7ΔAV

V7 1 7ΔVV

B7Þ5

7ΔAVB7:

Continuing, Archimedes “exhaust ed” the pa rabolic

segment by a sequence {R

} of regions composed of

triangles: R

5 ΔAVB; R

5 ΔAV

V,ΔVV

B; R

ΔAV

,ΔV

V,ΔVV

,ΔV

B; R

,....In

general, |R

| 5 4

n

|. Using the formula for the sum

of a geometric progression, Archimedes obtained

,,R



1 1



3 jΔAVBj



1

N11



jΔAVBj:

He ﬁnished this proof with an airtight argument

that 1=4

N11

-0andA5 4jΔAVBj=3.

The Method

The translation of Archimedes’ works into Latin in the

late 1500s introduced European mathematicians to the

power of the inﬁnite process. Although these mathe-

maticians found elegant formulas, rigorous proofs, and

glimpses of what might be attained, they did not ﬁnd

the methods by which Archimedes discovered his

results. The 17th-century mathematician John Wallis

even protested that Archimedes “covered up the traces

of his investigations, as if he has grudged posterity the

secret of his method of inquiry.” So the matter stood

for 200 more years.

m Figure 1 Archimedes proved that the

area of the parabolic segment AVB is

equal to (2/3) hb

m Figure 2a Vertex V creates two

parabolic segments with vertices V1

and V2.

m Figure 2b Repeat the process of

adding vertices.

465

In 1906, Danish scholar Johan Ludvig Heiberg

learned of a manuscript that had be en catalogued in a

cloister in Constantinople (now Istanbul). The manu-

script contained a religious text of the Eastern

Orthodox Church and was a so-called palimpsest —a

parchment of which the original script had been washed

and overwritten. Because it was reported that the ori-

ginal mathematical text had been imperfectly washed,

Heiberg voyaged from Copenhagen to Constantino ple

to investigate. What Heiberg uncovered was a mathe-

matical manuscript containing numerous works of

Archimedes. It had been copied in Greek in the 10th

century only to be washed and written over in the 13th

century. As luck would have it, most of the original text

could be restored.

Heiberg’s chief ﬁnd was the Method Concerning

Mechanical Theorems, dedicated to Eratosthenes.

Scholars had known from the references of later Greek

mathematicians that such a work had existed until at

least the 4th century

CE but, until Heiberg’s discovery,

the text was presumed to be lost. In the Method, we

ﬁnd, to use the words of Wallis, Archimedes writing for

posterity:

Archimedes to Eratosthenes, Greeting: . . . since I

see that you are an excellent scholar . . . and lover of

mathematical research, I have deemed it well to

explain to you and put down in this same book, a

special method whereby the possibility will be

offered to you to investigate any mathematical

question by means of mechanics . . . . [I]f one has

previously gotten a conception of the problem by

this method, it is easier to produce the proof than to

ﬁnd it without a provisional conception. . . . [W]e

feel obliged to make the method known partly . . . in

the conviction that there will be instituted thereby a

matter of no slight utility in mathematics.

Theory of Indivisibles

The ﬁrst half of the 17th century saw a number of

attempts to use inﬁnite processes in the computation of

areas, volumes, and center s of gravity. Among the most

important of the early contributors were Pierre de

Fermat and Gilles Rober val in France, Bonaventura

Cavalieri and Evangelista Torricelli in Italy, and John

Wallis and Isaac Barrow in England. In 1635, Cavalieri

introduced his Theory of Indivisibles, a relatively

effective albeit cumbersome procedure for calculating

areas and volumes. Using his method, Cavalieri

became the ﬁrst to show that

dx 5 1=ðn 1 1Þ for

natural numbers n. Although Cavalieri’s student

Evangelista Torricelli became the foremost proponent

of the Theory of Indivisibles, Torricelli believed that

Cavalieri had only rediscovered a method that Archi-

medes must have known:

I should not dare conﬁrm that this geomet ry of

indivisibles is actually a new discovery. I should

rather believe that the ancient geometers availed

themselves of this method in order to discover the

more difﬁcult theorems, alt hough in their demon-

stration they may have preferred another way, either

to conceal the secret of their art or to afford no

occasion for criticism by invidious detractors.

Fermat and the Integral Calculus

The extension of Cavalieri’s formula

dx 5

1=ðn 1 1 Þ from natural numbers to rational values of n

was obtained independently by Fermat and Torricelli.

Fermat’s clever demonstration runs as follows: Given

natural numbers p and q 6¼0, let f (x) 5 x

p/q

. Fix a

number r 2(0, 1), and set x

5 r

for k 5 0,1,2,....The

points {x

} serve to (nonuniformly) partition [0, 1] into

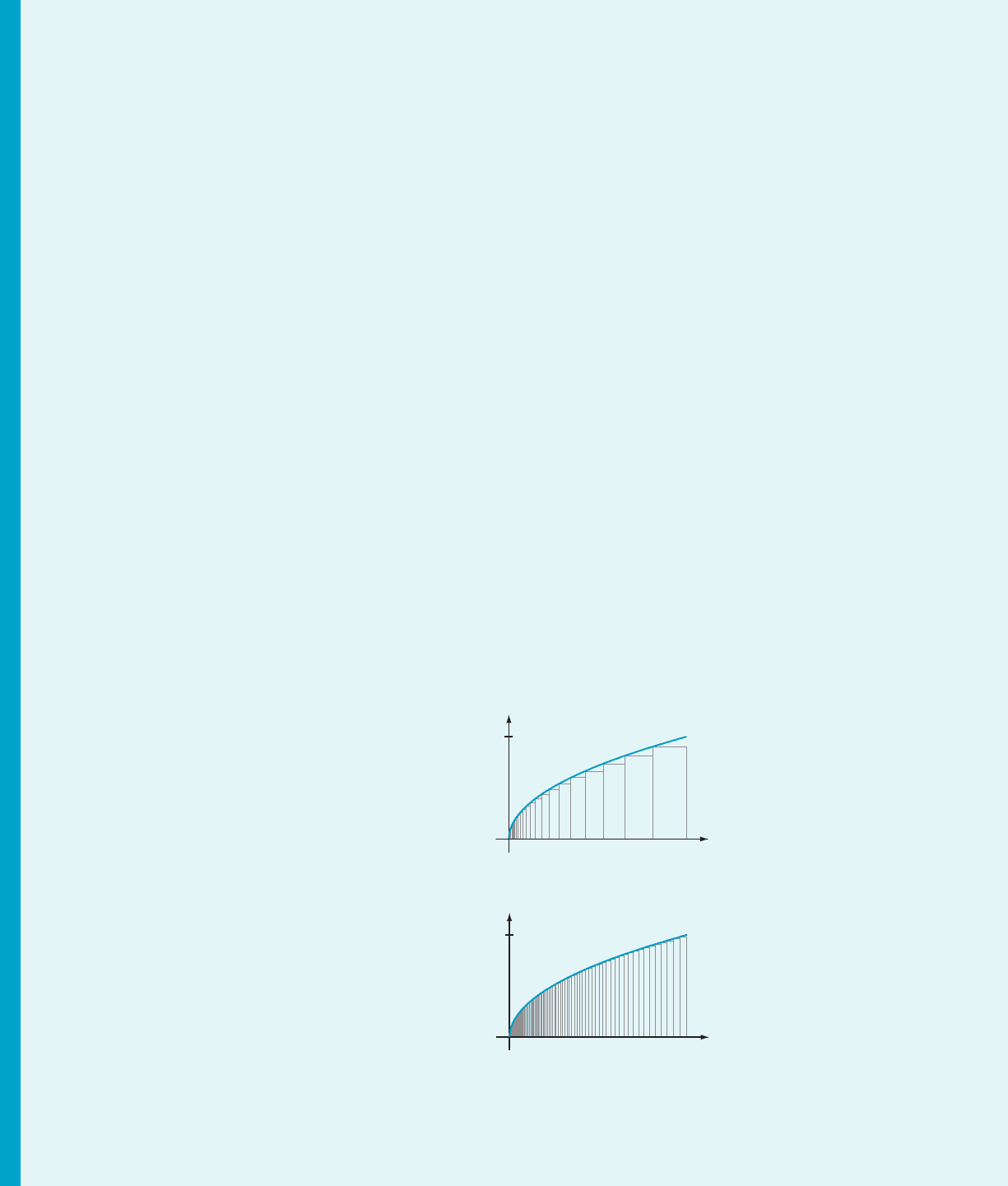
inﬁnitely many subin tervals. Fermat’s idea was to use the

sum

k5 0

f ðx

Þðx

 x

k11

Þ to approximate the area

under y 5 x

p/q

for 0 # x # 1. Figure 3a shows the

approximation for r 5 0.9. By taking a larger value of r

(with r still less than 1), we obtain a better approximation

(see Figure 3b). In the limit, we obtain the exact area:

y  x

p/q

r  0.98

m Figure 3b

y  x

p/q

r  0.9

m Figure 3a

466 Chapter

5 The Integral

f ðxÞdx 5 lim

r-1



lim

N-N

k5 0

f ðx

Þðx

 x

k11

Þ:

For f (x) 5 x

p/q

, we calculate

p=q

dx 5 lim

r-1



lim

N-N

k5 0

ðr

p=q

ðr

 r

ðk11Þq

5 lim

r-1



lim

N-N

k5 0

ð1  r

5 lim

r-1



ð1  r

Þ lim

N-N

k5 0

ðp1qÞk

Because 0 , r

p 1 q

, 1, we may apply formula (2.5.3)

to obtain

p=q

dx 5 lim

r-1



1  r

p1q

Although we know how to compute the inde-

terminate form on the right by using l’Ho

pital’s Rule,

Fermat had to resort to the following tricky backward

application of formu la (2.5.2):

p=q

dx 5 lim

r-1



1  r

p1q

5 lim

r-1



ð1  r

Þ=ð1  rÞ

ð1  r

p1q

Þ=ð1  rÞ

lim

r-1

q1

k5 0

lim

r-1

p1q1

k5 0

p 1 q

p=q 1 1

Kepler and Roberval

Before the discovery of the Fundamental Theorem of

Calculus, many mathematicians succeeded in evaluat-

ing particular integrals by ad hoc methods. For exam-

ple, in his last great astronomical work, the Epitome

Astronomiae Copernicanae (16181621), Kepler cal-

culated what we would now call a limit of Riemann

sums for

sinðtÞdt. In effect, Kepler discovered the

formula

sinðtÞdt 5 1  cosðβÞ:

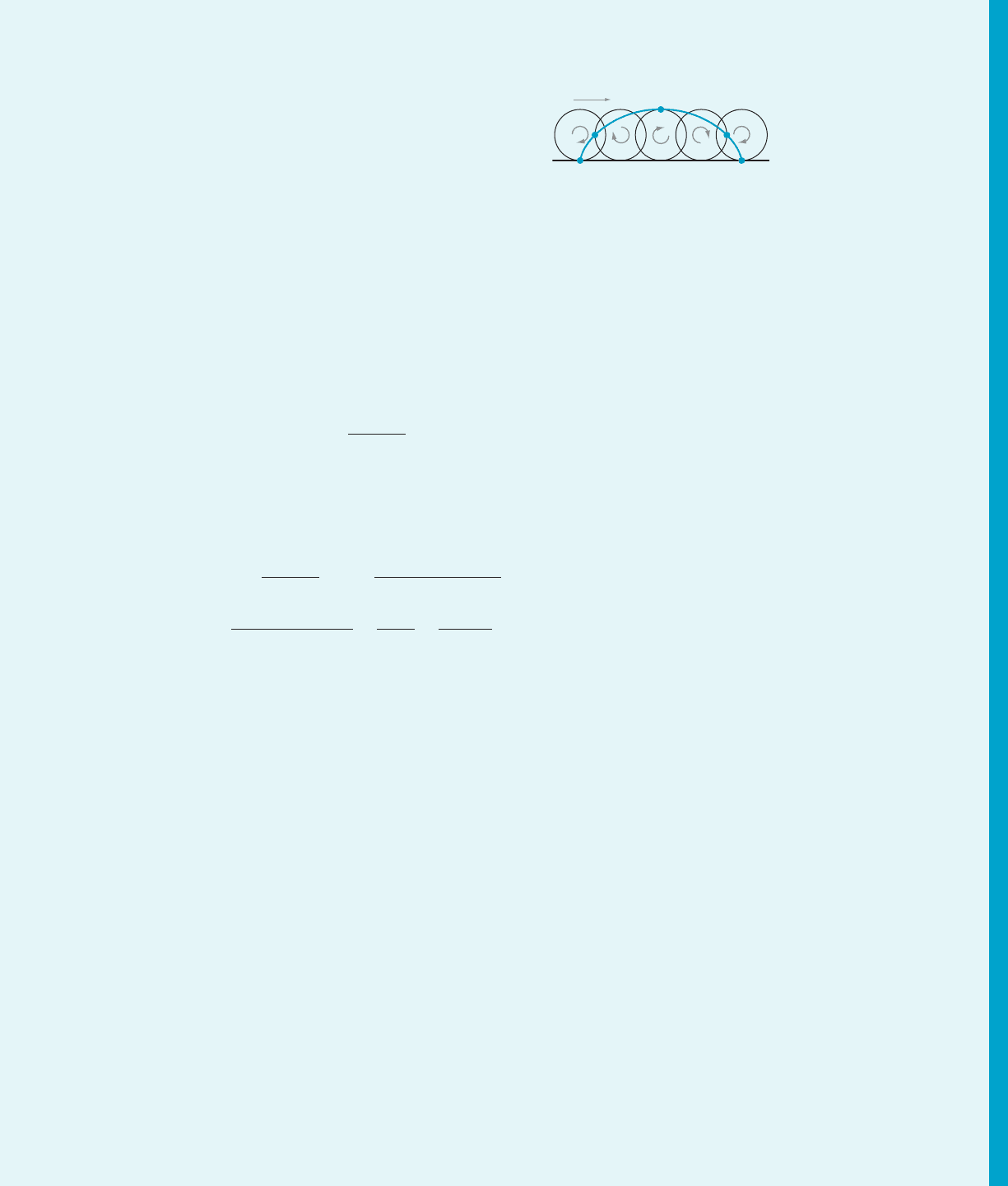
One curve that attracted the attention of promi-

nent mathematicians throughout the 17th century was

the cycloid, which is the locus of points generated by a

ﬁxed point on a circle as the circle rolls along a line at

constant speed without spinning or slipping (see Figure

4). Early in the 17th century, Galileo conjectured that

the area under one arch of the cycloid is three times the

area of the rolling circle. To reach this conjecture, this

leading scientist resorted to cut-out paper ﬁgures. By

1636, Roberval was able to rigorously verify Galileo’s

conjecture. Roberval’s work is a measure of how much

progress had been made in a very short period of time.

Newton and the Fundamental Theorem of

Calculus

Sir Isaac Newton’s ﬁrst contact with higher mat he-

matics came as a college senior in 1664 when he was 21

years old. He learned the tangent problem from the

eom

etrie of Descartes, and he learned Cavalieri’s

Theory of Indivisibles from Wallis’s Arithmetica Inﬁ-

nitorum. It was Wallis’s work that, in the winter of

16641665, led Newton, who was still an under-

graduate, to evaluate the area of the region unde r the

graph of y 5 (1 2 t

)

and over the t-interval [0, x]. In

doing so, he had already taken the decisive step of

letting the endpoint of integration be a variable, a key

idea of the Fundamental Theorem of Calcul us.

When plague closed Cambridge University in 1665

and 1666, Newton returned home to reorganize his

discoveries. He wrote his discoveries in “The October

1666 Tract on Fluxi ons,” a manuscript that was not

published until 1967. It was in this tract that Newton

ﬁrst stated both parts of the Fundamental Theorem of

Calculus. This remarkable manuscript also contains

several other theorems, including the Chain Rule and

Integration by Substitution.

Notation

The notation that we use today for the indeﬁnite inte-

gral was introduced by Gottfried Wilhelm Leibniz

(16461716) in a manuscript of 1675. At that time,

Leibniz did not include the differential. For example,

he wrote

5 x

=3. By the time his work appeared in

print in 1686, however, Leibniz included the differ-

ential in his notation and stressed its importance. The

modern notation

f ðxÞdx for the deﬁnite integral—

that is, the positioning of the limits of integration on the

integral sign—was introduced in 1822 by Joseph

Genesis & Development 467

m Figure 4 A point on a rolling

circle generates a cycloid.

Fourier (17681830). It was Fourier’s work that indir-

ectly led to the Riemann integral. Fourier’s study of

heat transfer required the evaluation of deﬁnite inte-

grals of the form

2π

f ðxÞsinðnxÞdx for quite general

functions f. However, the deﬁnition of integration then

in use was far too limited for the desir ed generality.

Georg Friedrich Bernhard Riemann (18261866) rea-

lized that, to further develop Fourier’s work, a new

concept of the integral was needed. Toward that end, in

1854, Riemann introduced what we now call the Rie-

mann integral.

Bernhard Riemann

Riemann’s short life was marked by nervous break -

downs and serious illness—pleurisy, jaundice, and,

ultimately, tuberculosis. Although he began his studies

in theology, he was able to convince his father to allow

him to pursue what was then an “economically value-

less” subject—mathematical physics. In quantity, his

mathematical output was relatively modest for a

mathematician of his stature. Yet his work has assumed

fundamental importance in several ﬁelds— he is

remembered for the Riemann inte gral in analysis, the

Riemann zeta function in number theory, Riemann

surfaces and Cauchy-Riemann eq uations in the theory

of functions of a complex variable, and Riemannian

manifolds in geomet ry. The importance that his work

has had in physics is also noteworthy. Indeed, Rie-

mannian geometry proved to be essential for Einstein’s

theory of relativity, as well as for much of the physics

that has been done since.

468 Chapter 5 The Integral

CHAPTER 6

Techniques

of Integration

The purpose of the present chapter is to develop some facility with calculating

integrals. Until now, the integrals that we have calculated have been of two types.

Either we have immediately recognized an antiderivative, or we have converted an

integral into one of that type by making a direct substitution. In fact, an array of

diverse procedures can be brought to bear on the calculation of integral s. In this

chapter, we will study the most important of those methods.

Tables of integrals a nd computer algebra systems are valuable resources for

calculating integrals. However, neither renders obsolete the need for a basic

working knowledge of integration theory. In this section, you will learn funda-

mental algebraic and trigonometric mani pulations that are the basis for evaluating

integrals that arise frequently in mathematical applications. But the methods you

will learn in this section have a signiﬁcance that transcends integration theory.

Indeed, these techniques are needed for the development of many important topics

in engineering, science, and mathematics.

PREVIEW

469

6.1 Integration by Parts

The integral of the sum of two functions is the sum of their integrals. This simple

observation enables us to break up some complicated integration problems into

several simple ones. But what if we want to integrate the product of two functions?

A technique called integration by parts enables us to evaluate many integrals that

involve a product of two functions.

The Product Rule

in Reverse

If u and v are two differentiable functions, then we know that

ðu  vÞ5

 v 1

 u:

We rewrite this equation as

u 

ðu  vÞ2 v 

Integrating both sides gives

u 

dx 5

ðu  vÞdx 2

v 

Observing that u v is the antiderivative of

ðu  vÞ, we obtain the integration by

parts formula:

u 

dx 5 u  v 2

v 

dx: ð6:1:1Þ

INSIGHT

Often we use “differential notation” and summarize the integration by

parts formula as

udv5 uv 2

vdu: ð6:1:2Þ

Here dv is shorthand for

dx,anddu is shorthand for

dx. This compact form of

the integration by parts formula is the easiest to remember. Bear in mind, however, that it

conceals the original variable of integration (x in our discussion).

We may use a version of formula (6.1.2) for deﬁnite integrals provided that we use

appropriate limits of integration:

x5b

x5a

udv5 u  v



x5b

x5a

x5b

x5a

vdu: ð6:1:3Þ

Some Examples ⁄ EXAMPLE 1 Calculate

xcosðxÞdx:

Solution We

integrate by parts. Our ﬁrst job is to decide what function will play

the role of u an d what function will play the role of v. Let us take u 5 x and

dv 5

dx 5 cos ðxÞdx : Then

x  cosðxÞdx 5

udv: Therefore according to

equation (6.1.2),

xcosðxÞdx 5 uv 2

u: ð6:1:4Þ

Our task is to work out the right-hand side. We already know what u is. What is v?

Because dv 5 cos(x) dx, we see that v 5

dv 5

cosðxÞdx is an antiderivative of cos(x).

470 Chapter 6 Techniques of Integration

Therefore we may take v 5 sin(x). Likewise, because u 5 x,itfollowsthat

du 5

 dx 5 1  dx: If we substitute for u, v,anddu on the right side of equation

(6.1.4), then we obtain

x cosðxÞdx 5 x sinðxÞ2

sinðxÞ1dx:

We have converted our original integration problem into a much easier one.

Because

sinðxÞdx 52cosðxÞ1 C; we conclude that

x cosðxÞdx 5 x sinðxÞ2 ð2cosðxÞ1 CÞ5 xsinðxÞ1 cosðxÞ2 C:

If you prefer, you may replace the arbitrary constant C with 2C. Then, the answer

takes on the more familiar form x sin(x) 1 cos(x) 1 C.

As usual with indeﬁnite

integrals, we can check our work by differentiating our answer and verifying that

the result equals the integrand:



xsinðxÞ1 cosðxÞ2 C





xcosðxÞ1 sinðxÞ





2sinðxÞ



2 0 5 x cosðxÞ: ¥

INSIGHT

The purpose of integration by parts is to convert a difﬁcult integral into a

new integral that will be easier. We must bear this notion in mind as we make our choice

of u and v. In Example 1, if we had selected u 5 cos(x) and dv 5 xdx, then we would

calculate du 5

dx 52sinðxÞdx; v 5

dv 5

xdx5 x

=2, and the new integral

vdu52

sinðxÞdx

would have been harder, not easier, than the one we started with.

When a power of x is present in the integrand, it is often a good rule to choose u to

be the power of x. Then, when integration by parts is applied, the power of x will be

decreased. In this way, we guarantee in advance that one factor becomes simpler.

Of course, we must also consider the role of dv. As the next example shows, that

consideration may be more signiﬁcant.

⁄ EXAMPLE 2 Calculate

2x ln(x) dx.

Solution In

this example, it would be foolish to take dv 5 ln(x) dx because we do not

know an antiderivative for ln(x). So we take dv 5 2xdxand u 5 ln(x). Then we have

u 5 lnðxÞ dv 5 2xd

du 5 ð1=xÞdx v 5 x

Thus

2x lnðxÞdx 5

lnðxÞ2xdx5

udv5 uv



vdu5 lnðxÞx



dx:

Once again, we have converted a difﬁcult integral into a new integral that is much

simpler. We continue calculating:

2x lnðxÞdx 5 x

lnðxÞ



dx 5



lnð4Þ2 1

 0





8 2



5 16 lnð4Þ2

5 32 lnð2Þ2

: ¥

6.1 Integration by Parts 471

⁄ EXAMPLE 3 Calculate

lnðxÞdx

Solution This

is a new type of problem for us. On the one hand, we do not know an

antiderivative for ln(x). On the other hand, the integrand does not appear to be a

product because we see only the one function, ln(x). However, we can rewrite the

integral as

lnðxÞ1dx:

take u 5 ln(x)anddv 5 1 dx. We have

u 5 lnðxÞ dv 5 1dx

du 5 ð1=x Þ dx v 5 x:

Thus

lnðxÞ1dx 5

udv5 u  v 2

vdu5 lnðxÞx 2

x 

5 x lnðxÞ2

1dx 5 x lnðxÞ2 x 1 C: ¥

Advanced Examples Sometimes applying integration by parts just once is not sufﬁcient to solve the

problem at hand, and further applications might be needed. The next two exampl es

will illustrate.

⁄ EX

AMPLE 4 In atomic measurements, it is sometime s convenient to use

the Bohr radius α

5 0.52917725 3 10

210

m as a unit of distance. Let ρ denote any

(unitless) positive number. At a given instant of time, the probability of ﬁnding the

hydrogen electron at a distance no greater than ρ  α

from the atomic nucleus is

22r

dr:

What is the probability p that the hydrogen electron is less than twice the Bohr

radius from the nucleus?

Solution The

answer is given by

p 5

22r

dr 5 4

22r

dr 5 4 J where J 5

22r

dr:

To calculate this integral, we take u 5 r

and dv 5 e

22r

dr. Then

u 5 r

dv 5 e

22r

du 5 2rdr v 52e

22r

=2:

We therefore have

J 5

22r

dr 5

r52

r50

udv5 u  v



r52

r50

r52

r50

vdu

5 r





22r





r52

r50



22r



 2rdr 522e

22r

dr:

Thus J 5 K 2 2e

where K 5

22r

dr: We see that our new integral K is simpler

than the one we started with (because the power of r is lower), but it still requires

472 Chapter 6 Techniques of Integration

work. Another application of integration by parts is needed. We apply the inte-

gration by parts formu la to K, taking u 5 r and dv 5 e

22r

dr We now have

u 5 rdv5 e

22r

du 5 1dr v 52e

22r

=2:

Therefore

K 5

22r

dr 5

r52

r50

udv5 u  v



r52

r50

r52

r50

vdu

5 r



22r





r52

r50



22r



dr 52e

22r

52e



22r





r52

r50

52e









Finally, the required probability p is given by

p 5 4J 5 4ðK 2 2e

Þ5 4





2 2e



5 1 2 13e

 0:7618967: ¥

INSIGHT

If we organize our work properly, keeping repeated applications of the

integration by parts formula separate, then there is no harm in reusing the symbols u and

v each time. Also notice that we can use the integration by parts formula with a variable

of integration other than x.

In our next example, it will appear that we have evaluated an integral indir-

ectly. After integrating by parts, the original integral arises on the right side of the

equation with a coefﬁcient not equal to 1. We treat the original integral as an

unknown variable, isolate it on one side of the equation, and divide the equation by

its coefﬁcient.

⁄ EX

AMPLE 5 Calculate the integral

S 5

cosðxÞdx:

Solution We

take u 5 e

and dv 5 cos( x ) dx. Then

u 5 e

dv 5 cos ðxÞdx

du 5 e

dx v 5 sinðxÞ:

Therefore

S 5

udv5 u  v 2

vdu 5 e

sinðxÞ2

sinðxÞe

dx:

6.1 Integration by Parts 473

We have converted the original integral problem S to a new integration problem,

but the new one does not look any simpler than the original—indeed, all we have

done is to replace cos(x) with sin(x). Nevertheless, we integrate by parts again,

taking u 5 e

and dv 5 sin(x) dx. As a result,

u 5 e

dv 5 sinðxÞdx

du 5 e

dx v 52cosðxÞ:

We have

S 5 e

sinðxÞ2

sinðxÞe

dx 5 e

sinðxÞ2



cosðxÞ1

cosðxÞe



Using the symbol S, we can rewrite this last equation as

S 5 e

sinðxÞ1 e

cosðxÞ2 S:

We can solve this equation for S, obtaining

S 5



sinðxÞ1 e

cosðxÞ



Finally, let us observe that, because S is an indeﬁnite integral, a constant of inte -

gration should be added to obtain the most general solution:

S 5



sinðxÞ1 cosðxÞ



1 C: ¥

INSIGHT

The integral that we have just calculated arises naturally in signal pro-

cessing and may be found in many engineering texts. A remarkable feature of the method

of Example 5 is that we never “calculate” the integral in the usual way. We express the

integral (which we think of as the unknown S) in terms of itself, and then we obtain

the solution by using algebra.

The method of Example 5 can also be applied to integrals of expressions of

the form sin(ax) cos(bx), sin(ax) sin(bx), and cos(ax) cos(bx). Because these

integrals are sometimes needed in applications, we will record them for reference

(not for memorization):

sinðaxÞcosðbxÞdx 52

cosðða 2 bÞxÞ

a 2 b

cosðða 1 bÞ x Þ

a 1 b

1 C; a 6¼ 6b ð6:1:5Þ

sinðaxÞsinðbxÞdx 5

sinðða 2 bÞ x Þ

a 2 b

sinðða 1 bÞxÞ

a 1 b

1 C; a 6¼ 6b ð6: 1 :6Þ

cosðaxÞcosðbxÞdx 5

sinðða 2 b ÞxÞ

a 2 b

sinðða 1 bÞ x Þ

a 1 b

1 C; a 6¼ 6b: ð6:1: 7 Þ

In our next example, we must appeal to a trigonometric identity before we can

apply the method of Example 5.

474 Chapter 6 Techniques of Integration