Chow T.L. Mathematical Methods for Physicists: A Concise Introduction
Подождите немного. Документ загружается.


and the general solut ion can be written as
y Ae
p
1
t
Be
p
2
t
e
rt
A Bcos st iA ÿ Bsin st
e
rt
A
0
cos st B
0
sin st2:20
with A
0
A B; B
0
iA ÿ B:
The solution (2.20) may be expressed in a slightly diÿerent and often more
useful form by writi ng B
0
=A
0
tan . Then
y A
2
0
B
2
0
1=2
e
rt
cos cos st sin sin stCe
rt
cosst ÿ ; 2:20a
where C and are arbitrary constants.
Example 2.10
Solve the equation D
2
4D 13y 0, given that y 1 and y
0
2 when t 0.
Solution: The auxiliary equation is p
2
4p 13 0, and hence p ÿ2 3i.
The general solution is therefore, from Eq. (2.20),
y e
ÿ2t
A
0
cos 3t B
0
sin 3t:
Since y l when t 0, we have A
0
1. Now
y
0
ÿ2e
ÿ2t
A
0
cos 3t B
0
sin 3t3e
ÿ2t
ÿA
0
sin 3t B
0
cos 3t
and since y
0
2 when t 0, we ha ve 2 ÿ2A
0
3B
0
. Hence B
0
4=3, and the
solution is
3y e
ÿ2t
3 cos 3t 4 sin 3t:
(iii) Coin cident roots
When a
2
4b, the auxiliary equation yields only one value for p, namely
p ÿa=2, and hen ce the solution y Ae
t
. This is not the general solution
as it does not contain the necessary two arbitrary constants. In order to obtain the
general solution we proceed as follows. Assume that y ve
t
, where v is a func-
tion of t to be determined. Then
y
0
v
0
e
t
ve
t
; y
00
v
00
e
t
2v
0
e
t
2
ve
t
:
Substituting for y; y
0
, and y
00
in the diÿerential equation we have
e
t
v
00
2v
0
2
v av
0
vbv0
and hence
v
00
v
0
a 2v
2
a b0:
76
ORDINARY DIFFERENTIAL EQUATIONS

Now
2
a b 0; and a 2 0
so that
v
00
0:
Hence, integrating gives
v At B;
where A and B are arbitrary constants, and the general solution of Eq. (2.16) is
y At Be
t
2:21
Example 2.11
Solve the equation (D
2
ÿ 4D 4y 0 given that y 1andDy 3 when t 0:
Solution: The auxiliary equation is p
2
ÿ 4p 4 p ÿ 2
2
0 which has one
root p 2. The general solution is therefore, from Eq. (2.21)
y At Be
2t
:
Since y 1 when t 0, we have B 1. Now
y
0
2At Be
2t
Ae
2t
and since Dy 3 when t 0,
3 2 B A:
Hence A 1 and the solution is
y t 1e
2t
:
Finding the particular integral
The particular integral is a solution of Eq. (2.15) that takes the term f t on the
right hand side into account. The complementary function is transient in nature,
so from a physical point of view, the particular integral will usually dominate the
response of the system at large times.
The method of determining the particular integral is to guess a suitable func-
tional form containing arbitrary constants, and then to choose the constants to
ensure it is indeed the solution. If our guess is incorrect, then no values of these
constants will satisfy the diÿerential equation, and so we have to try a diÿerent
form. Clearly this procedure could take a long time; fortunately, there are some
guiding rules on what to try for the common examples of f (t):
77
SECOND-ORDER EQUATIONS WITH CONSTANT COEFFICIENTS

(1) f ta polynomial in t.
If f t is a polynomial in t with highest power t
n
, then the trial parti cular
integral is also a polynomial in t, with terms up to the same power. Note
that the trial particular integral is a power series in t, even if f t contains
only a single terms At
n
.
(2) f tAe
kt
.
The trial particular integral is y Be
kt
.
(3) f tA sin kt or A cos kt.
The trial particular integral is y A sin kt C cos kt. That is, even though
f t contains only a sine or cosine term, we need both sine and cosine terms
for the particular integ ral.
(4) f tAe
t
sin ÿt or Ae
t
cos ÿt.
The trial particular integral is y e
t
B sin ÿt C cos ÿt.
(5) f t is a polynomial of order n in t, multiplied by e
kt
.
The trial particular integral is a polynomial in t with coecients to be
determined, multiplied by e
kt
.
(6) f t is a polynomial of order n in t, multiplied by sin kt.
The trial particular integral is y
n
j0
B
j
sin kt C
j
cos ktt
j
. Can we try
y B sin kt C cos kt
n
j0
D
j
t
j
? The answer is no. Do you know why?
If the trial particular integral or part of it is identi cal to one of the terms of the
complementary function, then the trial particular integ ral must be multiplied by
an extra power of t. Therefore, we need to ®nd the complementary function before
we try to work out the particular integral. What do we mean by `identical in
form'? It means that the ratio of their t-dependences is a constant. Thus ÿ2e
ÿt
and Ae
ÿt
are identical in form, but e
ÿt
and e
ÿ2t
are not.
Particular integral and the operator D d=dx
We now describe an alternative method that can be used for ®nding particular
integrals. As compared with the method described in previous section, it involves
less guesswork as to what the form of the solution is, and the constants mu lti-
plying the functi onal forms of the answer are obtained automatically. It does,
however, require a fair amount of practice to ensure that you are familiar with
how to use it.
The technique involves using the diÿerential operator D d=dt, which is an
interesting and simp le example of a linear operator without a matrix repres enta-
tion. It is obvious that D obeys the relevant laws of operator algebra: suppose f
and g are functions of t, and a is a con stant, then
(i) Df gDf Dg (distributive);
(ii) Daf aDf (commutative);
(iii) D
n
D
m
f D
nm
f (index law).
78
ORDINARY DIFFERENTIAL EQUATIONS

We can form a polynomial function of D and write
FD a
0
D
n
a
1
D
nÿ1
a
nÿ1
D a
n
so that
FDf ta
0
D
n
f a
1
D
nÿ1
f a
nÿ1
Df a
n
f
and we can interpret D
ÿ1
as follows
D
ÿ1
Df tf t
and
Z
Df dt f :
Hence D
ÿ1
indicates the operation of integration (the inverse of diÿerentiation).
Similarly D
ÿm
f means `integrate f tm times'.
These properties of the linear operator D can be used to ®nd the particular
integral of Eq. (2.15):
d
2
y
dt
2
a
dy
dt
by D
2
aD b
ÿ
y f t
from which we obtain
y
1
D
2
aD b
f t
1
FD
f t; 2:22
where
FD D
2
aD b:
The trouble with Eq. (2.22) is that it contains an expression involving Ds in the
denominator. It requires a fair amount of practice to use Eq. (2.22) to express y in
terms of conventional functions. For this, there are several rules to help us.
Rules for D operators
Given a power series of D
GDa
0
a
1
D a
n
D
n
and since D
n
e
t
n
e
t
, it follows that
GDe
t
a
0
a
1
D a
n
D
n
e
t
Ge
t
:
Thus we have
Rule (a): GDe
t
Ge
t
provided G is convergent.
When GD is the expansion of 1=FD this rule gives
1
FD
e
t
1
F
e
t
provided F 60:
79
SECOND-ORDER EQUATIONS WITH CONSTANT COEFFICIENTS
Now let us operate GD on a product function e
t
Vt:
GDe
t
Vt GDe
t
Vte
t
GDVt
e
t
GGDVte
t
GD Vt:
That is, we have
Rule (b): GDe
t
Vt e
t
GD Vt :
Thus, for example
D
2
e
t
t
2
e
t
D
2
t
2
:
Rule (c): GD
2
sin kt Gÿk
2
sin kt:
Thus, for example
1
D
2
sin 3tÿ
1
9
sin 3t:
Example 2.12 Damped oscillations (Fig. 2.2)
Suppose we have a spring of natural length L (that is, in its unstretched state). If
we hang a ball of mass m from it and leave the system in equilibrium, the spring
stretches an amount d, so that the ball is now L d from the suspension point.
We measure the vertical displacement of the ball from this static equilibrium
point. Thus, L d is y 0, and y is chosen to be positive in the downward
direction, and negative upward. If we pull down on the ball and then release it,
it oscillates up and down about the equilibrium position. To analyze the oscilla-
tion of the ball, we need to know the forces acting on it:
80
ORDINARY DIFFERENTIAL EQUATIONS
Figure 2.2. Damped spring system.
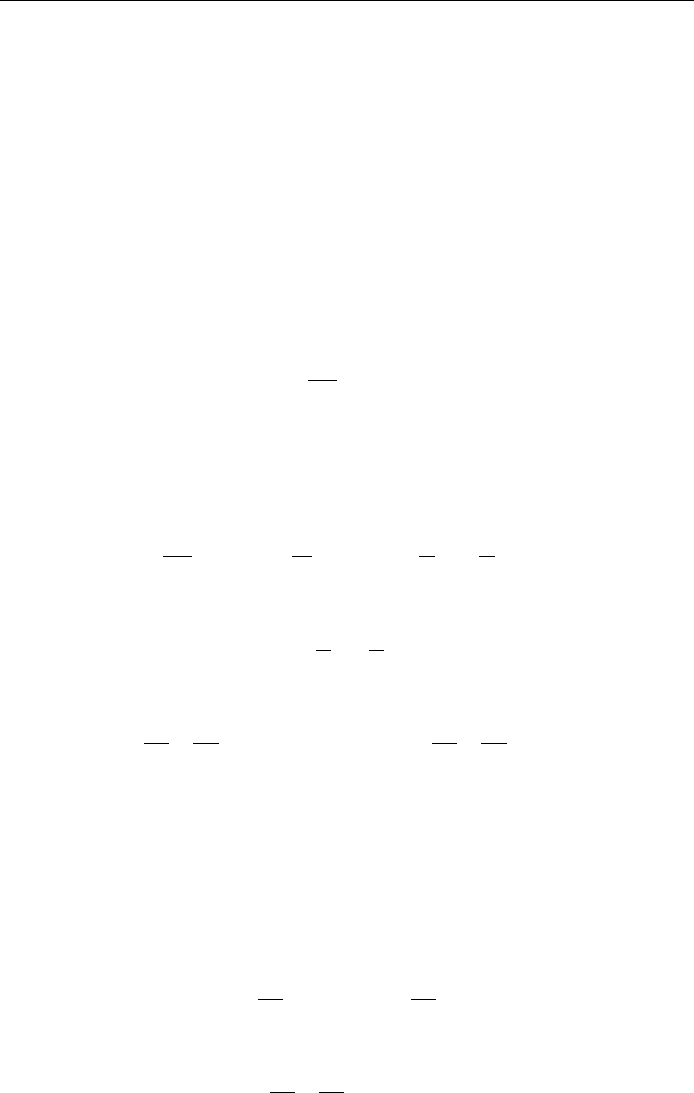
(1) the downward force of gravity, mg:
(2) the restoring force ky which always opposes the motion (Hooke's law),
where k is the spring constant of the spring. If the ball is pulled down a
distance y from its static e quilibrium position, this force is ÿkd y.
Thus, the total net force acti ng on the ball is
mg ÿ kd ymg ÿ kd ÿ ky:
In static equilibrium, y 0 and all forces balances. Hence
kd mg
and the net force acting on the spring is just ÿky; and the equation of motion of
the ball is given by Newton's second law of motion:
m
d
2
y
dt
2
ÿky;
which describes free oscil lation of the ball. If the ball is connected to a dashpot
(Fig. 2.2), a damping force will come into play. Experiment shows that the damp-
ing force is given by ÿbdy=dt, where the constant b is called the damping constant.
The equation of motion of the ball now is
m
d
2
y
dt
2
ÿky ÿ b
dy
dt
or y
00
b
m
y
0
k
m
y 0:
The auxiliary equation is
p
2
b
m
p
k
m
0
with roots
p
1
ÿ
b
2m
1
2m
b
2
ÿ 4km
p
; p
2
ÿ
b
2m
ÿ
1
2m
b
2
ÿ 4km
p
:
We now have three cases, resulting in quite diÿerent motions of the oscillator.
Case 1 b
2
ÿ 4km > 0 (overdamping)
The solution is of the form
ytc
1
e
p
1
t
c
2
e
p
2
t
:
Now, both b and k are positive, so
1
2m
b
2
ÿ 4km
p
<
b
2m
and accordingly
p
1
ÿ
b
2m
1
2m
b
2
ÿ 4km
p
< 0:
81
SECOND-ORDER EQUATIONS WITH CONSTANT COEFFICIENTS

Obviously p
2
< 0 also. Thus, yt!0ast !1. This means that the oscillation
dies out with time and eventually the mass will assume the static equilibrium
position.
Case 2 b
2
ÿ 4km 0 (critical damping)
The solution is of the form
yte
ÿbt=2m
c
1
c
2
t:
As both b and m are positive, yt!0ast !1as in case 1. But c
1
and c
2
play a
signi®cant role here. Since e
ÿbt=2m
6 0 for ®nite t, yt can be zero only when
c
1
c
2
t 0, and this happens when
t ÿc
1
=c
2
:
If the number on the right is positive, the mass passes through the equilibrium
position y 0 at that time. If the number on the right is negative, the mass never
passes throu gh the equilibrium position.
It is interesting to note that c
1
y0, that is, c
1
measures the initial position.
Next, we note that
y
0
0c
2
ÿ bc
1
=2m; or c
2
y
0
0by0=2m:
Case 3 b
2
ÿ 4km < 0 (underdamping)
The auxiliary equation now has complex roots
p
1
ÿ
b
2m
i
2m
4km ÿ b
2
p
; p
2
ÿ
b
2m
ÿ
i
2m
4km ÿ b
2
p
and the solution is of the form
yte
ÿbt=2m
c
1
cos
4km ÿ b
2
p
t
2m
c
2
sin
4km ÿ b
2
p
t
2m
hi
;
which can be rewritten as
ytce
ÿbt=2m
cos!t ÿ ;
where
c
c
2
1
c
2
2
q
; tan
ÿ1
c
2
c
1
; and !
4km ÿ b
2
p
=2m:
As in case 2, e
ÿbt=2m
! 0ast !1, and the oscillation gradually dies down to
zero with increasing time. As the oscillator dies down, it oscillates with a fre-
quency !=2 . But the oscillation is not periodic.
82
ORDINARY DIFFERENTIAL EQUATIONS

The Euler linear equation
The linear equation with variable coecients
x
n
d
n
y
dx
n
p
1
x
nÿ1
d
nÿ1
y
dx
nÿ1
p
nÿ1
x
dy
dx
p
n
y f x; 2:23
in which the derivative of the jth order is multiplied by x
j
and by a constant, is
known as the Euler or Cauchy equation. It can be reduced, by the substitution
x e
t
, to a linear equation with constant coecients with t as the independent
variable. Now if x e
t
, then dx=dt x,and
dy
dx
dy
dt
dt
dx
1
x
dy
dt
; or x
dy
dx
dy
dt
and
d
2
y
dx
2
d
dx
dy
dx
d
dt
1
x
dy
dt
dt
dx
1
x
d
dt
1
x
dy
dt
or
x
d
2
y
dx
2
1
x
d
2
y
dt
2
dy
dt
d
dt
1
x
1
x
d
2
y
dx
2
ÿ
1
x
dy
dt
and hence
x
2
d
2
y
dx
2
d
2
y
dt
2
ÿ
dy
dt
d
dt
dy
dt
ÿ 1
y:
Similarly
x
3
d
3
y
dx
3
d
dt
d
dt
ÿ 1
d
dt
ÿ 2
y;
and
x
n
d
n
y
dx
n
d
dt
d
dt
ÿ 1
d
dt
ÿ 2
d
dt
ÿ n 1
y:
Substituting for x
j
d
j
y=dx
j
in Eq. (2.23) the equation transforms into
d
n
y
dt
n
q
1
d
nÿ1
y
dt
nÿ1
q
nÿ1
dy
dt
q
n
y f e
t
in which q
1
, q
2
; ...; q
n
are constants.
Example 2.13
Solve the equation
x
2
d
2
y
dx
2
6x
dy
dx
6y
1
x
2
:
83
THE EULER LINEAR EQUATION
Solution: Put x e
t
, then
x
dy
dx
dy
dt
; x
2
d
2
y
dx
2
d
2
y
dt
2
ÿ
dy
dt
:
Substituting these in the equation gives
d
2
y
dt
2
5
dy
dt
6y e
t
:
The auxiliary equation p
2
5p 6 p 2p 30 has two roots: p
1
ÿ2,
p
2
3. So the complementary function is of the form y
c
Ae
ÿ2t
Be
ÿ3t
and the
particular integral is
y
p
1
D 2D 3
e
ÿ2t
te
ÿ2t
:
The general solution is
y Ae
ÿ2t
Be
ÿ3t
te
ÿ2t
:
The Euler equation is a special case of the general linear second-order equation
D
2
y pxDy qxy f x;
where px, qx, and f x are given functions of x. In general this type of
equation can be solved by series approximation methods which will be introduced
in next section, but in some instances we may solve it by means of a variable
substitution, as shown by the following exampl e:
D
2
y 4x ÿ x
ÿ1
Dy 4x
2
y 0;
where
px4x ÿ x
ÿ1
; qx4x
2
; and f x0:
If we let
x z
1=2
the above equation is transformed into the following equation with constant
coecients:
D
2
y 2Dy y 0;
which has the solution
y A Bze
ÿz
:
Thus the general solution of the original equation is y A Bx
2
e
ÿx
2
:
84
ORDINARY DIFFERENTIAL EQUATIONS

Solutions in power series
In many problems in physics and engineering, the diÿerential equations are of
such a form that it is not possible to express the solution in terms of elementary
functions such as exponential, sine, cosine, etc.; but solutions can be obtained as
convergent in®nite series. What is the basis of this method? To see it, let us
consider the following simple second-order linear diÿerential equation
d
2
y
dx
2
y 0:
Now assuming the solution is given by y a
0
a
1
x a
2
x
2
, we further
assume the series is convergent and diÿerentiable term by term for suciently
small x. Then
dy=dx a
1
2a
2
x 3a
3
x
2
and
d
2
y=dx
2
2a
2
2 3a
3
x 3 4a
4
x
2
:
Substituting the series for y and d
2
y=dx
2
in the given diÿerential equation and
collecting like powers of x yields the identity
2a
2
a
0
2 3a
3
a
1
x 3 4a
4
a
2
x
2
0:
Since if a power series is identically zero all of its coecients are zero, equating to
zero the term independent of x and coecients of x, x
2
; ...; gives
2a
2
a
0
0; 4 5a
5
a
3
0;
2 3a
3
a
1
0; 5 6a
6
a
4
0;
3 4a
4
a
2
0;
and it follows that
a
2
ÿ
a
0
2
; a
3
ÿ
a
1
2 3
ÿ
a
1
3!
; a
4
ÿ
a
2
3 4
ÿ
a
0
4!
a
5
ÿ
a
3
4 5
a
1
5!
; a
6
ÿ
a
4
5 6
ÿ
a
0
6!
; ...:
The required solution is
y a
0
1 ÿ
x
2
2!
x
4
4!
ÿ
x
6
6!
ÿ
ý!
a
1
x ÿ
x
3
3!
x
5
5!
ÿ
ý!
;
you should recognize this as equivalent to the usual solution
y a
0
cos x a
1
sin x, a
0
and a
1
being arbitrary constants.
85
SOLUTIONS IN POWER SERIES
