Jacques I. Mathematics for Economics and Business

Подождите немного. Документ загружается.

Differentiation

340

Practice Problem

6 If the demand equation is

P = 200 − 40 ln(Q + 1)

calculate the price elasticity of demand when Q = 20.

Practice Problems

7 Write down the derivative of

(a)

y = e

(b) y = e

−342x

−x

+ 4e

(d) y = 10e

− 2x

+ 7

8 Write down the derivative of

(a)

y = ln(3x)(x > 0) (b) y = ln(−13x)(x < 0)

9 Use the chain rule to differentiate

(a)

y = e

(b) y = ln(x

+ 3x

)

10 Use the product rule to differentiate

(a)

y = x

(b) y = x ln x

11 Use the quotient rule to differentiate

(a)

y = (b) y =

12 Use the rules of logarithms to expand each of the following functions. Hence write their derivatives.

(a)

y = ln (b) y = ln(x√(3x − 1)) (c) y = ln

13 Find and classify the stationary points of

(a)

y = xe

−x

(b) y = ln x − x

Hence sketch their graphs.

14 Find the output needed to maximize profit given that the total cost and total revenue functions are

TC = 2Q and TR = 100 ln(Q + 1)

respectively.

15 If a firm’s production function is given by

Q = 700Le

−0.02L

find the value of L which maximizes output.

16 The demand function of a good is given by

P = 100e

−0.1Q

Show that demand is unit elastic when Q = 10.

17 The growth rate of an economic variable, y, is defined to be ÷ y.

Use this definition to find the growth rate of the variable, y = Ae

x + 1

−

x − 1

ln x

+ 2

MFE_C04h.qxd 16/12/2005 11:19 Page 340

chapter 5

Partial Differentiation

This chapter continues the topic of calculus by describing how to differentiate func-

tions of more than one variable. In many ways this chapter can be regarded as the

climax of the whole book. It is the summit of the mathematical mountain that we

have been merrily climbing. Not only are the associated mathematical ideas and

techniques quite sophisticated, but also partial differentiation provides a rich source

of applications. In one sense there is no new material presented here. If you know

how to differentiate a function of one variable then you also know how to partially

differentiate a function of several variables because the rules are the same. Similarly,

if you can optimize a function of one variable then you need have no fear of uncon-

strained and constrained optimization. Of course, if you cannot use the elementary

rules of differentiation or cannot find the maximum and minimum values of a

function as described in Chapter 4 then you really are fighting a lost cause. Under

these circumstances you are best advised to omit this chapter entirely. There is

no harm in doing this, because it does not form the prerequisite for any of the later

topics. However, you will miss out on one of the most elegant and useful branches

of mathematics.

There are six sections to this chapter. It is important that Sections 5.1 and 5.2 are

read first, but the remaining sections can be studied in any order. Sections 5.1 and

5.2 follow the familiar pattern. We begin by looking at the mathematical techniques

and then use them to determine marginal functions and elasticities. Section 5.3

describes the multiplier concept and completes the topic of statics which you stud-

ied in Chapter 1.

The final three sections are devoted to optimization. For functions of several vari-

ables, optimization problems are split into two groups, unconstrained and constrained.

MFE_C05a.qxd 16/12/2005 10:40 Page 341

Unconstrained problems, tackled in Section 5.4, involve the maximization and min-

imization of functions in which the variables are free to take any values whatsoever.

In a constrained problem only certain combinations of the variables are examined.

For example, a firm might wish to minimize costs but is constrained by the need to

satisfy production quotas, or an individual might want to maximize utility but is sub-

ject to a budgetary constraint, and so on. There are two ways of solving constrained

problems: the method of substitution and the method of Lagrange multipliers,

described in Sections 5.5 and 5.6 respectively.

MFE_C05a.qxd 16/12/2005 10:40 Page 342

section 5.1

Functions of several

variables

Most relationships in economics involve more than two variables. The demand for a good

depends not only on its own price but also on the price of substitutable and complementary

goods, incomes of consumers, advertising expenditure and so on. Likewise, the output from

a production process depends on a variety of inputs, including land, capital and labour. To

analyse general economic behaviour we must extend the concept of a function, and particularly

the differential calculus, to functions of several variables.

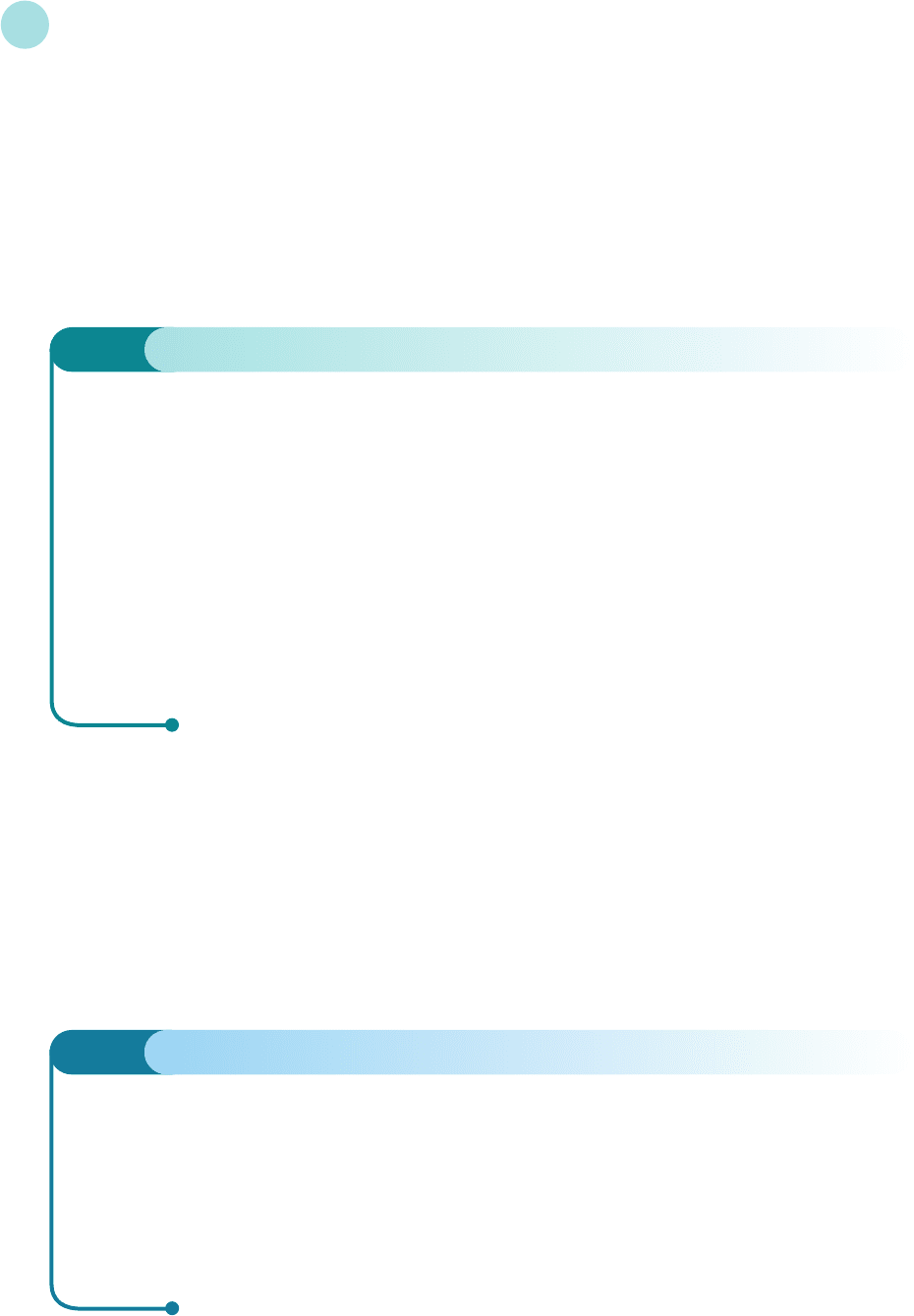
A function, f, of two variables is a rule that assigns to each incoming pair of numbers,

(x, y), a uniquely deﬁned outgoing number, z. This is illustrated in Figure 5.1. The ‘black box’

Objectives

At the end of this section you should be able to:

 Use the function notation, z = f(x, y).

 Determine the ﬁrst-order partial derivatives, f

and f

 Determine the second-order partial derivatives, f

, f

and f

 Appreciate that, for most functions, f

= f

 Use the small increments formula.

 Perform implicit differentiation.

Figure 5.1

MFE_C05a.qxd 16/12/2005 10:40 Page 343

f performs some arithmetic operation on x and y to produce z. For example, the rule might be

‘multiply the two numbers together and add twice the second number’. In symbols we write

this either as

f(x, y) = xy + 2y

or as

z = xy + 2y

In order to be able to evaluate the function we have to specify the numerical values of both x

and y.

Partial Differentiation

344

Example

If f(x, y) = xy + 2y evaluate

(a) f(3, 4) (b) f(4, 3)

Solution

(a) Substituting x = 3 and y = 4 gives

f(3, 4) = 3(4) + 2(4) = 20

(b) Substituting x = 4 and y = 3 gives

f(4, 3) = 4(3) + 2(3) = 18

Note that, for this function, f(3, 4) is not the same as f (4, 3), so in general we must be careful to write down

the correct ordering of the variables.

Practice Problem

1 If

f(x, y) = 5x + xy

− 10

and

g(x

, x

) = x

+ x

evaluate

(a)

f(0, 0) (b) f(1, 2) (c) f (2, 1) (d) g(5, 6, 10) (e) g(0, 0, 0) (f) g(10, 5, 6)

We have used the labels x and y for the two incoming numbers (called the independent

variables) and z for the outgoing number (called the dependent variable). We could equally

well have written the above function as

y = x

+ 2x

say, using x

and x

to denote the independent variables and using y this time to denote the

dependent variable. The use of subscripts may seem rather cumbersome, but it does provide an

obvious extension to functions of more than two variables. In general, a function of n variables

can be written

y = f(x

, x

,..., x

)

MFE_C05a.qxd 16/12/2005 10:40 Page 344

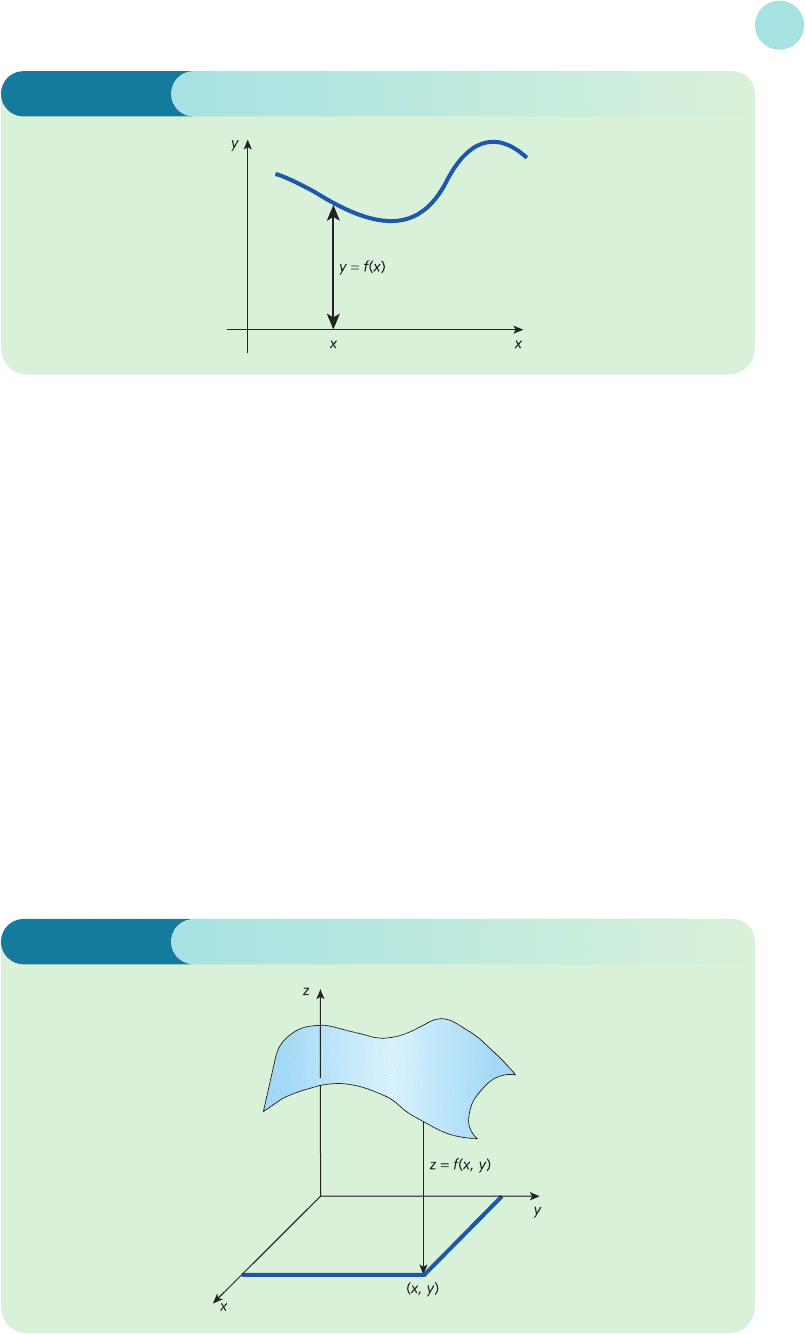
A function of one variable can be given a pictorial description using graphs, which help to

give an intuitive feel for its behaviour. Figure 5.2 shows the graph of a typical function

y = f(x)

in which the horizontal axis determines the incoming number, x, and the vertical axis deter-

mines the corresponding outgoing number, y. The height of the curve directly above any point

on the x axis represents the value of the function at this point.

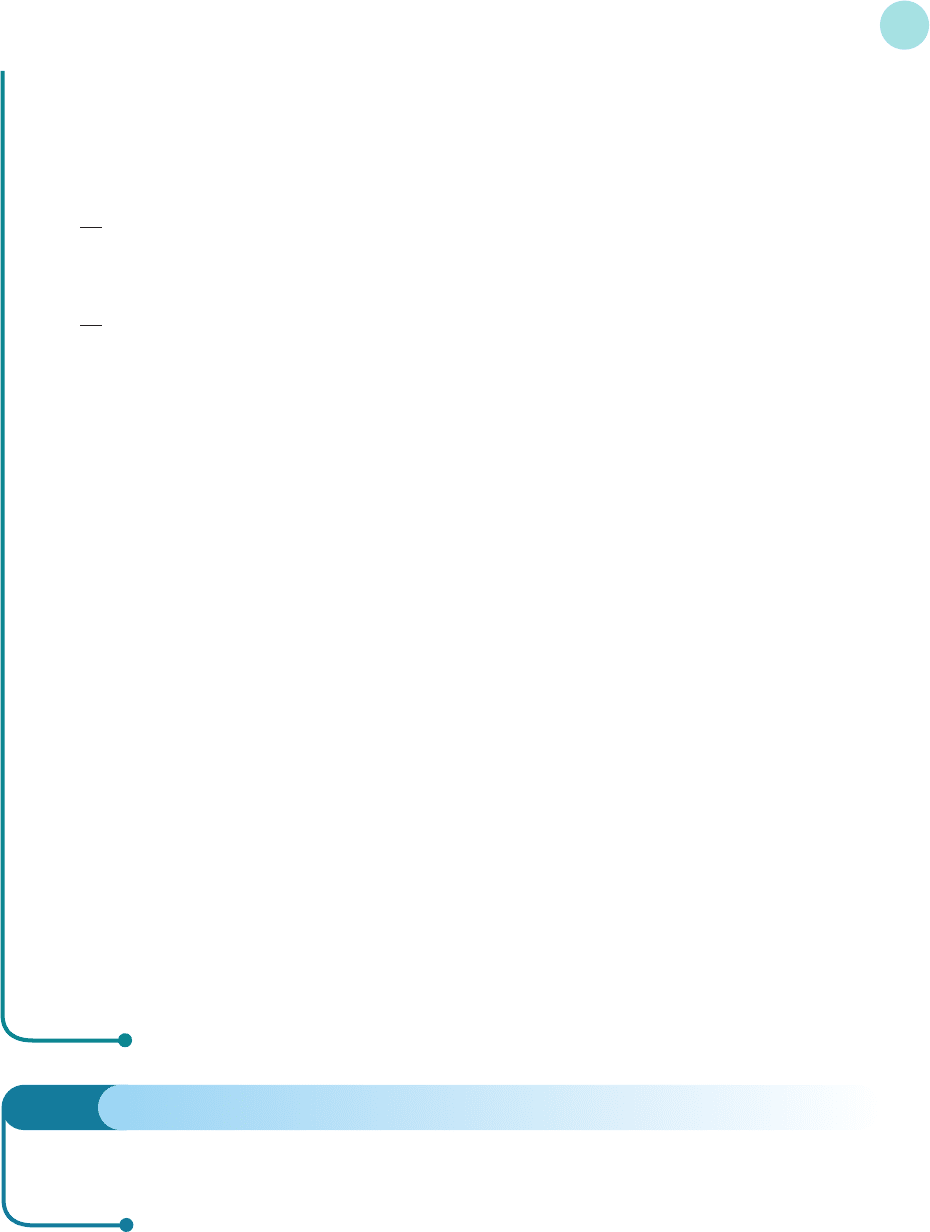
An obvious question to ask is whether there is a pictorial representation of functions of

several variables. The answer is yes in the case of functions of two variables, although it is not

particularly easy to construct. A function

z = f(x, y)

can be thought of as a surface, rather like a mountain range, in three-dimensional space as

shown in Figure 5.3. If you visualize the incoming point with coordinates (x, y) as lying in a

horizontal plane then the height of the surface, z, directly above it represents the value of the

function at this point. As you can probably imagine, it is not an easy task to sketch the surface

by hand from an equation such as

f(x, y) = xy

+ 4x

5.1 • Functions of several variables

345

Figure 5.2

Figure 5.3

MFE_C05a.qxd 16/12/2005 10:40 Page 345

although three-dimensional graphics packages are available for most computers which can

produce such a plot.

Partial Differentiation

346

Advice

There is an example which describes how to use Maple to produce a three-dimensional

plot at the end of the next section. If you are interested, you might like to read the

example now and see if you can produce graphs of some of the functions considered here.

It is impossible to provide any sort of graphical interpretation for functions of more than

two variables. For example, a function of, say, four variables would require ﬁve dimensions,

one for each of the incoming variables and a further one for the outgoing variable! In spite of

this setback we can still perform the task of differentiating functions of several variables and,

as we shall see in the remaining sections of this chapter, such derivatives play a vital role in

analysing economic behaviour.

Given a function of two variables,

z = f(x, y)

we can determine two ﬁrst-order derivatives. The partial derivative of f with respect to x is

written as

or or f

and is found by differentiating f with respect to x, with y held constant. Similarly, the partial

derivative of f with respect to y is written as

or or f

and is found by differentiating f with respect to y, with x held constant. We use curly dees in

the notation

to distinguish partial differentiation of functions of several variables from ordinary differenti-

ation of functions of one variable. The alternative notation, f

, is analogous to the f ′ notation

for ordinary differentiation.

∂f

∂x

∂f

∂y

∂z

∂y

∂f

∂x

∂z

∂x

Example

Find the ﬁrst-order partial derivatives of the functions

(a) f(x, y) = x

+ y

(b) f(x, y) = x

Solution

(a) To differentiate the function

f(x, y) = x

+ y

MFE_C05a.qxd 16/12/2005 10:40 Page 346

with respect to x we work as follows. By the sum rule we know that we can differentiate each part sep-

arately and add. Now, when we differentiate x

with respect to x we get 2x. However, when we differ-

entiate y

with respect to x we get 0. To see this, note from the deﬁnition of partial differentiation with

respect to x that the variable y is held constant. Of course, if y is a constant then so is y

and, as we dis-

covered in Chapter 4, constants differentiate to zero. Hence

= 2x + 0 = 2x

In the same way

= 0 + 3y

= 3y

This time x is held constant, so x

goes to zero, and when we differentiate y

with respect to y we get 3y

(b) To differentiate the function

f(x, y) = x

with respect to x, we differentiate in the normal way, taking x as the variable while pretending that y is

a constant. Now, when we differentiate a constant multiple of x

we differentiate x

to get 2x and then

multiply by the constant. For example,

differentiates to 7(2x) = 14x

−100x

differentiates to −100(2x) =−200x

and

differentiates to c(2x) = 2cx

for any constant c. In our case, y, plays the role of a constant, so

y differentiates to (2x)y = 2xy

Hence

= 2xy

Similarly, to ﬁnd f

we treat y as the variable and x as a constant in the expression

f(x, y) = x

Now, when we differentiate a constant multiple of y we just get the constant, so cy differentiates to c. In

our case, x

plays the role of c, so x

y differentiates to x

. Hence

= x

∂f

∂y

∂f

∂x

5.1 • Functions of several variables

347

Practice Problem

2 Find expressions for the first-order partial derivatives for the functions

(a)

f(x, y) = 5x

− y

(b) f(x, y) = x

− 10x

In general, when we differentiate a function of two variables, the thing we end up with is

itself a function of two variables. This suggests the possibility of differentiating a second time.

In fact there are four second-order partial derivatives. We write

MFE_C05a.qxd 16/12/2005 10:40 Page 347

or or f

for the function obtained by differentiating twice with respect to x,

or or f

for the function obtained by differentiating twice with respect to y,

or or f

for the function obtained by differentiating ﬁrst with respect to x and then with respect to y, and

or or f

for the function obtained by differentiating ﬁrst with respect to y and then with respect to x.

∂

∂x∂y

∂

∂x∂y

∂

∂y∂x

∂

∂y∂x

∂

∂y

∂

∂y

∂

∂x

∂

∂x

Partial Differentiation

348

Example

Find expressions for the second-order partial derivatives f

, f

and f

for the functions

(a) f(x, y) = x

+ y

(b) f(x, y) = x

Solution

(a) The ﬁrst-order partial derivatives of the function

f(x, y) = x

+ y

have already been found and are given by

= 2x, f

= 3y

To ﬁnd f

we differentiate f

with respect to x to get

= 2

To ﬁnd f

we differentiate f

with respect to y to get

= 6y

To ﬁnd f

we differentiate f

with respect to y to get

= 0

Note how f

is obtained. Starting with the original function

f(x, y) = x

+ y

we ﬁrst differentiate with respect to x to get 2x and when we differentiate this with respect to y we keep

x constant, so it goes to zero. Finally, to ﬁnd f

we differentiate f

with respect to x to get

= 0

Note how f

is obtained. Starting with the original function

f(x, y) = x

+ y

we ﬁrst differentiate with respect to y to get 3y

and when we differentiate this with respect to x we keep

y constant, so it goes to zero.

MFE_C05a.qxd 16/12/2005 10:40 Page 348

(b) The ﬁrst-order partial derivatives of the function

f(x, y) = x

have already been found and are given by

= 2xy, f

= x

Hence

= 2y, f

= 0, f

= 2x, f

= 2x

5.1 • Functions of several variables

349

Practice Problem

3 Find expressions for the second-order partial derivatives of the functions

(a)

f(x, y) = 5x

− y

(b) f(x, y) = x

− 10x

[Hint: you might find your answer to Practice Problem 2 useful.]

Looking back at the expressions obtained in the previous example and Practice Problem 3,

notice that in all cases

It can be shown that this result holds for all functions that arise in economics. It is immaterial

in which order the partial differentiation is performed. Differentiating with respect to x then

y gives the same expression as differentiating with respect to y then x. (In fact, there are

some weird mathematical functions for which this result is not true, although they need not

concern us.)

Although we have concentrated exclusively on functions of two variables, it should be

obvious how to work out partial derivatives of functions of more than two variables. For the

general function

y = f(x

, x

,..., x

)

there are n ﬁrst-order partial derivatives, written as

or f

(i = 1, 2,..., n)

which are found by differentiating with respect to one variable at a time, keeping the remain-

ing n − 1 variables ﬁxed. The second-order partial derivatives are determined in an analogous

way.

∂f

∂x

∂

∂x∂y

∂

∂y∂x

Example

Find the derivative, f

, for the function

f(x

, x

) = x

+ x

+ 5x



MFE_C05a.qxd 16/12/2005 10:40 Page 349