Mavko G., Mukerji T., Dvorkin J. The Rock Physics Handbook
Подождите немного. Документ загружается.

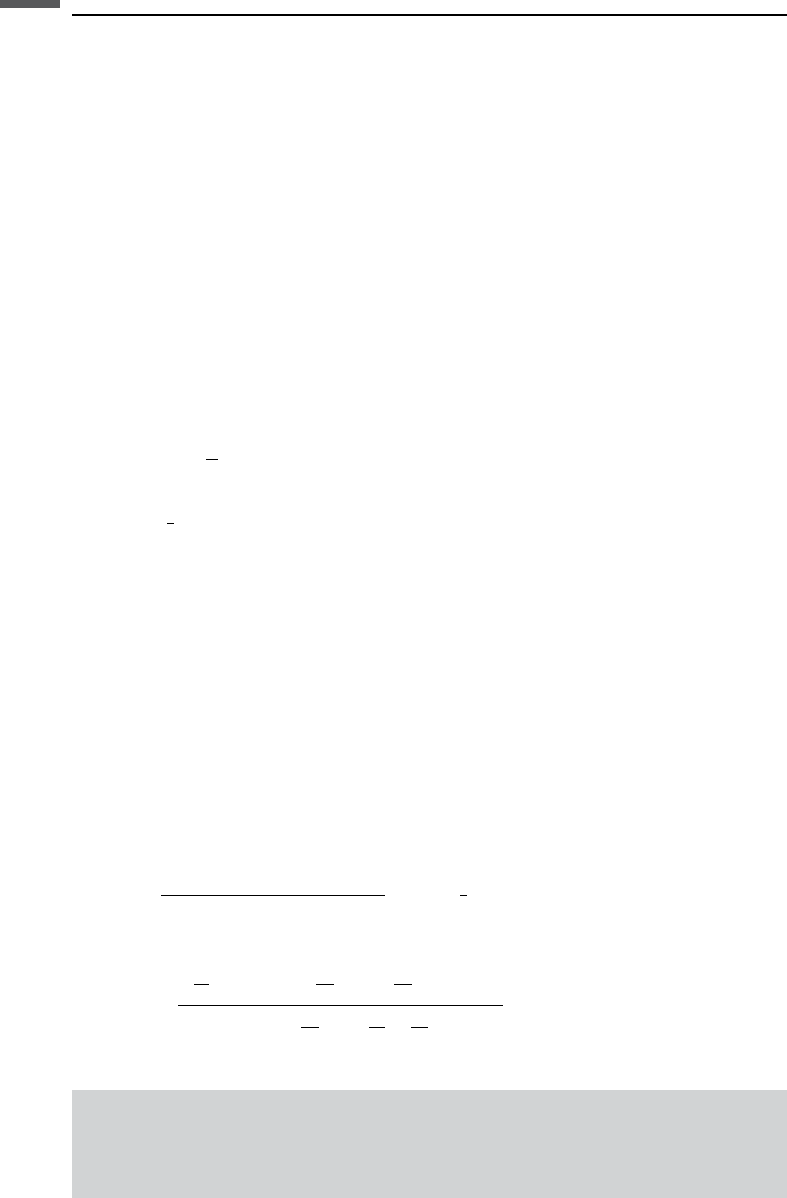
12
¼ð1 aÞ
fl
¼
0
ð1 Þþ
fl
where K
fr
and m
fr
are the effective bulk and shear moduli of the rock frame,
respectively – either the dry-frame moduli or the high-frequency, unrelaxed, “wet-frame”
moduli predicted by the Mavko–Jizba squirt theory (Section 6.10); K
0
is the bulk
modulus of the mineral material making up the rock; K
fl
is the effective bulk modulus
of the pore fluid; f is the porosity; r
0
is the mineral density; r
fl
is the fluid density;
and a is the tortuosity parameter, which is always greater than or equal to 1.
The term r
12
describes the induced mass resulting from inertial drag caused by the
relative acceleration of the solid frame and the pore fluid. The tortuosity, a (some-
times called the structure factor), is a purely geometrical factor independent of the
solid or fluid densities. Berryman (1981) obtained the relation
a ¼ 1 r 1
1
where r ¼
1
2
for spheres, and lies between 0 and 1 for other ellipsoids. For uniform
cylindrical pores with axes parallel to the pore pressure gradient, a equals 1 (the
minimum possible value), whereas for a random system of pores with all possible
orientations, a ¼3 (Stoll, 1977). The high-frequency limiting velocities depend quite
strongly on a, with higher fast P-wave velocities for lower a values.
The two solutions given above for the high-frequency limiting P-wave velocity,
designated by , correspond to the “fast” and “slow” waves. The fast wave is the
compressional body-wave most easily observed in the laboratory and the field, and it
corresponds to overall fluid and solid motions that are in phase. The slow wave is a
highly dissipative wave in which the overall solid and fluid motions are out of phase.
Another approximate expression for the high-frequency limit of the fast P-wave
velocity is (Geertsma and Smit, 1961)
V
P1
¼
(
1
0
ð1 Þþ
fl
ð1 a
1
Þ
"
K
fr
þ
4
3
m
fr
þ
fl
a
1
þ 1
K
fr
K
0
1
K
fr
K
0
2a
1
1
K
fr
K
0
1
K
0
þ
K
fl
#)
1=2
Caution
This form predicts velocities that are too high (by about 3–6%) compared with the
actual high-frequency limit.
267 6.1 Biot’s velocity relations

The complete frequency dependence can be obtained from the roots of the disper-
sion relations (Biot, 1956; Stoll, 1977; Berryman, 1980a):
H=V
2
P
fl
C=V
2
P
C=V
2
P
fl
q M=V
2
P
¼ 0
m
fr
=V
2
S
fl
fl
q
¼ 0
The complex roots are
1
V
2
P
¼
ðHq þ M 2C
fl
Þ
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ðHq þ M 2C
fl
Þ
2
4ðC
2
MHÞð
2
fl
qÞ
q
2ðC
2
MHÞ
1
V
2
S
¼
q
2
fl
qm
fr
The real and imaginary parts of the roots give the velocity and the attenuation,
respectively. Again, the two solutions correspond to the fast and slow P-waves. The
various terms are
H ¼ K
fr
þ
4
3
m
fr
þ
ðK
0
K
fr
Þ
2
ðD K
fr
Þ
C ¼
ðK
0
K
fr
ÞK
0
ðD K
fr
Þ
M ¼
K
2
0
ðD K
fr
Þ
D ¼ K
0
1 þ
K
0
K
fl
1
¼ð1 Þ
0
þ
fl
q ¼
a
fl
iFðzÞ
ok
where is the viscosity of the pore fluid, k is the absolute permeability of the rock,
and o is the angular frequency of the plane wave.
The viscodynamic operator F(z) incorporates the frequency dependence of viscous
drag and is defined by
FðzÞ¼
1
4
zTðzÞ
1 þ 2iTðzÞ=z
TðzÞ¼
ber
0
ðzÞþibei
0
ðzÞ
berðzÞþibeiðzÞ
¼
e
i3p=4
J
1
ðze
ip=4
Þ
J
0
ðze
ip=4
Þ
268 Fluid effects on wave propagation

z ¼ðo =o
r
Þ
1=2
¼
oa
2
fl
1=2
where ber( ) and bei( ) are real and imaginary parts of the Kelvin function, respectively,
J
n
( ) is a Bessel function of order n,anda is the pore-size parameter.
The pore-size parameter a depends on both the dimensions and the shape of the
pore space. Stoll (1974) found that values between
1
6
and
1
7
of the mean grain
diameter gave good agreement with experimental data from several investigators.
For spherical grains, Hovem and Ingram (1979) obtained a ¼ fd/[3(1 – f)], where d
is the grain diameter. The velocity dispersion curve for fast P-waves can be closely
approximated by a standard linear solid viscoelastic model when k/a
2
1 (see
Sections 3.8 and 6.12). However, for most consolidated crustal rocks, k/a
2
is usually
less than 1.
At very low frequencies, F(z) ! 1 and at very high frequencies (large z), the
asymptotic values are TðzÞ!ð1 þ iÞ=
ffiffiffi
2
p
and FðzÞ!ðk=4Þð1 þ iÞ=
ffiffiffi
2
p
.
The reference frequency, f
c
, which determines the low-frequency range, f f
c
, and
the high-frequency range, f f
c
, is given by
f
c
¼
2p
fl
k
One interpretation of this relation is that it is the frequency where viscous forces
acting on the pore fluid approximately equal the inertial forces acting on it. In the
high-frequency limit, the fluid motion is dominated by inertial effects, and in the low-
frequency limit, the fluid motion is dominated by viscous effects.
As mentioned above, Biot’s theory predicts the existence of a slow, highly attenuated
P-wave in addition to the usual fast P- and S-waves. The slow P-wave has been
observed in the laboratory, and it is sometimes invoked to explain diffusional loss
mechanisms.
Slow S-wave
In the Biot theory the only loss mechanism is the average motion of the fluid with
respect to the solid frame, ignoring viscous losses within the pore fluid. The fluid
strain-rate term is not incorporated into the constitutive equations. As a result,
the Biot relaxation term includes only a part of the drag force involving permeability,
but does not account for the dissipation due to shear drag within the fluid. Incorpor-
ation of the viscous term is achieved by volume averaging of the pore-scale consti-
tutive relations of the solid and fluid constituents (de al Cruz and Spanos, 1985;
Sahay et al., 2001; Spanos, 2002). This gives rise to two propagating shear processes,
corresponding to in-phase and out-of-phase shear motion of the phases, with fast and
slow S-wave velocities V
SI
and V
SII
, respectively. The slow S-wave has the charac-
teristics of a rapidly decaying viscous wave in a Newtonian fluid. The slow S-wave
269 6.1 Biot’s velocity relations

may play a role in attenuation of fast P- and S-waves by drawing energy from fast
waves due to mode conversion at interfaces and discontinuities. Incorporation of the
fluid strain-rate term introduces an additional relaxation frequency, the saturated
shear frame relaxation frequency (Sahay, 2008), given by o
b
¼ m
fr
=, which is
typically above Biot’s peak relaxation frequency. Expressions for the complex
fast and slow S-wave velocities derived from the extended theory are given by Sahay
(2008)as
V
SI;SII
¼
T
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
T
2
4
p
2
!
1=2
T ¼
m
fr
1 þ
1
1 þ i ðo
i
=oÞ
d
f
m
f
i
o
o
b
g þ
1
1 þ iðo
i
=oÞ
d
f
m
s
m
f
g
¼io
1
1 þ i ðo
i
=oÞ
m
fr
d
f
n
n ¼ =
fl
¼ kinematic shear viscosity of pore fluid
g ¼ 1 m
fr
=m
0
m
fr
; m
0
¼ shear moduli of dry rock frame and solid mineral, respectively
m
s
¼ð1 Þ
0
= ¼ solid mass fraction
m
f
¼
fl
= ¼ fluid mass fraction
d
f
¼
1
a m
f
o
i
¼ d
f
o
c
¼ d
f
2pf
c
¼
1
a m
f
fl
k
The frequency o
i
interpreted as the Biot critical frequency scaled by tortuosity, is
the peak frequency associated with Biot relaxation. Asymptotic approximations for
o o
i
and o
i
o < o
b
are derived by Sahay (2008):
V
SI
ffiffiffiffiffiffi
m
fr
r
1
i
2
o
o
i
d
f
m
f
o o
i
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
m
fr
1 m
f
=aðÞ
r
1
i
2
o
i
o
m
f
a
þ
o
o
b
g 1 a
1
þ
m
s
m
f
a
o
i
o < o
b
8
>
>
>
>
<
>
>
>
>
:
V
SII
o
ffiffiffiffiffi
n
o
i
r
o
o
i
a
2
i
o o
i
o
1=2
ffiffiffiffiffi
n
2a
r
1 iðÞo
i
o < o
b
8
>
>
>
>
<
>
>
>
>
:
270 Fluid effects on wave propagation

The slow S-wave mode in the regime above the Biot relaxation frequency is a
diffusive viscous wave with a phase velocity that has a square-root frequency
dependence, and a diffusion constant given by the kinematic shear viscosity scaled
by the tortuosity. Below the Biot relaxation frequency the viscous drag of the fluid on
the solid frame dominates over inertial effects. The slow S-wave is highly attenuating
with a linear and a quadratic frequency dependence of its attenuation and phase
velocity, respectively (Sahay, 2008).
Uses
Biot’s theory can be used for the following purposes:
estimating saturated-rock velocities from dry-rock velocities;
estimating frequency dependence of velocities; and
estimating reservoir compaction caused by pumping using the quasi-static limit of
Biot’s poroelasticity theory.
Assumptions and limitations
The use of Biot’s equations presented in this section requires the following considerations:
the rock is isotropic;
all minerals making up the rock have the same bulk and shear moduli;
the fluid-bearing rock is completely saturated; and
the pore fluid is Newtonian.
Caution
For most crustal rocks the amount of squirt dispersion (which is not included in
Biot’s formulation) is comparable to or greater than Biot’s dispersion, and thus
using Biot’s theory alone will lead to poor predictions of high-frequency saturated
velocities. Exceptions include very-high-permeability materials such as ocean
sediments and glass beads, materials at very high effective pressure, or near open
boundaries, such as at a borehole or at the surfaces of a laboratory sample. The
recommended procedure is to use the Mavko–Jizba squirt theory (Section 6.10)
first to estimate the high-frequency wet-frame moduli and then to substitute them
into Biot’s equations.
The wavelength, even in the high-frequency limit, is much larger than the grain or
pore scale.
Extensions
Biot’s theory has been extended to anisotropic media (Biot, 1962).
271 6.1 Biot’s velocity relations

6.2 Geertsma–Smit approximations of Biot’s relations
Synopsis
Biot’s theoretical formulas predict the frequency-dependent velocities of saturated
rocks in terms of the dry-rock properties (see also Biot’s relations, Section 6.1). Low-
and middle-frequency approximations (Geertsma and Smit, 1961) of his relations
may be expressed as
V
2
P
¼
V
4
P1
þ V
4
P0
f
c
=fðÞ
2
V
2
P1
þ V
2
P0
f
c
=fðÞ
2
where V
P
is the frequency-dependent P-wave velocity of saturated rock, V
P0
is the Biot–
Gassmann low-frequency limiting P-wave velocity, V
P1
is the Biot high-frequency
limiting P-wave velocity, f is the frequency, and f
c
is Biot’s reference frequency, which
determines the low-frequency range, f f
c
, and the high-frequency range, f f
c
,givenby
f
c
¼
2p
fl
k
where f is porosity, r
fl
is fluid density, is the viscosity of the pore fluid, and k is the
absolute permeabililty of the rock.
Uses
The Geertsma–Smit approximations can be used for the following:
estimating saturated-rock velocities from dry-rock velocities; and
estimating the frequency dependence of velocities.
Assumptions and limitations
The use of the Geertsma–Smit approximations presented in this section requires the
following considerations:
mathematical approximations are valid at moderate-to-low seismic frequencies, so
that f < f
c
. This generally means moderate-to-low permeabilities, but it is in this
range of permeabilities that squirt dispersion may dominate the Biot effect;
the rock is isotropic;
all minerals making up the rock have the same bulk and shear moduli; and
fluid-bearing rock is completely saturated.
Caution
For most crustal rocks the amount of squirt dispersion (not included in Biot’s
theory) is comparable to or greater than Biot’s dispersion, and thus using Biot’s
272 Fluid effects on wave propagation

theory alone will lead to poor predictions of high-frequency saturated velocities.
Exceptions include very high-permeability materials such as ocean sediments and
glass beads, or materials at very high effective pressure. The recommended
procedure is to use the Mavko–Jizba squirt theory (Section 6.10), first to estimate
the high-frequency wet-frame moduli and then to substitute them into the Biot or
Geertsma–Smit equations.
6.3 Gassmann’s relations: isotropic form
Synopsis
One of the most important problems in the rock physics analysis of logs, cores, and
seismic data is using seismic velocities in rocks saturated with one fluid to predict
those of rocks saturated with a second fluid, or equivalently, predicting saturated-rock
velocities from dry-rock velocities, and vice versa. This is the fluid substitution
problem.
Generally, when a rock is loaded under an increment of compression, such as from a
passing seismic wave, an increment of pore-pressure change is induced, which resists
the compression and therefore stiffens the rock. The low-frequency Gassmann–Biot
(Gassmann, 1951; Biot, 1956) theory predicts the resulting increase in effective bulk
modulus, K
sat
, of the saturated rock using the following equation:
K
sat
K
0
K
sat
¼
K
dry
K
0
K
dry
þ
K
fl
ðK
0
K
fl
Þ
; m
sat
¼ m
dry
where K
dry
is the effective bulk modulus of dry rock, K
sat
is the effective bulk
modulus of the rock with pore fluid, K
0
is the bulk modulus of mineral material
making up rock, K
fl
is the effective bulk modulus of pore fluid, f is the porosity, m
dry
is the effective shear modulus of dry rock, and m
sat
is the effective shear modulus of
rock with pore fluid.
Gassmann’s equation assumes a homogeneous mineral modulus and statistical
isotropy of the pore space but is free of assumptions about the pore geometry. Most
importantly, it is valid only at sufficiently low frequencies such that the induced pore
pressures are equilibrated throughout the pore space (i.e., there is sufficient time for
the pore fluid to flow and eliminate wave-induced pore-pressure gradients). This
limitation to low frequencies explains why Gassmann’s relation works best for very
low-frequency in-situ seismic data (<100 Hz) and may perform less well as frequen-
cies increase toward sonic logging (10
4
Hz) and laboratory ultrasonic measurements
(10
6
Hz).
273 6.3 Gassmann’s relations: isotropic form

Caution: “dry rock” is not the same as gas-saturated rock
The “dry-rock” or “dry-frame” modulus refers to the incremental bulk deformation
resulting from an increment of applied confining pressure with the pore pressure
held constant. This corresponds to a “drained” experiment, in which pore fluids
can flow freely in or out of the sample to ensure constant pore pressure. Alterna-
tively, the “dry-frame” modulus can correspond to an undrained experiment in
which the pore fluid has zero bulk modulus, and in which pore compression
therefore does not induce changes in pore pressure. This is approximately the case
for an air-filled sample at standard temperature and pressure. However, at reservoir
conditions (high pore pressure), gas takes on a non-negligible bulk modulus and
should be treated as a saturating fluid.
Caution
Laboratory measurements on very dry rocks, such as those prepared in a vacuum
oven, are sometimes too dry. Several investigators have found that the first few
percent of fluid saturation added to an extremely dry rock will lower the frame
moduli, probably as a result of disrupting surface forces acting on the pore
surfaces. It is this slightly wet or moist rock modulus that should be used as the
“dry-rock” modulus in Gassmann’s relations. A more thorough discussion is given
in Section 6.17.
Although we often describe Gassmann’s relations as allowing us to predict saturated-
rock moduli from dry-rock moduli, and vice versa, the most common in-situ problem
is to predict the changes that result when one fluid is replaced with another. One
procedure is simply to apply the equation twice: transform the moduli from the initial
fluid saturation to the dry state, and then immediately transform from the dry moduli
to the new fluid-saturated state. Equivalently, we can algebraically eliminate the dry-
rock moduli from the equation and relate the saturated-rock moduli K
sat 1
and K
sat 2
in
terms of the two fluid bulk moduli K
fl 1
and K
fl 2
as follows:
K
sat 1
K
0
K
sat 1
K
fl 1
ðK
0
K
fl 1
Þ
¼
K
sat 2
K
0
K
sat 2
K
fl 2
ðK
0
K
fl 2
Þ
Caution
It is not correct simply to replace K
dry
in Gassmann’s equation by K
sat 2
.
274 Fluid effects on wave propagation

A few more explicit, but entirely equivalent, forms of the equations are
K
sat
¼K
dry
þ
1 K
dry
=K
0
2
=K
fl
þð1 Þ=K
0
K
dry
=K
2
0
K
sat
¼
1=K
0
1=K
fl
ðÞþ1=K
0
1=K
dry
ð=K
dry
Þ 1=K
0
1=K
fl
ðÞþð1=K
0
Þ 1=K
0
1=K
dry
1
K
sat
¼
1
K
0
þ
K
þ K
0
K
fl
=ðK
0
K
fl
Þ
K
dry
¼
K
sat
K
0
=K
fl
þ 1 ðÞK
0
K
0
=K
fl
þ K
sat
=K
0
1
Note that the dry pore-space compressibility is
1
K
¼
1
v
p
]v
p
]
P
where v
p
is the pore volume, s is the confining pressure, and P is the pore pressure.
Use Gassmann’s relation to compare the bulk modulus of a dry quartz sandstone
having the properties f ¼0.20, K
dry
¼12 GPa, and K
0
¼36 GPa with the bulk
moduli when the rock is saturated with gas and with water at T ¼80
C and pore
pressure P
p
¼300 bar.
Calculate the bulk moduli and density for gas and for water using the Batzle–
Wang formulas discussed in Section 6.21. A gas with gravity 1 will have the
properties K
gas
¼0.133 GPa and r
gas
¼0.336 g/cm
3
, and water with salinity
50 000 ppm will have properties K
water
¼3.013 GPa and r
water
¼1.055 g/cm
3
.
Next, substitute these into Gassmann’s relations
K
sat
-
gas
36 K
sat
-
gas
¼
12
36 12
þ
0:133
0:20ð36 0:133 Þ
to yield a value of K
sat-gas
¼12.29 GPa for the gas-saturated rock. Similarly,
K
sat
-
water
36 K
sat
-
water
¼
12
36 12
þ
3:013
0:20ð36 3:013Þ
which yields a value of K
sat-water
¼17.6 GPa for the water-saturated rock.
Compressibility form
An equivalent form of Gassmann’s isotropic relation can be written compactly in
terms of compressibilities:
C
sat
C
0
ðÞ
1
¼ C
dry
C
0
1
þ C
fl
C
0
ðÞ½
1
275 6.3 Gassmann’s relations: isotropic form

where
C
sat
¼
1
K
sat
C
dry
¼
1
K
dry
C
fl
¼
1
K
fl
C
0
¼
1
K
0
Reuss average form
An equivalent form can be written as
K
sat
K
0
K
sat
¼
K
dry
K
0
K
dry
þ
K
R
K
0
K
R
where
K
R
¼
K
fl
þ
1
K
0
1
is the Reuss average modulus of the fluid and mineral at porosity f. This is consistent
with the obvious result that when the dry-frame modulus of the rock goes to zero, the
fluid-saturated sample will behave as a suspension and lie on the Reuss bound.
Linear form
A particularly useful exact linear form of Gassmann’s relation follows from the
simple graphical construction shown in Figure 6.3.1 (Mavko and Mukerji, 1995).
Draw a straight line from the mineral modulus on the left-hand axis (at f ¼0) through
the data point (A) corresponding to the rock modulus with the initial pore fluid (in this
case, air). The line intersects the Reuss average for that pore fluid at some porosity,
f
R
, which is a measure of the pore-space stiffness. Then the rock modulus (point A
0
)
for a new pore fluid (in this case, water) falls along a second straight line from the
mineral modulus, intersecting the Reuss average for the new pore fluid at f
R
. Then
we can write, exactly,
K
Gass
ðÞ¼
R
K
R
R
ðÞ
where DK
Gass
¼K
sat 2
– K
sat 1
is the Gassmann-predicted change of saturated-rock
bulk modulus between any two pore fluids (including gas), and DK
R
(f
R
) is the
difference in the Reuss average for the two fluids evaluated at the intercept
porosity f
R
.
Because pore-fluid moduli are usually much smaller than mineral moduli, we can
approximate the Reuss average as
K
R
ð
R
Þ¼
K
fl
K
0
R
K
0
þð1
R
ÞK
fl
K
fl
R
276 Fluid effects on wave propagation
