Mavko G., Mukerji T., Dvorkin J. The Rock Physics Handbook

Подождите немного. Документ загружается.

bodies. The normal stiffness of the two-grain system remains the same as that given

by the Hertz solution. The shear stiffness is

8amð1  F

=F

1=3

2  n

; F

 F

where v and m are the Poisson ratio and shear modulus of the solid grains, respect-

ively, and F

and F

are the normal and tangential forces applied to the spheres,

respectively. Typically, if we are only concerned with acoustic wave propagation,

the normal force, which is due to the confining (overburden) stress imposed on the

granular system, is much larger than the tangential force, which is imposed by

the very small oscillatory stress due to the propagating wave: F

 F

. As a result, the

shear and normal stiffnesses are as follows (the latter being the same as in the Hertz

solution):

8am

2  n

; S

4am

1  n

The effective shear modulus of a dry, random, identical-sphere packing is then

eff

5  4n

5ð2  nÞ

ð1  Þ

ð1  nÞ

1=3

The ratio of the bulk modulus to the shear modulus and the effective Poisson ratio of

the dry frame of this system are

eff

5ð2  nÞ

3ð5  4nÞ

; n

eff

2ð5  3nÞ

The Hertz–Mindlin model can be used to describe the properties of precompacted

granular rocks.

One may also consider the case of absolutely frictionless spheres, where  ¼0

( ! 0; as F

=F

ðÞ!1) and therefore S

¼0. In this case, the effective bulk and

shear moduli of the pack become

eff

Cð1  Þ

12pR

; m

eff

Cð1  Þ

20pR

;

eff

and the effective Poisson ratio becomes exactly 0.25.

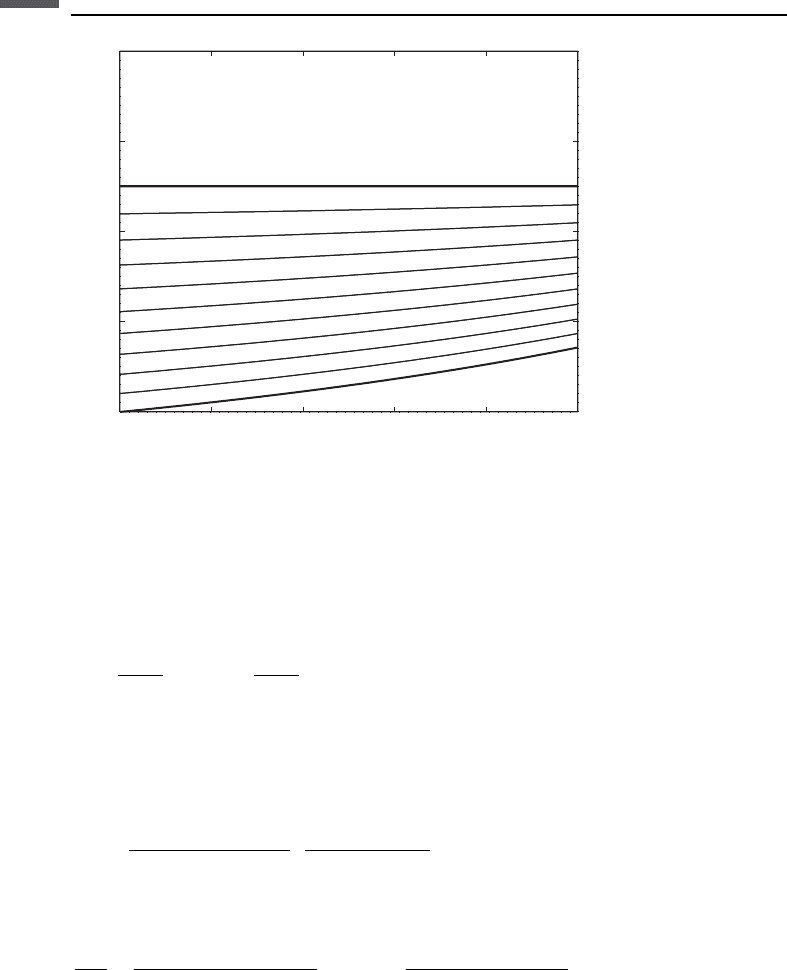
Figure 5.4.2 demonstrates that there is a large difference between the effective

Poisson ratio of a dry frictionless pack and that of a dry pack with perfect adhesion

between the particles. In the latter case, the effective Poisson ratio does not exceed 0.1

regardless of the grain material.

The absence of friction between particles may conceivably occur in an unconsoli-

dated system because of the presence of a lubricant at some contacts. It is virtually

impossible to predict in advance which fraction of the individual contacts is

247 5.4 Random spherical grain packings

frictionless (S

¼0), and which has perfect adhesion (S

¼ 8am=ð2  nÞ). To account

for all possibilities, an ad hoc coefficient f ð0  f  1Þ can be introduced such that

4am

1  n

; S

¼ f

8am

2  n

and the grains have perfect adhesion for f ¼1 and no friction for f ¼0. As a result, the

effective bulk modulus of the pack remains the same, but the expression for the

effective shear modulus becomes

eff

2 þ 3f  nð1 þ 3f Þ

5ð2  n Þ

ð1  Þ

ð1  nÞ

1=3

and

eff

5ð2  n Þ

3½2 þ 3f  nð1 þ 3f Þ

; n

eff

2  2f þ nð2f  1Þ

2½4 þ f  nð2 þ f Þ

Figure 5.4.2 demonstrates how the Poisson ratio of the dry pack gradually moves

from the frictionless line down to the perfect-adhesion curve.

The Walton model

It is assumed in the Walton model (Walton, 1987) that normal and shear deformation

of a two-grain combination occur simultaneously. This assumption leads to results

0 0.1 0.2 0.3 0.4 0.5

0.1

0.2

0.3

0.4

Poisson ratio of

rain material

Poisson ratio of grain pack

Perfect adhesion, f = 1

Frictionless spheres, f = 0

Figure 5.4.2 Poisson ratio of a grain pack versus that of the grain material. The upper bold curve

is for frictionless spheres while the lower bold curve is for spheres with perfect adhesion.

The thin curves in between are for values of f varying between 0 and 1, with a step size of 0.1.

248 Granular media

somewhat different from those given by the Hertz–Mindlin model. Specifically, there

is no partial slip in the contact area. The slip occurs across the whole area once

applied tractions exceed the friction resistance. The results discussed in the following

paragraphs are given for two special cases: infinitely rough spheres (where the

friction coefficient is very large) and ideally smooth spheres (where the friction

coefficient is zero).

Under hydrostatic pressure P, an identical-sphere random packing is isotropic.

Its effective bulk and shear moduli for the rough-spheres case (dry pack) are

described by

eff

3ð1  Þ

1=3

;

A ¼



m þ l



;

eff

5B þ A

2B þ A

B ¼

m þ l



where l is Lame

’s coefficient of the grain material. For the smooth-spheres case

(dry pack),

eff

3ð1  Þ

1=3

; K

eff

It is clear that the effective density of the aggregate is



eff

¼ð1  Þ

Under uniaxial pressure s

, a dry, identical-sphere packing is transversely isotropic,

and if the spheres are infinitely rough, it can be described by the following five

constants:

¼ 3ða þ 2bÞ; C

¼ a  2b; C

¼ 2C

¼ 8ða þ bÞ; C

¼ a þ 7b

where

a ¼

ð1  ÞCe

32p

; b ¼

ð1  ÞCe

32p

ð2B þ AÞ

e ¼

24p

Bð2B þ AÞ

ð1  ÞAC



1=3

The Digby model

The Digby model gives effective moduli for a dry, random packing of identical

elastic spherical particles. Neighboring particles are initially firmly bonded across

small, flat, circular regions of radius a. Outside these adhesion surfaces, the shape of

249 5.4 Random spherical grain packings

each particle is assumed to be ideally smooth (with a continuous first derivative).

Notice that this condition differs from that of Hertz, where the shape of a particle

is not smooth at the intersection of the spherical surface and the plane of

contact. Digby’s normal and shear stiffnesses under hydrostatic pressure P are

(Digby, 1981)

4mb

1  n

; S

8ma

2  n

where v and m are the Poisson ratio and shear modulus of the grain material,

respectively. Parameter b can be found from the relation

¼ d





1=2

where d satisfies the cubic equation



d 

3pð1  nÞP

2Cð1  Þm

¼ 0

Use the Digby model to estimate the effective bulk and shear moduli for a dry

random pack of spherical grains under a confining pressure of 10 MPa. The ratio of

the radius of the initially bonded area to the grain radius a/R is 0.01. The bulk and

shear moduli of the grain material are K ¼37 GPa and m ¼44 GPa, respectively.

The porosity of the grain pack is 0.36.

The Poisson ratio v for the grain material is calculated from K and m:

n ¼

3K  2m

2ð3K þ mÞ

¼ 0:07

The coordination number C ¼9. Solving the cubic equation for d



d 

3pð1  nÞP

2Cð1  Þm

¼ 0

and taking the real root, neglecting the pair of complex conjugate roots, we obtain

d ¼0.0547. Next, we calculate b/R as

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ



¼ 0:0556

The values of a/R and b/R are used to compute S

/R and S

/R:

4mðb=RÞ

1  n

¼ 10:5

8mða=RÞ

2  n

¼ 1:8

250 Granular media

which then finally give us

eff

Cð1  Þ

12p

ðS

=RÞ¼1:6 GPa

and

eff

Cð1  Þ

20p

½ðS

=RÞþ1:5ðS

=RÞ ¼ 1:2 GPa

The Jenkins et al. model

Jenkins et al. (2005) derive expressions for the effective moduli of a random packing

of identical frictionless spheres. Their approach differs from that of Digby (1981),

Walton (1987), and Hertz–Mindlin in that under applied deviatoric strain, the particle

motion of each sphere relative to its neighbors is allowed to deviate from the mean

homogeneous strain field of a corresponding homogeneous effective medium. Under

hydrostatic compression, the grain motion is consistent with homogeneous strain. The

additional degrees of freedom of particle motion result in calculated shear moduli that

are smaller than predicted by the above-mentioned models.

The expressions for dry effective shear modulus, m

, and effective Lame

con-

stant, l

, in terms of the coordination number, C, grain diameter, d, and porosity,

f, are

Cð1  Þ

5pd

f1  2½

1

ðo

þ 2o

Þ

2

ðk

þ 2k

3

ðx

þ 2x

Þg

Cð1  Þ

5pd

f1  2

1

ðo

þ 7o

Þþ2

2

ðk

þ 2k

þ 5k

 2

3

ðx

þ 2x

þ 5x

Þg

where

¼ C=3

¼ 166  11CðÞ=128

¼ 38  11CðÞ=128

¼C þ 14ðÞ=128

¼ 19C  22ðÞ=48

¼ 22  3CðÞ=16

¼ 18  9CðÞ=48

251 5.4 Random spherical grain packings

¼ða

þ 2

Þþ

½0:52ðC  2ÞðC  4Þ

þ 0:10CðC  2Þ0:13CðC  4Þ0:01C

=16p

¼a

þ o

þ½0:44ðC  2ÞðC  4Þ0:24CðC  2Þ

 0:11CðC  4Þ0:14C

=16p

¼ða

Þþo

½0:44ðC  2ÞðC  4Þ0:42CðC  2Þ

 0:11CðC  4Þþ0:04C

=16p



¼a

þ a

þ½1:96 C  2ðÞC  4ðÞþ3:30CC 2ðÞ

þ 0:49CC 4ðÞþ0:32C

=16p



¼ 2a



½2:16 C  2ðÞC  4ðÞþ2:30CC 2ðÞ

þ 0:54CC 4ðÞ0:06C

=16p

¼ 

þ 

þ 2

ðÞ

¼ 

þ 

ðÞ

In the above expressions, K

is the contact normal stiffness determined from

Hertz–Mindlin theory:

1  nðÞ

1  ðÞ



1=3

where m and n are the shear modulus and Poisson ratio of the solid material making up

the grains, and P is the pressure. Note that the normal stiffness K

defined above is

related to the S

, defined earlier, as K

¼S

/2. The difference occurs because S

defined in terms of the incremental change in grain radius, R, under compression,

while K

is defined in terms of the incremental change in grain diameter, d, under

compression.

The Brandt model

The Brandt model allows one to calculate the bulk modulus of randomly packed

elastic spheres of identical mechanical properties but different sizes. This packing is

subject to external and internal hydrostatic pressures. The effective pressure P is the

difference between these two pressures. The effective bulk modulus is (Brandt, 1955)

eff

1=3

9

1:75ð1  n



2=3

Z  1:5PZ

Z ¼

ð1 þ 30:75zÞ

5=3

1 þ 46:13z

; z ¼

3=2

ð1  v

ﬃﬃﬃ

In this case E is the mineral Young’s modulus and K is the fluid bulk modulus.

252 Granular media

The Johnson et al. model

Norris and Johnson (1997) and Johnson et al. (1998) have developed an effective

medium theory for the nonlinear elasticity of granular sphere packs, generalizing the

earlier results of Walton, based on the underlying Hertz–Mindlin theory of grain-to-

grain contacts. Johnson et al. (1998) show that for this model, the second-order elastic

constants, C

ijkl

, are unique path-independent functions of an arbitrary strain environ-

ment e

. However, the stress tensor s

depends on the strain path, and consequently

ijkl

(considered as a function of applied stresses) are path dependent. For a pack of

identical spheres of radius R with no-slip Hertz–Mindlin contacts, the elastic con-

stants in the effective medium approximation are given by Johnson et al. (1997)as

ijkl

3nð1  Þ

1=2

ð2B

þ C

 x

1=2

þ B

ðd

þ d



where hi denotes an average over all solid angles. f is porosity, n is the coordin-

ation number or average number of contacts per grain, N

is the unit vector along

sphere centers, and x N

R is the normal component of displacement at the

contacts:





1  n

2  n

where m

and n

are the shear modulus and the Poisson ratio of individual grains,

respectively.

When the strain is a combination of hydrostatic compression and uniaxial com-

pression (along the 3-axis),

¼ ed

þ e

;

the sphere pack exhibits transversely isotropic symmetry. Assuming the angular

distribution of contacts to be isotropic, explicit expressions for the five independent

elements of the stiffness tensor are given as (Johnson et al., 1998):

 C

1111

ðaÞI

ðaÞ½þ

ðaÞ2I

ðaÞþI

ðaÞ½

253 5.4 Random spherical grain packings

 C

1133

ðaÞI

ðaÞ½

 C

3333

ðaÞþ2C

ðaÞ½

 C

2323

ðaÞþI

ðaÞ½þC

ðaÞI

ðaÞ½

 C

1212

ðaÞI

ðaÞ

½

ðaÞ2I

ðaÞþI

ðaÞ

½

where

a ¼

ﬃﬃﬃﬃﬃﬃﬃﬃﬃ

e=e

g ¼

3nð1  ÞðeÞ

1=2

ð2B

þ C

ð1  ÞðeÞ

1=2

The integral I

ðaÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

þ x

dx can be evaluated analytically as

ðaÞ¼

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 þ a

þ a

1 þ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 þ a

!"#

ðaÞ¼

1 þ a



3=2

a

ðaÞ

ðaÞ¼

1 þ a



3=2

3a

ðaÞ

The results of the Johnson et al. model are consistent with the Walton model in the

limiting cases of pure hydrostatic strain (e

! 0) and pure uniaxial strain (e ! 0).

When a small uniaxial strain is superimposed on a large isotropic strain, the equations

can be expanded and simplified to the first order in e

/e (Johnson et al., 1998), giving

¼ g



¼ g



¼ g



¼ g



¼ g

105



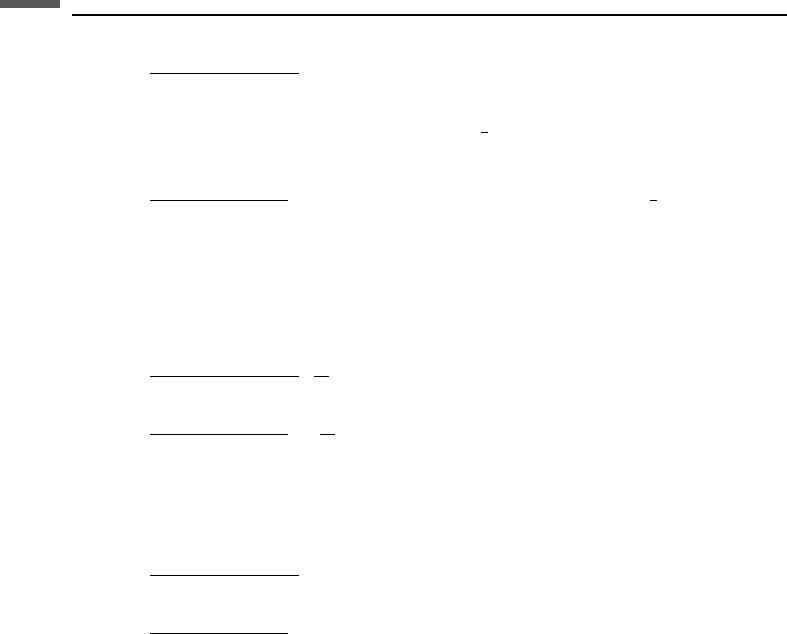
The components of the stress tensor s

depend on the strain path. When the system

first undergoes hydrostatic strain followed by a uniaxial strain, the nonzero compon-

ents of the stress tensor, s

and s

¼s

are given by

254 Granular media



¼

2ðeÞ

3=2

ð1  Þn

4pa

 C

ðaÞþð1  a

ÞI

ðaÞI

ðaÞ



þ C

ðaÞþI

ðaÞ





¼

ðeÞ

3=2

ð1  Þn

4pa

 C

ðaÞþð1  a

ÞI

ðaÞI

ðaÞ



þC

ðaÞþð1  a

ÞI

ðaÞI

ðaÞ



When a uniaxial compression is followed by an isotropic compression, the stresses are



¼

2ðeÞ

3=2

ð1  Þn

4pa

þ C

ðaÞþI

ðaÞ





¼

ðeÞ

3=2

ð1  Þn

4pa



þ C

ðaÞþð1  a

ÞI

ðaÞI

ðaÞ



and finally when the two strain components are applied simultaneously the stress

components are



¼

2ðeÞ

3=2

ð1  Þn

4pa

ðaÞI

ðaÞ½þC

ðaÞþI

ðaÞ





¼

ðeÞ

3=2

ð1  Þn

4pa

C

ðaÞI

ðaÞ½

þ C

ðaÞþð1  a

ÞI

ðaÞI

ðaÞ



The differences in the stresses for the three different strain paths are quite small

(Johnson et al., 1998).

The concept of third-order elastic constants described by nonlinear hyperelasticity

with three third-order constants breaks down for unconsolidated granular media with

uniaxial strain superposed on a large hydrostatic strain. The changes in second-order

elastic constants due to an imposed strain are governed (to the first order) by four

“third-order” moduli and not just the usual three third-order moduli (Norris and

Johnson, 1997). In granular media, the contact forces are path dependent and are

not derivable from a potential energy function. As a result, the system cannot be

described by an equivalent nonlinear hyperelastic medium.

The cemented-sand model

The cemented-sand model allows one to calculate the bulk and shear moduli of dry

sand in which cement is deposited at grain contacts. The cement is elastic and its

properties may differ from those of the spheres.

It is assumed that the starting framework of cemented sand is a dense, random pack

of identical spherical grains with porosity f

 0.36 and an average number of

255 5.4 Random spherical grain packings

contacts per grain C ¼9. Adding cement to the grains reduces porosity and increases

the effective elastic moduli of the aggregate. Then, these effective dry-rock bulk and

shear moduli are (Dvorkin and Nur, 1996)

eff

Cð1  

ÞM

eff

Cð1  

Þm

¼ 

where r

is the density of the cement and V

and V

are its P- and S-wave velocities,

respectively. Parameters

and

are proportional to the normal and shear stiff-

nesses, respectively, of a cemented two-grain combination. They depend on the

amount of contact cement and on the properties of the cement and the grains as

defined in the following relations:

¼ A

þ B

a þ C

¼0:024153 

1:3646

¼ 0:20405 

0:89008

¼ 0:000246 49 

1:9864

¼ A

þ B

a þ C

¼10

2

ð2:26n

þ 2:07n þ 2:3Þ

0:079n

þ0:1754n1:342

¼ð0:0573n

þ 0:0937n þ0:202Þ

0:0274n

þ0:0529n0:8765

¼ 10

4

ð9:654n

þ 4:945n þ 3:1Þ

0:01867n

þ0:4011n1:8186



ð1  nÞð1 n

ð1  2n



a ¼

where m and v are the shear modulus and the Poisson ratio of the grains, respectively;

and v

are the shear modulus and the Poisson ratio of the cement, respectively; a is

the radius of the contact cement layer; and R is the grain radius.

The amount of contact cement can be expressed through the ratio a of the radius of

the cement layer a to the grain radius R:

a ¼

The radius a of the contact cement layer is not necessarily directly related to the total

amount of cement; part of the cement may be deposited away from the intergranular

contacts. However, by assuming that porosity reduction in sands is due to

256 Granular media