Patrick F. Dunn, Measurement, Data Analysis, and Sensor Fundamentals for Engineering and Science, 2nd Edition
Подождите немного. Документ загружается.

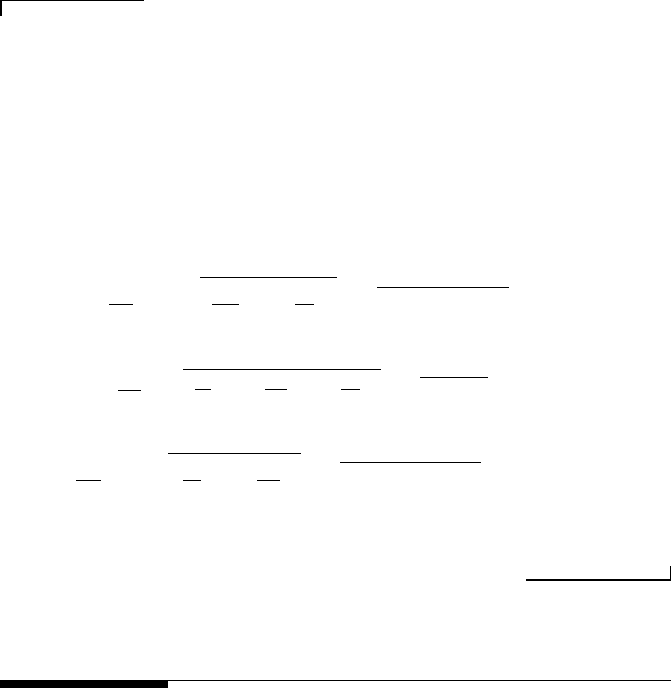
256 Measurement and Data Analysis for Engineering and Science
Finally, one may be interested in estimating the uncertainty of a result
that can be found by different measurement approaches. This process is
quite useful during the planning stage of an experiment. For example, take
the simple case of an experiment designed to determine the volume of a
cylinder, V . One approach would be to measure the cylinder’s height, h,
and diameter, d, and then compute its volume based upon the expression
V = πd
2
h/4. An alternative approach would be to obtain its weight, W , and
then compute its volume according to the expression V = W/(ρg), where
ρ is the density of the cylinder and g the local gravitational acceleration.
Which approach is chosen depends on the uncertainties in d, h, w, ρ, and g.
Example Problem 7.12
Statement: An experiment is being designed to determine the mass of a cube of
Teflon. Two approaches are under consideration. Approach A involves determining the
mass from a measurement of the cube’s weight and approach B from measurements
of the cube’s length, width, and height (l, w, and h, respectively). For approach A,
m = W/g; for approach B, m = ρV , where m is the mass, W the weight, g the
gravitational acceleration (9.81 m/s
2
), ρ the density (2 200 kg/m
3
), and V the volume
(lwh). The fractional uncertainties in the measurements are W (2 %), g (0.1 %), ρ,
(0.1 %), and l, w, and h (1 %). Determine which approach has the least uncertainty in
the mass.
Solution: Because both equations for the mass involve products or quotients of
the measurands, the fractional uncertainty of the mass can be computed using Equa-
tion 7.39. For approach A
u
m
|m|
A
=
s
u
W
W
2
+
u
g
g
2
=
q
(0.02)
2
+ (0.001)
2
= 2.0 %.
For approach B, the fractional uncertainty must be determined in the volume. This is
u
V
|V |
=
r
u
l
l
2
+
u
w
w
2
+
u
h
h
2
=
p
3 × 0.01
2
= 1.7 %.
This result can be incorporated into the fractional uncertainty calculation for the mass
u
m
|m|
B
=
s
u
ρ
ρ
2
+
u
V
V
2
=
q
(0.001)
2
+ (0.017)
2
= 0.017 = 1.7 %.
Thus, approach B has the least uncertainty in the mass. Note that the uncertainties in
g and ρ both are negligible in these calculations.
7.10 Detailed Uncertainty Analysis
Detailed uncertainty analysis is appropriate for measurement situations that
involve multiple measurements, either for a measurand or a result. System-
atic and random errors are identified in this approach. Their contributions

Uncertainty Analysis 257
to the overall uncertainty are treated separately in the analysis until they
are combined at the end. Detailed uncertainty analysis is performed after a
statistically viable set of measurand values has been obtained under fixed
operating conditions. Multiple measurements usually are made for one or
both of two reasons. One is to assess the uncertainty present in an exper-
iment due to uncontrollable variations in the measurands. The other is to
obtain sufficient data such that the average value of a measurand can be
estimated. Thus, detailed uncertainty calculations are more extensive than
for the cases of a single-measurement measurand or result.
In general, for the situation in which both systematic and random er-
rors are considered, application of Equation 7.19 for a result based upon J
measurands leads directly to
u
2
r
=
J
X
i=1
θ
i
2
S
2
B
i
+ 2
J−1
X
i=1
J
X
j=i+1
θ
i
θ
j
S
B
i
,B
j
+
J
X
i=1
θ
i
2
S
2
P
i
+ 2
J−1
X
i=1
J
X
j=i+1
θ
i
θ
j
S
P
i
,P
j
,
(7.47)
where
S
2
B
i
=
M
B
X
k=1
(S
B
i
)
2
k
, (7.48)
S
2
P
i
=
M
P
X
k=1
(S
P
i
)
2
k
, (7.49)
S
B
i
,B
j
=
L
B
X
k=1
(S
B
i
)
k
(S
B
j
)
k
, (7.50)
and
S
P
i
,P
j
=
L
P
X
k=1
(S
P
i
)
k
(S
P
j
)
k
. (7.51)
M
B
is the number of elemental systematic uncertainties, M
P
is the num-
ber of elemental random uncertainties. (S
B
i
)
k
represents the k-th elemental
error out of M
B
elemental errors contributing to S
B
i
, the estimate of the
systematic error of the i-th measurand. Equation 7.48 is analogous to Equa-
tion 7.27 for the case of a single-measurement measurand. Further, L
B
is
the number of systematic errors that are common to B
i
and B
j
, and L
P
the number of random errors that are common to P
i
and P
j
. S
2
B
i
and S
2
P
i
are estimates of the variances of the systematic and random errors of the
i-th measurand, respectively. S
B
i
,B
j
and S
P
i
,P
j
are estimates of the covari-
ances of the systematic and random errors of the i-th and j-th measurands,
respectively.
The number of effective degrees of freedom of a multiple-measurement
result, based upon the Welch-Satterthwaite formula, is
ν
r
=
{
P
J
i=1
[θ
2
i
S
2
B
i
+ θ
2
i
S
2
P
i
]}
2
P
J
i=1
h
(θ
4
i
S
4
P
i
)/ν
P
i
) + (
P
M
B
k=1
θ
4
i
(S
B
i
)
4
k
/ν
(S
B
i
)
k
)
i
, (7.52)

258 Measurement and Data Analysis for Engineering and Science
where contributions are made from each i-th measurand. The random and
systematic numbers of degrees of freedom are given by
ν
P
i
= N
i
− 1, (7.53)
where N
i
denotes N measurements of the i-th measurand and
ν
(S
B
i
)
k
∼
=
1
2
∆(S
B
i
)
k
(S
B
i
)
k
−2
. (7.54)
When S
B
i
and S
P
i
have similar values, the value of ν
r
, as given by Equa-
tion 7.52, is approximately that of ν
P
i
if ν
B
i
>> ν
P
i
. Conversely, the value
of ν
r
is approximately that of ν
B
i
if ν
P
i
>> ν
B
i
. Further, if S
B
i
<< S
P
i
,
then the value of ν
r
is approximately that of ν
P
i
. The converse also is true.
Once ν
r
is determined from Equation 7.52, the overall uncertainty in the
result, U
r
, can be found. This can be expressed using the definition of the
overall uncertainty (Equation 7.18) and Equation 7.47 as
U
2
r
= t
2
ν
r
,C
u
2
r
= B
2
r
+ (t
ν
r
,C
S
r
)
2
= B
2
r
+ P
2
r
, (7.55)
where
B
r
= t
ν
r
,C
J
X
i=1
θ
i
2
S
2
B
i
+ 2
J−1
X
i=1
J
X
j=i+1
θ
i
θ
j
S
B
i
,B
j
1/2
(7.56)
and
P
r
= t
ν
r
,C
J
X
i=1
θ
i
2
S
2
P
i
+ 2
J−1
X
i=1
J
X
j=i+1
θ
i
θ
j
S
P
i
,P
j
1/2
. (7.57)
The first term on the right side of Equation 7.56 is the sum of the es-
timated variances of of the systematic errors. The second term is the sum
of the estimated covariances of the systematic errors. Likewise, for Equa-
tion 7.57, the first term is the estimated variance of the random errors and
the second term is the estimated covariance of the random errors. The co-
variance of two systematic errors is zero if the errors are not correlated, or, in
other words, they are independent of each other. Non-zero covariances arise
when the error sources are common, such as when two pressure transducers
are calibrated against the same standard. Correlated random errors can be
identified by examining the amplitude variations in time of two measurands.
When they follow the same trends, they may be correlated. Usually, how-
ever, the covariances of the systematic errors are assumed negligible in most
uncertainty analysis.

Uncertainty Analysis 259
When there are no correlated uncertainties,
u
2
r
=
J
X
i=1
θ
i
2
S
2
B
i
+
J
X
i=1
θ
i
2
S
2
P
i
, (7.58)
where
B
r
= t
ν
r
,C
"
J
X
i=1
θ
i
2
S
2
B
i
#
1/2
(7.59)
and
P
r
= t
ν
r
,C
"
J
X
i=1
θ
i
2
S
2
P
i
#
1/2
. (7.60)
For the situation when an experiment is replicated M times and a mean
result is determined from the M individual results, the expression for S
r
in
Equation 7.55 is replaced by either
S
r
=
v
u
u
t
1
M − 1
M
X
k=1
(r
k
− ¯r)
2
(7.61)
or
S
¯r
= S
r
/
√
M, (7.62)
depending upon which outcome is desired (r or ¯r, respectively) [10]. The
Welch-Satterthwaite formula is then
ν
r
=
{S
2
r
+
P
J
i=1
(θ
2
i
S
2
B
i
)}
2
(S
4
r
/ν
S
r
) +
P
J
i=1
P
M
B
k=1
h
(θ
4
i
(S
B
i
)
4
k
)/ν
(S
B
i
)
k
)
i
, (7.63)
with ν
S
r
= M − 1.
Equations 7.47 and 7.52 can be applied to estimate the uncertainty in a
multiple-measurement measurand. For this case, these equations reduce to
u
2
m
=
J
X
i=1
S
2
B
i
+ 2
J−1
X
i=1
J
X
j=i+1
S
B
i
,B
j
+
J
X
i=1
S
2
P
i
+ 2
J−1
X
i=1
J
X
j=i+1
S
P
i
,P
j
(7.64)
and
ν
m
=
{
P
J
i=1
[S
2
B
i
+ S
2
P
i
]}
2
P
J
i=1
h
(S
4
P
i
/ν
P
i
) + (
P
M
B
k=1
(S
B
i
)
4
k
/ν
(S
B
i
)
k
)
i
. (7.65)
Further simplification occurs where there are no correlated uncertainties.
Equation 7.64 becomes
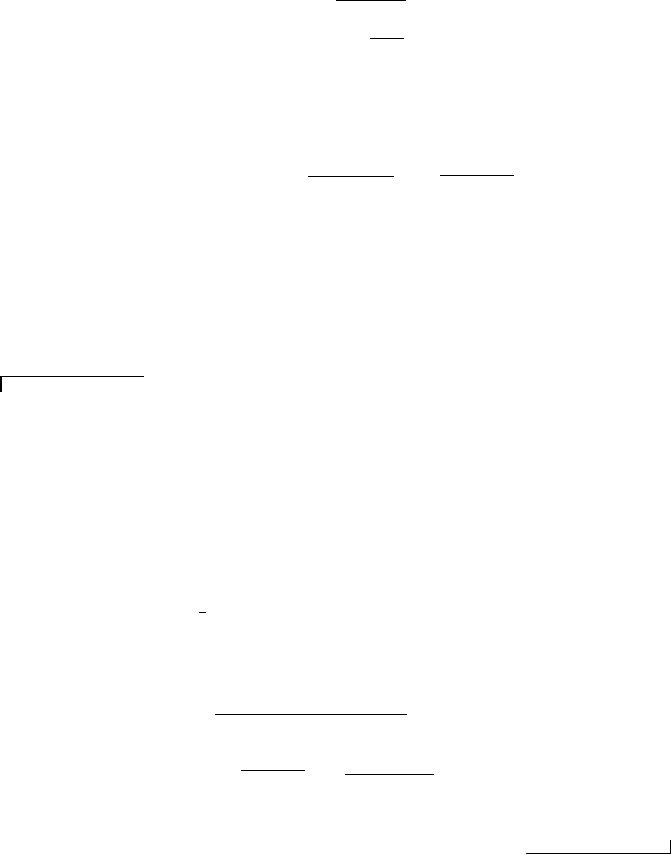
260 Measurement and Data Analysis for Engineering and Science
u
2
m
=
J
X
i=1
S
2
B
i
+
J
X
i=1
S
2
P
i
. (7.66)
When estimating the uncertainty in a mean value, S
P
i
is replaced in Equa-
tion 7.66 by S
¯
P
i
, where
S
¯
P
i
=
v
u
u
t
M
P
X
i=1
S
2
P
i
N
P
i
. (7.67)
For most engineering and scientific experiments, ν ≥ 9 [10]. When this
is the case, it is reasonable to assume for 95 % confidence that t
ν,95
∼
=
2
(when ν ≥ 9, t
ν,95
is within 10 % of 2). This implies that
U
x,95
∼
=
2u
c
= 2
q
S
2
B
i
+ S
2
P
i
=
q
B
2
i
+ P
2
i
, (7.68)
using Equations 7.17, 7.10, and 7.13 where S
2
B
i
is shorthand notation for
P
N
i=1
S
2
B
i
and S
2
P
i
for
P
N
i=1
S
2
P
i
. Equation 7.68 is known as the large-scale
approximation. Its utility is that the overall uncertainty can be estimated
directly from the systematic and random uncertainties. This is illustrated
in the following example.
Example Problem 7.13
Statement: A load cell is used to measure the central load applied to a structure.
The accuracy of the load cell as provided by the manufacturer is stated to be 2.3 N.
The experimenter, based upon previous experience in using that manufacturer’s load
cells, estimates the relative systematic uncertainty of the load cell to be 10 %. A series
of 11 measurements are made under fixed conditions, resulting in a standard deviation
of the random error equal to 11.3 N. Determine the overall uncertainty in the load cell
measurement at the 95 % confidence level.
Solution: The overall uncertainty can be determined using Equations 7.18 through
7.68. The standard deviations and the degrees of freedom for both the systematic
and random errors need to be determined first. Here ν
P
x
= N − 1 = 10 and, from
Equation 7.14, ν
B
x
=
1
2
(0.10)
−2
= 50. Thus, at 95 % confidence, t
10,95
= 2.228
and t
50,95
= 2.010 for the random and systematic errors, respectively. Now S
P
x
=
P
x
/t
10,95
= 11.3/2.228 = 5.072 from Equation 7.10, and, from Equation 7.13, S
B
x
=
B
x
/t
50,95
= 2.3/2.010 = 1.144. Substitution of these values into Equation 7.65 yields
ν
eff
=
(5.072
2
+ 1.144
2
)
2
(5.072
4
/10) + (1.144
4
/50)
= 11.02
∼
=
11.
Because ν
m
≥ 9, the large-scale approximation at 95 % confidence can be used, where
U
x,95
∼
=
q
B
2
i
+ P
2
i
=
p
2.3
2
+ 11.3
2
= 11.5 N.
In the following two sections, the application of the above equations to
multiple-measurement measurand and result experiments is presented.

Uncertainty Analysis 261
7.10.1 Multiple-Measurement Measurand Experiment
Consider the uncertainty estimation of a measurand involving multiple mea-
surements done to assess the contribution of temporal variability (temporal
precision error) of a measurand under fixed conditions. For this situation
the temporal variations of a measurand are treated as a random error and
all other errors are considered to be systematic. The following example il-
lustrates this process.
Example Problem 7.14
Statement: The supply pressure of a blow-down facility’s plenum is to be maintained
at set pressure for a series of tests. A compressor supplies air to the plenum through a
regulating valve that controls the set pressure. A dial gauge (resolution: 1 psi; accuracy:
0.5 psi) is used to monitor pressure in the vessel. Thirty trials of pressurizing the vessel
to a set pressure of 50 psi are attempted to estimate pressure controllability. The results
show that the standard deviation in the set pressure is 2 psi. Estimate the combined
standard uncertainty at 95 % confidence in the set pressure that would be expected
during normal operation.
Solution: The uncertainty in the set pressure reading results from the instrument
uncertainty, u
d
, and the additional uncertainty arising from the temporal variability in
the pressure under controlled conditions, u
1
. That is,
u
p
=
q
u
2
d
+ u
2
1
,
where u
1
= P
x
= t
ν,P
S
x
according to Equation 7.10. The instrument uncertainty is
u
d
=
q
u
2
o
+ u
2
I
,
where
u
o
=
1
2
(1.0 psi) = 0.5 psi
u
I
= 0.5 psi
⇒ u
d
=
p
0.5
2
+ 0.5
2
= 0.7 psi.
Also,
u
1
= t
29,95
S
x
= (2.047)(2) = 4.1 psi
where ν = N − 1 = 29 for this case. So,
u
p
=
p
0.7
2
+ 4.1
2
= 4 psi.
Note that the uncertainty primarily is the result of repeating the set pressure and not
the resolution or accuracy of the gauge.
Next, consider the multiple-measurement situation of estimating the un-
certainty in the range that contains the true value of a measurand. This is
shown in the following example in which the contributory systematic and
random errors are specified, having their elemental uncertainties already
summed using Equations 7.48 and 7.49.
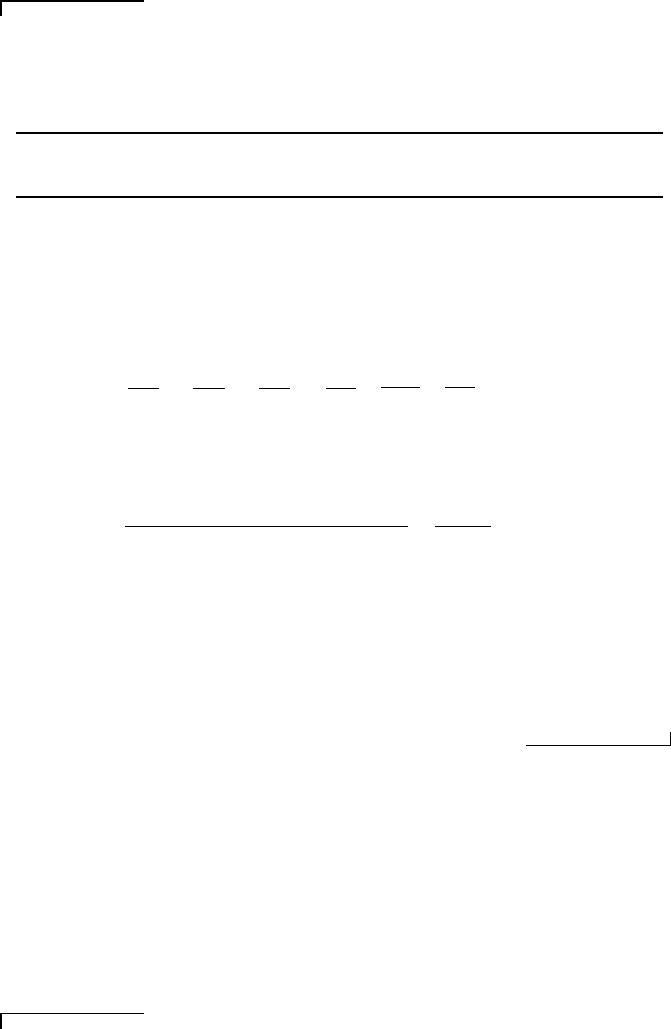
262 Measurement and Data Analysis for Engineering and Science
Example Problem 7.15
Statement: The stress on a loaded electric-powered remotely piloted vehicle (RPV)
wing is measured using a system consisting of a strain gage, Wheatstone bridge, ampli-
fier, and data acquisition system. The following systematic and random uncertainties
arising from calibration, data acquisition, and data reduction are as follows:
Calibration: S
B
1
= 1.0 N/cm
2
S
P
1
= 4.6 N/cm
2
N
P
1
= 15 ⇒ ν
P
1
= 14
Data Acquisition: S
B
2
= 2.1 N/cm
2
S
P
2
= 10.3 N/cm
2
N
P
2
= 38 ⇒ ν
P
2
= 37
Data Reduction: S
B
3
= 0.0 N/cm
2
S
P
3
= 1.2 N/cm
2
N
P
3
= 9 ⇒ ν
P
3
= 8
Assume 100 % in the values of all systematic errors and that there are no correlated
uncertainties. Determine for 95 % confidence the range that contains the true mean
value of the stress, σ
0
, given that the average value is ¯σ = 223.4 N/cm
2
.
Solution: The systematic variances are
S
2
B
= S
2
B
1
+ S
2
B
2
+ S
2
B
3
= 1
2
+ 2.1
2
+ 0
2
= 5.4 N
2
/cm
4
.
The random variances are
S
2
¯
P
=
S
P
1
N
P
1
2
+
S
P
2
N
P
2
2
+
S
P
3
N
P
3
2
=
4.6
2
15
+
10.3
2
38
+
1.2
2
9
= 4.4 N
2
/cm
4
.
It follows directly from Equation 7.66 that u
2
σ
= 5.4 + 4.4 = 9.8 N
2
/cm
4
. So, u
σ
=
3.1 N/cm
2
.
The number of effective degrees of freedom are determined using Equation 7.65,
which becomes
ν
σ
=
[1
2
+ 2.1
2
+ 0
2
+ 4.6
2
+ 10.3
2
+ 1.2
2
]
2
[(4.6
4
/14) + (10.3
4
/37) + (1.2
4
/8)]
=
17982.8
336.4
= 53.5 = 54,
noting that each ν
B
i
= ∞ because of its 100 % reliability. This yields t
54,95
∼
=
2.
Thus, the true mean value of the stress is
σ
0
= ¯σ ± U
σ
with U
σ
= t
54,95
× u
σ
= 6.2 N/cm
2
(95 %),
where the range is ±6.2 N/cm
2
. Thus,
σ
0
= 223.4 ± 6.2 N/cm
2
(95 %).
7.10.2 Multiple-Measurement Result Experiment
The uncertainty estimation of a result based upon multiple measurements of
measurands can be made using Equations 7.47 through 7.54. This estimation
is slightly more complicated than for the multiple-measurement measurand
because it involves determinations of the absolute sensitivity coefficients.
The following example illustrates the process in which the true mean value
of a result is estimated.
Example Problem 7.16
Statement: This problem is adapted from [18]. An experiment is performed in which
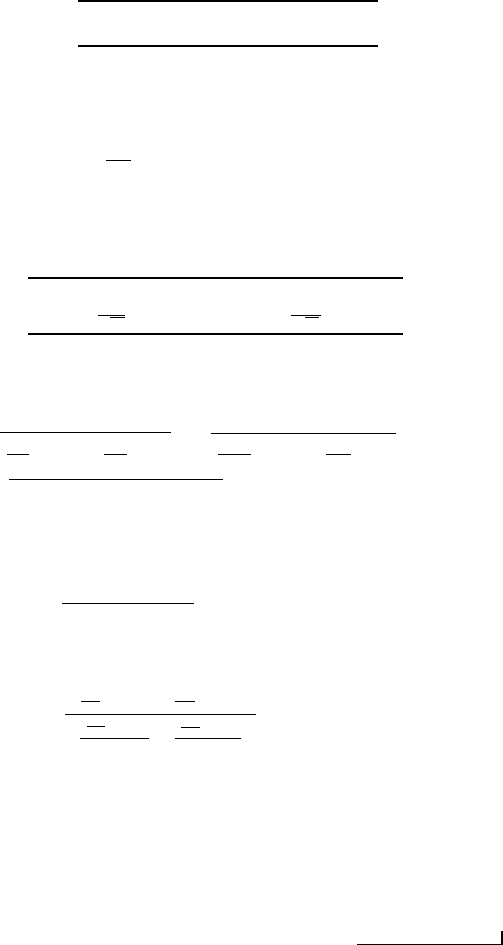
Uncertainty Analysis 263
the density of a gas, assumed to be ideal, is determined from different numbers of
separate pressure and temperature measurements. The gas is contained within a vessel
of fixed volume. Pressure is measured within 1 % accuracy; temperature is measured
to within 0.6
◦
R. Twenty measurements of pressure (N
p
= 20) and ten measurements
of temperature (N
T
= 10) were performed, yielding
¯p = 2253.91 psfa S
P
p
= 167.21 psfa
¯
T = 560.4
◦
R S
P
T
= 3.0
◦
R
Here, psfa denotes pound-force per square foot absolute. Estimate the true mean value
of the density at 95 % confidence.
Solution: Assuming ideal gas behavior, the sample mean density, ¯ρ, becomes
¯ρ =
¯p
R
¯
T
= 0.074 lbm/ft
3
.
There two sources of error in this experiment. One is from the variability in the pressure
and temperature readings as signified by the values of S
P
p
and S
P
T
given above. These
are random errors. The other is from the specified instrument inaccuracies. These are
systematic errors. These errors are
S
B
p
= 1 % = 22.5 psfa S
B
T
= 0.6
◦
R
S
P
¯p
=
S
P
p
√
20
= 37.4 psfa S
P
¯
T
=
S
P
T
√
10
= 0.9
◦
R
Here, the degrees of freedom are ν
P
= 19 and ν
T
= 9. Because the true mean value
estimate is made from multiple measurements, the random uncertainties are based upon
the standard deviations of their means. Thus, the combined random error becomes
S
¯
P
=
r
[
∂ρ
∂T
S
P
¯
T
]
2
+ [
∂ρ
∂P
S
P
¯p
]
2
=
r
[
−¯p
R
¯
T
2
S
P
¯
T
]
2
+ [
1
R
¯
T
S
P
¯p
]
2
=
q
[1.2 × 10
−4
]
2
+ [1.2 × 10
−3
]
2
= 0.0012 lbm/ft
3
.
Likewise, the combined systematic error becomes 0.0007 lbm/ft
3
. Using Equation 7.47,
assuming no correlated uncertainties, yields
u
ρ
=
p
0.0012
2
+ 0.0007
2
= 0.0014 lbm/ft
3
.
Further, assuming 100 % certainty in the stated accuracies of the instruments, the
effective number of degrees of freedom as determined from Equation 7.52 is
ν =
{[
∂ρ
∂T
S
P
¯
T
]
2
+ [
∂ρ
∂P
S
P
¯p
]
2
}
2
[
∂ρ
∂T
S
P
¯
T
]
4
ν
T
+
[
∂ρ
∂P
S
P
¯p
]
4
ν
P
= 23.
This yields t
23,95
= 2.06.
Thus, the true mean value of the pressure is
ρ
0
= ¯ρ ± U
ρ
with U
ρ
= t
23,95
· u
ρ
= 0.0029 lbm/ft
3
(95 %),
where the range is ±0.0029 lbm/ft
3
. Thus,
ρ
0
= 0.074 ± 0.003 lbm/ft
3
(95 %),
which is an uncertainty of ±3.4 %.

264 Measurement and Data Analysis for Engineering and Science
7.11 Uncertainty Analysis Summary
Most uncertainty estimation situations involve a single-measurement mea-
surand, a single-measurement result, a multiple-measurement measurand,
or a multiple-measurement result. Expressions for the uncertainty in either
a single- or multiple-measurement result contain absolute sensitivity coeffi-
cients. These coefficients are evaluated at typical measurand values. Those
expressions for the uncertainty in either a single- or multiple-measurement
measurand differ only in that the values of all absolute sensitivity coeffi-
cients become unity. When multiple measurements are considered, random
uncertainties are expressed in terms of the standard deviations of the means
of their random errors (Equation 7.11). This is the main difference between
the single- and multiple-measurement cases.
The objective of any uncertainty analysis is to obtain an estimate of
the overall uncertainty, U
x
. The summary expression containing U
x
involves
either x
next
or x
true
(Equations 7.15 and 7.16). The overall uncertainty is
expressed as the product of Student’s t variable based upon the number of
effective degrees of freedom (evaluated with %C confidence), t
ν
eff
,C
, and
the combined standard uncertainty, u
c
(Equation 7.18). The values of ν
eff
and u
c
depend upon the particular uncertainty estimation situation. When
the effective number of degrees of freedom is greater than or equal to 9, the
overall uncertainty can be estimated using the large-scale approximation
(Equation 7.68) for 95 % confidence where U
x
= 2u
c
. This greatly simplifies
the steps required to estimate U
x
.
For single-measurement situations, generalized uncertainty analysis is
the most appropriate (Section 7.9). No differentiation is made between sys-
tematic and random uncertainties. Expressions are available for the com-
bined standard uncertainty of either a measurand or a result with (Equa-
tions 7.23 and 7.19) and without (Equations 7.24 and 7.21) correlated un-
certainties. These expressions involve standard uncertainties for the mea-
surands that originate primarily from instrument uncertainties determined
from previous calibrations and assessments. There are associated expres-
sions for the effective number of degrees of freedom (Equations 7.25 and
7.22 for a measurand and for a result, respectively).
The uncertainties for multiple-measurement situations are assessed best
using detailed uncertainty analysis (Section 7.10). Errors are categorized
as either systematic, S
B
i
, or random, S
P
i
. Expressions are developed for
the combined standard uncertainty of either a measurand or a result with
(Equations 7.64 and 7.47) and without (Equations 7.66 and 7.58) correlated
uncertainties. There are associated expressions for the effective number of
degrees of freedom (Equations 7.65 and 7.52 for a measurand and a result,
respectively).

Uncertainty Analysis 265
In summary, the overall uncertainty of either a measurand or a result
can be estimated using the following steps:
1. Determine which experimental situation applies. Is the uncertainty esti-
mate for a measurand or a result, and is it based upon single or multiple
measurements?
2. Identify all measurands and, if applicable, all results.
3. Identify all factors affecting the measurands and, if applicable, the re-
sults. What instruments are used? What information is available about
their calibrations? Are there any circumstances that lead to correlated
uncertainties, such as the same instrument used for two different mea-
surands?
4. Define all functional relationships between the measurands and, if appli-
cable, the results. What are the nominal values of each measurand and
result? Be sure to use the same system of units throughout all calcula-
tions.
5. If the uncertainty in a result is estimated, determine the values of the ab-
solute sensitivity coefficients from the functional relationships and nom-
inal values.
6. Identify all uncertainties. What are the instrument errors, systematic
errors, and random errors? Are there temporal or spatial variations in
the measurands that contribute to uncertainty?
7. Calculate and propagate all uncertainties for the measurands and, if ap-
plicable, the results. Proceed from estimates of the elemental uncertain-
ties and calculate the systematic and random uncertainties.
8. Propagate all uncertainties to obtain the combined standard uncertainty.
9. Determine the number of effective degrees of freedom.
10. Determine the value of the coverage factor based upon the assumed con-
fidence and the number of effective degrees of freedom. Use a value of
two for the coverage factor if 95 % confidence is assumed and ν
eff
≥ 9.
11. Determine the overall uncertainty.
12. Present your findings in the proper form. x ± U
x
(%C).
Example Problem 7.17
Statement: Very accurate weight standards were used to calibrate a force-
measurement system. Nine calibration measurements were made, including repetition
