Stacey F.D., Davis P.M. Physics of the Earth

Подождите немного. Документ загружается.

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

257

– [239–266] 13.3.2008 11:41AM

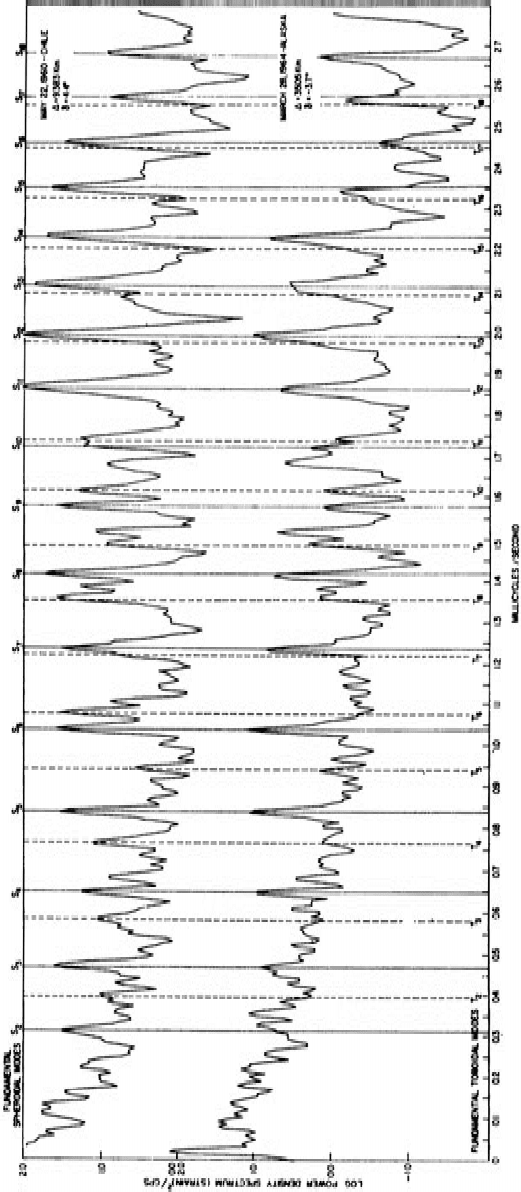
FIG U R E 16.10 Spectra of Earth strain recorded at Isabella, California, for the Chilean 1960 and Alaskan 1964 earthquakes.  is the angle between the

strain seismometer axis and the great circle path to the epicentre. Reproduced, by permission, from Smith (1967).

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

258

– [239–266] 13.3.2008 11:41AM

signalled a change in scientific direction. At least

in the mantle, lateral variations in structure now

attract more attention than further refinements

of the spherically averaged model, although

equation of state studies (Chapter 18) have

reached the point that an improved model is

needed.

As mentioned above, there are two funda-

mentally different kinds of oscillation, spheroi -

dal (S) and toroidal (T). In both cases the patterns

of surface deformation take the form of sphe-

rical harmonic functions (Appendix C), which

appear in solutions of the seismic wave equa-

tion in spherical geometry (Eq. (C.3)). The sim-

plest to envisage are the radial modes, a special

case of spheroidal oscillations in which the

motion is purely radial. They are alternating

dilations and compressions of the whole Earth

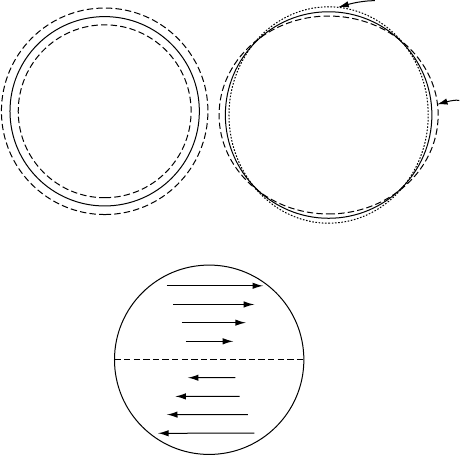
(Fig. 16.11(a)), designated

;

... wit h

spherical nodal surfaces within the Earth, the

instantaneous motion being opposite in adja-

cent layers. The prefix gives the number of

these nodal surfaces and the following sub-

script is t he number of nodal line s on the sur-

face, that is the harmonic degree, l,ofthe

surface pattern.

The slowest mode is

(Fig. 16.11(b)), with a

54-minute period. This has been called the foot-

ball mode, because the ellipsoidal deformation

of the Earth alternates between prolate and

oblate. There is no

mode, because that

would imply oscillation of the whole Earth

mass, but

occurs, the internal motion being

opposite to that of the outer shell. An infinite

series of fundamental spheroidal modes

each

has an infinite series of overtones

. The

general case,

, involves a tesseral harmonic

deformation of the surface in the form

cos ðÞcos ml (see Appendix C), but the fre-

quencies are almost the same for different values

of m with the same l and n. They would be iden-

tical for a non-rotating, spherically layered

Earth, but this degeneracy is broken by rotation,

causing the line splitting mentioned earlier.

Ellipticity and lateral heterogeneity give smaller

contributions to the line splitting. The splitting

decreases with mode frequency and is important

only for the low degree modes. The superscript

m is generally omitted in referring to free oscil-

lation modes.

Of the toroidal modes,

(Fig. 16.11(c)) is the

simplest, being an alternating twist between two

Prolate

Oblate

(a) (b)

(c)

FI G U R E 16.11 The simplest

representative modes of free oscillation.

(a) Radial oscillation

. (b) Spheroidal

‘football’ mode

. (c) Instantaneous

angular motion in toroidal mode

258 SEISMIC WAVE PROPAGATION

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

259

– [239–266] 13.3.2008 11:41AM

hemispheres. Higher modes involve the subdivi-

sion of the surface motion into 3, 4, ..., l zones

and, as with spheroidal oscillations, there are

overtones with internal nodal surfaces, whose

number is given by the prefix n. As in the case

of spheroidal oscillations, modes represented

by tesseral harmonics have almost the same

frequencies as zonal harmonics of the same

degrees.

The spheroidal modes are standing Rayleigh

waves and the toroidal modes are standing Love

waves in spherical geometry. A particular

advantage in analysing surface waves by free

mode theory is that the sphericity of the Earth

is properly accounted for. Clearly this is more

important for the longer wavelengths and is

essential at the longest wave periods in Fig. 16.9.

The surface patterns of free oscillation

motions are spherical harmonics, as mentioned

above. However, the coordinate axes are not the

geographic axes but are controlled by the loca-

tions and fault orientations of the exciting earth-

quakes. Thus, any particular observatory lies

close to some nodal lines for one earthquake,

but to a different set after another earthquake.

When this geometrical variation is allowed for,

estimation of the relative excitation amplitudes

of the various modes gives information about the

earthquake mechanisms. For this purpose the

amplitudes should be extrapolated back to a

time immediately after an earthquake as there

is a westward drift of the nodal pattern that can

confuse amplitude observations for the lower

modes. This drift must be recognized also in

using amplitude decay as a measure of damping.

The nodal drift is related to the mode splitting

and arises from the slightly different phase

speeds of eastward and westward propagating

waves in the rotating Earth.

Early Earth models, developed by K. E. Bullen,

used the seismic wave velocities, V

and V

, con-

straining the density structure to match the total

mass and moment of inertia. Since the 1960s,

Earth models have increasingly relied on the

frequencies of the modes of free oscillation.

The torsional modes involve only horizontal

motion, but the spheroidal modes involve

changes in shape, with radial motion and there-

fore gravitational as well as elastic restoring

forces. This means that they provide an independ-

ent fix on the density structure. We can under-

stand this in terms of a simplified model that is

amenable to elementary calculus, a homoge-

neous sphere subject to self-gravitation, with den-

sity and elasticity uniform throughout, ignoring

the effect of the steady internal compression.

Consider the spheroidal mode

, an oscilla-

tion with alternating prolate and oblate ellipsoi-

dal deformations. Using cylindrical coordinates,

with the origin at the centre and axes z along the

symmetry axis, r in the perpendicular plane and

 the angle to the z axis, the surface deformation

has the form of the zonal harmonic P

(cos )

(Appendix C) and is given by

 ¼ aP

sin !t ¼ a ð 3=2 cos

  1=2Þsin !t; (16:52)

for which the maximum axial elongation is a,

with corresponding maximum radial contraction

 a/2. We calculate the frequency of the oscilla-

tion, !, by using the fact that the total energy is

conserved, being entirely kinetic energy, E

,at

the instants of zero deformation and entirely

potential energy of elastic strain, E

, and dis-

placement of mass in the gravity field, E

,at

the instants of maximum deformation. Thus

¼ E

þ E

: (16:53)

has a simple form. The energy of elevation

by distance  of any surface element dA, relative

to the undeformed radius, R, is (1/2)g

because mass dA is elevated by an average dis-

tance /2 against gravity g. Areas for which  is

negative are included by noting that the missing

mass below R can be considered raised to R and

then transferred to the areas of positive . Thus,

with  given by Eq. (16.52), at sin !t ¼1,

g



dA ¼

ga

cos

 



2pR

sin  d

ga

(16:54)

With substitution for g by g ¼GM/R

¼(4/3)pGR,



: (16:55)

16.6 FREE OSCILLATIONS 259

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

260

– [239–266] 13.3.2008 11:41AM

At maximum deformation the strain can be

represented as two perpendicular shear strains,

each with the form of Fig. 16.11(b). Each compo-

nent of strain gives an extension by a/2 in the axial

direction and a/2 in the equatorial plane with

the equatorial strains at right angles to one

another, so that there is a uniform contraction

a/2 in all directions in the equatorial plane and

total extension a of the axis. Thus, we consider two

perpendicular shear strains, each of magnitude

" ¼ a=R,thatareuniformthroughoutthevolume

V of the sphere, and the strain energy is

¼ 2

"



V ¼

pRa

: (16:56)

In calculating the kinetic energy, E

,we

resolve the motion into axial (z) and radial (r)

directions, in which the amplitudes of the motion

are az/R ¼a cos  and (1/2)ar/R ¼(1/2)a sin  respec-

tively. There is no radial motion on the axis and

no axial motion in the equatorial plane. For

sinusoidal motion at angular frequency !, the

maximum particle velocities in the axial and

radial directions are a! cos  and (1/2)a! sin ,

and since they are mutually perpendicular,

their contributions to the energy are simply

added. For an elementary volume dV the ener-

gies are dE

¼(1/2)(a! cos )

 dV and dE

¼(1/8)

(a! sin )

 dV. To integrate the axial component

we divide the sphere into elementary discs of

radius R sin  and thickness dz ¼R sin  d at

z ¼R cos , so that dV ¼pR

sin

 d and

ða! cos Þ

pR

sin

 d

!

(16:57)

To integrate dE

we divide the sphere into ele-

mentary cylinders of thickness dr ¼R cos  d and

length 2z ¼2Rcos ,sothatdV¼4pR

cos

 sin  d,

giving

p=2

ða! sin Þ

4pR

cos

 sin  d

!

; ð16 :58Þ

and therefore

¼ E

þ E

¼ðp=5ÞR

!

: (16:59)

Substituting for E

, E

and E

by Eqs. (16.55),

(16.56) and (16.59) in Eq. (16.53), we have

G þ



R

: (16:60)

Recognizing that the shear wave speed is

¼ð=Þ

1=2

and that the travel time for a

shear wave across a diameter is T ¼2R/V

,we

can write the period,  ¼2p/!, of the

mode

for a uniform sphere as

 ¼

G þ



1=2

: (16:61)

In the absence of the gravity term this would be

1.217T, that is the mode period would be com-

parable to the shear wave travel time across a

diameter. But the important thing to notice

about this equation is that, with inclusion of

the gravity term, density enters the equation

for  independently of the travel time or velocity

term. This is the principle that allows the broad-

scale density profile of the Earth to be obtained

from spheroidal mode periods. For a fluid body

( ¼0), the gravity term is the only one on the

right-hand side of Eqs. (16.60) and (16.61). The

Sun is such a body and observations of its free

modes (helio-seismology) provide information

about its internal structure, but in this case the

assumption of homogeneity is a very poor one.

We can use a similar analysis to calculate the

period of the

mode for a homogeneous

sphere. The torsional modes involve no radial

motion and so give no independent information

about density, but this means that they give

details of the / structure without the compli-

cation of G terms, such as appear in Eqs. (16.60)

and (16.61), and so help in isolating the gravita-

tional effect on the spheroidal modes.

is the

simplest torsional mode, being a twisting

motion between two hemispheres, with the

equatorial plane of the motion as a node. The

angular displacement increases linearly with

distance from this plane in the manner of the

first degree zonal harmonic P

(cos ) ¼cos  ¼

z /R. Dividing the sphere into discs of radii

r ¼R sin  and thickness dz ¼R sin  d,asinthe

260 SEISMIC WAVE PROPAGATION

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

261

– [239–266] 13.3.2008 11:41AM

calculation of E

above, each disc has a mass

dm ¼pR

 sin

 d and moment of inertia

dI ¼(1/2)r

dm ¼(p/2) R

 sin

 d. Taking the

maximum angle of twist at the pole of the

motion to be w, and therefore wz=R ¼ w cos  at

co-latitude , the maximum angular velocity of

an elementary disc about its axis is !w cos  and

its kinetic energy is ð1=2ÞdIð!w cos Þ

. Thus, the

total kinetic energy is

ð!w cos Þ

w

cos

 sin

 d

105

w

(16:62)

Now we calculate the strain energy at an instant

of maximum twist between the hemispheres. Each

disc, of thickness dz ¼R sin  d,istwistedbyan

angle dw ¼ wdz=R ¼ w sin  d.Wecanusethisin

the standard formula for the torsional constant of a

rod of radius r and length l, that is the torque

per unit of angular twist,  ¼ðp=2Þr

=l,with

l ¼dz ¼sin  d, and write the strain energy as

¼ð1=2ÞðdwÞ

¼ðp=4ÞR

w

sin

 d;

(16:63)

which integrates to

¼ð4p=15ÞR

w

: (16:64)

Now we can equate Eqs. (16.62) and (16.64) to

give

¼ 7=R

: (16:65)

Rewriting this as the oscillation period, ,in

terms of the travel time of a shear wave across

a diameter, T ¼ 2Rð=Þ

1=2

;

 ¼ðp=

7ÞT ¼ 1:19T: (16:66)

This is very close to the

period if the gravita-

tional term in Eq. (16.61) is neglected. With

inclusion of this term the

mode would be

faster, but for the Earth it is significantly slower,

at least partly because radial motion in the fluid

core imparts inertia to the system, but there is

little corresponding slowing of

16.7 The moment tensor and

synthetic seismograms

Calculation of the normal modes of the Earth is

similar to calculating those of a stretched string

(or rod), with the one-dimensional geometry

replaced by the three-dimensional geometry of

the Earth. In the case of the string, the eigenfunc-

tions are sinusoids in space that satisfy the boun-

dary condition of zero displacement at the ends,

between which there is an integral number of

half wavelengths, allowing a series of discrete

frequencies. For the Earth the corresponding

boundary conditions are zero tractions on the

surface. The eigenfunctions or normal modes

are spherical Bessel functions that describe the

radial variation, and spherical harmonics for vari-

ation over spherical surfaces. Each mode has a

distinct frequency determined by the boundary

condition. In the general case, the displacement

field can be expressed as a weighted sum of

normal modes with weights adjusted to satisfy

the initial conditions of excitation. For an ea rth-

quake the normal mode displacements at time

zero mu st match the dislocation d escribed by

the earthquake moment tensor, which, as dis-

cussed in Chapter 14, can be expressed in terms

of force couples. The displacement from a force

couple was obtained by differentiating the dis-

placement field from a point force wi th resp ect

tothesourcelocation(Eq.(14.9)).Thus,ifwe

solve for the normal modes generated by appli-

cation of a point force within the Earth, we can

synthesize the modes generated b y any arbi-

trary moment tensor by ta king appropriate

derivatives and superimposing the solutions.

The resulting displacement field gives synthetic

seismograms at any place and time on the globe

with due account for sphericity and variation

with depth.

We illustrate the excitation of normal modes

by a point force by examining the one-dimensional

case of longitudinal oscillations of an elastic rod,

fixed at x ¼0 and x ¼L, with displacement u in

the x-direction (Fig. 16.12). The normal modes

are given by

¼ sinð!

x=cÞexpði!

tÞ; (16:67)

16.7 THE MOMENT TENSOR AND SYNTHETIC SEISMOGRAMS 261

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

262

– [239–266] 13.3.2008 11:41AM

with eigenfrequencies

¼ n

; n ¼ 1; 2; 3; ...; (16:68)

where c ¼

ﬃﬃﬃﬃﬃﬃﬃﬃ

E=

is the velocity that satisfies the

one-dimensional wave equation, assuming that

the wavelength is much greater than the rod

thickness,

u=@x

¼ @

u=@t

; (16:69)

and E and  are Young’s modulus and density.

Then

u=@x

¼ @

u=@t

: (16:70)

The effect at time t 0 of a disturbance at time

t ¼0, expressed as a weighted sum of normal

modes, is

uðx; tÞ¼

ðx; tÞ¼

sinðk

xÞ

ð1  expði!

tÞÞ; ð16:71Þ

where k

¼ !

=c and the mode shapes are ortho-

gonal, so that

sin

npx



sin

mpx



dx ¼ 

¼ 1

if m ¼ n; or ¼ 0ifm 6¼ n;

(16:72)

where m and n are integers. Suppose that at time

t ¼ 0 and location x ¼ x

a force f

is applied in

the x-direction and is maintained indefinitely. It

can be represented by

f ¼ f

ðx  x

HðtÞ; (16:73)

where f is the force per unit volume, a is the

cross-sectional area of the rod, ðxÞ is a delta func-

tion in x,andHðtÞis a Heaviside step function. The

equation of motion (Eq. (16.69)) becomes

u=@x

þ f

ðx  x

HðtÞ¼@

u=@t

: (16:74)

Substitution of Eq. (16.71) for u and solving for

the coefficients a

in that equation by taking a

Fourier transform and using Eq. (16.72) to resolve

individual coefficients (Problem 16.3) gives

uðx; tÞ¼

sinðk

Þsinðk

xÞ

1  cos !

;

(16:75)

where M ¼ aL is the mass of the rod. Equa-

tion (16.75) can be interpreted as displacement

equals a sum of modes, evaluated at the point

of application of the force, multiplied by the eigen-

functions and the time variation (Fig. 16.13).

We showed in Chapter 14 that dislocations are

equivalent to force couples and doublets. In the

rod case a force doublet corresponds to clamping a

small section of the rod at x

in compression and

releasing it at t ¼0. The solution for a force dou-

blet is obtained by placing a second oppositely

directed force a distance dx from x

and summing

the solutions. For this case the solution is obtained

by adding to Eq. (16.75) the displacement caused

by a second, oppositely directed force at distance

dx from x

. In effect this means differentiating

Eq. (16.75) with respect to x

and multiplying by

dx, and is completely equivalent to the differen-

tiation of Eq. (14.8) to produce Eq. (14.9). Following

the procedure in Section 14.2, we replace the force

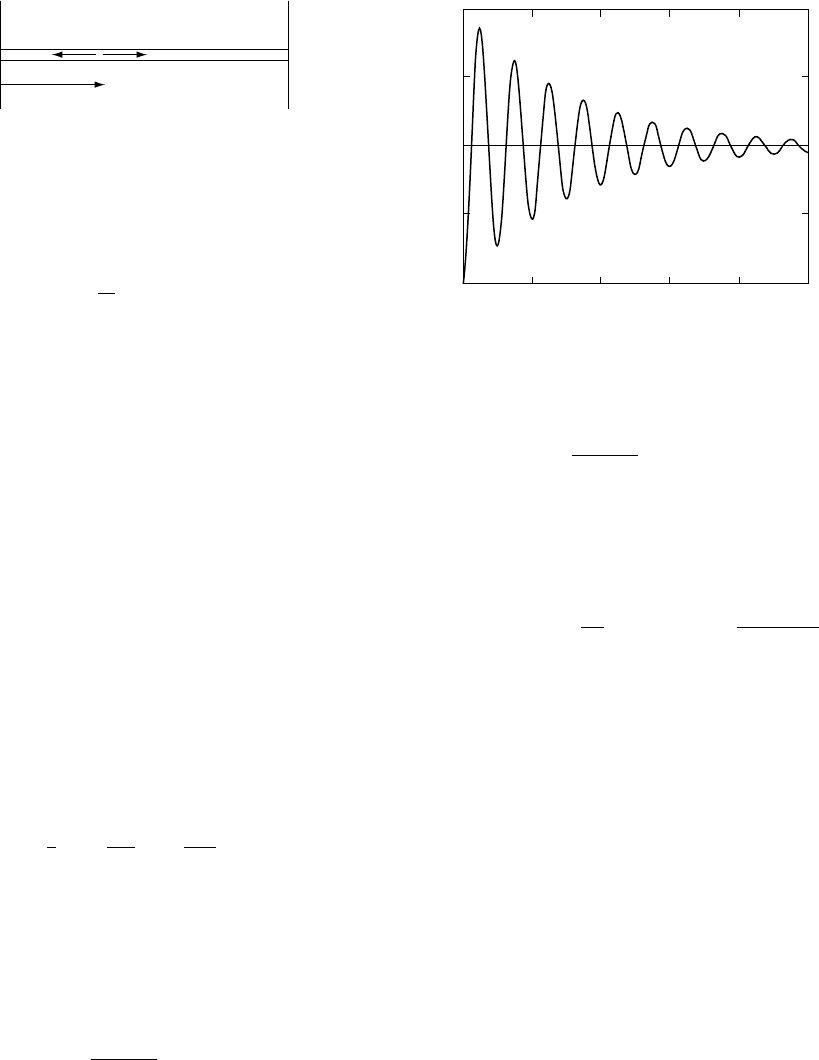
FI G U R E 16.12 Geometry of a force doublet excitation

of a clamped rod.

2.0

1.5

1.0

0.5

0.0

0 200 400 600 800 1000

Time

FI G U R E 16.13 Time variation of a normal mode excited

by a source that is a step function at t ¼0.

262 SEISMIC WAVE PROPAGATION

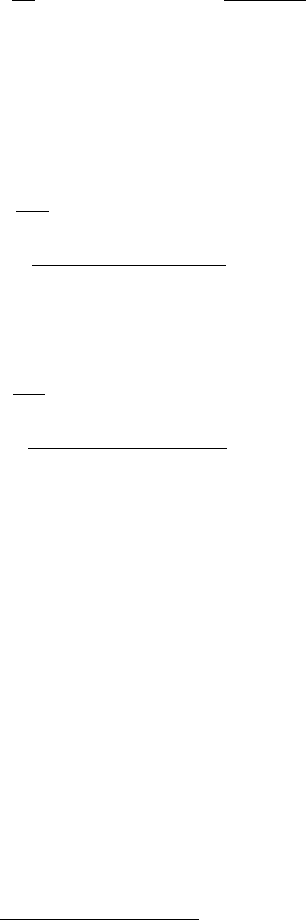
//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

263

– [239–266] 13.3.2008 11:41AM

moment f

dx with dislocation moment Eab ¼ M

where b is the dislo cation and a the area, to obtain

uðx; tÞ¼

cosðk

Þsinðk

xÞ

1  cos !

;

(16:76)

where, as in Eq. (14.9), we have the moment

term, M

, multiplied by the Green’s function,

here expressed as a sum of normal modes. If we

include attenuation,

uðx; tÞ¼

cosðk

Þsinðk

xÞ



1  exp½!

t=2Q

cos !

(16:77)

Note that k

cosðk

Þ is the strain @u/@x of the ith

mode or e

ðx

Þ: Then

uðx; tÞ¼

ðx

Þsinðk

xÞ



1  exp½!

t=2Q

cos !

(16:78)

Thus, the weights are equal to the spatial

derivatives of the eigenfunctions, that is, strains

evaluated at the source point x

, multiplied

by the corresponding elements of the moment

tensor and divided by mass. Equation (16.78)

describes a force doublet applied to a rod. To

use this approach to describe a dislocation in

the Earth, the one-dimensional eigenfunctions

are replaced with three-dimensional ones. So,

based on Eq. (16.78), we can write down the

solution directly. The displacement field uðx; tÞ

from a point source earthquake with arbitrary

moment tensor M

ðx

Þ (Aki and Richards, 2002),

represented as a sum of normal modes, is

uðx; tÞ¼

ðx

ÞM

ðtÞ

ðxÞ



1  expð!

t=2Q

Þcos !



;

(16:79)

where u

ðxÞ is the displacement attributed to the

ith normal mode and e

ðx

Þ is the corresponding

strain evaluated at the location of the source.

Once the normal modes are calculated,

Eq. (16.79) can be used to calculate the seismic

wave field for an arbitrary moment tensor, M

The term on the RHS of Eq. (16.79) represents a

damped sinusoidal oscillation with offset 1=!

(Fig. 16.13) and so includes the static displace-

ment (offset) of the dislocation. The normal

modes, u

ðxÞ, depend on the Earth model,

and are found by satisfying the equation of

motion and the boundary conditions of continu-

ity of stress and displacement at each layer boun-

dary including the surface. Calculation of the

modes has progressively improved with refine-

ments to take into account gravity, the rotation

of the Earth, its ellipticity, and lateral hetero-

geneity.

Equation (16.79) is used in inversion of seis-

mic data to determine M

. This is the basis of

the Harvard moment tensors that have been

calculated worldwide since about 1977 for

earthquakes above magnitude 5.6. By adding a

sufficient number of normal modes one obtains

all the transient waves that radiate from a

source, including all body waves and surface

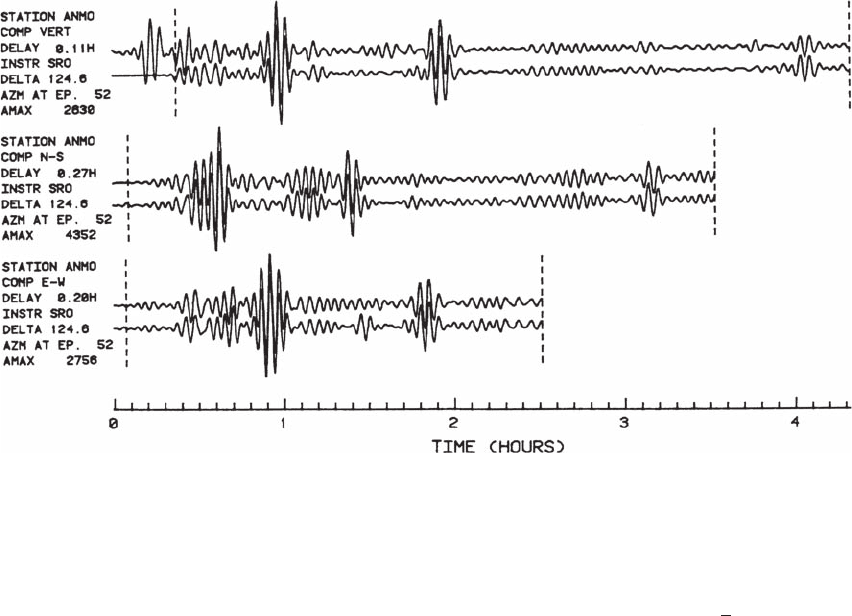
waves (Fig. 16.14). In that they can all be rep-

resented as normal modes, the distinction

between body and surface waves and free oscil-

lations is a matter of timing as to when the

observations are made. The transient waves

can only be observed early in the record, while

they remain isolated from other phases. As time

progresses multiple reflections and transmis-

sions around the globe and dispersion of wave

trains cause the energy to merge into a contin-

uous global vibration, which is better analysed

in the frequency domain for mode peaks and

attenuation. A fast method of calculating modes

(Woodhouse 1983, 1988) is capable of modell-

ing body wave phases at frequencies up to

0.16 Hz. However, in practice the moment ten-

sor obtained from normal modes is largely

made up of waves with periods much longer

than the earthquake duration, and so is repre-

sentative of the moment distribution averaged

in time and space, referred to as the centroid

moment tensor. In contrast, the moment deter-

mined from first-arriving P-body waves meas-

ures fault motion at the hypocentre, which,

unless the event expands symmetrically, will

be offset from the centroid.

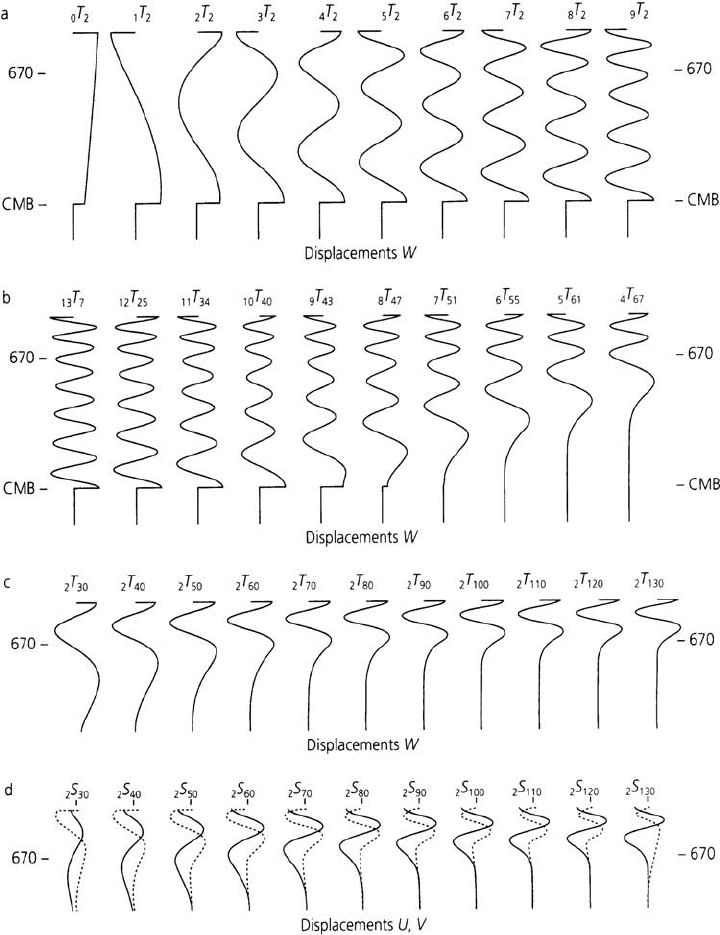
Normal mode eigenfunctions (Fig. 16.15) show

how the different frequencies give a weighted

16.7 THE MOMENT TENSOR AND SYNTHETIC SEISMOGRAMS 263

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

264

– [239–266] 13.3.2008 11:41AM

sampling of the Earth’s internal structure. From

Eq. (16.75) we see that the excitation of a mode

depends on its strain calculated at the location of

the earthquake. Earthquakes near the surface

excite fundamental modes which have their

eigenfunctions concentrated near the surface.

Deep earthquakes excite higher-order modes or

overtones with weaker excitation of fundamen-

tal modes.

Of particular interest are the modes that have

significant energy in the inner core, and have

played an important role in confirming the solid-

ity of the inner core. As early as 1946 Bullen used

the amplitudes of reflected P-waves from

the inner core surface, but the inference that it

represents a liquid–solid interface required

several assumptions. The reflection coefficient

depends on the impedance (V

) contrast

(Eq. (16.29)). Independent evidence from travel

times for P-waves suggested that the contrast

was caused by a significant increase in V

from

outer to inner core rather than a density change.

The jump in V

could be explained if the inner

core is solid, so that the P-wave modulus changes

from  ¼ K

outer

to  ¼ K

inner

 (Eq. (16.1)).

Alternatively, if the inner core remained liquid

the required change in K was unreasonably large,

and a decrease in density with depth is implau-

sible. However, the definitive test required

identification of inner core shear waves.

Because the outer core is fluid, the shear waves

have to be generated by conversions of P-waves

at the inner core boundary. Such conversions are

inefficient because incident angles are small.

Furthermore, as we mention below, S-waves

appear to be highly attenuated in the inner

core. After passage though the inner core they

convert back to P-waves, which must be distin-

guished from other phases that might arise from

heterogeneities in the mantle. Thus, early

reports of inner-core S-body wave phases (such

as PKJKP) have been questioned to the point that

skepticism was expressed as to whether they

would ever be observed in high frequency body

waves (Doornbos, 1974). Analysis of normal

modes can overcome these problems, because,

rather than relying on identification of indi-

vidual weak S phases, the mode energy is

FI G U R E 16.14 Synthetic seismograms from normal mode summations compared with observations. The top traces

are the observations and the bottom traces the synthetics for three earthquakes measured at the seismic station

ANMO in Albuquerque, New Mexico. From Woodhouse and Dziewonski (1984). R

, ...are Rayleigh waves from the

short and great circle paths from the source to the station. G

, ... are the corresponding Love waves.

264 SEISMIC WAVE PROPAGATION

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

265

– [239–266] 13.3.2008 11:41AM

effectively stacked by spectral analysis of

long wave-trains. Modes having eigenvectors

with significant particle motions in the

inner core (

and

) indicated an average

¼ 3:52  0:03 km=s (Masters and Gilbert,

1981). This apparently low value is expected for

solid iron at inner-core pressure, as explained in

Section 18.8. Now that anisotropy is recognized

(Section 17.7), we know that the inner core is

crystalline and aligned.

FI G U R E 16.15 Eigenfunctions of toroidal and spheroidal normal modes from the surface to the core–mantle

boundary (Dahlen and Tromp, 1998). (a), (b) and (c) show torsional modes. (d) shows spheroidal modes, with solid

lines representing radial displacements and dashed lines tangential displacements.

16.7 THE MOMENT TENSOR AND SYNTHETIC SEISMOGRAMS 265

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C16.3D

–

266

– [239–266] 13.3.2008 11:41AM

The usefulness of mode-generated synthetic

seismograms was illustrated in a resolution by

Deuss et al. (2000) of the debate over identifica-

tion of phases propagating as shear waves in the

inner core (in the notation illustrated by

Fig.17.7, PKJKP, SKJKP and also pPKJKP, which

has a surface reflection from a deep event).

These phases have very small amplitudes

because the necessary P to S and S to P conver-

sions of the inner core boundary are weak; addi-

tionally Doornbos (1974) pointed out that the

low Q for S-waves would make them completely

unobservable at high frequencies (1 Hz) and

reported observations have been disputed.

Using a method of phase stacking records from

the deep 1996 Flores Sea event, Deuss et al.

(2000) compared observed arrivals at the

expected times with synthetic seismograms for

the PREM model. They repeated the calculation

of synthetics for a modified PREM, with no inner

core shear wave but the same V

. By accepting

only arrivals seen with the solid inner core model

but not the fluid model, they were able to dis-

criminate against interfering minor signals from

the mantle. Superimposed pPKJKP and SKJKP

arrivals survived this test, but a similar analysis

for a deep 1994 Bolivia event found no unambig-

uous evidence for inner-core S-waves. Although

the Flores Sea event provided the only body wave

evidence for inner-core rigidity, it confirmed the

average V

 3:6km=s inferred from free oscilla-

tion modelling.

266 SEISMIC WAVE PROPAGATION