Baumgarte T., Shapiro S. Numerical Relativity. Solving Einstein’s Equations on the Computer

Подождите немного. Документ загружается.

12.1 Binary inspiral: overview 401

the previous minimum in the J = constant energy curves; for smaller values of J these

curves no longer have extrema. This behavior means that circular orbits can only exist for

sufﬁciently large values of J .

As a result of this correction, the equilibrium energy (12.27), which connects the extrema

in the J = constant energy curves and is represented by the dashed line in Figure 12.2,

now exhibits a turning point at

ISCO

= 6M, (12.28)

at which point equation (12.13) is satisﬁed. Beyond this turning point the extrema are

minima and thus represent stable circular orbits, while inside they are maxima and represent

unstable circular orbits. The turning point in the equilibrium energy therefore represents

an innermost stable circular orbit, or ISCO for short. Binary companions on quasicircular

inspiral trajectories start accelerating radially inward, plunging toward each other, after

they reach the ISCO. This dynamical transition from the inspiral phase to the plunge

and merger phase will induce a corresponding transition in the gravitational wave signal,

yielding an observable diagnostic of the transition.

For a point particle orbiting a Schwarzschild black hole, from which we constructed

the correction term (12.26), the location of the ISCO at r

ISCO

= 6M refers to the areal

radius of the orbit. It is more useful to express the critical binary separation in terms of

an invariant, like the orbital angular frequency 

orb

measured by a distant observer. For a

point particle orbiting a Schwarzschild black hole we have M

ISCO

= 1/6

3/2

≈ 0.0680.

In Section 12.4 we will ﬁnd similar values for binary black holes.

Exercise 12.4 Consider the orbit of a test-particle m

test

around a Schwarzschild

black hole M. The exact relativistic analog of equation (12.8) in this case is



dτ



+ V (r), (12.29)

where

V (r ) ≡



1 −



1 +



(12.30)

is the “effective potential”,

E = E/m

test

J = J/m

test

, r is the areal radius, and τ is

proper time.

(a) Circular orbits occur when dr/dτ = 0and∂ V /∂r |

= 0 = ∂

E/∂r |

,whichis

the relativistic counterpart of equation (12.9). Show that such orbits satisfy

(r − 2M)

r(r − 3M)

, (12.31)

r − 3M

(12.32)

as quoted in Chapter 1.2.

See, e.g., Shapiro and Teukolsky (1983), Chapter 12.3.

402 Chapter 12 Binary black hole initial data

(b) The transition from orbital stability to instability occurs when ∂

V/∂r

= 0,

which gives r

ISCO

= 6M. Show that this transition occurs when

∂

/∂r = 0 = ∂

/∂r, (12.33)

which is the counterpart of equation (12.13). Thus the point of marginal stability

corresponds to a simultaneous turning-point in the equilibrium energy and angular

momentum curves. The existence of simultaneous turning-points in the two curves

is a general result that follows from equation (12.14) in Newtonian gravitation and

equation (12.112) in general relativity.

that 

orb

is again given by equation (12.12) in these coordinates.

We emphasize, however, that for binaries with similar masses, q ≈ 1, the transition from

inspiral to plunge is not sharp. This can be seen from equation (12.24), which shows that

orbit

/P approaches unity close to the ISCO if q ≈ 1. For such a binary, the radial inspiral

is already appreciable over an orbital period as it reaches the ISCO, so that the transition

from inspiral to plunge and merger is gradual rather than sharp. Stated differently, the

deviations from circular orbit are already large as binaries with companions of comparable

mass approach our so-called ISCO. In Chapter 13 we will see that dynamical simulations

of binary black hole merger indeed verify this expectation. The notion of the ISCO is

nevertheless a useful concept for the comparison and characterization of initial data sets.

It is useful to note that any attractive interaction potential that falls off more steeply than

1/r

results in an ISCO.

Exercise 12.5 (a) Show that any attractive interaction potential energy of the form

−λa

n+1

,whereλ>0 is a dimensionless constant and a > 0 carries dimensions

of length, leads to a positive contribution to the equilibrium energy E

provided

that n > 2.

(b) Find the minimum of E

to locate the ISCO at

n−1

ISCO

= λn(n − 2)

n+1

Mµ

, (12.34)

which is again positive if n > 2.

The ISCO is therefore not only a relativistic phenomenon, but can also arise in Newtonian

gravitation. For example, two ﬂuid stars treated not as point masses but as hydrodynamic

equilibria of ﬁnite size, experience a tidal interaction.

For irrotational, identical stars, for

example, we can model these tidal effects by including a tidal interaction term

tidal

=−λ

µMR

. (12.35)

Here R is the stellar radius, and the dimensionless parameter λ depends on the equation of

state. For an incompressible ﬂuid λ = 3/2, and from equation (12.34) we ﬁnd the ISCO is

See Lai et al. (1993b, 1994b) and references therein for a semi-analytic analysis and models.

12.2 The conformal transverse-traceless approach 403

located at

ISCO

= (24λ)

1/5

R ≈ 2.05R, (12.36)

implying that such a binary would reach the ISCO just before the two stars touch. We

have plotted this result in Figure 12.2 for identical stars of compaction m/R = 0.2, where

m = M/2 is the mass of the individual stars; we also show the combined effects of

relativistic corrections and tidal interactions in determining the ISCO. For compressible

stars λ is smaller than 3/2, so that in Newtonian gravitation these stars would merge before

they could encounter any ISCO. However, in general relativity, for identical irrotational

companions constructed with a moderately stiff equation of state, the entire equilibrium

sequence actually terminates at a ﬁnite separation prior to merger, at which point the stellar

density proﬁles form a cusp (see Chapter 15.3). Orbital decay beyond this point will then

trigger some tidal disruption prior to eventual merger (see Chapter 16).

After reaching the ISCO, the binary companions plunge and merge on an orbital time

scale. Depending on the nature and masses of the compact companions, their coalescence

leads to the formation of a merged object, or remnant, that may either be a rotating neutron

star or a black hole. During the ﬁnal ringdown phase of the binary evolution this remnant

settles down into an equilibrium state. In the case of a hypermassive neutron star, secular

effects (viscosity or magnetic ﬁelds) can lead later to delayed collapse to a black hole (see

Chapters 14 and 16.)

During the inspiral phase, up to reasonably small binary separations, the binary can

be modeled very accurately by post-Newtonian expansions (see Appendix D). The ring-

down phase can be described very well by strong-ﬁeld perturbative techniques. Numerical

relativity simulations are needed to connect these two regimes, beginning with the late

inspiral phase, and continuing through the dynamical plunge and merger phase. Numerical

relativity is also required to treat delayed collapse. Not surprisingly, considerable effort in

numerical relativity has focused on compact binaries in close quasicircular orbits. In the

rest of this chapter we will construct initial data for binary black holes in such orbits.

12.2 The conformal transverse-traceless approach: Bowen–York

12.2.1 Solving the momentum constraint

In the transverse-traceless approach we assume both conformal ﬂatness, so that ¯γ

= η

and maximal slicing, so that K = 0. The momentum constraint (12.4) then reduces to

= 0, (12.37)

where

is now a ﬂat-space covariant derivative. In Cartesian coordinates this operator

reduces to the partial derivative ∂

. Our assumptions have simpliﬁed the momentum

constraint to the point where the decomposition of

into a transverse and a longitudinal

part (see equation 3.48) is somewhat pointless, since both of them now have vanishing

404 Chapter 12 Binary black hole initial data

divergence. It is nevertheless useful to keep this decomposition in mind, since it makes

the counting of freely speciﬁable variables more transparent. In particular we will choose

= 0, which amounts to making two arbitrary choices for its two independent variables.

We still have to ﬁnd solutions

, but as we have seen in Chapter 3.2 we can construct

these analytically given our assumptions of conformal ﬂatness and maximal slicing. This,

in fact, is the essence of this so-called Bowen–York approach: we can solve the momentum

constraint analytically, leaving only the Hamiltonian constraint to be solved numerically.

In Chapter 3.2 we found two such analytical black hole solutions to (12.37); one that

carries a linear momentum P



+ P

− (η

− n



(12.38)

(see equation 3.80), and one that carries an angular momentum (spin) S



j)kl

(12.39)

(see equation 3.66). Here we are assuming Cartesian coordinates, r =



+ y

+ z

the coordinate distance to the center of the black hole (which is located at the origin), and

= x

/r is the normal vector pointing away from the black hole’s center. In exercise 3.32

we evaluated the surface integral (3.195)

8π

(

∞

(12.40)

to show that the linear momentum associated with equation (12.38) is indeed P

,andin

exercise 3.29 we computed



ijk

8π

(

∞

(12.41)

to verify that the angular momentum associated with equation (12.39)isS

To allow for a black hole located at a point C

we introduce a subscript (or occasionally

superscript) C. We then have



+ P

− (η

− n



, (12.42)

and



j)kl

, (12.43)

where r

=||x

− C

|| is the coordinate distance to the center of the black hole located at

= C

,andn

= (x

− C

)/r

is the normal vector.

12.2 The conformal transverse-traceless approach 405

Given that the momentum constraint (12.37) is linear, we can construct a binary black

hole solution by superposition of single solutions

. (12.44)

This completes an analytic solution of the momentum constraint describing two black

holes with arbitrary momenta and spins.

Exercise 12.6 Show that

P = P

+ P

(12.45)

is the total linear momentum of the solution (12.44)and

J = C

× P

+ C

× P

+ S

(12.46)

its total angular momentum about the origin of the coordinate system.

For a binary system, S

and S

can be associated with the spin of the individual black

holes only in the limit of inﬁnite binary separation, but we nevertheless take the liberty to

deﬁne the orbital angular momentum L as

L ≡ J − S

− S

. (12.47)

12.2.2 Solving the Hamiltonian constraint

With a solution to the momentum constraint at hand we can now proceed to solve the

Hamiltonian constraint (12.3). Under the assumptions of conformal ﬂatness and maximal

slicing, this equation reduces to

ψ =−

−7

. (12.48)

We have reduced the construction of binary black hole initial data to solving a single

nonlinear elliptic equation. On the right hand side, the term

can be computed

analytically from (12.44). Unfortunately this term diverges at the black holes’ centers C

Dealing with this singularity requires some extra care.

Two different approaches have been adopted in the literature to solve this problem; they

differ in the topology of the resulting solution. Recall our discussion in Chapter 3.1,which

demonstrated that initial data sets representing multiple black holes are not unique. As a

starting point, we can look for generalizations of the time-symmetric solution

ψ = 1 +

, (12.49)

To represent the momenta P

and spins S

of these solutions it is convenient to use bold-face notation P and S instead

of index notation.

406 Chapter 12 Binary black hole initial data

which solves equation (12.48)for

= 0 (i.e., Laplace’s equation). This solution repre-

sents the three-sheeted topology sketched in Figure 3.2. In this topology each black hole

generates an Einstein–Rosen bridge to its own asymptotically ﬂat universe, meaning that

the solution is not symmetric across each throat. This symmetry can be recovered, how-

ever, by introducing “spherical inversion images”. This approach leads to a two-sheeted

topology, schematically represented in Figure 3.3, in which the two black holes connect

two identical asymptotically ﬂat universes.

In the conformal imaging approach

a two-sheeted topology is assumed. Establishing

the isometry between the two sheets requires adding image terms to the extrinsic curvature

(12.44).

Once this has been done, the two sheets, representing two asymptotically ﬂat

universes connected by two Einstein–Rosen bridges (see Figure 3.3), are exact mirror

images of each other. We therefore need to solve the Hamiltonian constraint (12.48) only

on one of these two sheets; we could always ﬁnd the conformal factor on the other sheet

from the symmetry. This means that we eliminate the black hole interior containing the

black hole singularities from the computational domain and solve (12.48) only in the black

hole exterior. This is an example of black hole excision, which we will encounter many

more times.

Clearly we need a boundary condition for the conformal factor ψ on the black hole

throats, the interior of which we excise. These boundary conditions follow immediately

from the isometry that is imposed on the throats.

While the isometry of the resulting two-sheeted solutions is aesthetically appealing, the

conformal-imaging approach requires complicated image terms for the extrinsic curvature

as well as the imposition of boundary conditions on internal boundaries. The puncture

approach,

which leads to a three-sheeted topology, avoids both of these difﬁculties.

The key idea of the puncture approach is to absorb the singularities arising in the solution

to equation (12.48) in analytical terms. As we have said before, the expression (12.49)

satisﬁes the Hamiltonian constraint (12.48)for

= 0. We may therefore write the general

solution as equation (12.49), the homogeneous solution, plus a new term correcting for

ﬁnite

. As it turns out, this new term satisﬁes an equation that is regular everywhere.

We begin by writing

ψ = 1 + 1/α + u, (12.50)

where α is deﬁned by

≡

. (12.51)

Here we may refer to the

as the puncture masses. It is important to realize, though, that

they can be equated to the black hole’s ADM mass or irreducible mass only in the limit of

Cook (1991); Cook et al. (1993); Cook (1994).

Kulkarni et al. (1983).

Beig and

O Murchadha (1994, 1996); Brandt and Br

ugmann (1997).

12.2 The conformal transverse-traceless approach 407

inﬁnite separation. For any ﬁnite separation these parameters have no immediate physical

meaning.

The Hamiltonian constraint now reduces to an equation for the correction term u,

u =−β

(

α(1 + u) + 1

)

−7

, (12.52)

where we have used the abbreviation

β ≡

. (12.53)

Notice that α goes to zero at each one of the “punctures” C

. It also enters β with a

sufﬁciently high power to suppress the divergence in

. As a consequence the source

term in equation (12.52) is regular everywhere. We have thus eliminated the need for both

the image terms in

and for black hole excision, and can, instead, solve for u with any

standard method for nonlinear elliptic equations (see, e.g., our discussion in Chapter 6.2.2

following equation 6.54). To impose asymptotic ﬂatness, we impose a Robin boundary

condition

∂(ru)

∂r

= 0 (12.54)

at large distances from the black holes.

Once we have found the conformal factor ψ numerically, we can compute the ADM

mass M

ADM

from equation (3.145),

ADM

=−

2π

(

∞

ψd

. (12.55)

Within the puncture approach we can insert the ansatz (12.50)toﬁnd

ADM

=−

2π

(

∞





−

2π



+ M

2π



(

α(1 + u) + 1

)

−7

x. (12.56)

Here the volume integral in the last term extends over all space.

12.2.3 Identifying circular orbits

Up to now we have discussed how, for particular choices of black hole positions C

momenta P

, spins S

and puncture masses M

, we can construct a solution to the constraint

equations. We now ask how we can pick these parameters in such a way that the resulting

black holes represent a binary in circular orbit.

In a zero-momentum frame we have P = 0 and therefore

=−P

. (12.57)

408 Chapter 12 Binary black hole initial data

For a circular orbit these momenta must also be perpendicular to C ≡ C

− C

C · P

= 0. (12.58)

This requirement corresponds to the condition P

= 0inexercise12.1 and dr/dτ = 0in

exercise 12.4. We will focus on black holes that have S

= 0, meaning that they are not

spinning as seen in an inertial frame. We will later refer to such binaries as nonspinning.

With this choice the problem has been reduced to a 4-dimensional parameter space. The

four independent parameters may be taken to be the puncture masses

and M

,the

momentum P

=||P

|| and the coordinate separation C =||C||. For spinning black holes

we also have to pick the black hole spins S

Given these input parameters, we can construct a binary conﬁguration by solving the

constraint equations as outlined above in Sections 12.2.1 and 12.2.2. For each conﬁguration

we can then compute the signiﬁcant physical quantities, including the total ADM mass

ADM

(equations 12.55 or 12.56) and the angular momentum J (equation 12.46). We then

deﬁne the binding energy E

≡ M

ADM

− m

. (12.59)

Here the black hole masses m

and m

are assigned to be their irreducible masses,

(

/16π

)

1/2

, (12.60)

where

is the proper area of the event horizon of the i th black hole (see equation 7.2).

Sometimes the quantity E

is referred to as the effective potential (recall exercise 12.4).

Exercise 12.7 Evaluate E

for two black holes in circular orbit at inﬁnite separation

and determine the sign of E

when the circular orbit is at ﬁnite separation.

As we discussed in Chapter 7.1, locating the black hole’s event horizon requires knowl-

edge of the entire future. We only construct data on one time slice , so clearly we cannot

compute the irreducible mass m

irr

exactly. For most of the binary inspiral, however, the

event horizons are very well approximated by the black hole’s apparent horizons (see

Chapter 7.3), and it is reasonable to compute m

irr

from these.

In the conformal imag-

ing approach these apparent horizons coincide with the throats on which the isometry is

imposed. The horizons are therefore at a ﬁxed coordinate location, and their proper area

can be computed quite easily. In the puncture method, however, we do not know the location

Pfeiffer et al. (2000).

Some authors deﬁne the binding energy as the difference between the ADM mass and the black holes’ Kerr masses (7.3)

(e.g., Cook 1994; Pfeiffer et al. 2000). For the nonspinning black holes that we focus on here this distinction clearly

does not make any difference. In general, however, the deﬁnition (12.59) seems more natural. Since the irreducible

mass M

irr

is constant along quasistationary evolutionary sequences, the deﬁnition (12.59) leads to simultaneous turning

points in the equilibrium binding energy and the ADM mass.

But see Pfeiffer et al. (2000) for a discussion of rapidly spinning black holes in close binaries.

12.2 The conformal transverse-traceless approach 409

of the apparent horizons a priori, and instead we have to ﬁnd them by implementing one

of the methods discussed in Chapter 7.3 before their area can be computed.

Lastly we compute the proper separation between the two horizons l. In practice it is

much easier to compute the proper separation along C, which is a very good approximation

to the shortest proper, or geodesic, separation (within ) for many conﬁgurations.

The condition (12.58) insures that each black hole’s momentum is perpendicular to the

separation axis, at least momentarily. Imposing this condition is not sufﬁcient for a circular

orbit, however, since it also holds at the apocenter and pericenter of a noncircular orbit. To

guarantee a circular orbit we must impose the additional condition that the binding energy

(i.e., the effective potential) E

be at a stationary point along a sequence of constant angular

momentum J for ﬁxed black hole masses m

and m

∂ E

∂l



J,m

= 0. (12.61)

We already employed this condition in exercises 12.1 and 12.4 to identify circular orbits.

Once we identify the circular orbits, we can construct an equilibrium sequence of binaries

of ﬁxed black hole masses m

and m

at different separations. As in equation (12.14)

(or equation 12.112) we can then ﬁnd the binary’s orbital angular velocity 

orb

at each

separation from



orb

∂ E

∂ J



. (12.62)

To identify the ISCO, we can apply the turning-point criterion to the function E

(l), as

in equations (12.13) and (12.33). Alternatively, we can apply the turning-point criterion

to the equilibrium angular momentum J

(l), as noted in exercise 12.4. As pointed out in

the same exercise, applying the turning-point criterion is equivalent to ﬁnding a point of

inﬂection in the effective potential, ∂

/∂l

= 0.

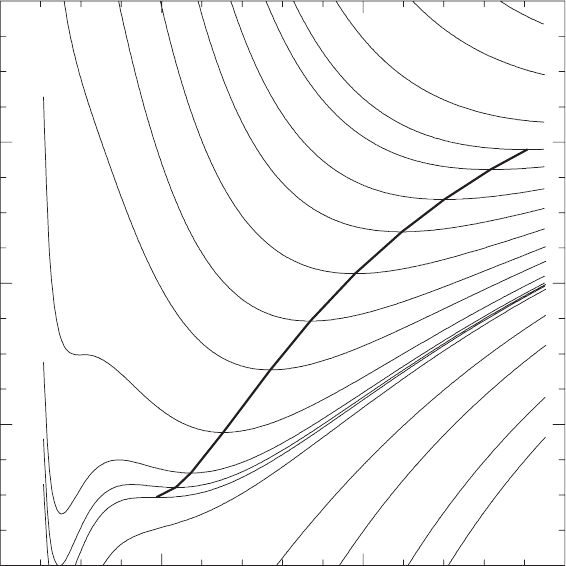
Figure 12.3 illustrates the numerical construction of a quasiequilibrium binary consisting

of equal-mass, nonspinning black holes in circular orbit using conformal imaging and

the effective potential approach.

Convenient ﬁtting formulae have been derived

for

the same system, using the puncture method. These handy formulae specify the puncture

masses

M,momentaP, and the quantities M

ADM

, J

ADM

and 

orb

as functions of the

puncture coordinate separation C, all normalized by the total ADM mass of the binary at

inﬁnite separation (which equals the total irreducible mass.)

We will review the resulting orbits from these numerical calculations in greater detail in

Section 12.4. But ﬁrst we will discuss an alternative approach to constructing binary black

holes that probably provides a better approximation to binaries in quasiequilibrium.

Baumgarte (2000).

Cook (1994).

Tichy and Br

ugmann (2004). Quasiequilibrium is achieved by imposing the relativistic virial relation M

= M

ADM

;

see Chapter 3.5 and exercise 12.9. A lapse is required for this condition and is obtained by imposing ∂ K /∂t = 0

(equation 4.12).

410 Chapter 12 Binary black hole initial data

−0.06

−0.08

−0.1

/ M

/ m

−0.04

−0.02

10 15

Figure 12.3 The binding energy E

/µ as a function of separation l for various values of the angular momentum

J/µM (thin lines) as obtained by the Bowen–York conformal-imaging method for an equal mass, nonspinning

black hole binary. The thick line connects a sequence of stable quasicircular orbits, which correspond to minima

of the binding energy (equation 12.61), and yields E

as a function of l (“effective potential approach”). This

sequence terminates at the ISCO, identiﬁed where an E

curve at constant J exhibits a point of inﬂection. [From

Cook (1994).]

12.3 The conformal thin-sandwich approach

12.3.1 The notion of quasiequilibium

The appeal of the Bowen–York approach is that we can solve a large part of the problem

(i.e., the momentum constraints) analytically, which furnishes useful insight into how black

hole binary initial data can be constructed. However, in Section 12.4 we will ﬁnd some

evidence suggesting that the resulting binary solutions may not describe astrophysically

realistic, circular-orbit, binary black holes as closely as we might like. The core of the

problem is that the Bowen–York formalism does not provide any direct means of imposing

quasiequilibrium conditions on the metric. We invoke global energy minimum arguments