Bhushan B. Handbook of Micro/Nano Tribology, Second Edition

Подождите немного. Документ загружается.

the ﬁgure is curved with a slope of less than 1 shortly after the sample touches the tip, which suggests

that the tip has indented the sample. Later, the slope is equal to 1 suggesting that the tip no longer indents

the sample. This observation indicates that the tape surface is soft locally (polymer-rich) but it is hard

(as a result of magnetic particles) underneath. Since the curves in the extending and retracting modes

are identical, the indentation is elastic up to at a maximum load of about 22 nN used in the measurements.

A shown in Figure 14.48 for unlubricated and lubricated textured disks, the slope of the deﬂection

curves is 1 and remains constant as the disks touch and continue to push the AFM tip. The disks are not

indented.

14.6.2 Nanoscale Indentation

Nanoscale mechanical properties of magnetic media and single-crystal silicon have been measured by

Bhushan et al. (1994a–d, 1995a–e, 1997a, 1998) and Koinkar and Bhushan (1996a, 1997b). Bhushan et al.

(1994b) have reported that indentation hardness with a penetration depth as low as 1 nm can be measured

using AFM. Figures 14.49a and show the gray-scale plots and line plots of inverted images of indents

made on the as-received Si(111) at normal loads of 60, 65, 70, and 100 µN. Triangular indents can be

clearly observed with very shallow depths. It is found that below a normal load of 60 µN indents are

unobservable. At a normal load of 60 µN indents are observed and the depth of penetration is about

1 nm. As we increase the normal load, the indents become clearer and indentation depth increases.

Figure 14.50 shows a plot of hardness and normal load as a function of indentation depth. The indentation

depth increases with an increase in normal load. We note that the hardness at a small indentation depth

of 2.5 nm is 16.6 GPa and it drops to a value of 11.7 GPa at a depth of 7 nm and normal load of 100 µN.

The observed drop in the hardness values at higher loads (Figure 14.50) is comparable to the trends in

the nanohardness data measured using a commercial nanoindenter by Pharr et al. (1990) and Page et al.

(1992). Pharr et al. (1990) have reported a value of 11.4 GPa at large indentation depths (>50 nm) and

Page et al. (1992) have reported a value of about 14.2 GPa at an indentation depth of about 20 nm and

monotonic decrease in hardness to about 7.5 GPa at indentation depths of about 500 nm. Reported values

of microhardness values of silicon is about 9 to 10 GPa (Anonymous, 1988). If the silicon material is

used at very light loads such as in microsystems, the high hardness of surface ﬁlms would protect the

surface until it is worn.

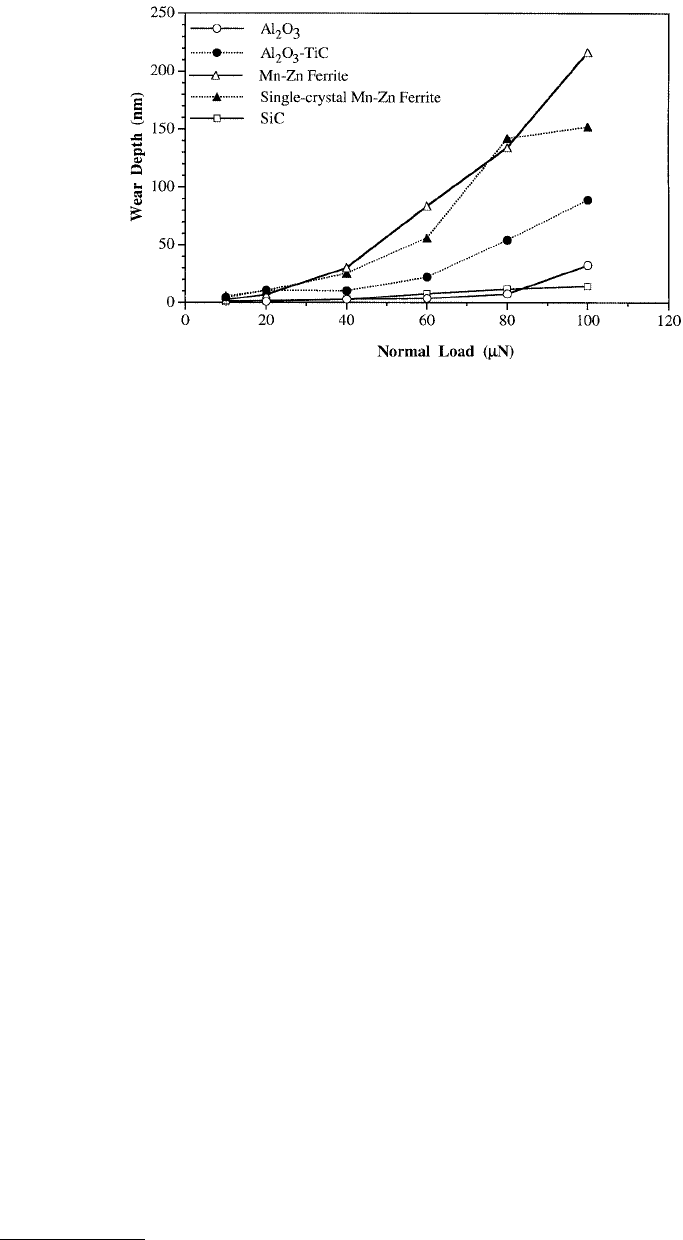
FIGURE 14.29 Wear depth as a function of normal load after one scan cycle for Al

, Al

–TiC, polycrystalline

Mn–Zn ferrite, single-crystal Mn–Zn ferrite, and SiC. (From Koinkar, V.N. and Bhushan, B., 1996, Wear 202, 110–122.

With permission.)

Higher hardness values obtained in low-load indentation may arise from the observed pressure-

induced phase transformation during the nanoindentation (Pharr, 1991; Callahan and Morris, 1992).

Additional increase in the hardness at an even lower indentation depth of 2.5 nm reported here may arise

from the contribution by complex chemical ﬁlms (not from native oxide ﬁlms) present on the silicon

surface. At small volumes, there is a high probability that indentation would be made into a region that

was initially dislocation free. Furthermore, at small volumes, it is believed that there is an increase in the

stress necessary to operate dislocation sources (Gane and Cox, 1970; Sargent, 1986). These are some of

the plausible explanations for the increase in hardness at smaller volumes.

Nanohardness values of virgin, coated, and treated silicon samples are presented in Table 14.5. Coatings

and treatments improved nanohardness of silicon. We note that dry-oxidized and PECVD oxide ﬁlms

are harder than wet-oxidized ﬁlms, as these ﬁlms may be porous (Bhushan and Venkatesan, 1993c). High

hardness of oxidized ﬁlms may be responsible for lower wear and scratch resistance (Table 14.5). Hardness

values of virgin and C

-implanted Si(111) at various indentation depths (normal loads) are presented

in Figure 14.51. We note that the surface layer of the implanted zone is much harder than that of the

subsurface, and may be brittle leading to higher wear on the surface. Subsurface of the implanted zone

is harder than the virgin silicon, resulting in high wear resistance (data presented earlier, Figure 14.46).

Bhushan et al. (1994c) measured nanohardness of polished thin-ﬁlm disks at loads of 80, 100, and

140 µN loads, Figure 14.52. Hardness values were 21 GPa (10 nm), 21 GPa (15 nm), and 9 GPa (40 nm);

the depths of indentation are shown in the parenthesis. The hardness value at 80 and 100 µN is much

higher than at 140 µN. This is expected since the indentation depth is only about 15 nm at 100 µN, which

is smaller than the thickness of DLC coating (~20 to 30 nm). The hardness value at lower loads is primarily

the value of the carbon coating. The hardness value at higher loads is primarily the value of the magnetic

ﬁlm, which is softer than the carbon coating. This result is consistent with the scratch and wear data

discussed previously.

For the case of hardness measurements made on thin-ﬁlm rigid disk at low loads, the indentation

depth is on the same order as the variation in the surface roughness. For accurate measurements of

indentation size and depth, it is desirable to subtract the original (unindented) proﬁle from the indented

proﬁle. Bhushan et al. (1994b,c, 1995d), and Lu and Bogy (1995) developed an algorithm for this purpose.

Because of hysteresis, a translational shift in the sample plane occurs during the scanning period, resulting

in a shift between images captured before and after indentation. Therefore, the image needs to be shifted

for perfect overlap before subtraction can be performed. For this purpose, a small region on the original

image was selected and the corresponding region in the indented image was found by maximizing the

correlation between the two regions. (Proﬁles were plane-ﬁtted before subtraction.) Once two regions

were identiﬁed, overlapped areas between the two images were determined and the original image was

shifted with the required translational shift and then subtracted from the indented image. An example

of proﬁles before and after subtraction is shown in Figure 14.53. The indent on the subtracted image can

be measured easily. At a normal load of 140 µN the hardness value of polished, unlubricated magnetic

thin-ﬁlm rigid disk (σ roughness = 3.3 nm) is 9.0 GPa and the indentation depth is 40 nm.

Figure 14.54a shows the hardness as a function of residual depth for three types of 100-nm-thick

amorphous carbon coatings deposited on silicon by sputtering, ion beam, and cathodic arc processes

(Kulkarni and Bhushan, 1997). Data on uncoated silicon are also included for comparisons. The cathodic

arc carbon coating exhibits highest hardness of about 24.9 GPa, whereas the sputtered and ion beam

carbon coatings exhibit hardness values of 17.2 and 15.2 GPa, respectively. The hardness of Si(100) is

13.2 GPa. High hardness of the cathodic arc carbon coating explains its high wear resistance, reported

earlier. Figure 14.54b shows the elastic modulus as a function of residual depth for various samples. The

cathodic arc coating exhibits the highest elastic modulus. Its elastic modulus decreases with an increasing

residual depth, while the elastic moduli for the other carbon coatings remain almost constant. In general,

hardness and elastic modulus of coatings are strongly inﬂuenced by their crystalline structure, stoichi-

ometry, and growth characteristics which depend on the deposition parameters. Mechanical properties

of carbon coatings have been known to change over a wide range with sp

–sp

bonding ratio and amount

of hydrogen. Hydrogen is believed to play an important role in the bonding conﬁguration of carbon

atoms by helping to stabilize tetrahedral coordination of carbon atoms.

14.6.3 Localized Surface Elasticity

AFM can be used in the force modulation mode to measure surface elasticities in a nondestructive manner

(Maivald et al., 1991; DeVecchio and Bhushan, 1997; Scherer et al., 1997). By using this technique, it is

possible to measure quantitatively the elasticity of soft and compliant materials with penetration depths

of less than 100 nm. This technique has been successfully used to get localized elasticity maps of particulate

magnetic tapes. An elasticity map of a tape can be used to identify relative distribution of hard mag-

netic/nonmagnetic ceramic particles and the polymeric binder on the tape surface, which has an effect

on friction and stiction at the head-tape interface.

Figure 14.55 shows surface height and elasticity maps on a sample of metal particle magnetic tape.

The elasticity image reveals sharp variations in surface elasticity due to the composite nature of the ﬁlm.

As can be clearly seen, regions of high elasticity do not always correspond to high or low topography.

Figure 14.56 shows measurements on three different formulations of magnetic tapes with a larger scan

size. It is seen that the number of stiff regions on the second two tapes is higher than that on the ﬁrst

tape. The trend in increasing number of stiff regions has been correlated to reduced stiction problems

in these three tapes (Bhushan et al., 1997c). Based on a Hertzian elastic-contact analysis, the static

indentation depth of these samples during the force modulation scan is estimated to be about 1 nm. We

conclude that the contrast seen is inﬂuenced most strongly by material properties in the top few nanom-

eters, independent of the composite structure beneath the surface layer.

14.7 Detection of Material Transfer

Ruan and Bhushan (1993) conducted indentation on C

-rich fullerene ﬁlms (Bhushan et al., 1993a,b)

using AFM in the force calibration mode. They observed transfer of fullerene molecules to the AFM tip

during indentation. The fullerene molecules transferred to the AFM tip were subsequently transported

to a diamond surface when the diamond sample was scanned with the contaminated tip, Figure 14.57.

The discontinuity in the tip deﬂection shown in Figure 14.57a is due to the transferred fullerene molecules

to the AFM tip. After repeated sliding of the tip against the diamond surface, the discontinuity in the tip

deﬂection disappeared as shown in Figure 14.57b. This demonstrates the capability of detection of

material transfer on a molecular scale using AFM.

14.8 Lubrication

The boundary ﬁlms are formed by physical adsorption, chemical adsorption, and chemical reaction. The

physisorbed ﬁlm can be either monomolecular or polymolecular thick. The chemisorbed ﬁlms are

monomolecular, but stoichiometric ﬁlms formed by chemical reaction can have a large ﬁlm thickness.

In general, the stability and durability of surface ﬁlms decrease in the following order: chemical reaction

ﬁlms, chemisorbed ﬁlms, and physisorbed ﬁlms. A good boundary lubricant should have a high degree

of interaction between its molecules and the sliding surface. As a general rule, liquids are good lubricants

when they are polar and thus able to grip solid surfaces (or be adsorbed). Polar lubricants contain reactive

functional groups with low ionization potential or groups having high polarizability (Bhushan, 1993).

Boundary lubrication properties of lubricants are also dependent upon the molecular conformation and

lubricant spreading (Novotny et al., 1989; Novotny, 1990; Mate and Novotny, 1991; Mate, 1992a).

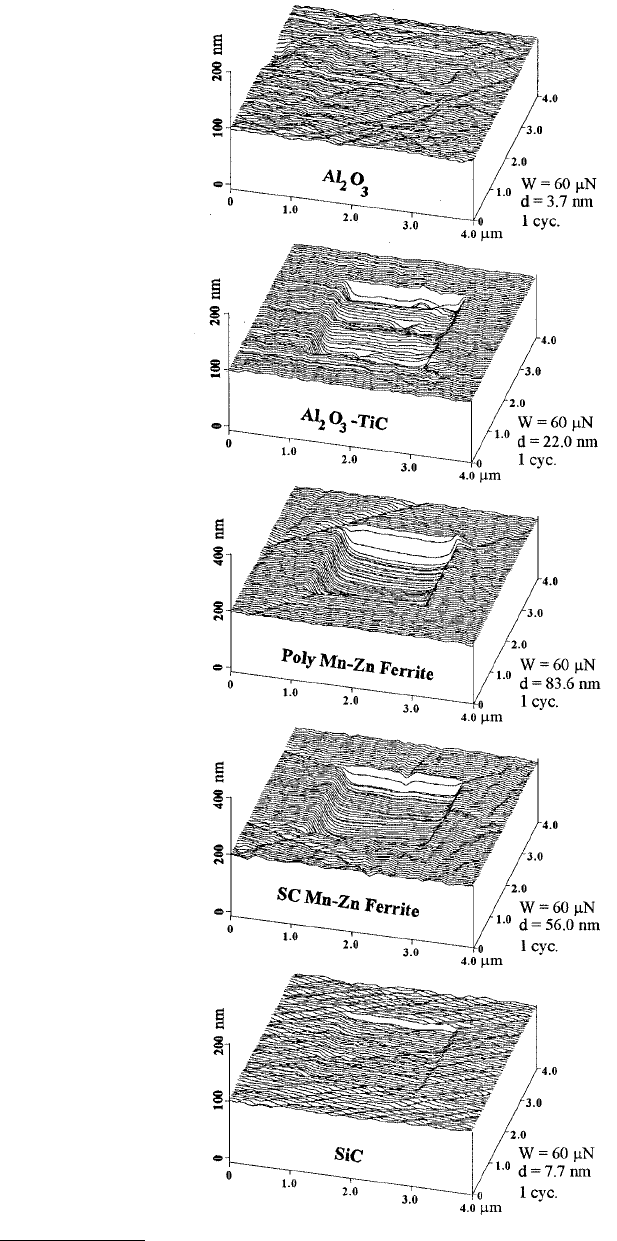
FIGURE 14.30 Surface proﬁles showing the worn region (center 2 × 2 µm) after one scan cycle at a normal load of

60 µN for Al

, Al

–TiC, polycrystalline Mn–Zn ferrite, single-crystal Mn–Zn ferrite, and SiC. (From Koinkar,

V.N. and Bhushan, B., 1996, Wear 202, 110–122. With permission.)

Mechanical interactions between the magnetic head and the medium in magnetic storage devices are

minimized by the lubrication of the magnetic medium. The primary function of the lubricant is to reduce

the wear of the medium and to ensure that friction remains low throughout the operation of the drive.

The main challenge, however, in selecting the best candidate for a speciﬁc surface is to ﬁnd a material

that provides an acceptable wear protection for the entire life of the product, which can be several years

in duration. There are many requirements that a lubricant must satisfy in order to guarantee an acceptable

life performance. An optimum lubricant thickness is one of these requirements. If the lubricant ﬁlm is

too thick, excessive stiction and mechanical failure of the head–disk is observed. On the other hand, if

the ﬁlm is too thin, protection of the interface is compromised, and high friction and excessive wear will

result in catastrophic failure. An acceptable lubricant must exhibit properties such as chemical inertness,

low volatility, high thermal, oxidative and hydrolytic stability, shear stability, and good afﬁnity to the

magnetic medium surface.

Fatty acid esters are excellent boundary lubricants, and esters such as tridecyl stearate, butyl stearate,

butyl palmitate, butyl myristate, stearic acid, and myrstic acid are commonly used as internal lubricants,

roughly 1 to 7% by weight of the magnetic coating in particulate ﬂexible media (tapes and particulate

ﬂexible disks). The fatty acids involved include those with acid groups with an even number of carbon

atoms between C

and C

, with alcohols ranging from C

to C

. These acids are all solids with melting

points above the normal surface operating temperature of the magnetic media. This suggests that the

decomposition products of the ester via lubrication chemistry during a head–ﬂexible medium contact

may be the key to lubrication.

Topical lubrication is used to reduce the wear of rigid disks and thin-ﬁlm tapes. Perﬂuoropolyethers

(PFPEs) are chemically the most stable lubricants with some boundary lubrication capability, and are

most commonly used for topical lubrication of rigid disks. PFPEs commonly used include Fomblin Z

and Fomblin Y lubricants, made by Ausimont, Milan, Italy; Krytox 143 AD, made by Dupont, U.S.; and

Demnum S, made by Diakin, Japan; and their difunctional derivatives containing various reactive end

groups e.g., hydroxyl or alcohol (Fomblin Z-Dol), piperonyl (Fomblin AM 2001), isocyanate (Fomblin

Z-Disoc), and ester (Demnum SP). The difunctional derivatives are referred to as reactive (polar) PFPE

lubricants. The chemical structures, molecular weights, and viscosities of various types of PFPE lubricants

are given in Table 14.6. We note that rheological properties of thin ﬁlms of lubricants are expected to be

different from their bulk properties. Fomblin Z and Demnum S are linear PFPE, and Fomblin Y and

Krytox 143 AD are branched PFPE, where the regularity of the chain is perturbed by –CF

side groups.

The bulk viscosity of Fomblin Y and Krytox 143 AD is almost an order of magnitude higher than the

Z-type. Fomblin Z is thermally decomposed more rapidly than Y (Bhushan, 1993). The molecular

diameter is about 0.8 nm for these lubricant molecules. The monolayer thickness of these molecules

depends on the molecular conformations of the polymer chain on the surface (Novotny et al., 1989; Mate

and Novotny, 1991).

The adsorption of the lubricant molecules on a magnetic disk surface is due to van der Waals forces,

which are too weak to offset the spin-off losses, or to arrest displacement of the lubricant by water or

other ambient contaminants. Considering that these lubricating ﬁlms are on the order of a monolayer

thick and are required to function satisfactorily for the duration of several years, the task of developing

a workable interface is quite formidable. An approach aiming at alleviating these shortcomings is to

enhance the attachment of the molecules to the overcoat, which, for most cases, is sputtered carbon.

There are basically two approaches which have been shown to be successful in bonding the monolayer

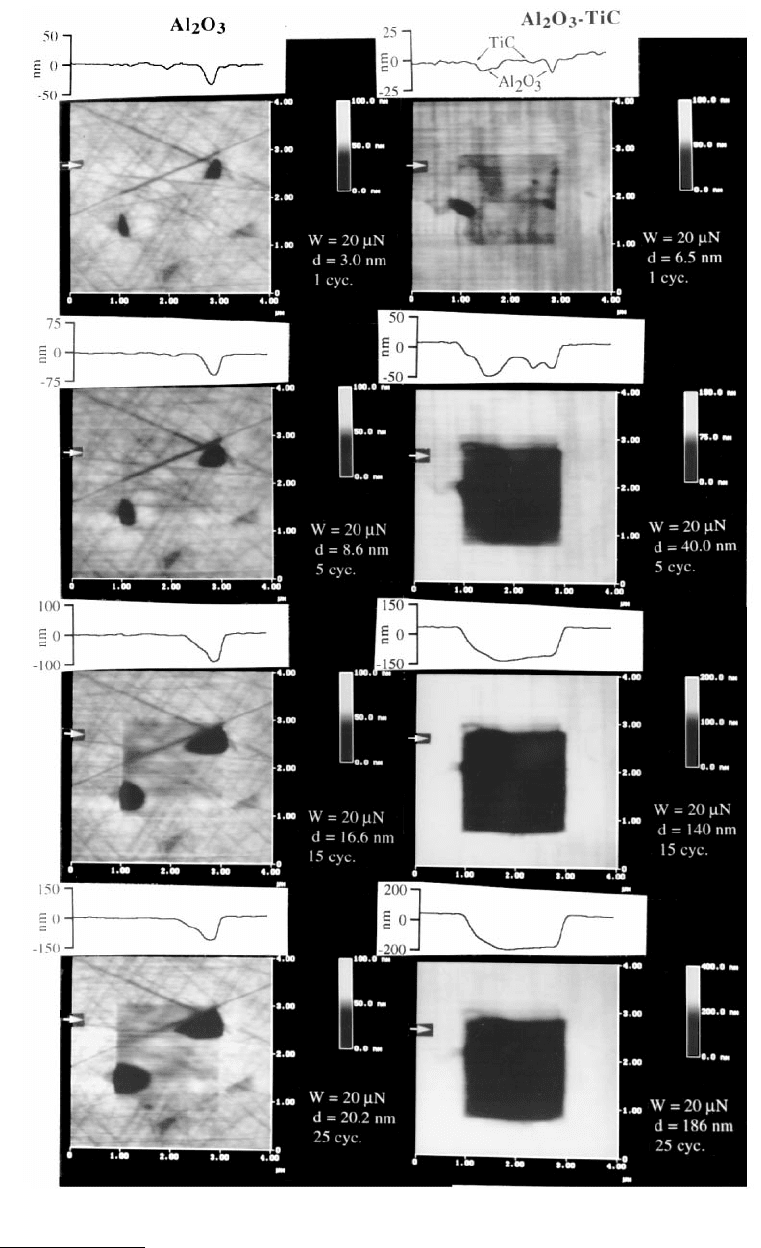
FIGURE 14.31 Gray-scale two-dimensional plots showing the worn region (center 2 × 2 µm) at a normal load of

20 µN and different number of scan cycles for Al

and Al

–TiC. The two-dimensional section plots taken at a

location shown by an arrow are shown on the top of corresponding gray-scale plot. Note the change in vertical scale

for gray-scale and two-dimensional section plots. (From Koinkar, V.N. and Bhushan, B., 1996, Wear 202, 110–122.

With permission.)

to the carbon. The ﬁrst relies on exposure of the disk lubricated with neutral PFPE to various forms of

radiation, such as low-energy X ray (Heidemann and Wirth, 1984), nitrogen plasma (Homola et al.,

1990), or far ultraviolet (e.g., 185 nm) (Saperstein and Lin, 1990). Another approach is to use chemically

active PFPE molecules, where the various functional (reactive) end groups offer the opportunity of strong

attachments to speciﬁc interface. These functional groups can react with surfaces and bond the lubricant

to the disk surface, which reduces its loss due to spin off and evaporation. Bonding of lubricant to the

disk surface depends upon the surface cleanliness. After lubrication, the disk is generally heated at 150°C

for 30 min to 1 h to improve the bonding. If only a bonded lubrication is desired, the unbonded fraction

can be removed by washing it off for 60 s with a nonfreon solvent (FC-72). Their main advantage is their

ability to enhance durability without the problem of stiction usually associated with weakly bonded

lubricants (Bhushan, 1996a).

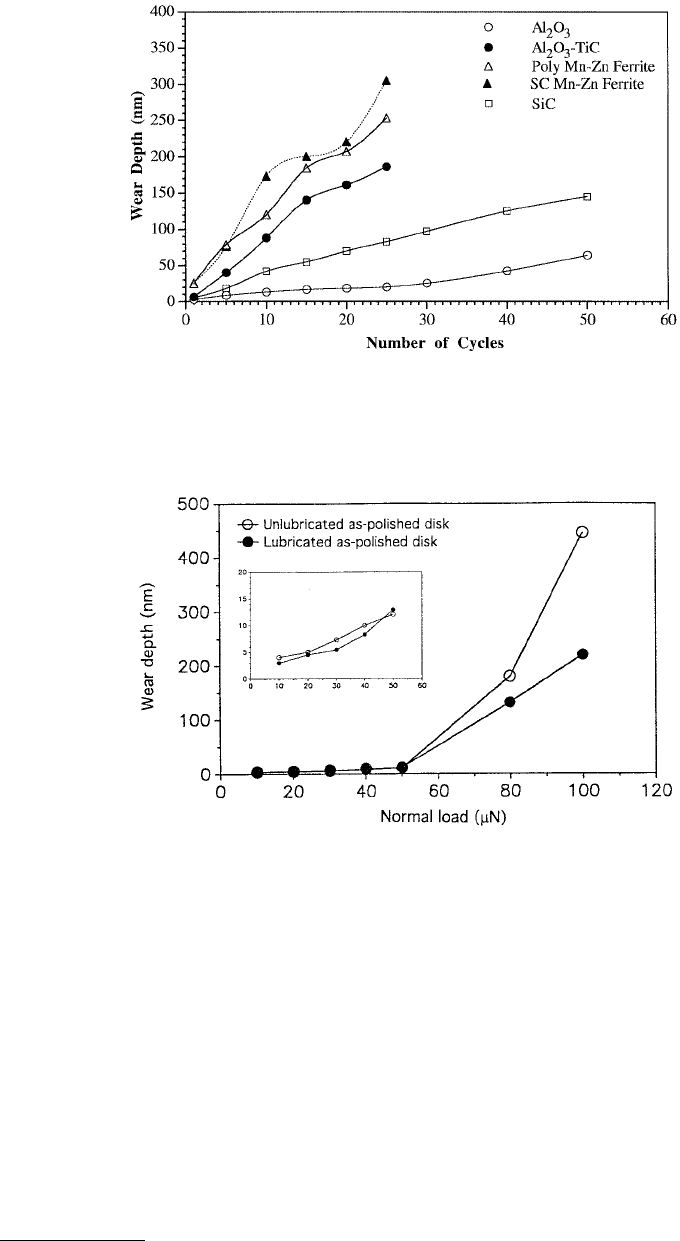
FIGURE 14.32 Wear depth as a function of number of cycles at a normal load of 20 µN for Al

, Al

–TiC,

polycrystalline Mn–Zn ferrite, single-crystal (SC) Mn–Zn ferrite, and SiC. (From Koinkar, V.N. and Bhushan, B.,

1996, Wear 202, 110–122. With permission.)

FIGURE 14.33 Wear depth as a function of normal load for polished, lubricated and unlubricated thin-ﬁlm rigid

disks after one cycle. (From Bhushan, B. et al., 1994, Proc. Inst. Mech. Eng. Part J: J. Eng. Tribol. 208, 17–29. With

permission.)

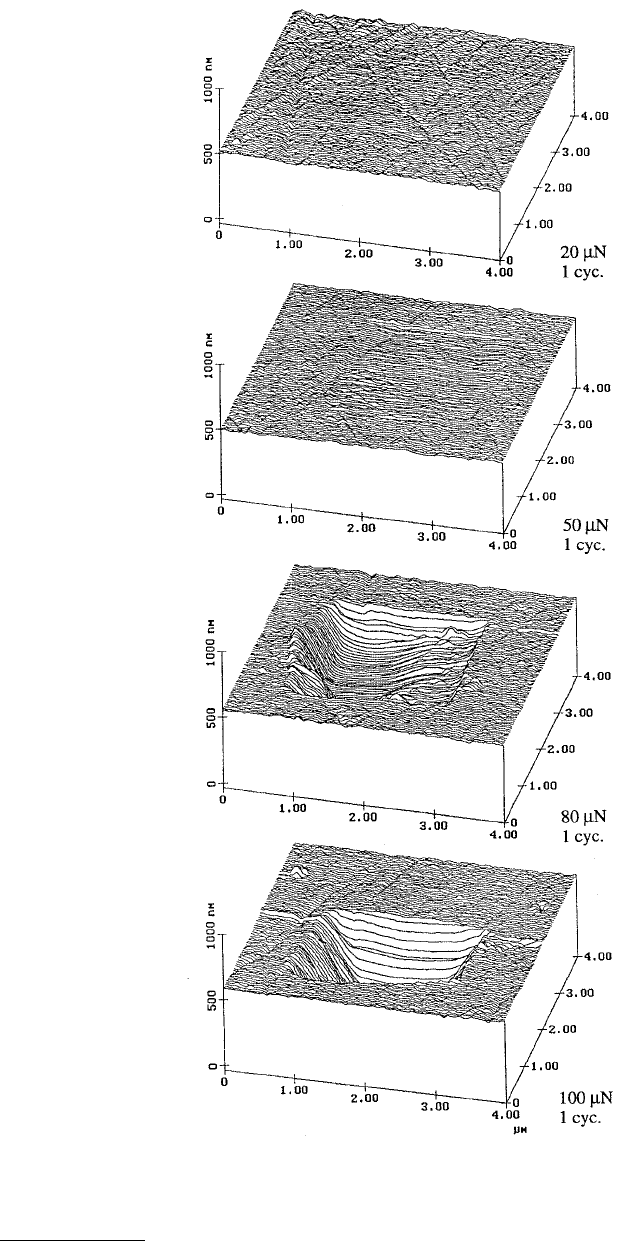
FIGURE 14.34 Surface maps of a polished, unlubricated thin-ﬁlm rigid disk showing the worn region (center 2 ×

2 µm) after one cycle. The normal loads are indicated in the ﬁgure. (From Bhushan, B. et al., 1994, Proc. Inst. Mech.

Eng. Part J: J. Eng. Tribol. 208, 17–29. With permission.)

Fomblin Y and Z are most commonly used for lubrication of particulate and thin-ﬁlm rigid disks,

respectively. Lubricants with lower viscosity (such as Fomblin Z-types) with polar end groups (Z-Dol

and AM 2001) are most commonly used in thin-ﬁlm disks in order to minimize stiction.

14.8.1 Imaging of Lubricant Molecules

Kaneko et al. (1991b) and Andoh et al. (1992) used scanning tunneling microscope (STM) to observe

the conﬁguration, adsorption, and mobility of perﬂuoropolyether lubricants on HOPG (defect-free and

inactive surface), MoS

, and sputtered carbon (active surface). (Also see Foster and Frommer, 1988.)

Their study revealed that the lubricant molecules can easily change position and are mobile. They found

that adsorption of the lubricant molecules on a sputtered carbon surface is more than that on the HOPG.

14.8.2 Measurement of Localized Lubricant Film Thickness

The local lubricant thickness is measured by Fourier transform infrared spectroscopy (FTIR), ellipsom-

etry, angle-resolved X-ray photon spectroscopy (XPS), STM, and AFM. Ellipsometry and angle-resolved

XPS have excellent vertical resolution, on the order of 0.1 nm, but their lateral resolutions are on the

order of 1 and 0.2 mm, respectively. AFM can measure the thickness of the liquid ﬁlm with a lateral

resolution on the order of the tip radius, about 100 nm, which it is not possible to achieve by other

techniques (Bhushan, 1993).

The schematic of AFM used for measurement for localized lubricant-ﬁlm thickness by Mate et al.

(Mate et al., 1989, 1990; Bhushan and Blackman, 1991) is shown in Figure 14.58. The lubricant thickness

is obtained by measuring the forces on the tip as it approaches, contacts, and pushes through the liquid

ﬁlm. In the left part of Figure 14.58 is a diagram of an AFM tip interacting with a lubricant-covered

particulate rigid disk. A typical force-vs.-distance curve for a tungsten tip of radius ~100 nm dipped into

a disk surface coated with a perﬂuoropolyether lubricant is shown in Figure 14.59. As the surface

approaches the tip, the liquid wicks up, causing a sharp onset of attractive force. The so-called meniscus

force experienced by the tip is ~4πRγ, where R is the radius of the tip and γ is the surface tension of the

liquid. In Figure 14.59, the attractive force measured is about 5 × 10

-8

N. While in the liquid ﬁlm, the

forces on the lever remain constant until repulsive contact with the disk surface occurs. The distance

between the sharp snap-in at the liquid surface and the hard wall of the substrate is proportional to the

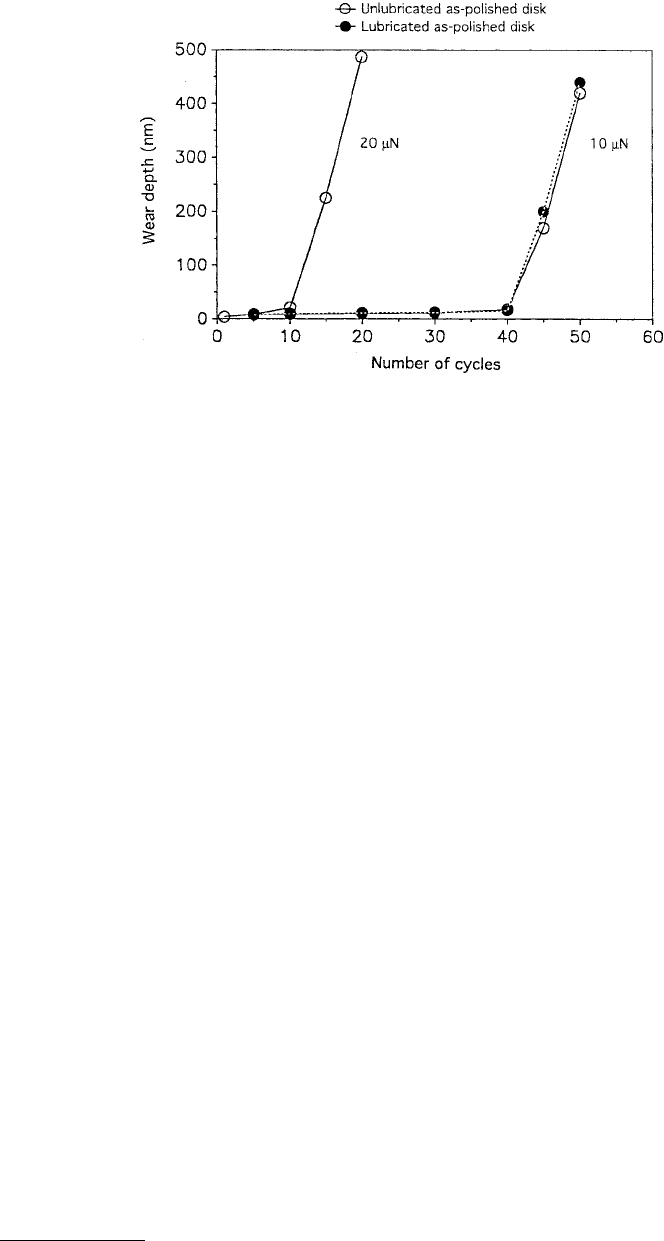
FIGURE 14.35 Wear depth as a function of number of cycles for polished, lubricated, and unlubricated thin-ﬁlm

rigid disks at 10 µN and for polished, unlubricated disk at 20 µN. (From Bhushan, B. et al., 1994, Proc. Inst. Mech.

Eng. Part J: J. Eng. Tribol. 208, 17–29. With permission.)

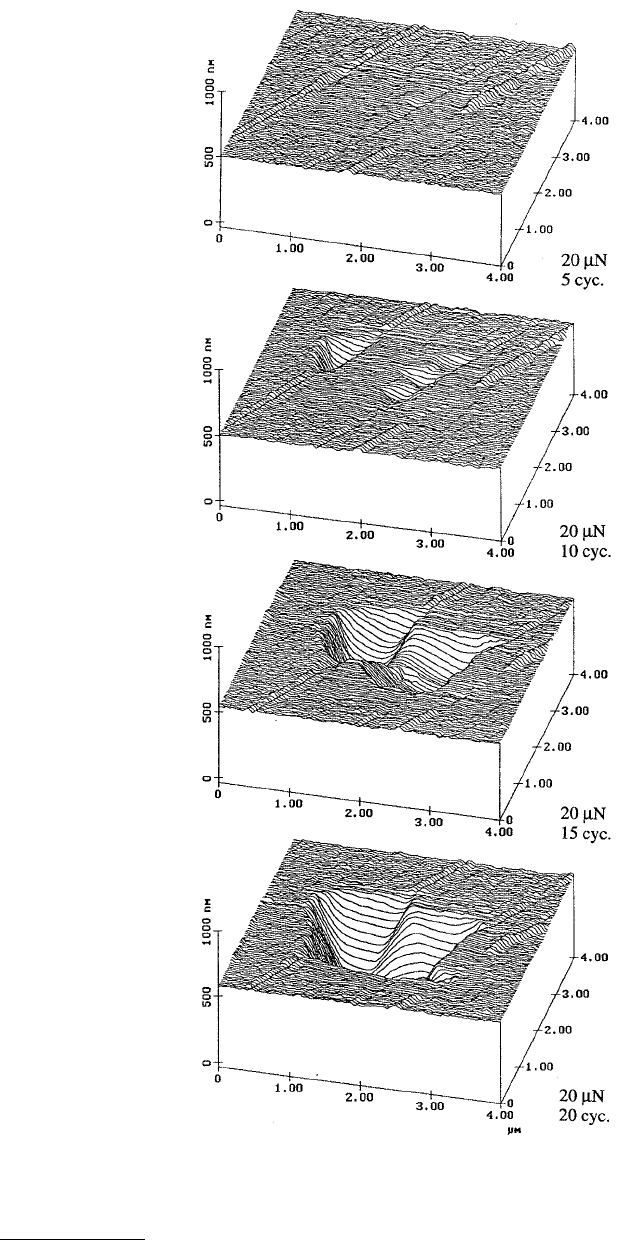
FIGURE 14.36 Surface maps of a polished, unlubricated thin-ﬁlm rigid disk showing the worn region (center 2 ×

2 µm) at 20 µN. The number of cycles are indicated in the ﬁgure. (From Bhushan, B. et al., 1994, Proc. Inst. Mech.

Eng. Part J: J. Eng. Tribol. 208, 17–29. With permission.)