Blank B.E., Krantz S.G. Calculus: Single Variable

Подождите немного. Документ загружается.

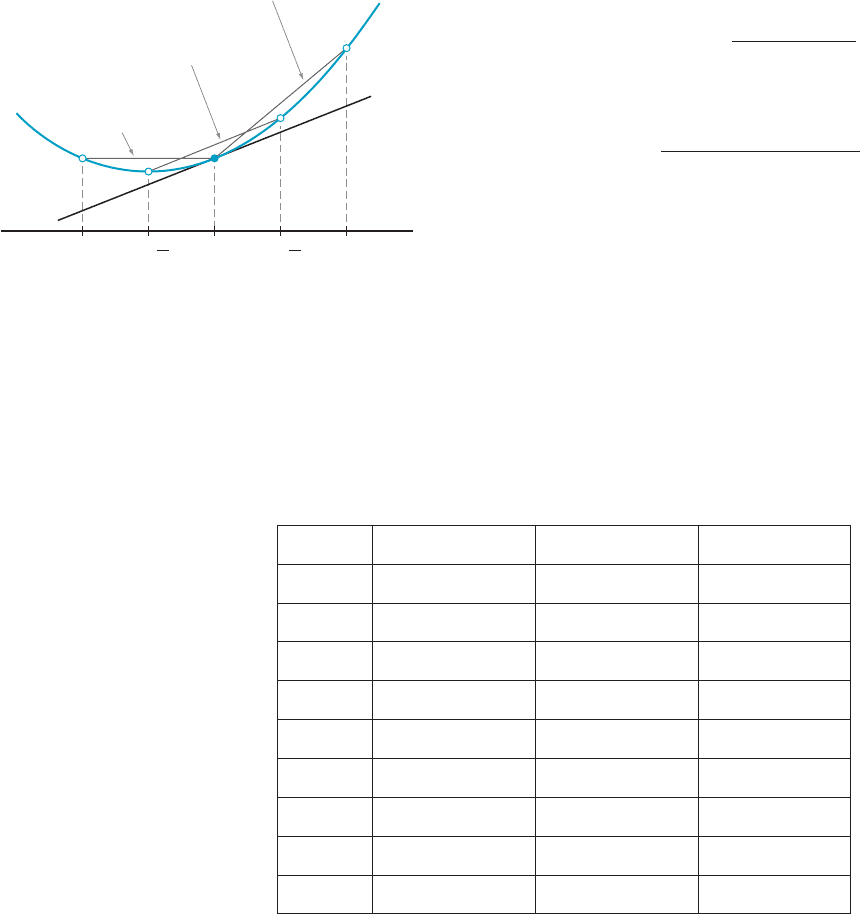
is known as a forward difference quotient. The backw ard

difference quotient is deﬁned by

f ðc; hÞ5

f ðcÞ2 f ðc 2 hÞ

where h . 0. The central difference quotient is deﬁned by

f ðc; hÞ5

f ðc 1 h=2Þ2 f ðc 2 h=2Þ

: ð3:3:1Þ

Each of these three difference quotients can be used to

approximate f

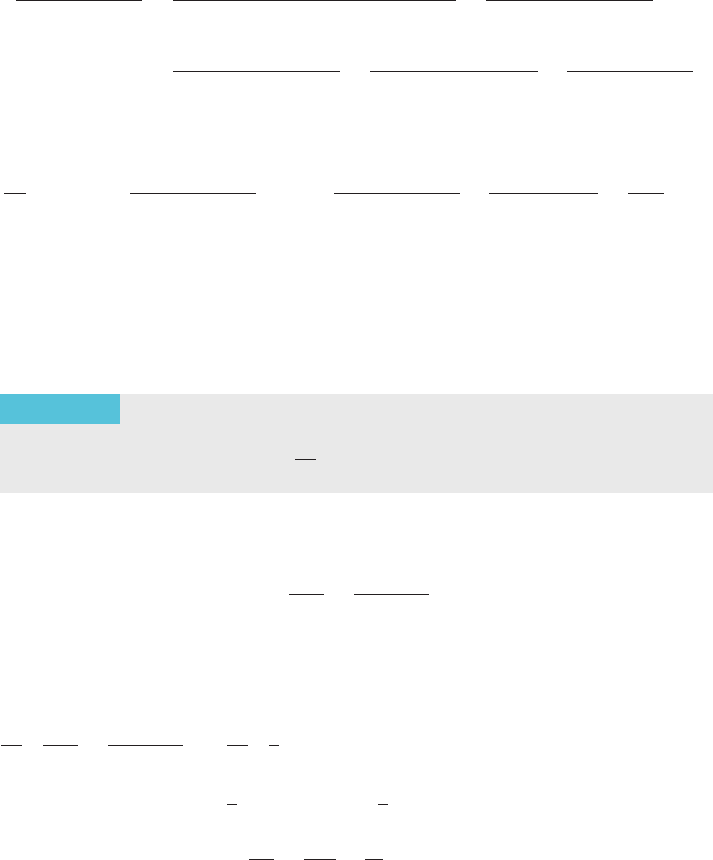
ðcÞ. However, the situation illustrated by

Figure 1 is typical: For a given value of h, the central dif-

ference quotient generally gives the best approximation to

the derivative.

When we use the approximation

f ðc; hÞf

ðcÞ;

the question of how to choose h in an optimal way is not easy to answer. Consider

f (x) 5 x

and c 5 1/2. The exact value of f

ðcÞ is 1/2. Now consider the following

table of difference quotients (which were computed using ten signiﬁcant digits, of

which six are exhibited):

f (c, h ) D

f (c, h) D

f (c, h)

0.671 0.369 0.505

0.505201 0.485199 0.50005

0.501502 0.498502 0.500001

0.50015 0.49985 0.5

0.500002 0.499998 0.5

0.499999 0.499999 0.500002

0.500222 0.499995 0.500222

211

0.500222 0.500222 0.477485

212

0.454747 0.500222 0

Table 1

you read down each column, you will notice that, with decreasing h, each

difference quotient seems to approach the limit 0.5 at ﬁrst. Observe that the central

difference quotient converges quickest. In each column, howeve r, accuracy is

degraded when h becomes too small. This is the result of loss of signiﬁcance,a

problem that is discussed in Section 1.1.

It is difﬁcult to predict the value of h at which accuracy begins to deteriorate.

There are two types of error that result when we use D

f (c, h) to approximate f

ðcÞ.

c  hc  hc

Tangent line

Slope  Central

difference

quotient

Slope  Forward

difference

quotient

Slope  Backward

difference

quotient

c 

c 

m Figure 1

3.3 Rules for Differentiation 195

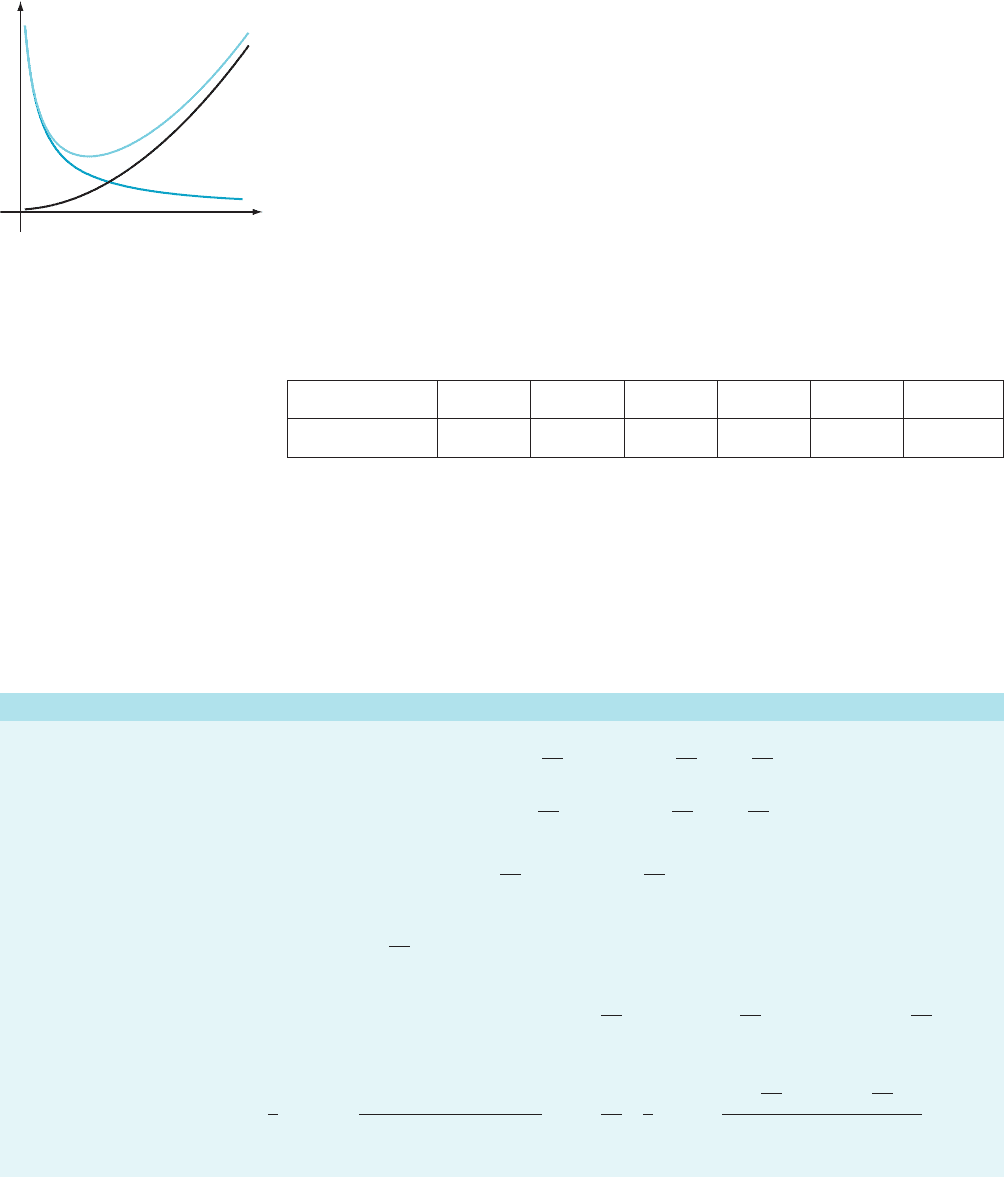
As we have seen, there is a roundoff error r(h). Terminating the limit process

ðcÞ 5 lim

h-0

f ðc; hÞ by stopping at a particular value of h also introduces a

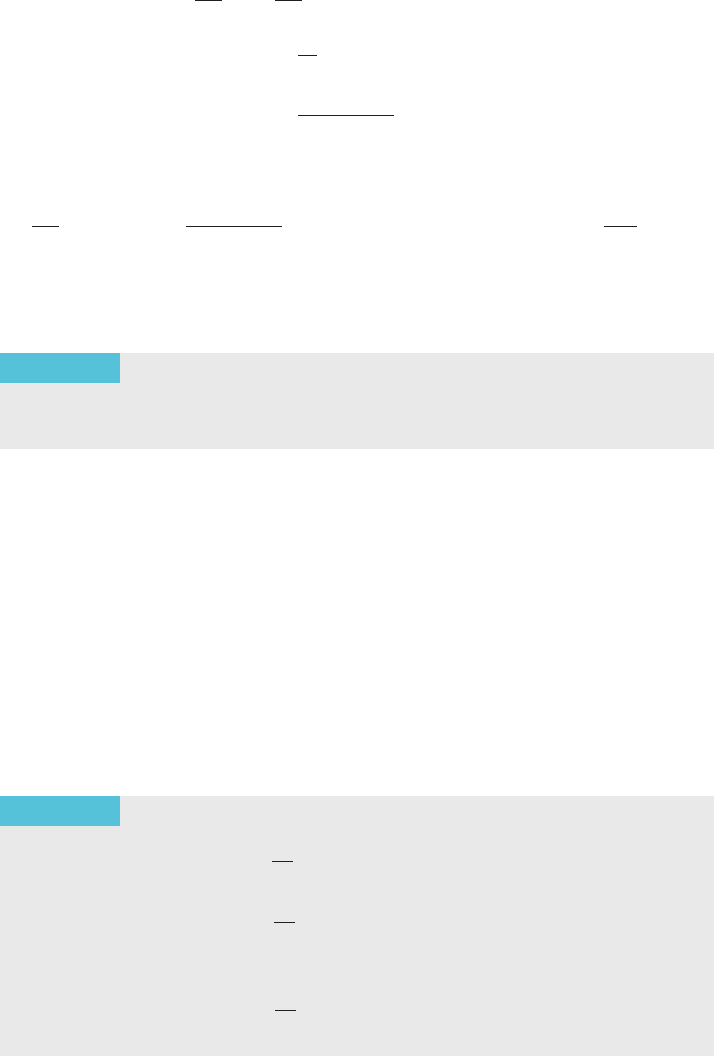
truncation error t(h). The total error e(h)isgivenbye(h) 5 t(h) 1 r(h). Typical

graphs of these error functions can be seen in Figure 2. Unfortunately, there is no

way to ensure that t(h)andr(h)areboth very small. One strategy is to let h become

smaller and smaller until either the desired accuracy is attained or the total error

begins to increase.

⁄ EX

AMPLE 6 Let VðxÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 1 sinðxÞ

: Approximate V

(0.4174) to four

signiﬁcant digits.

Solution Table

2 presents the results of our central difference quotient

calculations. (Ten digits have been carried in the computations, six digits are

tabulated, but no more than four are signiﬁcant.)

h 0.1 0.01 0.001 0.0001 0.00001 0.000001

V(0.4174, h) 0.294630 0.294708 0.294709 0.294710 0.294700 0.295000

Table 2

Bec

ause the leftmost four digits of D

V(0.4174, h) agree when h 5 10

and 10

we can be reasonably certain that V

(0.4174) 5 0.2947 to four signiﬁcant digits

(and that the last column represents a decline in accuracy caused by using too small

a value for h). In Section 3.5, we will be able to show that V

ðxÞ5 cos ðxÞ=



ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 1 sinðxÞ



: With this formula for V

(x), we can verify that our numerical

differentiation is correct to four signiﬁcant digits.

Summary of Differentiation Rules

Sum Rule

ðf 1 g Þ

ðxÞ5 f

ðxÞ1 g

ðxÞ or

ðf 1 gÞðxÞ5

ðxÞ1

ðxÞ

Difference Rule

ðf 2 gÞ

ðxÞ5 f

ðxÞ2 g

ðxÞ or

ðf 2 gÞðxÞ5

ðxÞ2

ðxÞ

Constant Multiple Rule

ðα  f Þ

ðxÞ5 α ðf

ðxÞÞ or

ðα  f ÞðxÞ5 α

ðxÞ

Constant Rule

ðαÞ

5 0or

α 5 0ifα is any constant

Product Rule

ðf  gÞ

ðxÞ5 f

ðxÞgðxÞ1 f ðxÞg

ðxÞ or

ðf  gÞðxÞ5



ðxÞ



gðxÞ1 f ð x Þ

ðxÞ

Quotient Rule





ðxÞ5

gðxÞf

ðxÞ2 f ðxÞg

ðxÞ

gðxÞ





ðxÞ5

gðxÞ

ðxÞ2 f ðxÞ

ðxÞ

gðxÞ

e(h)  t(h)  r(h)

r(h)

t(h)

m Figure 2

196 Chapter

3 The Derivative

Reciprocal Rule





ðxÞ52

ðxÞ

gðxÞ





ðxÞ52

ðxÞ

gðxÞ

Approximate Derivative Rule

ðcÞ

f ðc 1 h=2Þ2 f ð c 2 h=2Þ

Derivatives of Basic Functions

ðx

Þ 5 nx

n21

for n 521; 0; 1; 2; 3

sinðxÞ 5 cosðxÞ

cosðxÞ 52sinðxÞ

secðxÞ 5 secðxÞtanðxÞ

QUICK QUIZ

1. What is the derivative of x sin (x)?

2. What is the derivative of cos (x)/x?

3. What is the derivative of sec (x)?

4. Use the central difference quotient with h 5 0.1 to app roximate the derivative of

tan (x)atx 5 0.

Answers

1. sin (x) 1xcos

(x)2.2(x sin (x ) 1 cos (x))/x

3. sec (x) tan (x) 4. 1.00083

EXERCISES

Problems for Practice

c In Exercises 16, use the rules for differentiating sums and

differences, as in Example 1, to compute the derivative of the

given expression with respect to x. b

1. 5x

2 3x

1 9

2. 6 2 4/x 1 4x2x

/2 2 2x

3. x

/π 1 π cos (x) 1

ﬃﬃﬃ

4. 3x

2 2x

1π sin (x) 1 1/π

ð4sinðxÞ2 3cosðxÞÞ1 5x

ﬃﬃﬃ

ðsinðxÞ2 2cosðxÞÞ1 2x

2 3=x

c In Exercises 718, use the Product Rule to compute the

derivative

of the given expression with respect to x. (In each

of Exercises 7, 8, 14, 16, and 18, do not avoid using the

Product Rule by ﬁrst expanding the product.) b

7. x

(x 1 3)

8. 4x(x

1 5)

9. x

cos (x)

10. 5 sin (x) 2 6x cos ( x)

11. sin

(x)

12. cos

(x)

13. sin (x) cos (x)

14. (x

2 5)(3x 1 2)

15. 7(x

1 x) sin (x)

16. 3x

1 7)

17. (1/x) sin (x)

18. (1/x) (5x

1 4/x)

c In Exercises 1926, use the Reciprocal Rule to compute

the

derivative of the given expression with respect to x. b

19. 1/(x 1 1)

20. 1/(11x

)

21. 2/(3x

1 4)

22. 3/(2 1 sin (x))

23. 1/(x 1 3 cos (x))

24. 5/(cos (x) 1 sin (x))

25. 9/(3 cos (x) 1 x

)

26. 1/(x

1 1)

3.3 Rules for Differentiation 197

c In Exercises 2738, use the Quotient Rule to compute the

derivative of the given expression with respect to x. b

27. x/(x 1 1)

28. 3x/(x

1 1)

29. (x 2 1)/(x 1 1)

30. (x

1 1)/(x

1 2)

31. (5x 2 2/x)/x

32. x/sin (x)

33. sin (x)/x

34. cos (x)/(x 2 5)

35. (x

1 7)/cos (x)

36. 2x

/(1 1 cos (x))

37. (1 2 cos (x))/(1 1 cos (x))

38. (x 1 sin (x))/(x 1 cos (x))

c Calculate the derivative of each of the expressions in

Exercises 3944 by applying both the Product and Quotient

Rules. b

39. x sin

(x)/(x 1 1)

40. x cos (x)/(x

1 3)

41. x (x

1 1) (x

1 2)

42. sin

(x)/x

43. sin

(x) sec (x)

44. x sin (x)/(x 1 sin (x))

c In Exercises 4550, ﬁnd the tangent line to the graph of

the

given function at the given point. b

45. f (x) 5 (x 1 3)/(x 1 1), P 5 (1,

46. f (x) 5 x cos (x), P 5 (π, 2π)

47. f (x) 5 6/(11x

), P 5 (1, 3)

48. f (x) 5 4 sin (x) cos (x), P 5 (π/4, 2)

49. f (x) 5 sin (x)/(x 1 1), P 5 (0, 0)

50. f (x) 5 (3x

2 5)/(x 1 4), P 5 (1, 22/5)

c In Exercises 5154, use the result of Example 4 to compute

e derivative of the given expression with respect to x. b

51. x sec

(x)

52. sec (x)/x

53. 1/(1 1 2 sec (x))

54. (sec (x) 2 cos (x))/sin (x)

c In Exercises 5558, use the given information to estimate

ðcÞ at the given point c. b

55. f (4) 5 5.7

and f (4.1) 5 6.2, c 5 4

56. f (4) 5 5.7 and f (4.1) 5 6.2, c 5 4.1

57. f (π 1 0.01) 5 f (π) 1 0.2, c 5 π

58. f (3.47) 5 2.61 and f (3.49) 5 2.67, c 5 3.48

Further Theory and Practice

59. Given f (2) 5 3, f

(2) 5 5, g(2) 5 8, and g

(2)526, ﬁnd

( f g)

(2) and ( f/g)

(2).

60. Given f (3)524 and f

(3) 5 6, ﬁnd (1/f )

(3) and ( f

)

(3).

61. Given f (21) 5 5, g(21) 5 4, f

(21) 5 2, and g

(21)528,

ﬁnd ( f g)

(21), ( f

)

(21), and (g

)

(21).

62. Given f (4) 5 2, g(4) 5 1, f

(4)525, and g

(4)529, ﬁnd

(f  g

)

(4), (g  f

)

(4), (1/f

)

(4), and (g/ f )

(4).

63. Use the Quotient Rule to differentiate

x 1 1=x

1=x

Verify your answer by simplifying the expression and

then differentiating.

64. Derive a formula for

by applying the Product Rule

to x x

. Verify your answer by applying the Product

Rule to x

x

c In Exercises 6570, calculate the derivative of the given

expression. b

65. x

66. (x

1 1) (x

1 2) sin (x)

67. x sin (x) cos (x)

68. sin

(x)

69. (x

1 1)

70. sin (2x)

71. The graph of f (x) 5 2x/(x 1 1) and the point P 5 (2, 4),

which lies above the graph, are shown in Figure 3. The

purpose of this exercise is to ﬁnd the equation of every

tangent line to the graph of f that passes through P. Copy

Figure 3 on your paper. To your sketch, add the tangent

lines that pass through P. How many do there appear to

be? You will use this predicted number N as a “reality

check” in part c.

a. What is the slope of the tangent line to the graph of f

at (c, f ( c))? (By inspection, the tangents to the graph

of f all slope upward. Before continuing, make sure

that your algebraic formula for the slope is consistent

with this property.)

b. What is the equation of the tangent line to the graph

of f at (c, f (c )).

c. What equation must c satisfy if the tangent line at

(c, f (c)) passes through P? (How many solutions does

this equation have? Before continuing, make sure that

the equation for c has the same number of solutions as

your predicted number N.)

4 2

2

y 

x  1

m Figure 3 The graph of f ðxÞ5

x 1 1

198 Chapter 3 The Derivative

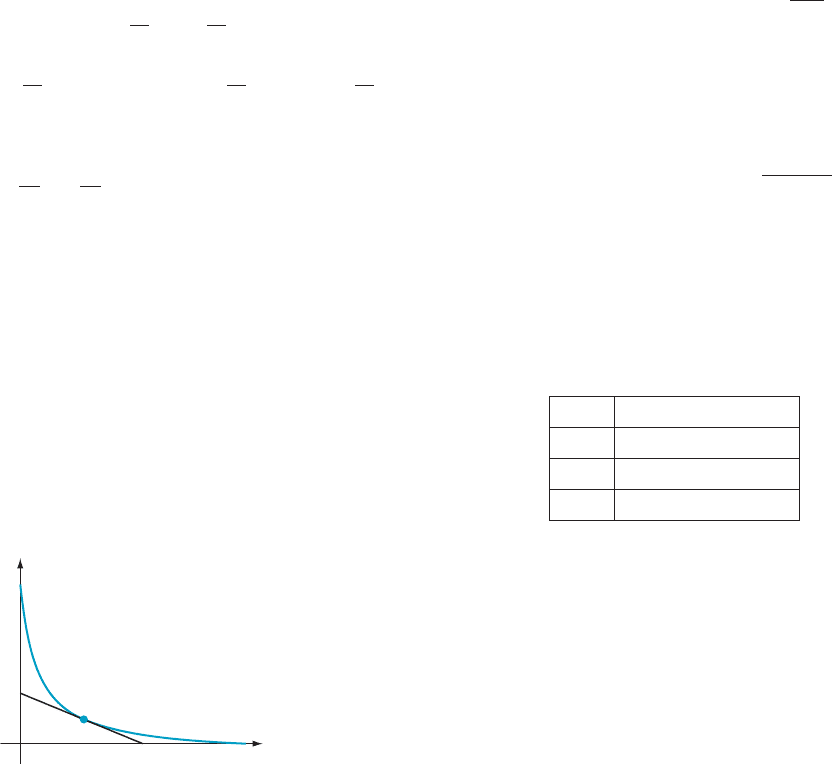
d. Find the equations of all tangent lines to the graph of f

that pass through P.

72. If f is a differentiable function, ﬁnd a formula for ( f

)

(x).

Next ﬁnd a formula for ( f

)

(x). What do you expect the

formula for ( f

)

(x) is? (Predict by analogy, but do not

prove this last formula. These matters will be taken up

later in the chapter.)

73. Find constants A and B such that f (x) 5 A cos (x) 1

B sin (x) satisﬁes

ðxÞ1 f ðxÞ5 3cosðxÞ2 5sinðxÞ:

74. Suppose that f (x) 5 (x 2 r)

p(x) where p(x) is a differ-

entiable function with p(r) 6¼0. Show that there is a dif-

ferentiable function q with q(r)6¼ 0 such that f

(x) 5

(x 2 r)

q(x).

75. Show that if f (x) 5 |x|, then ( f

)

(0) exists even though

(0) does not.

76. We know that

x 5 1;

1 5 0; and

ðxÞ1 1 f

ðxÞg5

ðxÞ1 1

ðxÞ:

Explain what is wrong with the following reasoning:

x ¼

1 þþ1

|ﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄ}

x summands

;

¼ 0 þþ0

|ﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄ}

;

¼ 0

77. Assume that g is differentiable and nonvanishing and that

1/g is differentiable. Derive the Reciprocal Rule for g

from the Product Rule applied to g and 1/ g.

78. Show that if f (x) 5 x

, then the central difference quo-

tient D

f (c, h) for approximating f

ðcÞ is exactly equal to

ðcÞ.

79. Show that the central difference quotient D

f (c, h)isan

average of a forward and a backward difference quotient.

80. Suppose that a consumer’s satisfaction with purchasing x

units of good X and y units of good Y can be quantiﬁed.

The locus of points (x, y) for which the consumer’s satis-

faction is a ﬁxed value is called an indifference curve: The

consumer is equally satisﬁed at all points on the curve.

A typical indifference curve is illustrated in Figure 4. Most

consumers have a ﬁxed budget for the purchase of goods

X and Y. The locus of points that represent the combi-

nation of goods X and Y that the consumer can actually

purchase on his budget is a straight line called the budget

line. The point of tangency between the budget line and

an indifference curve is called the consumer equilibrium.

See Figure 4. If (2, 1/5) is the equilibrium point for a

consumer whose indifference curve is

3yðx

þ 1Þþ5xðy þ 1Þ¼15;

ﬁnd the consumer’s budget line in the form

Ax þ By ¼ C:

81. If q( p) is the demand for a product at price p, that is, the

number of units of the product that are sold at price p,

then

EðpÞ52q

ðpÞ

qðpÞ

is deﬁned to be the elasticity of demand.

a. Let Δp denote a small change in p, and let Δq 5

q(p 1 Δp) 2 q(p) be the corresponding change in q.

Show that, for Δp sufﬁciently small, we have the

approximation

EðpÞ2

Δq=qðpÞ

Δp=p

b. Elasticity of demand for potatoes at standard super-

market prices has been estimated to be 0.31.

Approximately what percentage change in potato

consumption will result from a 2% increase in the

price of potatoes?

c. Over a ﬁxed period, a television repair service recor-

ded the number of repairs that it made at three dif-

ferent prices:

Price Contracts Obtained

$90 96

$80 200

$70 300

Estimate the elasticity of demand at p 5 $70 and at

p 5 $90. Use a central difference quotient to estimate

elasticity of demand at p 5 $80. (Elasticity of demand

for television repair was estimated to be 3.84 in the

1970s.)

82. Let f

(x) 5 x

for each nonnegative integer n. Notice that

n11

(x) 5 xf

(x). Use the Product Rule to show that

nþ1

ðxÞ¼f

ðxÞþf

ðxÞ:

Use this equation to show that f

n11

(x) 5 (n 1 1)x

(x) 5 nx

n 2 1

. In other words, if the formula

Indifference curve for

good X and good Y

Consumer equilibrium

Budget

line

m Figure 4

3.3 Rules for Differentiation 199

5 nx

n21

is valid for a nonnegative integer n, then it is valid for the

next integer n 1 1. In fact, we know that this differ-

entiation formula is true for n 5 0, 1, 2, and 3. It follows

that the formula is true for the next integer 4. Because

the formula is true for 4, it is true for the next integer 5,

and so on. We deduce that

5 nx

n21

for all nonnegative integers n:

Calculator/Computer Exercises

c In Exercises 8386, a function f and a point c are given.

Prepare a table of the forward, backward, and central dif-

ference quotients, D

f (c, h), D

f (c, h) respec-

tively, for h 5 10

,1# n # 5. b

83. f (x) 5 cos

(πx), c 5 1

84. f (x) 5 sin (πx), c 5 1/4

85. f ðxÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 1=x

; c 5 3

86. f (x) 5 (x

1 3x

1 2) /(x

2 2), c 5 2

c In Exercises 8790, ﬁnd f

ðcÞ (exactly) for the given

function f and the given value c of x. Then approximate f

ðcÞ

to 5 decimal places by (1) ﬁnding a ﬂoating point evaluation

of the exact answer and (2) using a central difference quotient

f (c, h). Record the value of h used. b

87. f (x) 5 sin

(x), c5 π /6

88. f ðxÞ5

ﬃﬃﬃﬃﬃ

; c 5 1

89. f ðxÞ5 1=x; c 5 1=

ﬃﬃﬃ

90. f (x) 5 cos (x), c 5 π/4

91. Let f (x) 5 cos (x). Graph x / to D

f (x,10

) for

0 # x # 2π. What is f

(x)? Add the graph of f

(x) to your

viewing window. (The graphs should nearly coincide.)

92. Let f (x) 5 x

/4. Graph x/ D

f (x,10

)for21.5 # x # 1.5.

What is f

(x)? Add the graph of f

(x) to your viewing

window. (The graphs should nearly coincide.)

93. The purpose of this exercise is to locate a value a

such

that



x50

5 1:

Let f

(x) 5 a

. Set L(a) 5 D

(0, 10

). Compute L(2.70)

and L(2.73). Graph L for 2.70 # a # 2.73 to see that there

is a value a

in [2.70, 2.73] such that L(a

) 5 1. Narrow

down the interval in which a

must lie until you have

found a

to three decimal places.

94. Let f (x) 5 sin

(x). Graph x / D

f (x,10

) for 0 # x #

2π. Now compute f

, and add the graph to the viewing

window. (The graphs should nearly coincide.)

3.4 Differentiation of Some Basic Functions

In the preceding section, we learned how to differentiate functions that are formed

arithmetically from simpler functions, provided that we know how to differentiate

the simpler ones. The purpose of this section is to build up the library of basic

functions that we can differentiate. Your work in calculus will be easier if you

commit these derivatives to memory.

Powers of x By now we have learned to differentiate x

for the integers p 521, 0, 1, 2, and 3.

For these values of p, we know that

5 px

p21

: ð3:4:1Þ

In our ﬁrst example, we extend equation (3.4.1) to include all positive integer

values of p.

⁄ EX

AMPLE 1 Suppose that p is a positive integer. Let f (x) 5 x

. Show that

ðcÞ5 pc

p21

Solution Because

we already know that the asserted formula is true for p 5 1, we

assume that 1 , p. Because p21 is positive, we have lim

x-0

p21

5 0. Thus formula

(3.2.3) with c 5 0 simpliﬁes as follows:

200 Chapter 3 The Derivative

ð0Þ5 lim

x-0

f ðxÞ2 f ð0Þ

x 2 0

5 lim

x-0

2 0

x 2 0

5 lim

x-0

p21

5 0 5 p  0

p 21

Having establi shed the desired formula for c 5 0, we now assume that c 6¼0 and set

r 5 x/c. Notice that r - 1asx-c. Using formula (3.2.3), we have

ðcÞ5 lim

x-c

f ðxÞ2 f ðcÞ

x 2 c

5 lim

x-c

2 c

x 2 c

5 lim

x-c

ðx=cÞ

2 1

x=c 2 1

5 c

p21

lim

r-1

2 1

r 2 1

We now appeal to equation (2.5.2) with N 5 p to obtain

ðcÞ5 c

p21

lim

r-1

1 1 r 1 r

1 1 r

p21

|ﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ}

p summands

5 c

p21

 1 1 1 1 1

1 1 1

p21

|ﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ}

p summands

5 pc

p21

: ¥

INSIGHT

Equation (3.4.1) refers to a variable x. By contrast, the derivative formula

of Example 1 was obtained by treating c as a ﬁxed point. This choice was made to

facilitate the notation used in the solution. We should now observe that Example 1

establishes f

ðcÞ5 pc

p21

for every value of c. In other words, we may regard c as a

variable in the formula. With that understanding, we may replace c with the more

conventional choice of variable x. We then obtain f

(x) 5 px

p21

, which is precisely

equation (3.4.1) with a different choice of notation for the derivative.

We now know that equation (3.4.1) holds for all integer values of p with

21 # p. In our next example, we extend this result to all integer values of p.

⁄ EX

AMPLE 2 Use the Reciprocal Rule to show that formula (3.4.1) holds

for all integer values of p with p #22.

Solution Suppos

e that p #22. Write p 52jpj, and set g(x) 5 x

jpj

. From Example

1, we know that g

(x) 5 jpj x

jpj21

. Therefore using the Reciprocal Rule, we have

2jpj



jpj





gðxÞ



ðxÞ

jpjx

jpj21

2jpj

5 px

2jpj21

5 px

p21

The next example shows that formula (3.4.1) is also valid for p 5 1/2.

⁄ EXAMPLE 3 For p 5 1/2, formula (3.4.1) can be written as

ﬃﬃﬃ

: ð3:4: 2 Þ

Verify that equation (3.4.2) holds for all x . 0.

3.4 Differentiation of Some Basic Functions 201

Solution We verify this formula by referring to the deﬁnition of the derivative.

Since

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx



ﬃﬃﬃ

Δx

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx



ﬃﬃﬃ

Þð

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx

ﬃﬃﬃ

Δxð

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx

ﬃﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x1Δx

ð

ﬃﬃﬃ

Δxð

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx

ﬃﬃﬃ

ðx 1 ΔxÞx

Δxð

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx

ﬃﬃﬃ

Δx

Δxð

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx

ﬃﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx

ﬃﬃﬃ

;

we conclude that

ﬃﬃﬃ

5 lim

Δx-0

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx



ﬃﬃﬃ

Δx

5 lim

Δx-0

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 Δx

ﬃﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x 1 0

ﬃﬃﬃ

: ¥

Now that we have veriﬁed formula (3.4.1) for one value of p that

is not an

integer, it is reasonab le to wonder if (3.4.1) is valid for other values of p. Indeed, it

is. We record this fact as a theorem for now, but we defer the proof until Section

5.6. In the meantime, you should use this differentiation formula whenever it is

needed.

THEOREM 1

(The Power Rule)Ifp is any real number, then

5 px

p21

⁄ EX

AMPLE 4 Differentiate

7=3

2 2

with respect to x .

Solution After

rewriting the given expression as a sum of three monomials, we use

Theorem 1 with p 5212, p 5 1/3, and p 522:



7=3

2 2





212

1 6x

1=3

2 2x



ð212Þx

213

1 6





22=3

2 2ð22Þx

2=3

: ¥

⁄ EXAMPLE 5 In an adiabatic (no heat in or out) argon system, the third

wer of pressure (P) is inversely proportional to the ﬁfth power of volume (V). At a

pressure of 1000 atmospheres (atm), the volume of the argon sample is 34 cm

. What

is the rate of change of pressure with respect to volume when the sample is 50 cm

Solution According

to the given information, there is a proportionality constant k

such that P

5 k/V

,orP 5 cV

25/3

where c 5 k

1/3

. Our ﬁrst step is to ﬁnd the value of

c by substituting P 5 1000 atm and V 5 34 cm

into the equation P 5 cV

25/3

.We

obtain

202 Chapter 3 The Derivative

1000 atm 5 c ð34 cm

25=3

;

or c 5 1000  34

5/3

atm 3 cm

. Therefore using Theorem 1 with p 525/3, we calculate

P 5

ðcV

25=3

28=3

5000  34

5=3

28=3

atm cm

and



V550 cm

5 

5000  34

5=3

3 ð50 cm

8=3

atm 3 cm

17:5

atm

: ¥

Before we turn our attention to other types of functions, we prove one simple

consequenc

e of the Power Rule.

THEOREM 2

The derivative p

of a polynomial p is a polynomial. If p is not a

constant, then the degree of p

is one less than the degree of p.Ifp is a constant,

then p

is identically 0.

Proof. If p(x) 5 a

1 a

N21

1 1 a

1 a

x 1 a

with a

6¼0, then, on

applying the Power Rule to each term of p(x), we obtain

ðxÞ5 N  a

N21

1 ðN 2 1Þa

N21

N22

1 1 2 a

x 1 a

;

from which the asserted results follow. ’

Trigonometric

Functions

We continue to build our library of differentiation formulas by ﬁnding the de ri-

vatives of the remaining trigonometric functions. We have already seen how to

differentiate sine and cosine (Section 3.2) and secant (Example 4 of Section 3.3).

The other trigonometric functions can now be handled easily. Before doing so,

recall that we often write sin

(x) and tan

(x) rather than (sin (x))

and (tan (x))

THEOREM 3

The derivatives of tan (x), cot (x), and csc (x) are

tanðxÞ5 sec

ðxÞ; ð3:4:3Þ

cotðxÞ52csc

ðxÞ; ð3:4:4Þ

and

cscðxÞ52csc ðxÞcotðxÞ: ð3:4:5Þ

3.4 Differentiation of Some Basic Functions 203

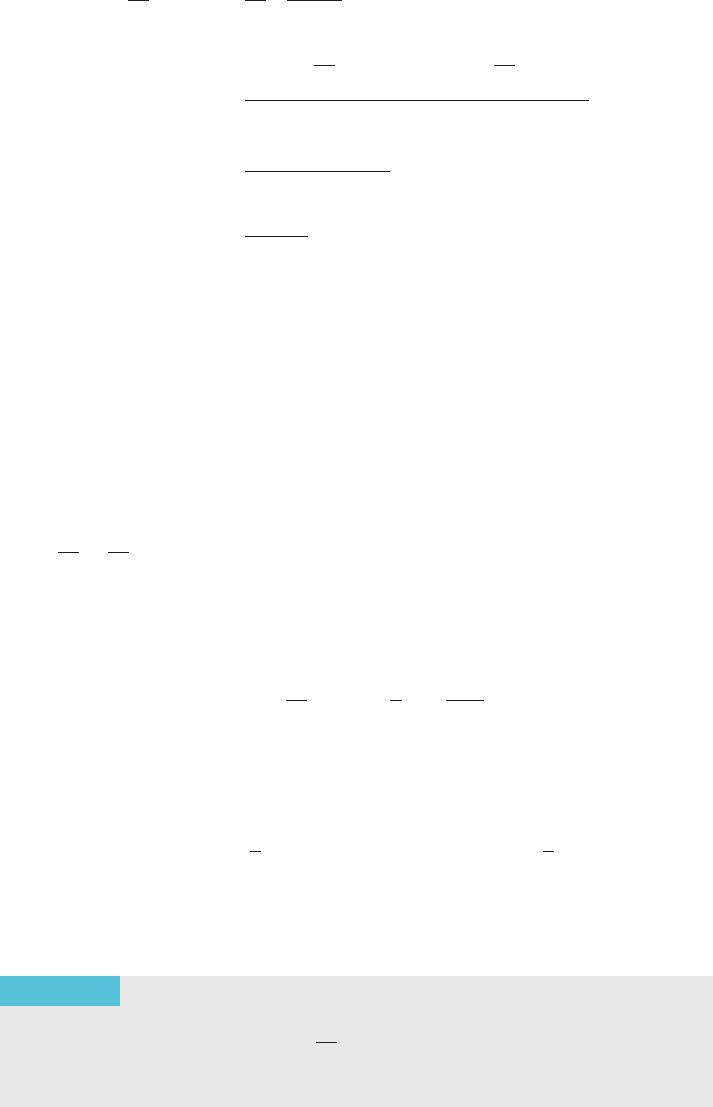
Proof. We use the Quotient Rule to prove formula (3.4.3):

tanðxÞ 5



sinðxÞ

cosðxÞ



cosðxÞ

ðsinðxÞÞ2 sinðxÞ

ðcosðxÞÞ

cos

ðxÞ

cos

ðxÞ1 sin

ðxÞ

cos

ðxÞ

cos

ðxÞ

5 sec

ðxÞ:

The proof of formula (3.4.4) is similar and is left as Exercise 66. The proof of

equation (3.4.5) is analogous to the derivation of formula (3.3.1) and is left as

Exercise 67. ’

⁄ EX

AMPLE 6 Find the tangent line to the graph of y 5 x cot

(x) at the point

ðπ=6; π

ﬃﬃﬃ

=6Þ:

Solution We

ﬁrst ﬁnd the slope. Using the Product Rule, we calculate:



xcotðxÞ



5 1  cotðx Þ1 x 



 csc

ðxÞ



5 cotðxÞx  csc

ðxÞ:

Evaluating at x 5 π/6, we ﬁnd the slope to be

ﬃﬃﬃ

2 ðπ=6Þ2

ﬃﬃﬃ

2 2π=3:

According to formula (3.1.12), the point-sl ope equation of the tangent line is

y 5



ﬃﬃﬃ

2π







x 2



ﬃﬃﬃ

: ¥

The Derivative of the

Natural Exponential

Function

In Section 2.6, we introduced the number e, and, in Example 8 from that section,

derived the basic formulas

5 lim

n-N





and e

5 lim

n-N





: ð3:4:6Þ

We will now see that the exponential function x/e

, or exp(x), has the remarkable

property that it is its own derivative.

THEOREM 4

For every x, we have

5 e

ð3:4:7Þ

or, equivalently,

204 Chapter 3 The Derivative