Henini M. Handbook of Self Assembled Semiconductor Nanostructures for Novel devices in Photonics and Electronics
Подождите немного. Документ загружается.

Effects of the Electron–Phonon Interaction in Semiconductor Quantum Dots 349
where |β〉
labels eigenstates of the exciton Hamiltonian and ρ
n
is the probability of the phonon state
|n〉
in the equilibrium statistical ensemble of the phonon systems:
ρ
n
n
B
kT
1
Z
E
ph
exp
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
Z
ph
is the partition function of the free phonon subsystem and E
n
is the energy of the phonon
state
|
n
〉
. Using the well-known Feynman relations [50] for operator exponentials, one obtains
ee e
ph
ex
i
ii
Ht
Ht H t
Ut
()
(11.5)
where the evolution operator:
Ut
i
dt H t
t
() exp ( )T
int
11
0
∫
⎛
⎝
⎜
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
⎟
⎟
(11.6)
is represented in terms of the chronological ordering operator T , while
At A
ii
HHt HHt
()
()()
ee
ex ph ex ph
denotes the operator A in the interaction representation. Hence, Eq. (11.1) takes the form:
αββ
β
β
β
β
()
()
Re ( )
*
()
,
Ω
Ω
Ω
ΩΩ
8
3
0
π ᏺ
cn
dd dt Ut
it
′
∞
〈
′
〉
∫
e||
i
ph
〈〉
′′
∑
β
(11.7)
where Ω
β
is the Franck–Condon frequency of generation of an exciton in the state
|β〉
and
dd
β
β≡〈 〉||0
is the dipole matrix element of a transition between the exciton vacuum state and
the state
|β〉
,
〈…〉 ≡ 〈 … 〉
∑
ph
ρ
n
n
nn||
denotes the averaging over the phonon ensemble.
Using Eq. (11.3) and neglecting the dispersion of the frequency of the optical phonons ω
0
,
one obtains the following result of the averaging over the equilibrium phonon ensemble in Eq.
(11.7):
U t dt dt n t t
t
t
tt
() Texp ( )e ( ) (
ph
i( )
1
1
2
1
0
2
0
1
1
01 2
∫∫
∑
λ
ω
λ
λ
γγ
†
22
12
01 2
)
e()()
i( )
⎡
⎣
⎢
⎧
⎨
⎪
⎪
⎪
⎩
⎪
⎪
⎪
⎤
⎦
⎥
}
ntt
ttω
λ
λ
γγ
†
(11.8)
where:
n
k
B
T
e
ω
0
1
1
⎛
⎝
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
.
(11.9)
The absorption coeffi cient α ( Ω ) of Eq. (11.7) with Eq. (11.8) can be analytically calculated
at arbitrary exciton–phonon coupling (i) when the exciton levels are non-degenerate (this case
was analysed by Pekar [35] and Huang-Rhys [36] ) and (ii) when the vibrational modes inter-
acting with an exciton are adiabatic, i.e. the matrices
|| | | ||〈
′
〉βγ β
λ
in the basis of the degener-
ate level can be simultaneously diagonalized (this case is referred to as a static Jahn–Teller effect
[41–44] ).
However, these analytical approaches cannot be applied for the exciton–phonon systems
in quantum dots, because these systems are essentially non-adiabatic as already stated in the
Introduction. The fact that the exciton–phonon interaction in quantum dots is weak enables to
adequately describe the effect of the non-adiabaticity on phonon-assisted optical transitions.
Here we will concentrate on the intensity of phonon satellites in the optical spectra; the infl uence
of the Jahn–Teller effect on the energy spectrum of the system will be considered in section 11.4.
CH011-I046325.indd 349CH011-I046325.indd 349 6/24/2008 3:32:57 PM6/24/2008 3:32:57 PM

350 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics
Under the condition of weak exciton–phonon interaction:
η
βγ β
ω
λ
≡
〈〉max| | | |
0
1 (11.10)
contributions to the absorption coeffi cient from transitions, accompanied by a change of the
number of phonons by K, can be calculated to leading ( K th) order in the small parameter η
2
. For
the details, we refer to [11] .
The average absorption coeffi cient on the narrow frequency intervals of width Δ Ω is repre-
sented as (cf. [11] ):
α
π
δω
β
β
β
β
()
()
() ( )
()
Ω
Ω
Ω
ΩΩ
8
3
1
2
0
0
ᏺ
cn
nf K
nf
K
K
K
K
K
∞
∑∑
⎡
⎣
⎢
⎢
⎢
(()
()
δω
β
ΩΩK
K
0
1
∞
∑
⎤
⎦
⎥
⎥
⎥
.
(11.11)
Here, the coeffi cients
ff
KKββ
() ()
()
defi ned by:
fd
β
β
0
2
()
||
±
(11.12)
f
k
K
KK K
KK
kk
β
ββ
ββ
ββ ββ
δξβ β
θωθ
()
()
(| | ) (|
±
…
…
±±
∑
0
0
,,
,,
ΩΔΩΩkk
kk
K
kk
k
K
ω
ωω
ββ ββ
0
00
1
1
|)
() ( )
,
ΔΩ
ΩΩ±±
≥
∏
(11.13)
are proportional to the probabilities for the generation of an exciton in the state | β with simulta-
neous emission (absorption) of K phonons;
θ()
,
, <
x
x
x
10
00
≥
⎧
⎨
⎪
⎪
⎩
⎪
⎪
(11.14)
ΩΩΩ
ββ
β
β
′′
.
(11.15)
In Eq. (11.13), there are three kinds of terms which contain non-diagonal matrix elements
〈〉βγ β
λκi
||
in the coeffi cients ξ
K
:
1. terms with all the states
|,,|ββ
KK
〉… 〉
belonging to the same energy level. Those terms
describe the infl uence of the internal non-adiabaticity (or the proper Jahn–Teller effect) on
the optical transition probabilities;
2. terms with
|,,|ββ
KK
〉… 〉
pertaining to different energy levels; they take into account the
effects of the external non-adiabaticity (or the so-called pseudo Jahn–Teller effect);
3. terms proportional to
dd
KK
ββ
*
with β
K
⬆ β
K
; such terms describe the intermultiplet mixing
between the exciton states with the same symmetry. This mixing occurs due to the exci-
ton–phonon interaction.
When an exciton energy level is degenerate, Eq. (11.13) differs from the result of the adiabatic
theory [35, 36] , which gives for weak exciton–phonon interaction
f
d
K
K
K
β
β
λ
λ
βγ β
ω
||
!
||
2
0
2
〈〉
⎛
⎝
⎜
⎜
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
⎟
⎟
∑
,
(11.16)
the difference taking place even if one neglects the effects mentioned in points (2) and (3) above.
For example, the shape of the absorption band edge of a spherical quantum dot can be obtained
using the spherical model [37–39] for the exciton Hamiltonian (see appendix to [11] ). The coef-
fi cients
f
Kβ
()±
, which are proportional to the probabilities of generation of an exciton in the ground
CH011-I046325.indd 350CH011-I046325.indd 350 6/24/2008 3:32:58 PM6/24/2008 3:32:58 PM

Effects of the Electron–Phonon Interaction in Semiconductor Quantum Dots 351
state with emission or absorption of K phonons of non-adiabatic (or, equivalently, Jahn–Teller)
modes, are then given by:
f
f
KK
K
K
β
β
()±
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
⎛
⎝
⎜
4
3
1
2
1
2
1
2
3
2
⎜⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
(11.17)
where [ x ] denotes the integer part of x. The adequate account of the exciton interaction with
the Jahn–Teller vibrations leads to a signifi cant increase in the multiphonon transition probabili-
ties and to a more complicated dependence of these probabilities on the number of emitted or
absorbed phonons in comparison with the results of the theory by Pekar [35] and Huang-Rhys
[36] . Therefore, the multiphonon optical spectrum determined by Eq. (11.11) with Eq. (11.17) is
considerably different from the Franck–Condon progression described by Eq. (11.11), where the
f
Kβ
()±
are replaced by the
f
Kβ
given through Eq. (11.16).
11.3.2 The photoluminescence spectrum
The relaxation processes during the time interval t between the generation and the recombina-
tion of an exciton substantially infl uence the photoluminescence spectrum. Two limiting cases
are examined here: (i) the thermodynamic equilibrium photoluminescence which takes place
when τ
0
the time of the relaxation between the exciton energy levels is much smaller than the
radiative lifetime of an exciton τ and (ii) the opposite case relevant to slow relaxation, τ
0
τ .
The energy level broadening due to the fi nite values of τ
0
and τ is disregarded in [11] , i.e. the
inequalities
ττ ω
0
11
0
,
are supposed to be satisfi ed. This supposition is in agreement with
the theoretical estimations of the exciton lifetime (for example, the value τ ~ 1 ns was obtained
in [12] for CdSe quantum dots) and with the experimental observation of ultra-narrow
( 0.15 meV) luminescence lines from single quantum dots [51] . The spectral broadening of the
multiphonon photoluminescence lines will be considered below in section 11.3.4.
The spectra of equilibrium luminescence and of the slow relaxation photoluminescence were
obtained in [11] on the basis of the optical absorption spectrum analysed above.
11.3.3 Models for quantum dots
Both the quantum dots in glass and the colloidal quantum dots of small size (1 to 2 nm) are
known [12, 22–24] to have a geometrical shape close to spherical. For the samples investigated in
[12, 23] , small-angle X-ray measurements have indicated that the function ᏺ ( R ) which describes
the distribution of quantum dots over radii is close to the logarithmic standard distribution
ᏺ
ᏺ
() exp
ln( / )
tot
R
R
RR
22
0
2
π
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
⎧
⎨
⎪
⎪
⎪
⎩
⎪
⎪
⎪
⎫
⎬
⎪
⎪
⎪
⎭
⎪
⎪
⎪
,
(11.18)
the average radius of quantum dots being determined as
〈〉
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
RR
0
2
2
exp
.
(11.19)
Here R
0
and
are distribution parameters, ᏺ
tot
is the total concentration of quantum dots:
ᏺᏺ
tot
() RdR
0
∞
∫
.
In section 11.3.4, the function ᏺ ( R ) (Eq. 11.18) is used to model the distribution of quantum
dots over radii.
In the exciton–phonon interaction Hamiltonian H
int
(Eq. 11.3), the operators γ
λ
are
γγ γ
λλ λ
() ()rr
e h
(11.20)
CH011-I046325.indd 351CH011-I046325.indd 351 6/24/2008 3:32:59 PM6/24/2008 3:32:59 PM
352 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics
where r
e
and r
h
are the coordinates of an electron and a hole, respectively. In a spherical quan-
tum dot the interaction operators
γ
λ
B
()r
and
γ
λ
I
()r
describing the electron interaction, respec-
tively, with the bulk and interface phonons are expressed according to [52] by
γγυ ϑϕ
λ
() () ()
||
() () () (, )
B
nlm
B
nl
B
lm m
lm
ri Yrr≡
(11.21)
γγυ ϑϕ
λ
() () ()
||
() () () (, )
I
lm
I
l
I
lm m
lm
ri Yrr≡
(11.22)
where Y
lm
( ϑ , ϕ ) are the spherical harmonics. The radial parts of the interaction operators are
described by
υ
ω
nl
B
lln
ln l
re
R
jzrR
zjz
()
()
() (0)
(/)
(
0
0
11
εε ε∞
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
lln
)
(11.23)
υ
ω
l
I
re
R
l
ll
()
()
() (0)
()
()( )(
0
0
11
1εε ε
ε
εε∞
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
∞
∞
∞∞
⎛
⎝
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
)
r
R
l
(11.24)
where j
l
( z ) are the spherical Bessel functions, z
ln
is the n th zero of the function j
l
( z ),
0
is the
permittivity of vacuum, ε (
∞ ) and
ε()
∞
are the optical dielectric constants, respectively, of a
quantum dot and of its surrounding medium, and ε (0) is the static dielectric constant of a quan-
tum dot.
The exciton Hamiltonian for the spherical model [37–39] supplemented to account for the
electron–hole exchange interaction [53, 54] is as follows:
H
mmm
Va
e
eh
Ceh
ex
() ()
exch
()
(,)
1
229
2
3
2
1
0
2
2
0
22
0
3
pp PJ
rr
γγ
ε
⋅
δδσ() ().rr J
eh
⋅
(11.25)
Here p
e
and p
h
are the momenta of an electron and a hole, respectively, γ
1
and γ
2
are the
Luttinger parameters, P
(2)
and J
(2)
are the irreducible second-rank tensor operators of the
momentum and the spin-
3
2
angular momentum of a hole, σ and J are the spin operators of an
electron and a hole, a
0
is the lattice constant, and ε
exch
is the exchange strength constant [55] ,
which is equal to 320 meV in CdSe [54] . In a spherical quantum dot, the Coulomb electron–hole
interaction may be approximately described by the Hamiltonian [52]
V
e
P
r
rr
r
Ceh l eh
l
e
l
h
l
he
h
l
e
(, )
()
(cos ) ( )rr
2
0
0
1
4π
ϑ
εε∞
∞
∑
rr
θ
ll
eh
eh
l
l
rr
rr
R
l
1
21
1
θ()
( ) [ ( ) ( )] ( )
()
⎧
⎨
⎪
⎪
⎩
⎪
⎪
∞∞
∞
εε
εε
(() ( )∞
⎫
⎬
⎪
⎪
⎭
⎪
⎪
l 1
(11.26)
where P
l
( x ) is a Legendre polynomial of degree l and ϑ
eh
is the angle between r
e
and r
h
. The
Franck–Condon frequency for generation of an exciton in the eigenstate
|β〉
is determined by
the corresponding eigenvalue E
β
of the Hamiltonian (Eq. 11.25) as follows:
Ω
β
β
E
g
E
(11.27)
where E
g
is the energy band gap for the substance of quantum dot.
For typical [12, 22, 23] quantum dot radii, the exciton states are determined mainly by con-
fi nement while the electron–hole interaction can be treated as a perturbation. To zeroth order
in this perturbation, the hole states [39] , designated as n S
3/2
, n P
l/2
, n P
3/2
, n P
5/2
, n D
1/2
, n D
5/2
, … ,
are characterized by defi nite values of the hole angular momentum F L J (where L is the
orbital angular momentum of a hole) and the parity; n labels the solutions of the equations for
the radial components of the hole wave function [39] .
CH011-I046325.indd 352CH011-I046325.indd 352 6/24/2008 3:32:59 PM6/24/2008 3:32:59 PM
Effects of the Electron–Phonon Interaction in Semiconductor Quantum Dots 353
Under size-selective excitation, the excitation frequency Ω
exc
is chosen to lie in the absorp-
tion band tail. Thus, only the largest quantum dots are involved in the absorption processes and
excitons are generated in the lowest states. The exciton ground state (1S,1S
3/2
) and the state
(1S,1P
3/2
) separated from the ground state by an energy comparable with the phonon energy
ω
0
play the main role in the absorption and photoluminescence. (As for an electron, the energy
of its size quantization is large and only the state 1S must be taken into account.)
A detailed analysis of the lowest energy levels of an exciton as a function of the radius in a
spherical CdSe quantum dot with zinc-blende (cubic) structure and with wurtzite (hexagonal)
structure was performed in [11] . In quantum dots with both the zinc-blende structure and the
wurtzite structure, the zero-phonon generation of an exciton in the ground state and the radi-
ative recombination from this state are forbidden in the dipole approximation. Therefore, the
intensity of zero-phonon line is due to transitions from high energy levels. As a consequence, a
decrease of temperature must result in a decrease of the zero-phonon-line intensity in the equi-
librium-luminescence spectra. Such behaviour is in agreement with the experimental data [22] .
11.3.4 Numerical results and comparison with the experiment
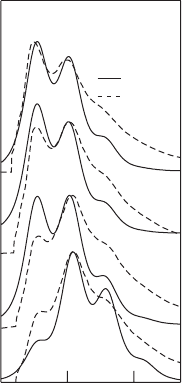
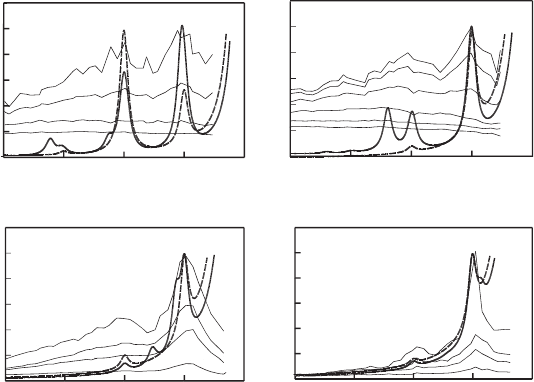
In Fig. 11.1 , the calculated fl uorescence spectra I ( Ω ) are presented together with the experimen-
tal data of [22] on colloidal CdSe quantum dots of wurtzite structure, respectively, at different
temperatures T . The following values of the parameters were used: ε ( ) 6.23, ε (0) 9.56,
m
e
0 . 1 3 m
0
, E
g
1.75 eV [33] ; γ
1
2.04, γ
2
0.58 [56] . From a comparison of the calculated
absorption spectrum (Eq. 11.11) with the experimental absorption spectrum of [22] , the value
0.06 was obtained for the parameter
of the distribution function ᏺ ( R ) (Eq. 11.18). Because
of the chaotic nature of the energy spectrum of excitons in real quantum dots (e.g. owing to the
strain or non-perfect geometrical form) and also due to a limited spectral resolution of experi-
mental equipment, photoluminescence lines described by δ -functions turn into broadened peaks.
The shape of these peaks was modelled by Lorentzians of fi nite width Γ 1 5 m e V.
Figure 11.1 Fluorescence spectra of CdSe quantum dots with wurtzite structure at various temperatures. Solid
lines were calculated by using the non-adiabatic approach, dashed lines represent the experimental data of [22] .
Different lines are shifted along the vertical axis for clarity. (Reprinted with permission after [11] . © 1998 by the
American Physical Society.)
Wavelength (nm)
I (a.u.)
10 K
5K
3K
1.75 K
<R> 1.2 nm
Theory
Exper.
498 508 518
Colloidal CdSe quantum dots
(wurtzite)
It is worthwhile noting that in CdSe quantum dots of wurtzite structure, the magnitude of
the (1S, 1S
3/2
)-level splitting by the crystal fi eld for a wide range of radii R is close to the optical
CH011-I046325.indd 353CH011-I046325.indd 353 6/24/2008 3:33:00 PM6/24/2008 3:33:00 PM
354 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics
phonon energy
–
h ω
0
25 meV. Therefore, the observed peak of the equilibrium photolumines-
cence in the spectral region Ω Ω
exc
K ω
0
is caused not only by K -phonon processes (with
probability ~ η
2
K
) , but also by (K 1)-phonon processes (with probability ~ η
2(
K
1)
). The latter
processes are related to generation of an exciton in the upper group of states (resulting from the
splitting of the (1S, 1S
3/2
) level) and the subsequent radiationless relaxation to the lower group
of states. This feature of the energy spectrum of the exciton–phonon system in quantum dots, in
combination with the pseudo Jahn–Teller effect, leads to a substantial difference of the observed
spectrum of multiphonon photoluminescence from the Franck–Condon progression, as shown
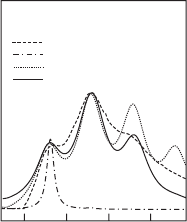
in Fig. 11.2 . It is important to note that a straightforward calculation in the framework of the
adiabatic theory leads to intensities of the phonon satellites, which do not fi t the observed spec-
trum well for any value of the Huang-Rhys parameter.
Figure 11.2 Fluorescence spectra of CdSe quantum dots with wurtzite structure at the average radius
R 1.25 nm. Dashed line represents the experimental data of [22] , dot–dashed line displays a Franck–Condon
progression with the Huang-Rhys parameter S 0.06 calculated in [33] , dotted line shows another Franck–Condon
progression with the Huang-Rhys parameter S 1.7, which is obtained by fi tting the ratio of one-phonon and zero-
phonon peak heights to the experimental value, solid line results from the non-adiabatic approach. (Reprinted with
permission after [11] . © 1998 by the American Physical Society.)
0
I (a.u.)
ΩΩ
exc
(cm
1
)
T 1.75 K
Experiment
FC (non-fitted)
FC (fitted)
Present theory
Colloidal CdSe quantum dots
(wurtzite)
200 400 600
In Fig. 11.3 , the photoluminescence spectra I ( Ω ) calculated for a zinc-blende-type crystal lattice
are given and compared with the experimental data of [12] on photoluminescence of CdSe quan-
tum dots in borosilicate glass. The parameter values m
e
0.11m
0
, E
g
1.9 eV [57] were used for
the zinc-blende lattice. The theoretical curves are calculated for the case of the equilibrium photo-
luminescence [11]. The shape of photoluminescence peaks was modelled by Lorentzians of width
Γ 2 meV relevant to the limited spectral resolution of measurements (better than 5 meV in
[12] ). From a comparison of the calculated absorption spectra (Eq. 11.11) with the experimental
absorption spectra of [12] , the values 0.15, 0.18, and 0.20 of the parameter
were obtained for
average radii R equal to 1.4 nm, 1.8 nm, and 2.7 nm, respectively. The experimental photolumi-
nescence spectra at each value of the average radius R refer to different time intervals between
the pumping pulse (of duration 2.5 ps) and the measurement, the upper curve corresponding
to the time interval equal to 0. According to [12] the decay of fast photoluminescence compo-
nents is caused by trapping of holes onto deep (binding energy ~200 meV) local surface levels.
Depending on the average radius of the quantum dots, the decay time varies from some tens to
some hundreds of ps. The separation between neighbouring energy levels of a non-trapped exci-
ton is smaller than the depth of local levels. Therefore, the relaxation in the system of “ interior ”
exciton states, which were discussed in the Introduction, can be expected to proceed more quickly
than the hole trapping. This supposition is in agreement with the behaviour of fast photolumines-
cence components under the excitation by light whose frequency lies near the absorption band
maximum (no size-selective excitation). In this case a signifi cant shift of the photoluminescence
intensity maximum from the excitation frequency appears 10 ps after the excitation pulse [12] .
Therefore, it is the limiting case of the equilibrium photoluminescence rather than the opposite
case of slow relaxation which seems to be relevant to the experimental data of [12] .
CH011-I046325.indd 354CH011-I046325.indd 354 6/24/2008 3:33:00 PM6/24/2008 3:33:00 PM
Effects of the Electron–Phonon Interaction in Semiconductor Quantum Dots 355
The quantum dots in glass are characterized by broad distribution functions over the radii
R [31, 32] . Therefore, even under size-selective excitation some excitons are generated in states
with relatively high energy (fi rst of all, in the states (1S,2S
3/2
), (1S,1P
5/2
), (1S,1D
5
/
2
) whose ener-
gies differ slightly from each other). In the case of the equilibrium photoluminescence, the radia-
tionless relaxation of such excitons into the lowest states (1S,1S
3
/2
) leads to the appearance of
zero-phonon luminescence peaks shifted from Ω
exc
to lower frequencies. These peaks hide the
phonon satellites (see Fig. 11.3c ). This effect becomes less pronounced when the excitation is
deeper in the absorption band tail (see Fig. 11.3d ).
The observed photoluminescence spectra in InAs/GaAs self-assembled quantum dots were
attributed to the large effi ciency of the Fröhlich interaction between a strain-induced polarized
exciton and the longitudinal optical phonons [58, 59] . The theoretical analysis in [60] has shown
that the phonon-assisted photoluminescence due to the intraband transitions of an electron
between the size-quantized states in self-assembled quantum dots modelled as rectangular paral-
lelepiped InAs quantum dots ( “ quantum bricks ” ) embedded into GaAs is strongly enhanced by
two processes. First, the effi ciency of the electron–phonon interaction in an individual quantum
dot is enhanced in suffi ciently small dots. Second, the ratio between the intensities of the zero-
phonon line and the one-phonon line in the photoluminescence spectrum is effi ciently controlled
by both the shape and the size distribution of those quantum dots. The relative enhancement of
the phonon-assisted photoluminescence for narrow size distributions is demonstrated to provide
an effective method to characterize vibrational states in single InAs/GaAs quantum dots [60] .
Recently, the size, shape, and composition of self-assembled InAs/GaAs and InAs/AlAs quantum
dots have been accurately determined by cross-sectional scanning tunnelling microscopy [61] .
Those structural results provide a basis for the further, more detailed, analysis of the phonon-
assisted transitions in the photoluminescence spectra of self-assembled quantum dots.
Figure 11.3 Photoluminescence spectra of CdSe quantum dots embedded in borosilicate glass at different
average radii and excitation energies: R 1.4 nm, Ω
exc
2.50 eV (a), R 1.8 nm, Ω
exc
2.28 eV (b),
R 2.7 nm, Ω
exc
2.00 eV (c), R 2 . 7 n m , Ω
exc
1.95 eV (d). Thin solid lines represent the families
of experimental time-resolved photoluminescence spectra of [12] measured at different time intervals between the
pumping pulse and the measurement, the upper curve corresponding to the time interval equal to 0. Theoretical
results are displayed for the equilibrium–photoluminescence spectra (heavy solid lines) and for the photoluminescence
spectra in the case of slow relaxation of the exciton energy (dashed lines). (Reprinted with permission after [11] . ©
1998 by the American Physical Society.)
100 75 50 25 0
CdSe quantum dots in borosilicate
glass (zinc-blende)
I (a.u.)
Ω
exc
2.5 eV
R 1.4 nm
ΩΩ
exc
(meV)
CdSe quantum dots in borosilicate
glass (zinc-blende)
I (a.u.)
Ω
exc
2.0 eV
R 2.7 nm
100 75 50 25 0
ΩΩ
exc
(meV)
CdSe quantum dots in borosilicate
glass (zinc-blende)
I (a.u.)
Ω
exc
1.95 eV
R 2.7 nm
100 75 50 25 0
ΩΩ
exc
(meV)
100 75 50 25 0
CdSe quantum dots in borosilicate
glass (zinc-blende)
I (a.u.)
Ω
exc
2.28 eV
R 1.8 nm
ΩΩ
exc
(meV)
(a) (b)
(c)
(d)
CH011-I046325.indd 355CH011-I046325.indd 355 6/24/2008 3:33:00 PM6/24/2008 3:33:00 PM

356 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics
11.4 Non-adiabaticity of the exciton–phonon systems in stacked quantum dots
Recently, stacked quantum dots have received increasing attention (see, e.g., [62–68] ) due to
the possibility of fi nely controlling their energy spectra. This makes stacked quantum dots very
promising for future nanodevices [67, 68] . In the present work, the non-adiabatic approach is
applied to stacked InAs/GaAs quantum dots, which reveal a richer structure of phonon and exci-
ton spectra in comparison to those for a single quantum dot. Namely, in the stacked quantum
dots, as distinct from a single quantum dot, the exciton (phonon) spectra contain groups of states
(modes) of different symmetry with close energies (frequencies). Owing to small energy differ-
ences between the exciton energy levels within these groups, additional channels for non-adi-
abatic transitions as compared to a single quantum dot open in stacked quantum dots. Therefore,
optical absorption spectra of stacked quantum dots contain more phonon satellites than those of
a single quantum dot. These features of the optical absorption can be experimentally revealed,
e.g., in the photoluminescence excitation measurements.
In order to model coupled self-assembled InAs/GaAs quantum dots, we consider a stack of N
parallelepiped-shaped quantum dots with heights l
nz
( n 2, 4, … , 2 N ) and with the interdot dis-
tances l
nz
( n 3,5, … ,2 N 1) along the z -axis. Within the present approach, the lateral sizes
of each quantum dot in a stack are supposed to be much larger than its size l
nz
along the growth
axis. The stack is a system of (2 N 1) layers ( n 1 , … , 2 N 1) with parameters:
lnN
lnN
l
nz n
nz n
nz
,,,,
,,,,
εε
εε
InAs
GaAs
for
for
24 2
35 2 1
…
…
→∞∞
⎧
⎨
⎪
⎪
⎪
⎪
⎩
⎪
⎪
⎪
⎪
,,.εε
n
nN
GaAs
for 1 2 1
The bulk-like optical-phonon frequencies in InAs and GaAs layers of the stacked InAs/GaAs
quantum dots coincide with the LO-phonon frequencies in InAs and GaAs, respectively. The
interface frequencies belong to the stacked quantum dots as a whole and satisfy the dispersion
equation:
det|| ( )|| ( , , )aknN
kn
ω 012 … (11.28)
where a
kn
( ω ) is the dynamic matrix of the interface vibrations with the matrix elements:
aqlql
aa
nn n n n n
nn n n
() () ()
()
,,
ωεω εω
ω
coth coth
11
11
(() ()/sinhωεω
nn
ql
⎧
⎨
⎪
⎪
⎪
⎩
⎪
⎪
⎪
(11.29)
and all other matrix elements are equal to zero.
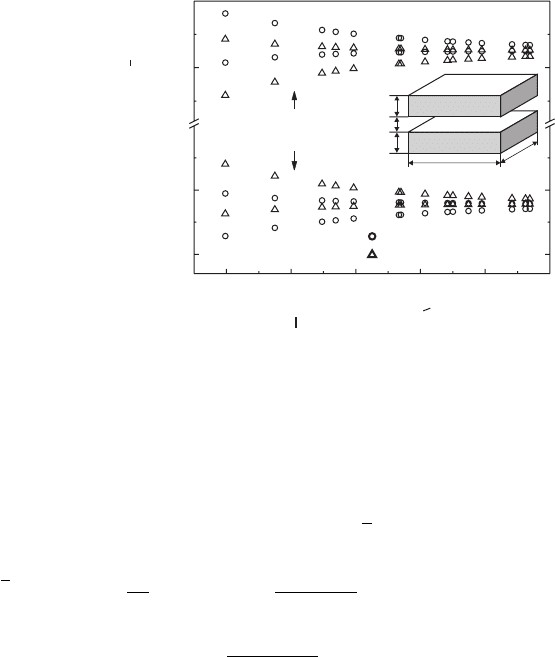
In Fig. 11.4 , typical interface-phonon spectra are represented for stacked InAs/GaAs quan-
tum dots formed by two InAs parallelepipeds. The frequencies are plotted as a function of the
in-plane wave number
q
||
, which takes discrete values due to the quantization of the phonons in the
xy -plane. In a stack of N quantum dots, each interface-phonon frequency of a single quantum
dot splits into N branches. The splitting of the interface-phonon frequencies is due to the electro-
static interaction between the optical polar vibrations of the different quantum dots.
These features of the optical-phonon spectrum of stacked quantum dots are manifested in
their optical properties. We calculate the optical absorption spectrum of polaronic excitons in
stacked quantum dots starting from the Kubo formula. Within the non-adiabatic approach [11]
the following expression results for the linear coeffi cient of the optical absorption (Eq. 11.7) by
the exciton–phonon system in a quantum-dot structure:
αββ
ββ
ββ
β
() ()
*
,
()
Ω
ΩΩ
∝〈〉
∑
∫
∞
Re | |
i
dd dte Ut
it
0
0
(11.30)
where Ω is the frequency of the incident light and d
β
and Ω
β
are, respectively, the electric dipole
matrix element and the Franck–Condon frequency of a transition between the exciton vacuum
CH011-I046325.indd 356CH011-I046325.indd 356 6/24/2008 3:33:01 PM6/24/2008 3:33:01 PM
Effects of the Electron–Phonon Interaction in Semiconductor Quantum Dots 357
state and the one-exciton state
|β〉
. Exciton states in stacked InAs/GaAs quantum dots are deter-
mined using an exact diagonalization of the exciton Hamiltonian with simple parabolic valence
and conduction bands within a fi nite-dimensional basis of the electron–hole states. The evolu-
tion operator averaged over the phonon ensemble,
Ut()
, Eq. (11.8), can be represented as:
U t dt dt
y
t
t
tt
()
()
T exp
e
i
1
1
2
1
0
2
0
1
12
∫∫
∑
⎡
⎣
⎢
⎢
⎧
⎨
⎪
⎪
⎪
⎩
⎪
⎪
⎪
ω
λ
λ
λ
γγ γγ
λ
λ
λ
ω
λ
λ
λ
λ
() () () ()
()
tt
y
y
tt
tt
12 12
12
1
††
e
i
⎤
⎦
⎥
⎥
⎫
⎬
⎪
⎪
⎭
⎪
⎪
..
(11.31)
In Eq. (11.31), T is the time ordering operator, the index λ labels the phonon modes specifi c for
the quantum-dot structure under consideration, ω
λ
are phonon frequencies, γ
λ
( t ) are the exci-
ton–phonon interaction amplitudes in the interaction representation, and y
λ
exp(
–
h ω
λ
/ k
B
T ).
Within the adiabatic approximation, which has been widely used to calculate the optical spectra
of quantum dots, non-diagonal matrix elements of the exciton–phonon interaction are neglected
when calculating α ( Ω ) as given by Eq. (11.30) with Eq. (11.31). In the adiabatic approach
[69, 70] one supposes that (i) both the initial and the fi nal states of a quantum transition
are non-degenerate and (ii) the energy differences between the exciton states are much larger than
the phonon energies. It has been shown in [11, 15–17] that these conditions are often violated for
optical transitions in small quantum dots, which have sizes less than the bulk exciton radius.
In general, the effi ciency of the exciton–phonon interaction, as revealed through the optical
spectra of quantum dots, depends on their geometric and material parameters in a complicated
way. On the one hand, an increase of the confi nement strength, which results from a decrease
of the quantum-dot size or from a rise of potential barriers at the quantum-dot boundary,
enhances the effi ciency of the interaction with phonons for a solitary electron (a solitary hole). On
the other hand, strengthening confi nement leads to an increasing overlap between the electron
and hole wave functions so that the net charge of the electron–hole pair and, correspondingly, the
effi ciency of the exciton–phonon interaction tend to decrease. As a result, the effi ciency of the
exciton–phonon interaction appears a non-monotonous function of the confi nement strength
even within the adiabatic approximation (see, e.g., [71] ). Effects of non-adiabaticity, related to
phonon-assisted transitions between different exciton states, drastically enhance intensities of pho-
non satellites in the optical spectra of quantum dots and, at the same time, make these intensities
dependent on a vast set of parameters, which characterize the phonon-induced coupling between
1.20
1.15
0.95
3nm
2nm
3nm
15 nm
20 nm
InAs
InAs
GaAs
0.90
4681012
GaAs-like phonons
InAs-like phonons
14
Symmetric modes
Antisymmetric modes
Phonon frequencies (in units hv
0
(InAs)
)
(in units (mv
0
(InAs)
/ h)
1/2
)
q
Figure 11.4 Interface-phonon frequencies for two stacked parallelepiped-shaped InAs/GaAs quantum dots.
ω
0
()InAs
is the frequency of LO phonons in InAs at the centre of the Brillouin zone. (Reprinted with permission from
[20] . © 2004 by the American Physical Society.)
CH011-I046325.indd 357CH011-I046325.indd 357 6/24/2008 3:33:02 PM6/24/2008 3:33:02 PM
358 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics
all the involved exciton states. Thus, side by side with the Fröhlich coupling constant α , energy
spacing between exciton states strongly infl uences the aforementioned intensities. When the
above spacing is comparable with the LO-phonon energy, non-adiabatic effects can strongly affect
the optical spectra even in quantum dots of III–V semiconductor compounds, where the Fröhlich
coupling constant is signifi cantly smaller ( α 0.0504 for InAs) than the values α ~ 0.5 typical
for II–VI semiconductors considered in [11, 16] .
In section 11.3, we described a method to calculate the absorption spectrum given by Eqs
(11.30) and (11.31) taking into account the effect of non-adiabaticity on the probabilities of
phonon-assisted optical transitions. The key step is the calculation of the matrix elements of the
evolution operator
〈〉ββ||Ut()
. In order to describe the effect of non-adiabaticity both on the
intensities and on the positions of the absorption peaks, a diagrammatic approach can be used.
When calculating these matrix elements we take into account that in a quantum dot, due to the
absence of momentum conservation, the product
〈〉〈〉βγ β β γ β
λλ1223
|| ||
*
can be non-zero for
β
1
⬆ β
3
, as distinct from the bulk case. Consequently, the evolution operator is in general non-
diagonal in the basis of one-exciton wave functions | β . For the absorption coeffi cient we obtain:
α
ββ
β
ββ
ββ
ββ β
() ( )
()
*
,
()
ΩΩ
Ω
∝
∑∑
Im | | i Im
i
dG dd
QQ
2
1
0
0
ββ
()
()
2
0Ω i
⎡
⎣
⎢
⎤
⎦
⎥
(11.32)
where:
GC Sj
j
j
j
β
β
βλβλ
λ
λλ
λ
β
λ
λ
ωω()
{}
()
{}
{}
,
()
ΩΩΩ
ΣΩ
∞
∞
∑∑∑
⎡
⎣
⎢
1
λλλ
λ
β
λλ
λ
ωω
∑∑
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
⎟
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
⎟
⎤
⎦
⎥
⎥
⎥
ΣΩ
()2
1
j
(11.33)
CnS
j
kT
IS
j
j
B
j
{}
()
() ( )
λ
λ
λ
β
λλβ
λλ
λ
λ
ω
121
2
exp
||
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
∏
ββ
λ
ω
2
2
1
sinh
kT
B
⎛
⎝
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
⎛
⎝
⎜
⎜
⎜
⎜
⎜
⎜
⎞
⎠
⎟
⎟
⎟
⎟
⎟
⎟
⎟
(11.34)
I
n
( x ) is a modifi ed Bessel function of the fi rst kind and S
λ β
is the Huang-Rhys parameter, which is
related to the interaction of the exciton in the state β with phonons of the λ th mode:
S
λβ
λ
λ
βγ β
ω
〈〉||
2
.
(11.35)
The self-energy terms
ΣΩ
β
()
()
1
and
ΣΩ
β
()
()
2
in Eq. (11.33) are obtained by summing diagrams,
which describe one- and two-phonon non-adiabatic contributions:
ΣΩ Ω
β
ββ
λβ
λ
λββββ
ω
()
,
()
() ( )
1
1
1
1
11
FjM
j
j
∑∑
(11.36)
and
ΣΩ Ω
Ω
β
ββ λ
βββλλ
ββ λ
ω
ω
()
,,,,
() ( )
(
2
1
1
2
11
1231212
3
Fj
Fj
jj
∑∑∑
222 1 2
1123
1
2123
2
12
)( )
() ()
Fjj
MM F
jj
ββ λ λ
λββββ λββββ
ββ
ωωΩ
⎡
⎣
⎢
22
1112
1
2233
2
2
1
()
()
() ()
Ω
MM
jj
λββββ λββββ
ββ
δ
⎤
⎦
⎥
(11.37)
CH011-I046325.indd 358CH011-I046325.indd 358 6/24/2008 3:33:02 PM6/24/2008 3:33:02 PM
