Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.

q-Analogues of Some Multivariable Biorthogonal Polynomials

Both Pn(x;q) and Pm(x;q) are Laurent polynomials in the variables

qizi

. . .

qiXp. Note that if we divide Pn(x; q) by (1

q)3N

and re-

place its parameters al,.

. .

ap,

bl,

. . .

bp,

d, respectively, by qal,. . .

qap, qbl, . .

qbp, qc, qd, and then let q

we obtain Pn(x) as

limit

case. Similarly, we see that Pm(x) is limit case of P~(x; q). In Sec-

tion

we shall do the integration and in Section

prove the following

q-analogue of (1.10)

j..

. /Pn(x;qlh(x;ql w(p)(x.q)

dok

0, if

-X

k=l

(1.22)

where w(p) (x; q) is given by (1.15)) and

when

M, with

and

0, and

is as defined in

(3.7).

Discrete multivariable extensions of the Racah polynomials were con-

sidered in (Tratnik, 1991b) as well

in (van Diejen and Stokman, 1998)

and in (Gustafson, 1990). For other related works see, for instance, (Gra-

novskiy and Zhedanov, 1992)) (Koelink and Van der Jeugt, l998), (Trat-

nik, 1989a)) (Tratnik, 1991b). We have found q-extensions of Tratnik's

systems of multivariable Racah and Wilson polynomials, complete with

their orthogonality relations, see this Proceedings (Gasper and Rahman,

1990b) for our multivariable extension of the Askey-Wilson polynomials.

192

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

However, there seems to be at least one more extension that, to our

knowledge, has not yet been investigated. The seed of this extension lies

in Rosengren's (Rosengren, 2001) multivariable extension of the q-Hahn

polynomials as well as in Rahman's (Rahman, 1981) 2-variable discrete

biorthogonal system. In Sections

and

we shall prove the following

2-variable extension of the q-Racah polynomial orthogonality (Gasper

and Rahman, 1990a, (7.2.18)):

where 0

m, n, m', n'

q-Analogues of Some Multivariable Biorthogonal Polynomials

and the weight function is

The normalization constant in (1.24) is given by

Notice that both F,,,(x,

and G,,,(x, y) are Laurent polynomials in

the variables qx and qy, and G,,,(x, y) is a polynomial of (total) degree

in the variables q-"

yy'qx-N-l

/ac and q-Y

c~Y-~.

We wish to make the observation that the summation in (1.24) is over

the square of length N, although the vanishing of the weight function

above the main diagonal, because of the factor (q-N;

q)x+y

in the nu-

merator, makes it effectively over the triangle

very

innocuous observation but it will help simplify the calculations some-

what as we shall see in Section

It seems reasonable to expect that there is a multivariable extension

of (1.24), but we were unable to find it, mainly because an extension of

the q-shifted factorials of the type

(a;

q)x-y doesn't appear too obvious

to us.

Calculation of

W(P)

(q)

The key to the proof of (1.17) is to observe that by periodicity we can

change 81,82,.

. .

to, say,

82,.

(so that

02),

with

the limits of integration unchanged. So the total weight transforms to

194

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

where

However, this integral matches exactly with the Askey-Roy integral

(Gasper and Rahman, 1990a, (4.11.1))) provided we assume that

max (\all,

lbll,

la21, Ib21)

(with, of course, Iql

1). By (Gasper and

Rahman, 1990a, (4.11.1)), it then follows that

Substitution of (2.3) into (2.1) makes it clear that the integration over

presents exactly the same situation, and so does the remaining integra-

tions up to and including

9,.

Finally, one is left with an Askey-Wilson

integral over

(p)

(q)

k=2

(4; 4)Z1

(akbk; q),

k=l

q-Analogues of Some Multivariable Biorthogonal Polynomials

195

by (Gasper and Rahman, 1990a, (6.1.1)), which completes the proof of

(1.17).

Computation

the

integral in

(1.22)

We shall carry out the integrations in (1.22) in much the same way

as we did in the previous section. We transform the integration variables

01,.

. .

,Op

to 02,.

. .

,Op and O as before; then we isolate the

82-integral by observing that the factors (alei(Q-03)-ie2

;

(a2eaZ

;

;)

(bl

ei(e3-e)+ie2

;

(b2e-ih; q)

ei~z(ji+kz)+i~z(Q3-Q)+~~iQ3

can be glued

on to the integrand of ~(p)(q), to get

which via (Gasper and Rahman, 1990a, (4.11.1)) equals, on a bit of

simplification,

Since O3

04,

we may now isolate the 03-integral in exactly the

same way, carry out a similar integration, simplify, and obtain

196

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

A clear pattern is now emerging. The 0, integral is

jikz+(ji+jz)k3+...+(jl+...+j

p-2)

kp-l

k3+-+kp--1

. .

(3.3)

The expression in

[

]

above can, once again, be computed by use of

(Gasper and Rahman, 1990a, (4.1

.I)), and simplified to

eie

-i~

(

$-*

(L.&

qibp&?

;

npP e

,AB~J+K;~)

apbpqjp+kp, AqJeiQ

BqKe-iQ

apbpq J+K-jp-kp; q

Since, by repeated application of (1.16) we get APp/ap

Pbl/B, the

Q-integral simply becomes the Askey-Wilson integral

~(ABC~

qJ+K;

q),

(q, cd, ABqJ+K, AcqJ, AdqJ, BcqK, BdqK; q),

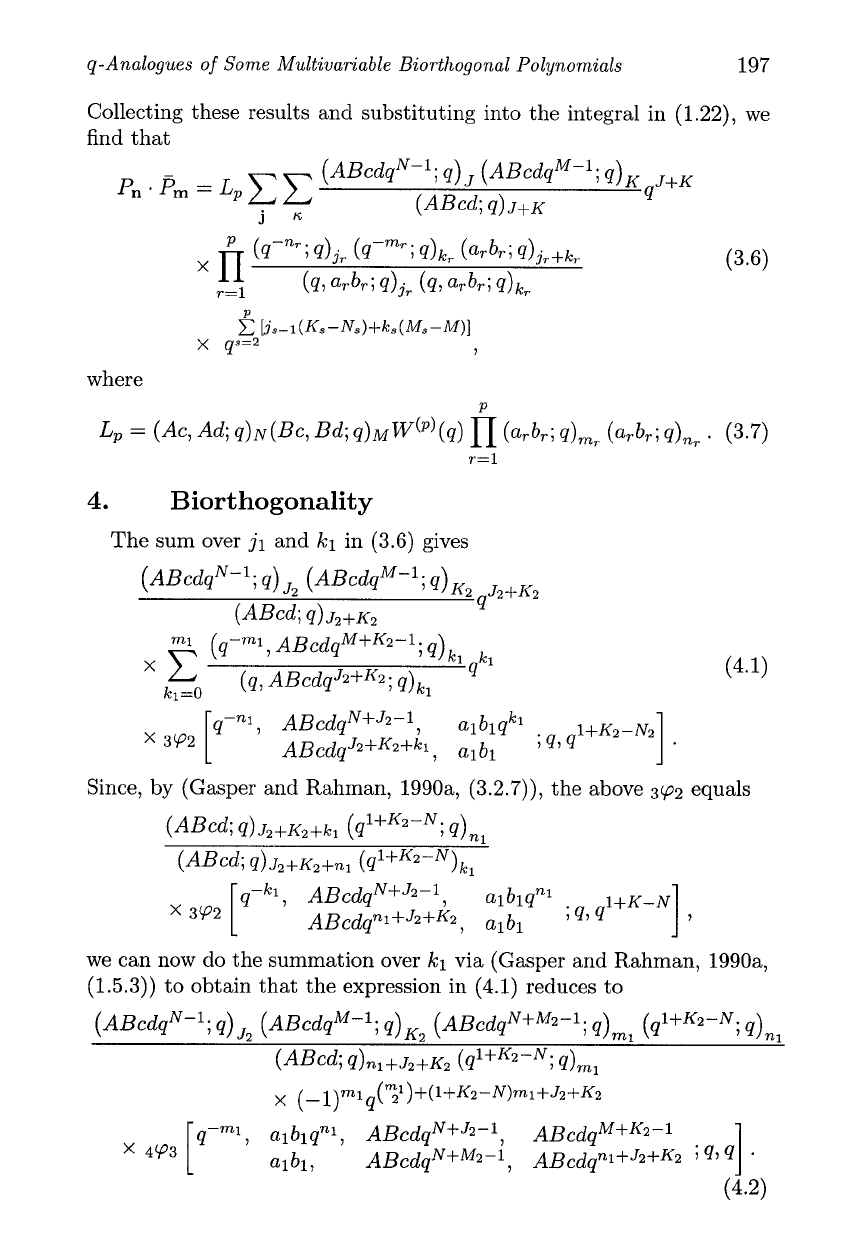
q-Analogues of Some Multivariable Biorthogonal Polynomials

197

Collecting these results and substituting into the integral in (1.22), we

find that

P,.,.%=

L,CC

(ABcdqN-l; q)

(ABcd~M-l;

J+K

(

ABcd; q)

J+K

Biort hogonality

The sum over

and

in (3.6) gives

Since, by (Gasper and Rahman, 1990a, (3.2.7)), the above

3(P2

equals

we can now do the summation over

Icl

via (Gasper and Rahman, 1990a,

(1.5.3)) to obtain that the expression in (4.1) reduces to

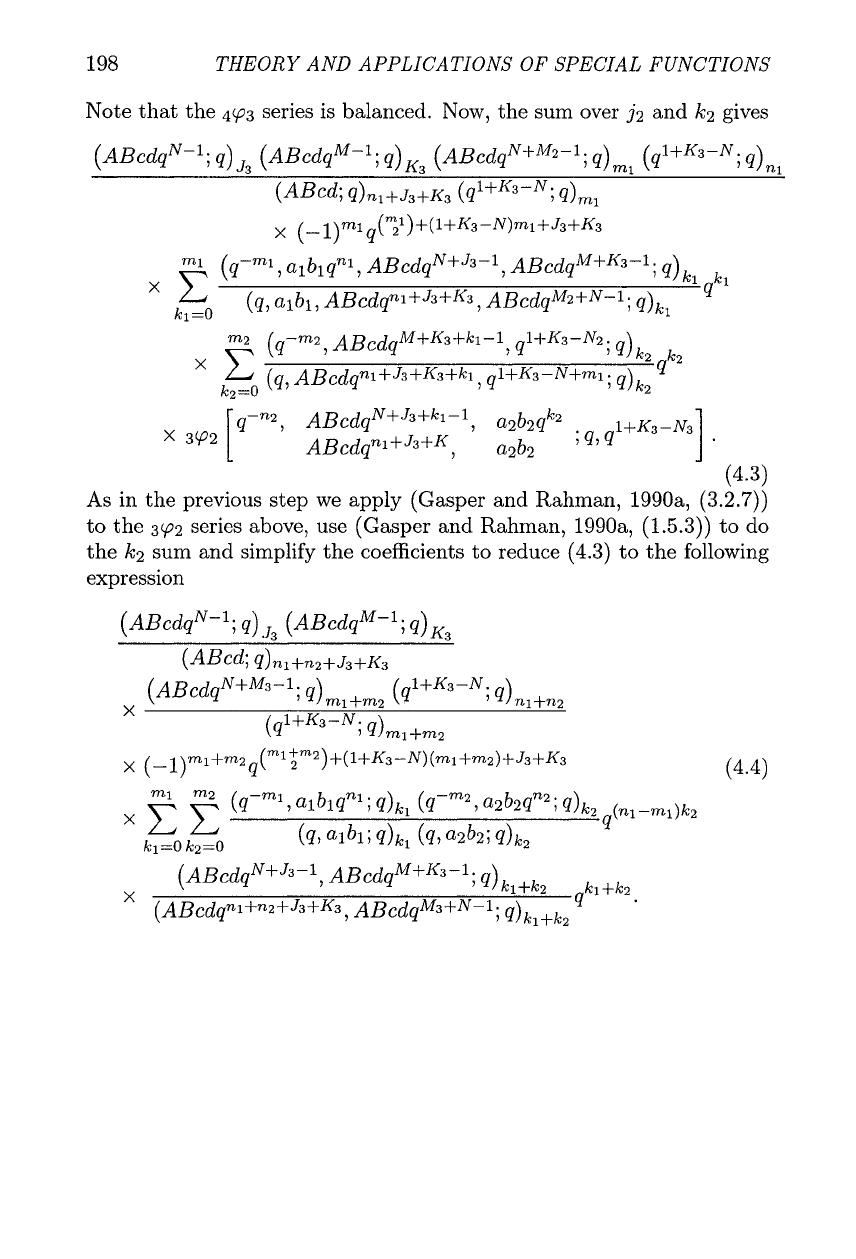
198

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Note that the

493

series is balanced. Now, the sum over

and

gives

(4.3)

As in the previous step we apply (Gasper and Rahman, 1990a, (3.2.7))

to the

392

series above, use (Gasper and Rahman, 1990a, (1.5.3)) to do

the

sum and simplify the coefficients to reduce (4.3) to the following

expression

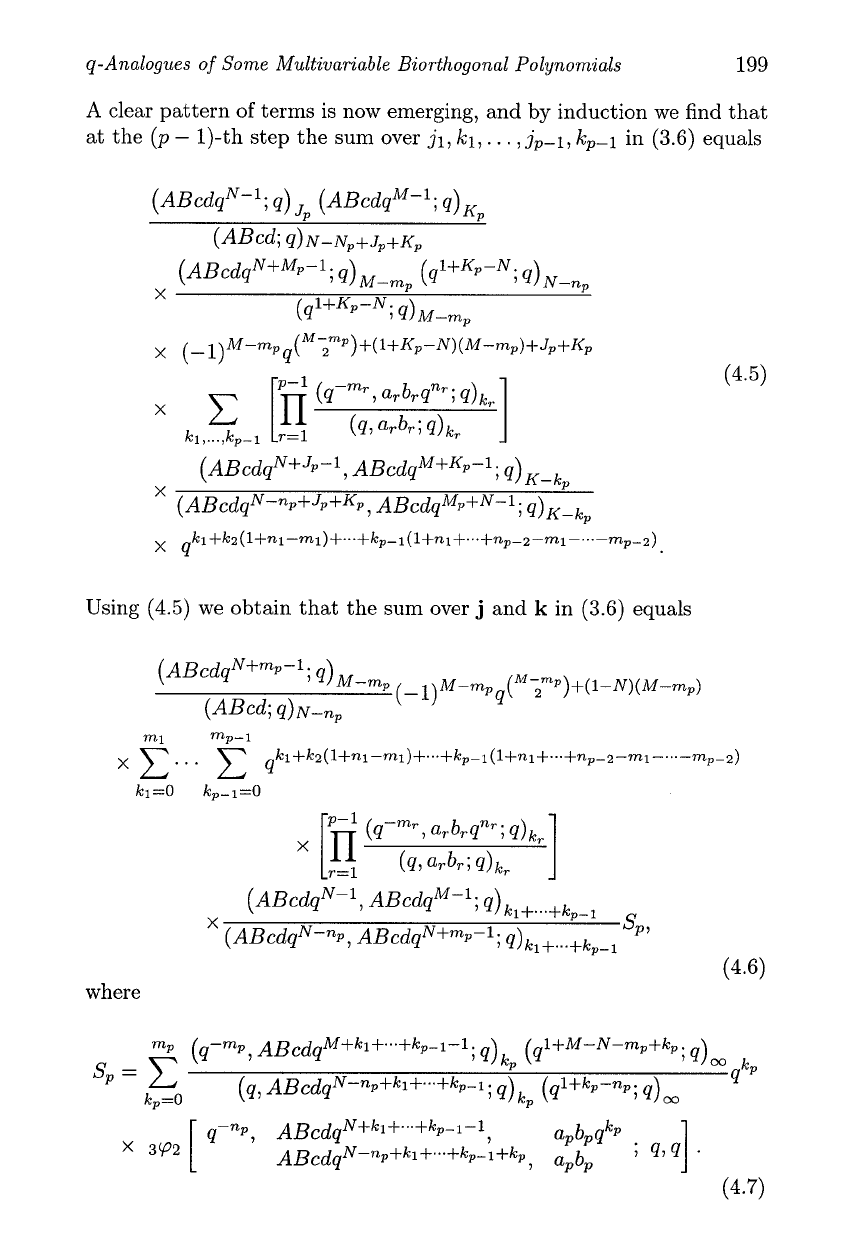
q-Analogues of Some Multivariable Biorthogonal Polynomials

199

clear pattern of terms is now emerging, and by induction we find that

at the

1)-th step the sum over

jl,

kl, . . .

jp-1,

kp-l in (3.6) equals

Using (4.5) we obtain that the sum over

and

in (3.6) equals

where

(4.6)

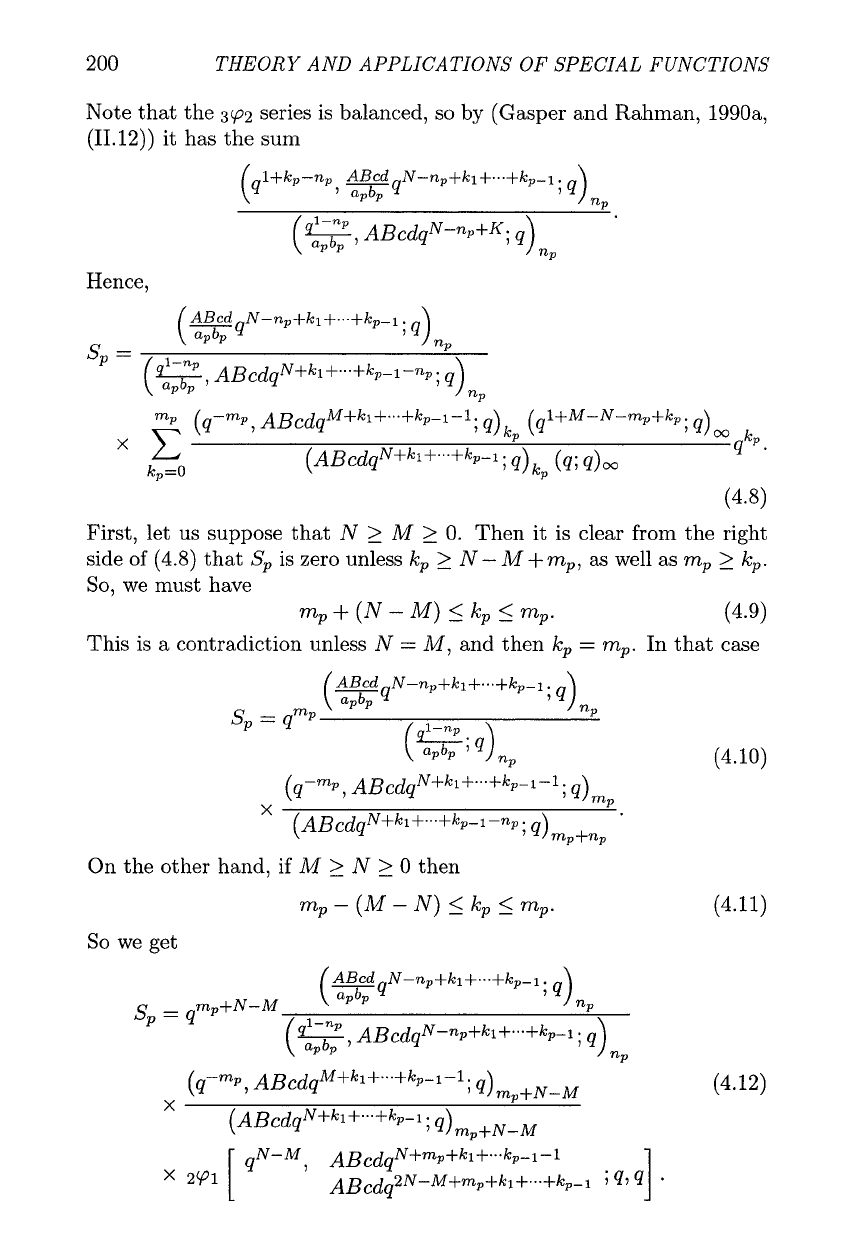
200

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Note that the

3992

series is balanced, so

(Gasper and Rahman, 1990a,

(11.12)) it has the sum

Hence,

(4.8)

First, let us suppose that N

0. Then it is clear from the right

side of

(4.8)

that

is zero unless kp

mp, as well as mp

kp.

So, we must have

mp+(N-M)SkpImp. (4.9)

This is a contradiction unless N

M, and then kp

mp. In that case

(q-mp

~~~d~N+kl+-+k~-l-l.

rnP

(~~~d~N+kl+-.+k~-l -np

q)mp+np

On the other hand, if M

then

mp-(M-N)<kp<mp.

So we get

ABcd N-np+kl+...+kp-l.

qm~+N-M

(xq

(5,

ABcdqN-np+ki+...+kp-1

;