Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.

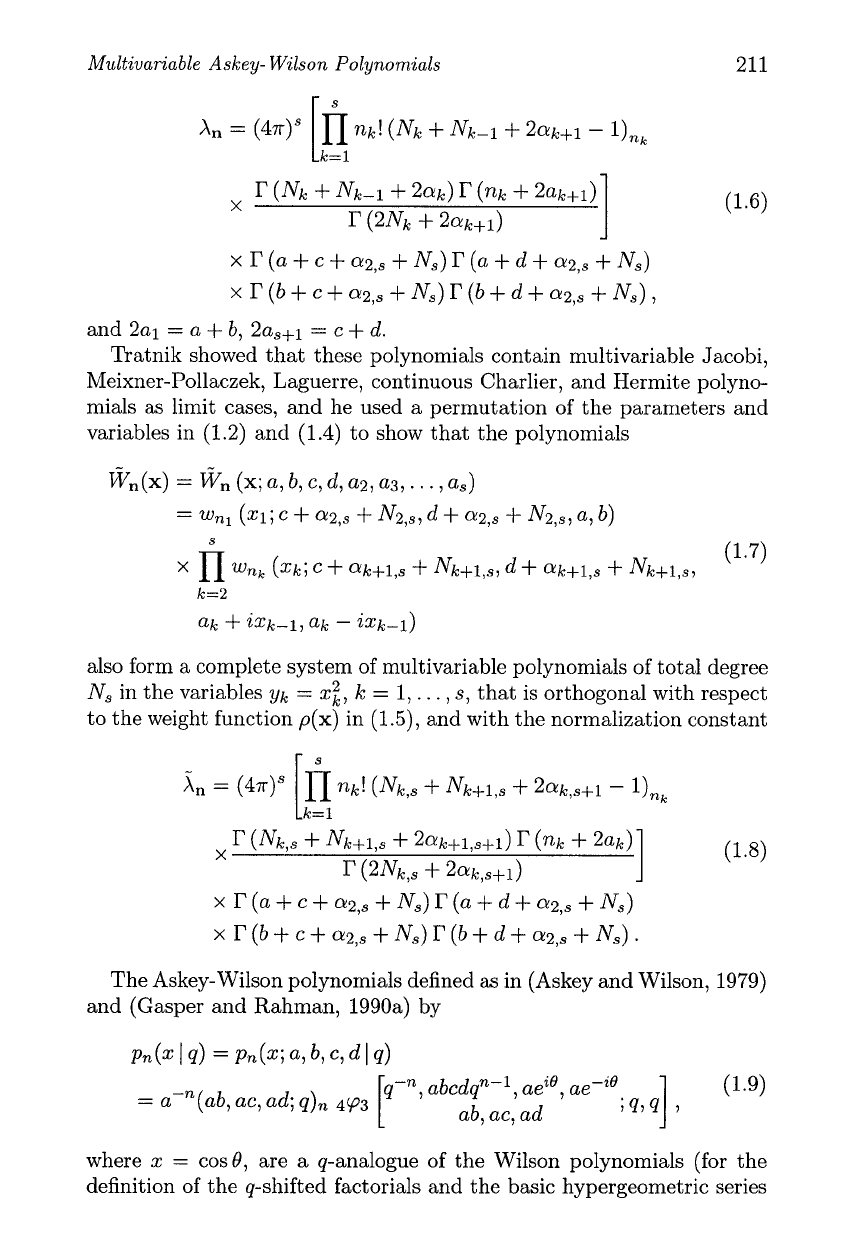
Multivariable Askey- Wilson Polynomials
211
An
=
(4~)~ [Gnk! (Nk
+
N~-I+
2W+l
-
X
r
(Nk
+
Nk-1
+
24 (nk
+
2ak+l)
r
(2Nk
+
2ak+l)
I
(1.6)
x
I?(a+~+az,~+N~)r(a+d+aa,,+N~)
x
I?
(b
+
c
+
aa,,
+
Ns)
(b
+
d
+
as,,
+
Ns)
,
and 2al
=
a
+
b,
2aS+l
=
c
+
d.
Tratnik showed that these polynomials contain multivariable Jacobi,
Meixner-Pollaczek, Laguerre, continuous Charlier, and Hermite polyno-
mials as limit cases, and he used a permutation of the parameters and
variables in (1.2) and (1.4) to show that the polynomials
w~(x)
=
Wn
(x;
a, b,
C,
dl aa, as,.
. .
,as)
=
wnl
(XI;
c
+
a2,~
+
N2,s, d
+
az,~
+
a,
b)
also form a complete system of multivariable polynomials of total degree
Ns in the variables yk
=
x:,
Ic
=
1,
.
.
.
,
s,
that is orthogonal with respect
to the weight function p(x) in (1.5), and with the normalization constant
The Askey-Wilson polynomials defined as in (Askey and Wilson, 1979)
and (Gasper and Rahman, 1990a) by
[I-",
abcdqn-I
,
aeie7 ~e-~
=
~-~(ab, ac, ad; q),
493
;414
2
I
(1.9)
ab, ac, ad
where x
=
cos8, are a q-analogue of the Wilson polynomials (for the
definition of the q-shifted factorials and the basic hypergeometric series

212
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
493
see (Gasper and Rahman, 1990a). These polynomials satisfy the
orthogonality relation
and
An
(q)
=
An
(a, b, c1 d
I
q)
- -
2r(abcd; q),
(q, ab, ac, ad, bc, bd, cd; q),
(q, ab, ac, ad, bc, bd, cd; q),
(1
-
abcdq-l)
X
(abcdq-l; q), (1
-
abcdq2,-l)
In this paper we extend Tratnik's systems of multivariable Wilson
polynomials to systems of multivariable Askey-Wilson polynomials and
consider their special cases. Some q-extensions of Tratnik's (Tratnik,
1989) multivariable biorthogonal generalization of the Wilson polyno-
mials are considered in (Gasper and Rahman, 1990b). q-Extensions of
Tratnik's (Tratnik, 1991b) system of multivariable orthogonal Racah
polynomials and their special cases will be considered in a subsequent
paper.
2.
Mult ivariable Askey- Wilson polynomials
In terms of the Askey-Wilson polynomials a q-analogue of the multi-
variable Wilson polynomials can be defined by
k
where xk
=
cosOk, Aj,k
=
n
ai,
Ak+1,k
=
1,
Ak
=
Allk,
1
5
j
5
k
5
s.
i=j
Our main aim in this section is to show that these polynomials satisfy
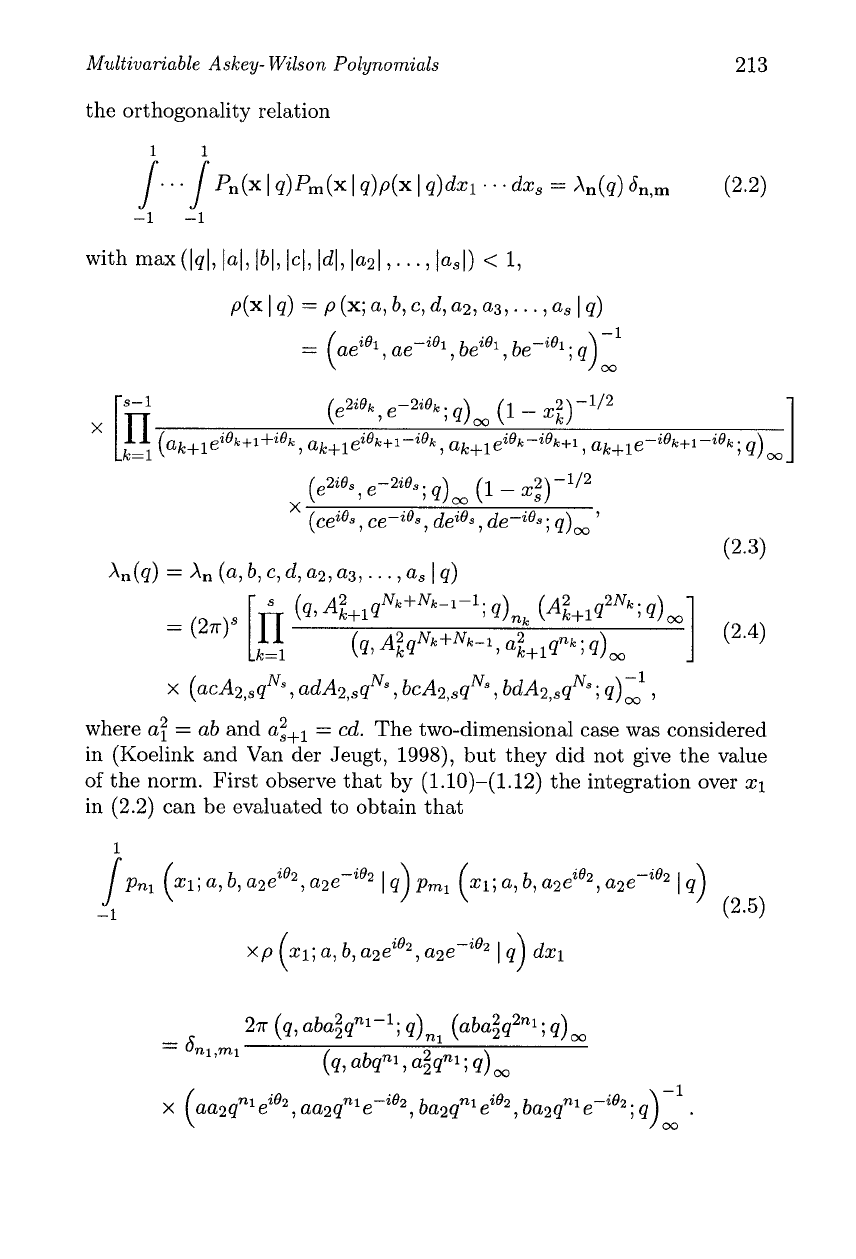
Multivariable Askey- Wilson Polynomials
213
the orthogonality relation
where
a:
=
ab
and
a:+l
=
cd.
The two-dimensional case was considered
in (Koelink and Van der Jeugt, 1998), but they did not give the value
of the norm. First observe that by (1.10)-(1.12) the integration over
xl
in (2.2) can be evaluated to obtain that
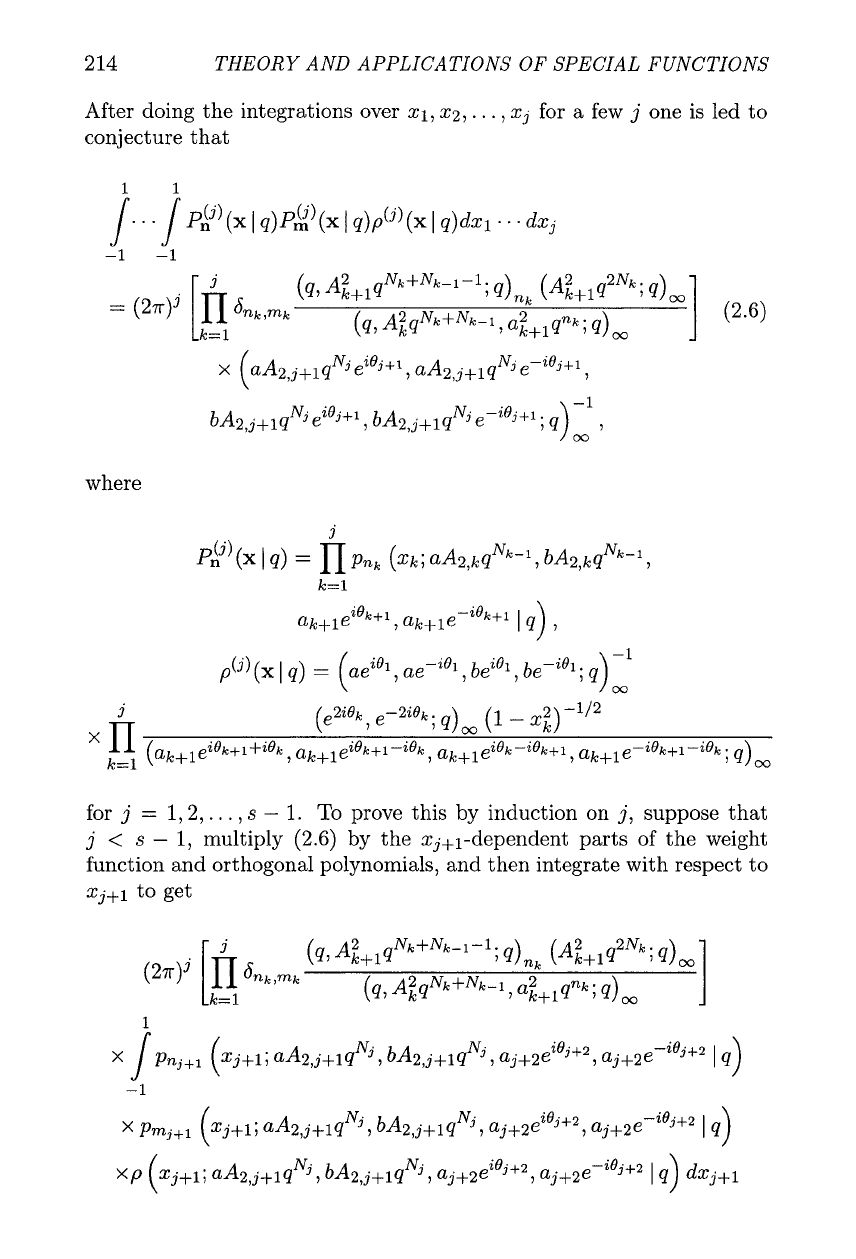
214
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
After doing the integrations over
XI,
x2,.
. .
,
xj for
a
few
j
one is led to
conjecture that
where
for
j
=
1,2,.
.
.
,
s
-
1.
To prove this by induction on
j,
suppose that
j
<
s
-
1,
multiply (2.6) by the xj+1-dependent parts of the weight
function and orthogonal polynomials, and then integrate with respect to
xj+l to get
(24j

Multivariable Askey- Wilson Polynomials
215
b~~,~+~~~~+l
eiej+z,
bA2
j+2qN~+l
e-iej+z
;
q)-l
)
ca
(2.7)
which is the
j
-t
j
+
1
case of (2.6), completing the induction proof.
Now set
j
=
s
-
1
in (2.6) and use it and (2.5) to find that
where Xn(q) is given by (2.4). This completes the proof of (2.2).
Note that the integration region and weight function in (2.2) and (2.3)
are invariant under the permutation of variables and parameters
Hence, when these permutations are applied to (2.2) and (2.3) the trans-
formed polynomials also form an orthogonal system with the same weight
function. Since the polynomials Pn(x
I
q) in (2.1) are not invariant un-
der (2.9), we obtain a second system of multivariable orthogonal Askey-
Wilson polynomials, which is a q-analogue of Tratnik's second system
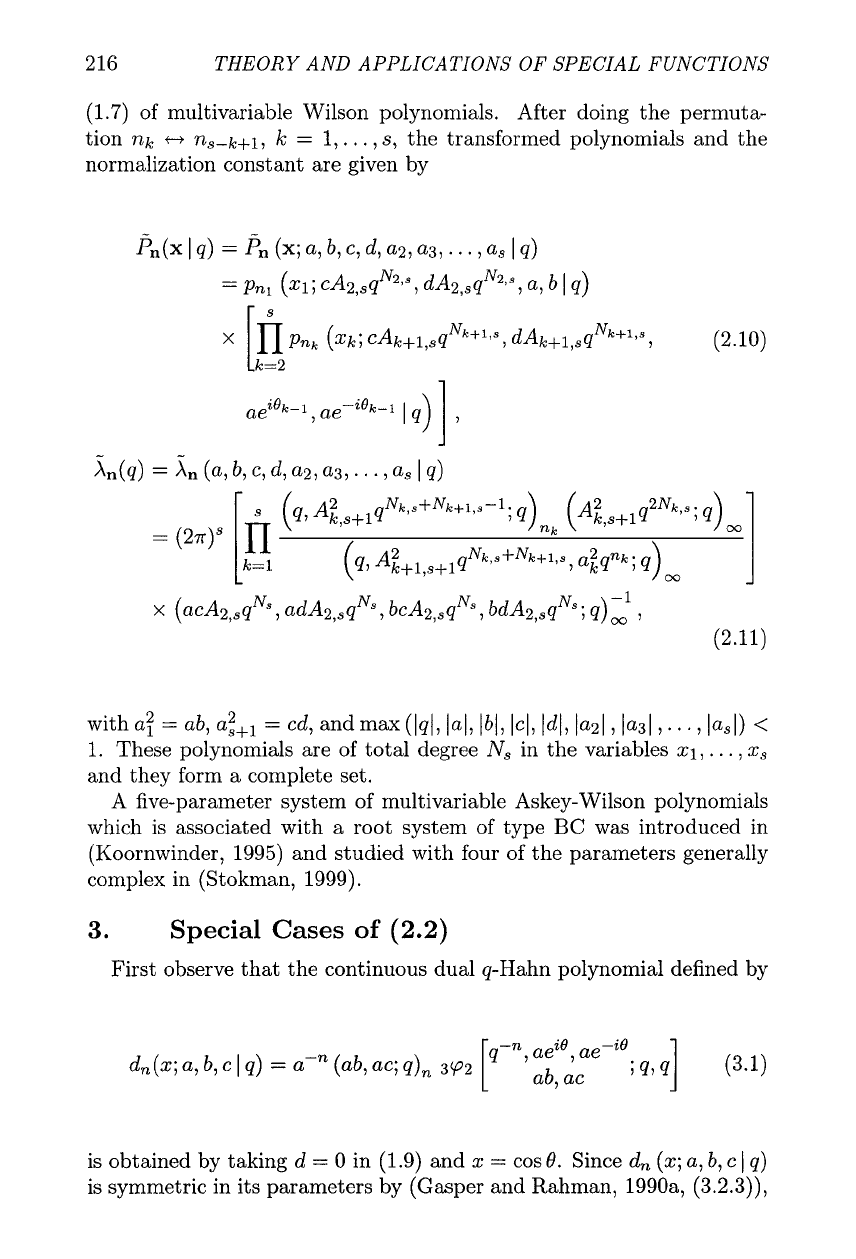
216
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
(1.7) of multivariable Wilson polynomials.
After doing the permuta-
tion nk
tt
ns-k+l,
k
=
1,.
.
.
,
s,
the transformed polynomials and the
normalization constant are given by
with a:
=
ab, a:+1
=
cd, and max (lql, la],
Ibl,
IcI,
Idl, la21,lasl,
.
. .
,
lasl)
<
1. These polynomials are of total degree
N,
in the variables
XI,.
.
.
,
x,
and they form a complete set.
A
five-parameter system of multivariable Askey-Wilson polynomials
which is associated with a root system of type
BC
was introduced in
(Koornwinder, 1995) and studied with four of the parameters generally
complex in (Stokman, 1999).
3.
Special Cases
of
(2.2)
First observe that the continuous dual q-Hahn polynomial defined by
q-n,
aeiO,
ae-ie
dn
(x;
a,
b,
c
1
q)
=
a-n (ab, ac; q),
392
ab, ac
is obtained by taking d
=
0 in (1.9) and x
=
cos
13.
Since dn (x; a,
b,
c
I
q)
is symmetric in its parameters by (Gasper and Rahman, 1990a, (3.2.3)))
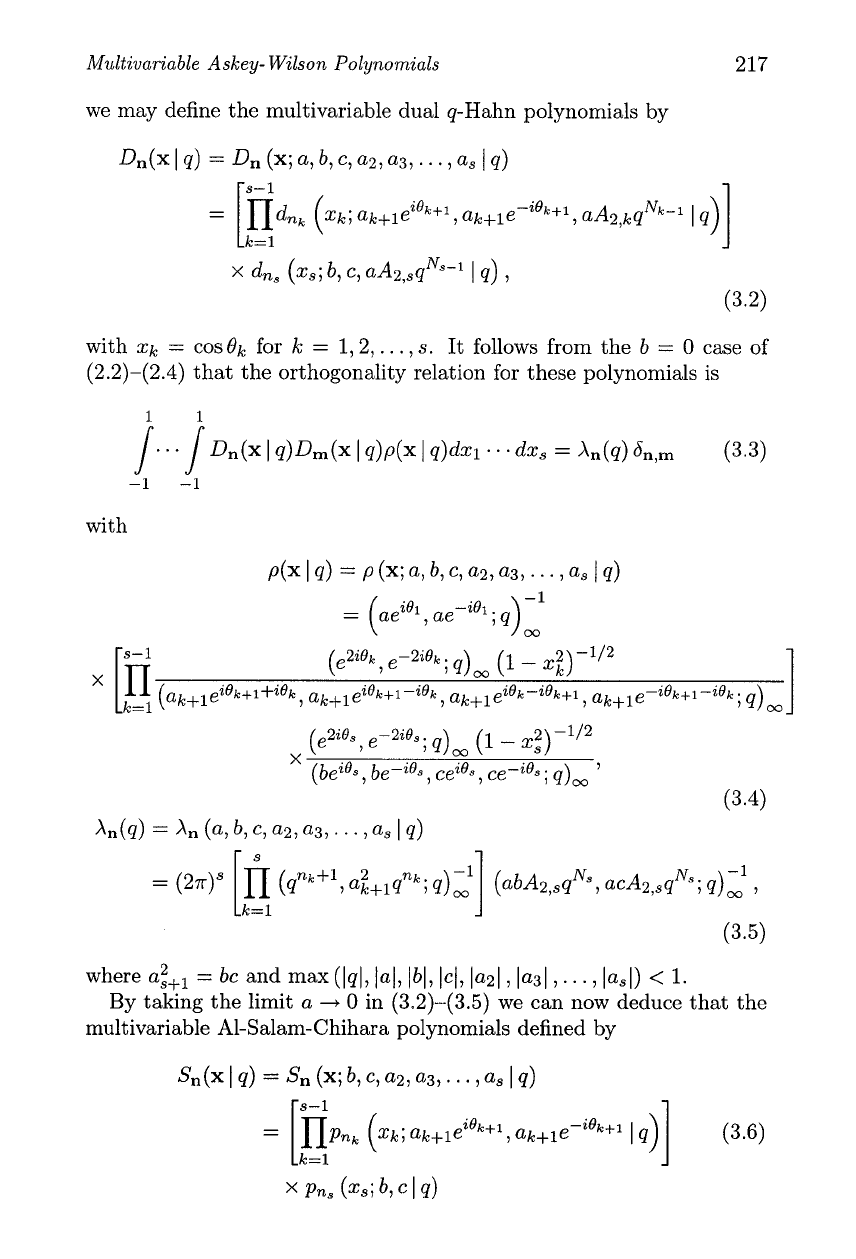
Multivariable Askey- Wilson Polynomials
217
we may define the multivariable dual q-Hahn polynomials by
with
xr,
=
cos
Ok
for
k
=
1,2,
. .
.
,
s.
It follows from the
b
=
0
case of
(2.2)-(2.4)
that the orthogonality relation for these polynomials is
with
where
=
bc
and max
(191, la[,
lbl,
lcl, la2111a31,.
. .
,
las[)
<
1.
By taklng the limit
a
-+
0
in
(3.2)-(3.5)
we can now deduce that the
multivariable Al-Salam-Chihara polynomials defined by
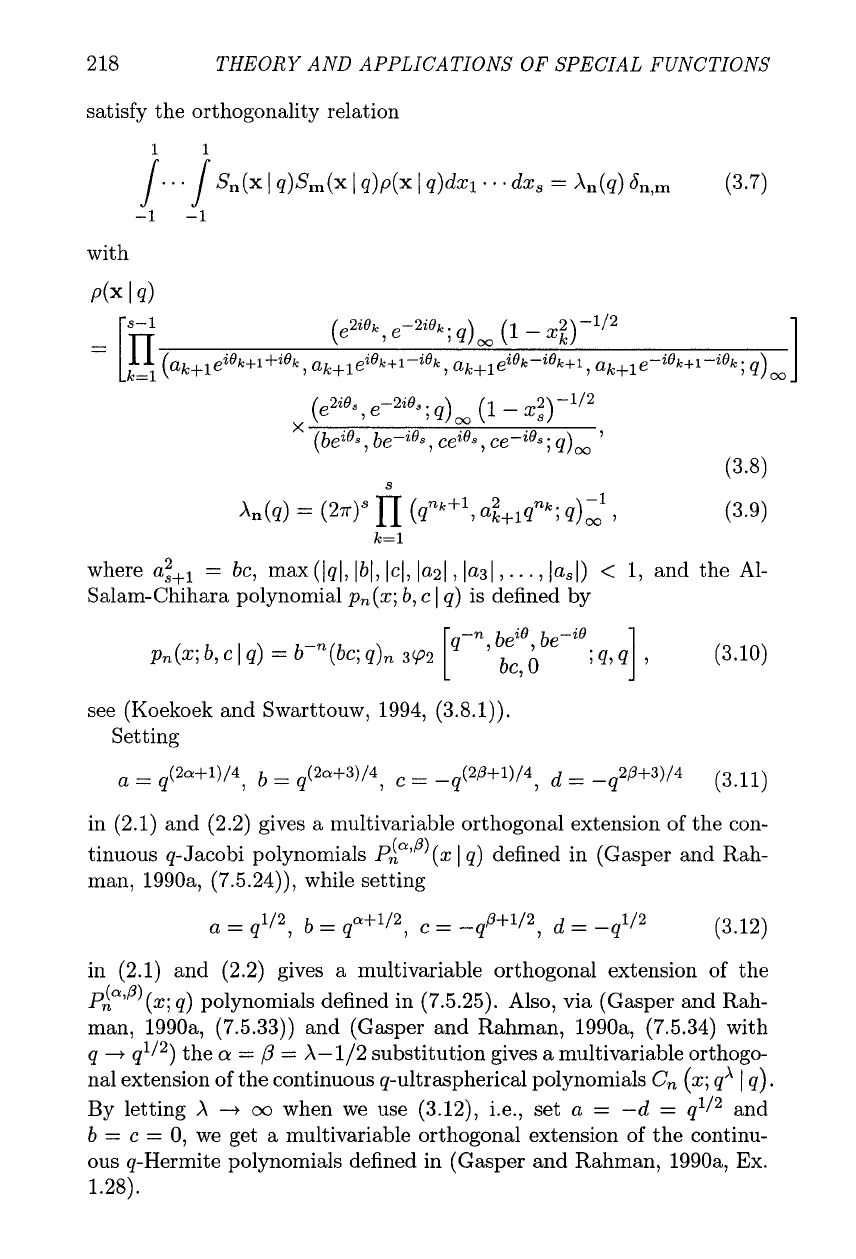
218
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
satisfy the orthogonality relation
with
where
a&l
=
kc,
max(lq1,
lbl,
Icl, lazl,
1~31,.
. .
,
lasl)
<
1,
and the Al-
Salam-Chihara polynomial p,(~;
b,
c
l
q) is defined by
see (Koekoek and Swarttouw, 1994, (3.8.1)).
Setting
in (2.1) and (2.2) gives a multivariable orthogonal extension of the con-
tinuous q- Jacobi polynomials P~'~)(X
1
q) defined in (Gasper and Rah-
man, 1990a, (7.5.24))) while setting
in (2.1) and (2.2) gives a multivariable orthogonal extension of the
ppy8)(x; q) polynomials defined in (7.5.25). Also, via (Gasper and Rah-
man, 1990a, (7.5.33)) and (Gasper and Rahman, 1990a, (7.5.34) with
q
+
q1I2) the
a
=
P
=
A-112 substitution gives a multivariable orthogo-
nal extension of the continuous q-ultraspherical polynomials
Cn
(x; qX
I
q)
.
By letting
X
+
oo
when we use (3.12)) i.e., set a
=
-d
=
q1I2 and
b
=
c
=
0, we get a multivariable orthogonal extension of the continu-
ous q-Hermite polynomials defined in (Gasper and Rahman, 1990a, Ex.
1.28).

Multivariable Askey- Wilson Polynomials
219
A multivariable orthogonal extension of the continuous q-Hahn poly-
nomials defined by
-n
a2b2qn-1,
ae2i(p+if3
ae-if3
=
(a2, ab, abe2";
q)n
(aew)
-"
4~
[9.
7
1
a2, ab, abe2ip
;Q,Q
1
,
(3.13)
see (Gasper and Rahman, 199Oa, (7.5.43)), is obtained from (2.1)-(2.4)
by replacing a,
b,
c,
d,
Qk
and xr,
=
cosQk by alez'+', ale-z", ~,+~e''+',
~,+~e-~q,
Qlc
+
cp
and cos(&
+
cp),
respectively.
It is clear that similar special cases of the second system of multivari-
able orthogonal Askey-Wilson polynomials can be obtained by appro-
priate specialization of the parameters in (2.10) and (2.11). Additional
systems of multivariable orthogonal polynomials will be considered else-
where.
References
Askey,
R.
and Wilson,
J.
A. (1979).
A
set of orthogonal polynomials that generalize
the Racah coefficients or 6-j symbols. SIAM J. Math. Anal., 10:1008-1016.
Askey,
R.
and Wilson,
J.
A. (1985). Some basic hypergeometric orthogonal polyno-
mials that generalize Jacobi polynomials. Memoirs Amer. Math. Soc., 319.
Gasper, G. and Rahman, M. (1990). Basic Hypergeometric Series. Cambridge Uni-
versity Press, Cambridge.
Gasper, G. and Rahman,
M.
(2003). q-analogues of some multivariable biorthogonal
polynomials. This Proceedings.
Koekoek,
R.
and Swarttouw, R.
F.
(1998). The Askey-scheme of hypergeometric or-
thogonal polynomials and its q-analogue. Report 98-17, Delft University of Tech-
nology.
Koelink,
H.
T.
and Van der Jeugt,
J.
(1998). Convolutions for orthogonal polynomials
from Lie and quantum algebra representations. SIAM J. Math. Anal., 29:794-822.
Koornwinder,
T.
H.
(1992). Askey-Wilson polynomials for root systems of type
BC.
In Hypergeometric functions on domains of positivity, Jack polynomials, and appli-
cations (Tampa,
FL,
1991), volume 138 of Contemp. Math., pages 189-204. Amer.
Math. Soc., Providence, RI.
Stokman,
J.
V.
(1999). On
BC
type basic hypergeometric orthogonal polynomials.
Trans. Amer. Math. Soc., 352:1527-1579.
Tratnik, M.
V.
(1989). Multivariable Wilson polynomials. J. Math. Phys., 30:2001-
2011.
Tratnik,
M.
V.
(1991a). Some multivariable orthogonal polynomials of the Askey
tableau--continuous families. J. Math. Phys., 32:2065-2073.
Tratnik, M.
V.
(1991b). Some multivariable orthogonal polynomials of the Askey
tableau-discrete families. J. Math. Phys., 32:2337-2342.
Wilson,
J.
A. (1980). Some hypergeometric orthogonal polynomials. SIAM J. Math.
Anal., 11:690-701.
CONTINUOUS HAHN FUNCTIONS AS
CLEBSCH-GORDAN COEFFICIENTS
Wolter Groenevelt and Erik Koelink
Technische Universiteit Delft
E WI- T WA
Postbus 5031
2600 GA Delft
THE NETHERLANDS
W.G.M.Groenevelt@ewi.tudelft.nl
Hjalmar Rosengren
Department of Mathematics
Chalmers University of Technology and Goteborg University
SE-4 12 96 Gote borg
SWEDEN
Abstract
An explicit bilinear generating function for Meixner-Pollaczek polynomi-
als is proved. This formula involves continuous dual Hahn polynomials,
Meixner-Pollaczek functions, and non-polynomial 3Fz-hypergeometric
functions that we consider as continuous Hahn functions. An integral
transform pair with continuous Hahn functions as kernels is also proved.
These results have an interpretation for the tensor product decomposi-
tion of a positive and a negative discrete series representation of
su(1,l)
with respect to hyperbolic bases, where the Clebsch-Gordan coefficients
are continuous Hahn functions.
1.
Introduction
The results and techniques in this paper are mainly analytic in na-
ture, but they are motivated by a Lie algebraic problem. As is well
known, many polynomials in the Askey-scheme of orthogonal polynomi-
als of hypergeometric type, see (Koekoek and Swarttouw, 1998), have
an interpretation in the representation theory of Lie groups and Lie al-
gebras, see, e.g., Vilenkin and Klimyk (Vilenkin and Klimyk, 1991) and
O
2005
Springer Science+Business Media, Inc.
