Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.

242
THEORY
AND APPLICATIONS OF SPECIAL FUNCTIONS
4.2
Hyperbolic basisvectors
We consider a self-adjoint element
X,
in
su(1,
I),
given by
The action of
X,+,
in the discrete series can be identified with the three-
term recurrence relation for the Meixner-Pollaczek polynomials
(2.3),
cf.
(Koelink and Van der Jeugt,
1998,
Prop.
3.1).
Proposition
4.2.
The operators
O',
defined
by
are unitary and intertwine
nkf
(X,) with M
(f
2x
sin
cp)
.
Here
M
denotes the multiplication operator, i.e.,
M(
f
)g(x)
=
f
(x)g(x).
Proposition
4.2
states that
-.
V~X)
=
c
pik'
(x;
9)
en,
are generalized eigenvectors of
nkf
(X,)
for eigenvalue
f
2x
sin
cp.
These
eigenvectors can be considered
as
hyperbolic basis vectors.
The action of
X,
in the principal unitary series can be identified with
the recurrence relation for the Meixner-Pollaczek functions
(2.6).
Then
the spectral decomposition of the corresponding doubly infinite Jacobi
operator gives the following.
Proposition
4.3.
The operator
Op"
defined
by
(u,
(.;
-
j
+
ip,
E,
n
-
cp)
en
H
u;"l(.;
-3
+
ip,
E,
n
-
cp)
is unitary and intertwines
(X,) with
M(2x
sin
cp),
and extends to a
unitary equivalence.
From Propostion
4.3
we obtain that
00
1
x;
--
+
ip,
E,
n
-
cp)
n=-00
f
2;-3 +ip,~,n-cp)
>
en

Continuous Hahn functions 243
is a generalized eigenvector of
np?&
(X,)
for eigenvalue
2x
sin
cp.
Next we consider the action of
X,
in the tensor product. Recall that
in the tensor product we need the coproduct
A,
defined by
A(Y)
=
1
8
Y
+
Y
8
1
for
Y
E
su(1,l).
Then from Proposition
4.2
we find the
following.
Proposition 4.4.
The operator
T
defined
by
is unitary and intertwznes
n;
8
nG
(A
(X,)) with
M
(2 (xl
-
x2)
sin
9).
In terms of the generalized eigenvectors
v+
and
v-,
we find from
Proposition
4.4
that
CO
+
(1)
8
V-
(2)
=
C
PA:')
(21
;
9)
pi:)
(12;
ip)
enl
0
en2
n1 ,n2=0
is a generalized eigenvector of
n:
0
nG
(A
(X,))
for eigenvalue
2
(xl
-
x2)
sin
cp.
To determine the action of
T
on the representation space of the direct
integral representation
J@
7rP7'dp,
we need to find the operator
T,
such
that
T
=
T
o
J.
Here
J
is the intertwiner defined in Theorem
4.1.
For
appropriate functions
gl
and
92
we define an operator
Tg
by
From Proposition
4.3
we see that
Tg
intertwines
J@
.rrP>'
(X,) dp
with
M
(2tsincp).
The functions
gl
and
g2
for which
T
=
Tg
o
J
are the
Clebsch-Gordan coefficients for the hyperbolic bases. To determine the
Clebsch-Gordan coefficients we use the summation formula in Theorem
3.1.
Define the continuous Hahn function by
244
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Theorem
4.5.
Let
where
pp
(x2; x1
-
x2)
=
pp
(x2; x1
-
x2, kl, k2, p) and m(p) is given by
then we have
T
=
Tg
o
J.
Proof. To show that
T
=
'fg
o
J,
we use the summation formula of
Theorem 3.1 with the orthogonal polynomials written in orthonormal
form. We multiply by a continuous dual Hahn polynomial of degree n2
with the same parameters as in Theorem 3.1, with
p
=
nl
-
n2
2
0.
Then integrating against the corresponding orthogonality measure gives
an equality with the following structure
The 2Fl-functions are the Meixner-Pollaczek functions as defined by
(2.5). From Proposition 4.2 we see that the left hand side is equal to
T
(en,
@
en,) (XI, x2). SO from Theorem 4.1, with nl
2
n2, and Propo-
sition 4.3 it follows that the right hand side must be equal to
where
g
is a vector containing the Clebsch-Gordan coefficients for the
hyperbolic bases. This gives the desired result. For nl
-
n2
<
0
the
theorem follows after using (3.1).
0
Remark
4.6.
The explicit expressions of the Clebsch-Gordan coefi-
cients as 3F2-series can also be found in Mukunda and Radhakrishnan
(Mukunda and Radhakrishnan,
1974).
The method used in (Mukunda
Continuous Hahn functions
245
and Radhakrishnan,
1974)
is completely different from the method used
here.
In terms of the generalized eigenvectors, Theorem
4.5
states that
4.3
The
continuous Hahn integral transform
Since the continuous Hahn functions occur as Clebsch-Gordan coeffi-
cients for hyperbolic bases, they should satisfy (generalized) orthogonal-
ity relations. We find these relations by formal computations with the
generalized eigenvectors.
For an element
f
E
e2
(Z>o)
-
8
e2
(Z>o)
-
we have the transform pair
Similarly for
f
E
e2(Z) we have the transform pair
Denoting the intertwiner
J
in Theorem
4.1
by
J
=
Jpdp, we find
I@
0
from Theorem
4.5
246
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
We want to invert this formula. From (4.10) and Theorem 4.5 we find
formally
We substitute x1
H
x
+
t,
22
H
x, then (4.11) with
f
replaced by
Jp
f
gives
We denote
and (.Fg)(x)
=
(T f)(x
+
t, x), then we have the following integral trans-
form pair with the continuous Hahn functions
as
a kernel
In Section 11.5 we give a rigorous proof for this integral transform pair
using spectral analysis of a difference operator for which the continuous
Hahn functions are eigenfunctions. In the next subsection we obtain the
difference operator from the action of the Casimir element in the tensor
product.
4.4
A
realization of the discrete series
representat ions.
The following lemma is based on the fact that sI(2,
@)
is semi-simple,
so [d(2,
@),
d(2,
@)I
=
d(2,
C).
Continuous Hahn functions
247
Lemma
4.7.
Proof.
This follows from the definition of
X,
and the commutation re-
lations
(4.1).
0
This lemma shows that to find the action of the generators
H,
B
and
C
on the Meixner-Pollaczek polynomials, it is enough to find the action
of
H,
since the action of
X,
is known. The action of
H
follows from the
difference equation
(2.4)
for the Meixner-Pollaczek polynomials.
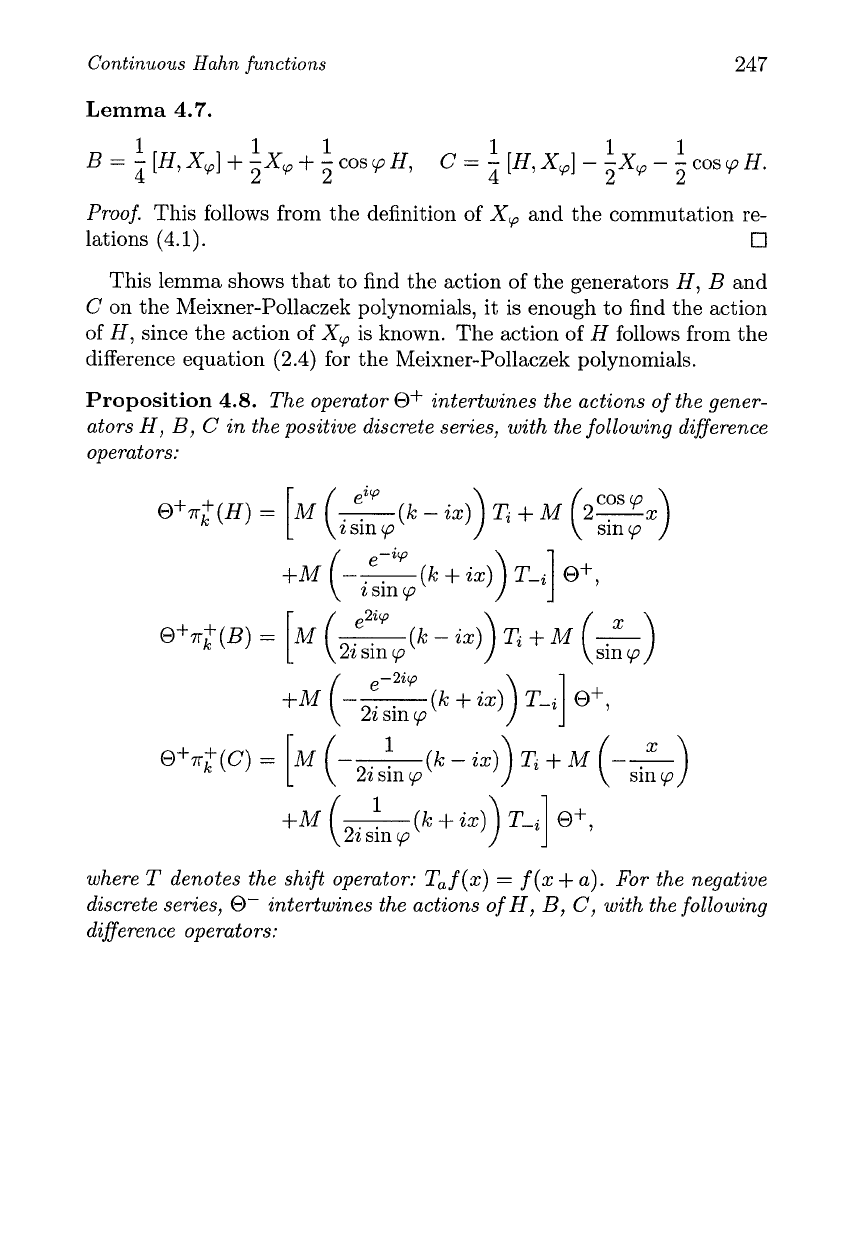
Proposition
4.8.
The operator
0+
intertwines the actions of the gener-
ators H,
B,
C
in
the positive discrete series, with the following difference
operators:
cos
cp
@+<(HI
=
[M
(S--(k
2
sm
cp
-
ix))
T~
+
M
(2-,)
sin
cp
e-%'
--(k
+
ix)
z
sin
cp
e2i~
@+T;(B)
=
[M
(-(k
22
sln
cp
-
ix))
TI
+
M
(L)
sin
cp
1
@+T:(c)
=
[
M
(
--(k-~x))T,+M
2zsincp
(-L)
sin
cp
1
+M
(-
2i
sin
cp
where
T
denotes the shift operator:
T,
f
(x)
=
f (x
+
a).
For the negative
discrete series,
0-
intertwines the actions of
H,
B,
C,
with the following
difference operators:

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
cos
cp
8-xi
(H)
=
[
M
(L:n',
--(k
-
ix))
T,
+
M
(-2-x)
sin
cp
i
sin
cp
1
@-ni(B)
=
M
--
[
(
2isincp (k -ix))
T,
+
M
(-2)
sin
cp
1
2i
sin
cp
e2i'P
e-ni
(c)
=
[M
(-
22
sm
cp
(k
-
ix))
T,
+
M
(2)
sin
cp
Proof.
We find the action of
H
from the difference equation
(2.4)
for the
Meixner-Pollaczek polynomials;
eic~
-
cos
$9
-
-(k- ix)
pik)
(x
+
i;
p)
+
2x7
pi"
(x;
cp)
z
sln
cp
sm
cp
-
-(k
+
ix)~i"(x
-
i;
cp).
z
sm
cp
The action of
X,
is given in Proposition
4.2:
0'
nz
(X,) en
=
22
sin
cp
pik)(%;
cp).
Then Lemma
4.7
proves the proposition for the positive discrete series.
We find the action in the negative discrete series in the same way, or
we use the Lie-algebra isomorphism
19,
given by
Then
IT:
(19(Y))
=
IT;(Y)
for
Y
E
su(1,l).
A
straightforward calculation shows that these operators indeed sat-
isfy the
su(1,l)
commutation relations.
0
Remark
4.9.
To simplify notations we denote
@+IT+
(Q+)* by
IT+,
and
similarly for IT-.
In the same way
as
in Proposition
4.8
it can be shown that
WE
in-
tertwines the actions of
H,
B
and
C
in the principal unitary series with

Continuous Hahn functions 249
2
x
2 diagonal matrices with difference operators as elements. This is
done by finding a difference equation for the Meixner-Pollaczek func-
tions u, and ui, using contiguous relations for 2F1-series. We do not
need these realizations here, so we will not work this out.
To express A(R) in terms of
H,
B
and C, the coproduct
A
is extended
to
U
(su(1,l))
as
an algebra homomorphism. Then from the definition
of the Casimir element (4.2) we find
1
A(n)=l@R+R@l-5H@H-(C@B+B@C).
(4.12)
Using this expression and Proposition 4.8, we find the following.
Proposition
4.10.
In the realizations of Proposition 4.8, we have
TL
@
~g
(A(W
1
x1=x+t,
x2=x
=
M
(kl
+
i(t
+
x)) (k2
+
is))
T-i
+
M (kl
(1
-
kl)
+
k2
(1
-
kz)
-
2(x
+
t)~)
+
M (-e2iv (kl
-
i(t
+
x)) (kZ
-
ix))
T,,
where the shift operator
T
acts with respect to x.
Proof. Let
Fl
(x) and
F2
(x) be polynomials in x, and let
f
(x, t)
=
Fl
(x+
t)
F2
(x)
,
then
a
large but straightforward computation yields
T;
en,; (A(n))
f(4
=
[kl
(1
-
kl)
+
Icz
(1
-
k2)
-
2(~ +t)x] f(x,t)
-
e-2iv (kl
+
i(t
+
x)) (k2
+
ix)
f
(x
-
i,
t)
-
e2iv (kl
-
i(t
+
x)) (k2
-
ix)
f
(x
+
i,
t).
0
Remark
4.11.
The action ofR in the tensor product can also be found
from
n$
(H)
=
M (2ix),
ni (H)
=
M(-2ix))
n$(~)
=
M(k
-
ix)Z,
ni(B)
=
M (e-2iv(k
+
ix))
T+
n$(C)
=
M(k
+
ix)T-i,
T;
(C)
=
M (e2i'+'(k
-
ix))
T,.
These realizations are equivalent to the realizations given in Proposition
4.8.
In the next
section we show that the continuous Hahn functions
vp
(x;
t,
kl, k2,
y)
are eigenfunctions of the difference operator of Propo-
sition 4.10, and we work out the corresponding integral transform.

250
THEORY AND APPLICATIONS
OF
SPECIAL FUNCTIONS
5.
The continuous Hahn integral transform
In this section we study a second order difference operator. This
difference operator is obtained from the action of the Casimir operator
on hyperbolic basis vectors in the tensor product of a positive and a
negative discrete series representation of su(1, I), see Section 11.4.2. The
spectral analysis of this operator, leads to an integral transform pair
with a certain type of 3F2-series as a kernel. We call these 3F2-series
continuous Hahn functions, because of their similarity to continuous
(dual) Hahn polynomials. The method we use is based on asymptotics,
and is essentially the same method as used by Gotze (Gijtze, 1965) and
Braaksma and Meulenbeld (Braaksma and Meulenbeld, 1967) for the
Jacobi function transform by approximating with the Fourier transform.
5.1
The difference operator
A
and the
Wronskian
For kl, k2
>
0, t
E
lR
and
0
<
cp
<
n
the weight function w(x) is
defined by
1
t)(2rp-?r)r
(k1+ it
+
ix)
I'
(kl
-
it
-
ix)
r
(k2
+
ix)
I'
(k2
-
ix)
.
27r
(5.1)
,
,
The difference operator
A
is defined by
where
a*(x)
=
-ef
2i~
(k2
ix) (kl
7
i(t
+
x))
,
P(x)
=
kl (1
-
kl)
+
k2
(1
-
k2)
-
2(t
+
X)X.
Initially
A
is defined for those g(x)
E
L2
(R,
w (x)dx) that have an ana-
lytic continuation to the strip
The difference operator
A
corresponds to the action of the Casimir op-
erator in the tensor product of a positive and a negative discrete series
representation on hyperbolic basis vectors, see Proposition
4.10.
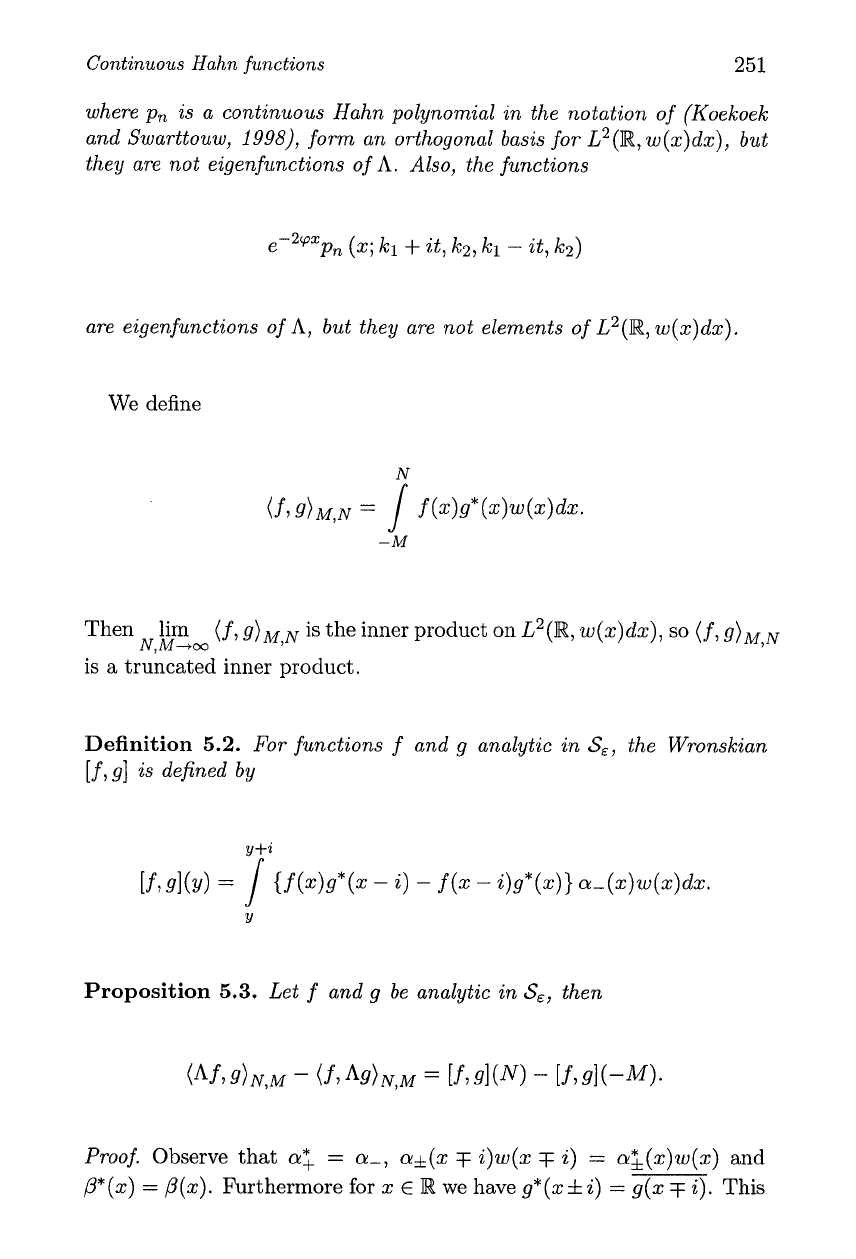
Remark
5.1.
Observe that the functions
-
e
x(2'p-?r)p,
(x; k1
+
it, k2, kl
-
it, k2)
,
Continuous
Hahn
functions
251
where pn is a continuous Hahn polynomial
in
the notation of (Koekoek
and Swarttouw,
1
998), form an orthogonal basis for L2
(R,
w(x)dx), but
they are not eigenfunctions of
A.
Also, the functions
-
e
2'pxpn
(x;
k1
+
it,
k2,
k1
-
it,
k2)
are eigenfunctions of
A,
but they are not elements of L2(R, w(x)dx)
We define
Then lim
(f
,
g)
M,N
is
the inner product on
L~
(w,
w (%)dm),
so
(f,
g)
M,N
N,M+oo
is
a truncated inner product.
Definition
5.2.
For functions f and g analytic
in
S,,
the Wronskian
[f, g] is defined by
Proposition
5.3.
Let f and g
be
analytic in
S,,
then
Proof.
Observe that
a;
=
a_,
a*(x
i)w(x
i)
=
a$(x)w(x)
and
P*
(x)
=
P(x).
Furthermore for
x
E
R
we have
g* (x
f
i)
=
g(x
i)
.
This
