Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.

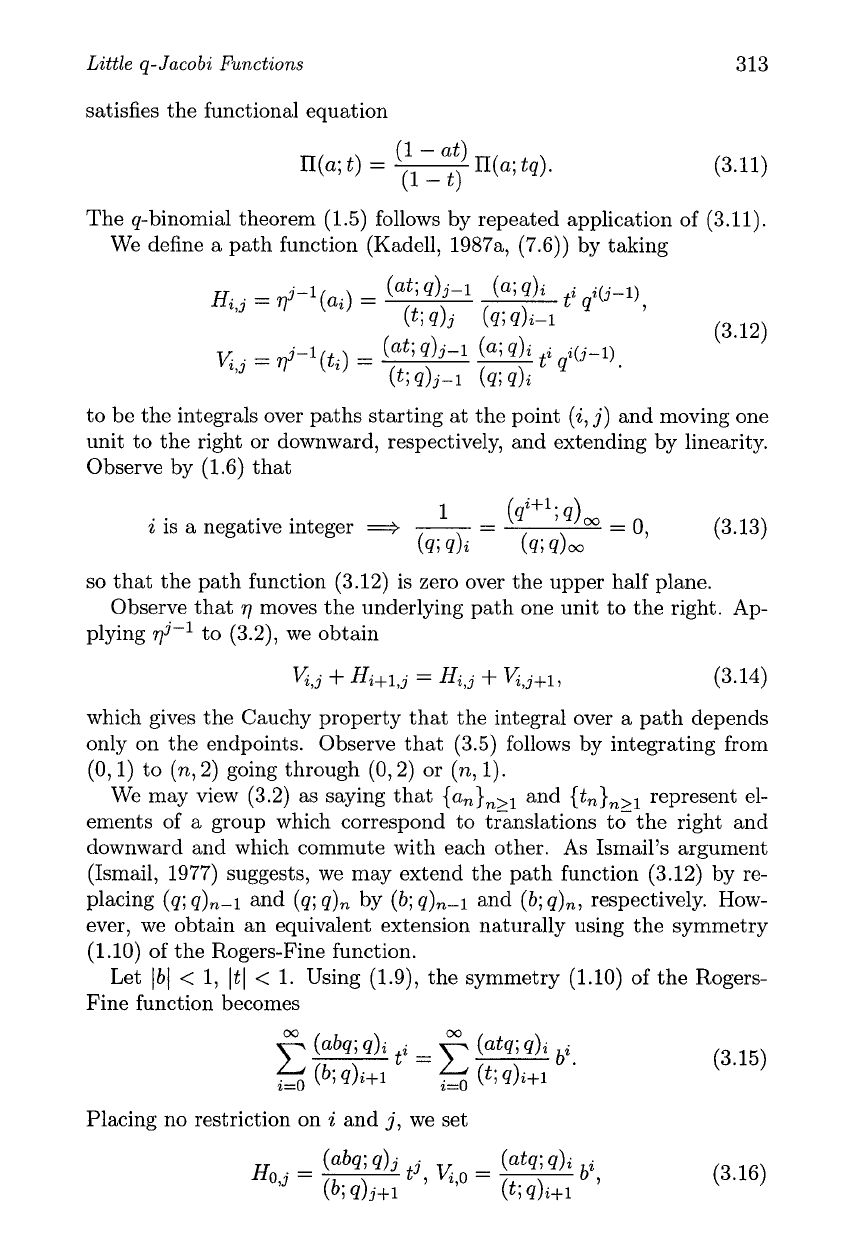
Little

q-Jacobi

Functions

313

satisfies the functional equation

The q-binomial theorem (1.5) follows by repeated application of (3.11).

We define a path function (Kadell, 1987a, (7.6)) by taking

to be the integrals over paths starting at the point (i,

and moving one

unit to the right or downward, respectively, and extending by linearity.

Observe by (1.6) that

so that the path function (3.12) is zero over the upper half plane.

Observe that q moves the underlying path one unit to the right. Ap-

plying

qj-l

to (3.2), we obtain

which gives the Cauchy property that the integral over a path depends

only on the endpoints. Observe that (3.5) follows by integrating from

(0,l) to

(n,

going through (0,2) or

(n,

1).

We may view (3.2) as saying that

{u,),,~

and {tn)n,l represent el-

ements of a group which correspond to translations to the right and

downward and which commute with each other. As Ismail's argument

(Ismail, 1977) suggests, we may extend the path function (3.12) by re-

placing (q;

q)n-l

and (q; q), by

(b;

q),-l and

(b;

q),, respectively. How-

ever, we obtain an equivalent extension naturally using the symmetry

(1.10) of the Rogers-Fine function.

Let

Ibl

It/

Using (1.9), the symmetry (1.10) of the Rogers-

Fine function becomes

Placing no restriction on

and

we set

314

THEORY AND APPLICATIONS

SPECIAL FUNCTIONS

so that

(3.15)

becomes

Observe that the operators

are invertible and commute with each other, and that we have

in agreement with

(3.16).

We see using

(3.19)

that the path function

satisfies the translation property

We easily check that

and, interchanging

and

1 (1

atd

abtq)

V0,o

H1,o

b) (1

(3.23)

Since these are equal, we see that the Cauchy integral formula

(3.14)

holds when

Using

(3.21),

we may extend

(3.14)

to the plane.

The formulation

(3.17)

of the symmetry

(1.10)

of the Rogers-Fine

function now follows using the Cauchy integral formula

(3.14)

and the

fact that

n n

lim

1/,,

lim

VnJ.

n+oo

n--00

(3.24)

i=O

j=O

Little q-Jacobi Functions 315

Andrews and Askey (Andrews and Askey, 1978) gave a simple proof

of Ramanujan's sum (1.8) which essentially used the q-binomial

theorem (1.5) and the extended path function (3.19).

We see by the Cauchy integral formula (3.14) that the integrals

of the path function (3.12) from

(n,

to infinity along horizontal and

vertical paths are equal. The reader may verify that (3.25) equals

tn (a; q),/(q; q)n-l times the case

qn of the symmetry (1.10) of the

Rogers-Fine function. Hence (1.10) also follows by applying Ismail's

argument (Ismail, 1977) to the parameter

Heine's q-Gauss sum

(1.4)

In this section, we recall (Kadell, 1987a, Section 7) the path function

proof of Heine's q-Gauss sum (1.4) which implies Ramanujan's sum

(1.8) which implies the symmetry (1.10) of the Rogers-Fine function. We

use Ismail's argument (Ismail, 1977) to establish Heine's q-Gauss sum

(1.4) using Ramanujan's sum (1.8) and the elementary combinatorial

identity of Section 2.

Since (3.2) reduces to

we have the separation

with respect to the operator

given by

Since (3.9) holds, we see by (3.5) that

316

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

satisfies the functional equation

Heine's q-Gauss sum (1.4) follows by repeated application of (4.5) and

the q-binomial theorem (1.5).

Observe by (1.6) that

Using (2.11), (4.6) and the fact that

(;)

(nzl),

we have

and hence

Substituting (4.8) into Ramanujan's

sum (1.8), replacing a,

by abq,

bq, respectively, and dividing by

b), we have the Laurent expansion

in the annulus lllal

It1

Observe that the function on the right side of (4.9) is symmetric in

and t. Recall that the Laurent expansion of a function which is analytic

in a given annulus is unique. Hence the sum of the terms in (4.9) with

nonnegative powers of t is symmetric in b and t, which is the symmetry

(1.10) of the Rogers-Fine function.

Let

[w]

denote the coefficient of the monomial

in the Laurent

expansion of the function

in a given annulus.

Let

0 be a nonnegative integer and let lABl

1. Using the q-

binomial theorem (1.5) to extract the coefficient of

in the non empty

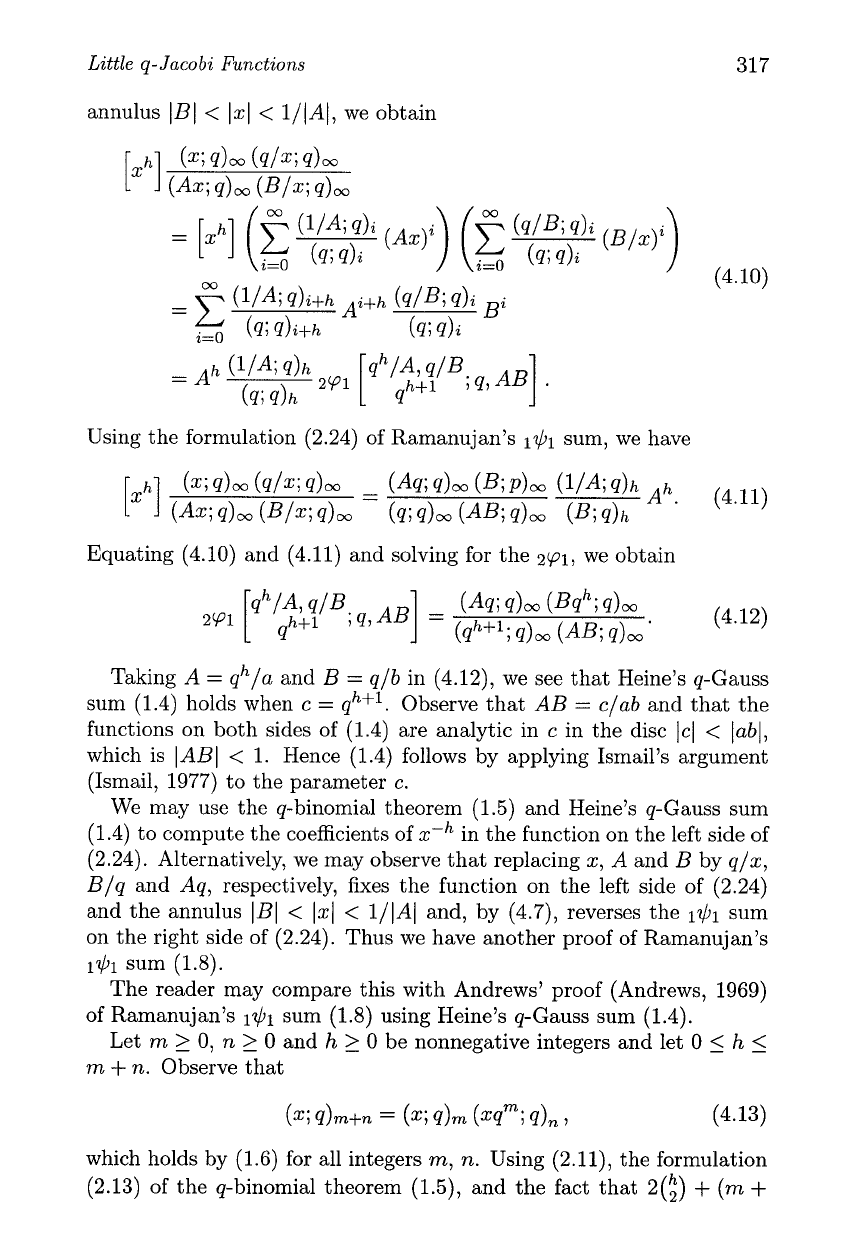
Little

Jacobi

Functions

annulus

IBI

1x1

1/IAl, we obtain

Using the formulation (2.24) of Ramanujan's

sum, we have

Equating (4.10) and (4.11) and solving for the apl, we obtain

Taking A

qh/a and B

q/b in (4.12), we see that Heine's q-Gauss

sum (1.4) holds when c

qh+l.

Observe that AB

club and that the

functions on both sides of (1.4) are analytic in c in the disc Icl

lab[,

which is lABl

1. Hence (1.4) follows by applying Ismail's argument

(Ismail, 1977) to the parameter c.

We may use the q-binomial theorem (1.5) and Heine's q-Gauss sum

(1.4) to compute the coefficients of

x-~

in the function on the left side of

(2.24). Alternatively, we may observe that replacing x, A and B by q/x,

Blq and Aq, respectively, fixes the function on the left side of (2.24)

and the annulus IBI

1x1

l/IAl and, by (4.7)) reverses the sum

on the right side of (2.24). Thus we have another proof of Ramanujan's

sum (1.8).

The reader may compare this with Andrews' proof (Andrews, 1969)

of Ramanujan's

sum (1.8) using Heine's q-Gauss sum (1.4).

Let m

0 and

0 be nonnegative integers and let 0

Observe that

which holds by (1.6) for all integers

Using (2.11)' the formulation

(2.13) of the q-binomial theorem (1.5), and the fact that 2

(';)

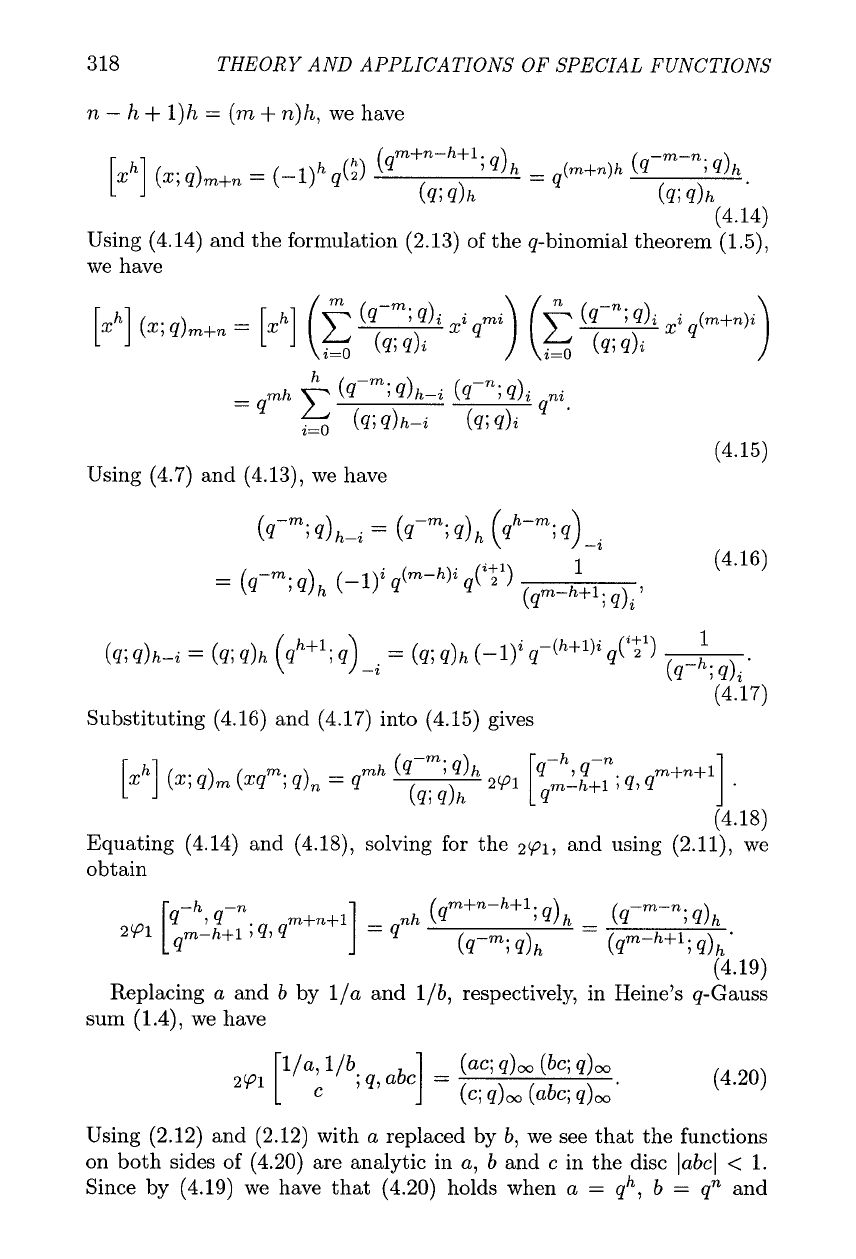
318

THEORY

AND APPLICATIONS OF SPECIAL FUNCTIONS

n-h+l)h= (m+n)h, we have

Using (4.14) and the formulation (2.13) of the q-binomial theorem (1.5),

we have

(4.15)

Using (4.7) and (4.13), we have

(9;

9)h-i

(q; q)h (qh+l; q)

(q; q)h

(-l)i

P-(h+l)i

q(z:l)

-i

(Q-~;

q)i

(4.17)

Substituting (4.16) and (4.17) into (4.15) gives

Equating (4.14) and (4.18), solving for the 291, and using (2.11), we

obtain

Replacing a and

by lla and

llb,

respectively, in Heine's q-Gauss

sum (l.4), we have

;

q, abc

(ac;

(bc;

291

[Ila2lb

]

(c;

q)m (abc; q),

Using (2.12) and (2.12) with a replaced by

we see that the functions

on both sides of (4.20) are analytic in a,

and c in the disc

label

Since by (4.19) we have that (4.20) holds when a

qh,

qn and

Little q-Jacobi Functions 319

qm-h+l, we see that (4.20) follows by three successive applications

of Ismail's argument (Ismail, 1977) to the parameters c,

and a.

Observe that the first two applications of Ismail's argument (Ismail,

1977) gives the q-Chu-Vandermonde sum (1.3). We may avoid the first

two applications of Ismail's argument and establish (1.3) directly by

considering [xh] (x; q),/(z/ab; q),

The nonterminat ing q-Chu-Vandermonde

sum

(1.12)

In this section, we give a path function proof of the case t

q of

Heine's

2p1

transformation (1.11) which implies the symmetry (1 .lo) of

the Rogers-Fine function. Following Gasper and Rahman (Gasper and

Rahman, 1990, Section 2.10), we write Ramanujan's sum (1.8) as

the nonterminating q-Chu-Vandermonde sum (1.12).

Since (3.2) reduces to

we have the separation

with respect to the operator 7 given by

Comparing (4.1) and (5.1), we see that there must be a relation be-

tween the separations (4.2) and (5.2). Applying 7-' to (3.2) and rear-

ranging the result, we have

Hence the separation

{an)ntl

of {tnJntl with respect to 7-' is given

by (Kadell, 1987a, (6.28))

The reader may check that the separation (5.2) is obtained by replacing

q by l/q in the separation (5.5) obtained from (4.2) and (4.3).

Observe that

lim an

(a; q)m

q)m

n+m

(Q;

(c;

(5.6)

320

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Observe by (3.5) that the function

satisfies the q-difference equation

Repeated application of (5.8) and multiplication and division by (1

yields

211

(5.9)

which is the case t

q of Heine's 2pl transformation

(1.11)

with a and

interchanged.

Replacing a,

and

by t,

and abtq, respectively, in (5.9) and rear-

ranging the result, we have

F(ab,b;t)=C

i=o

(5.10)

Since the function on the right side of (5.10) is symmetric in

and t, we

have another proof of the symmetry (1.10) of the Rogers-Fine function.

Replacing a,

and

by t, b/q and at, respectively, in (5.9), multiplying

by (1

b/q), and rearranging the result, we have

Replacing a,

and t by q2/b, q2/a and blat, respectively, in (5.11), we

have

(q2/b; q)i

(q;

(q2!ati q)

[p/a, Vat;

Z=O

(q2/a; q)i @/at)'

(q2/a; q), (blat; q),

q2/at

(5.12)

Let Iblal

It\

1. Observe using (4.8) that we may write Ramanu-

jan's

1+1

sum

(1.8)

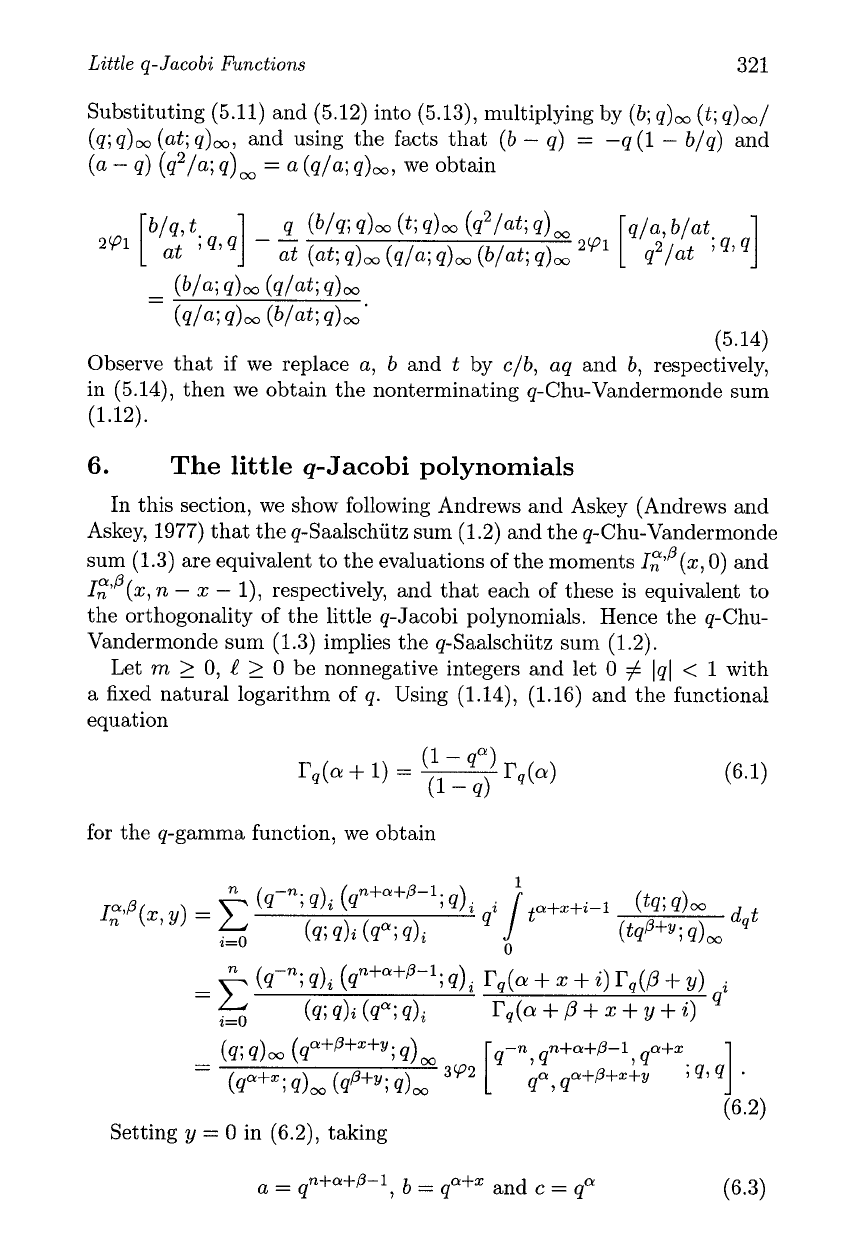
Little q-Jacobi Functions 321

Substituting (5.11) and (5.12) into (5.13), multiplying by

(b;

q), (t; q),/

(q; q)m (at; q),, and using the facts that

-q

b/q) and

q) (q2/a; q),

a (qla; q),, we obtain

(5.14)

Observe that if we replace a,

and t by c/b, aq and

respectively,

in (5.14), then we obtain the nonterminating q-Chu-Vandermonde sum

(1.12).

The little q-Jacobi polynomials

In this section, we show following Andrews and Askey (Andrews and

Askey, 1977) that the q-Saalschiitz sum (1.2) and the q-Chu-Vandermonde

sum (1.3) are equivalent to the evaluations of the moments

I~~~(X,

0) and

I:'~(X,

I), respectively, and that each of these is equivalent to

the orthogonality of the little q-Jacobi polynomials. Hence the q-Chu-

Vandermonde sum (1.3) implies the q-Saalschiitz sum (1.2).

Let

0 be nonnegative integers and let 0

14.1

with

a fixed natural logarithm of q. Using (1.14), (1.16) and the functional

equation

for the q-gamma function, we obtain

Setting

0 in (6.2), taking

(6.2)

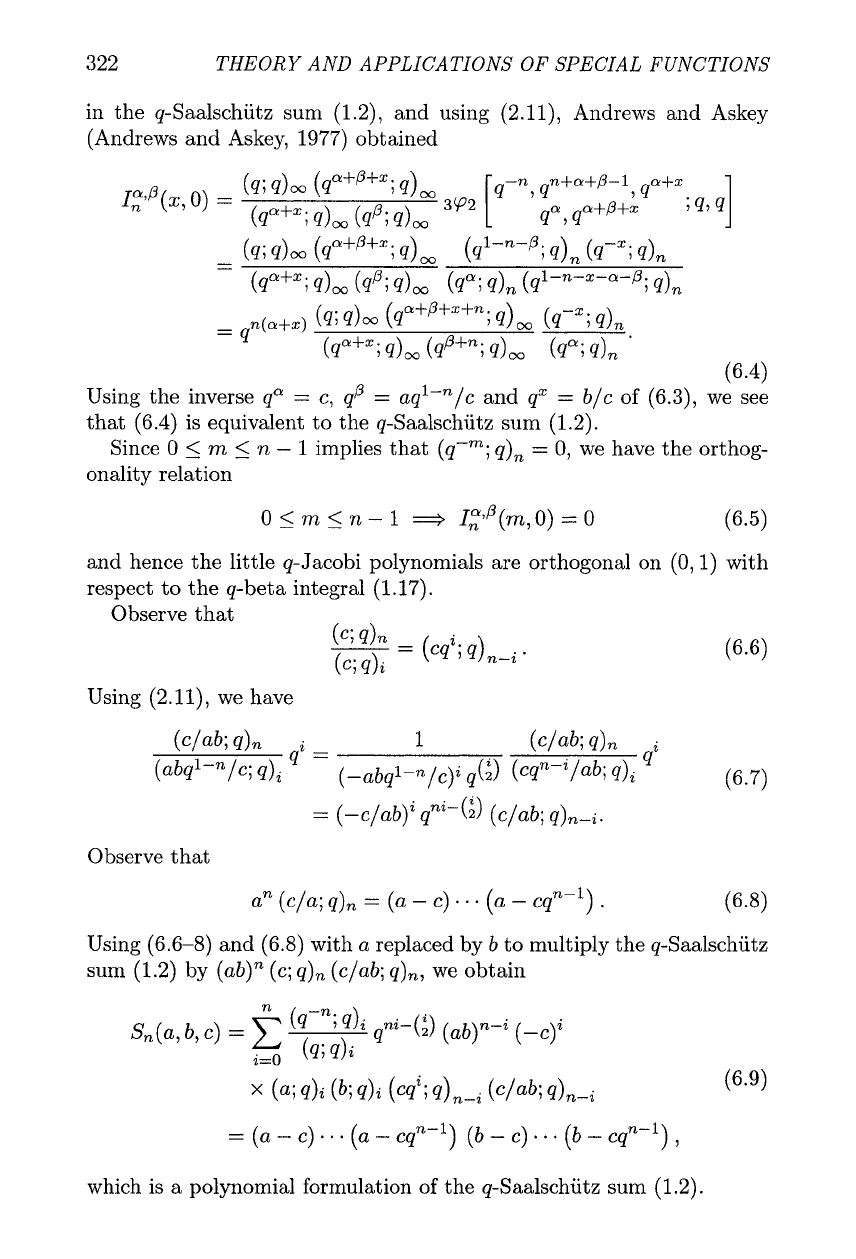
322

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

in the q-Saalschiitz sum (1.2), and using (2.11), Andrews and Askey

(Andrews and Askey) 1977) obtained

Using the inverse q"

c, qP

aql-n/c and qx

blc of (6.3)) we see

that (6.4) is equivalent to the q-Saalschutz sum (1.2).

Since 0

implies that (q-m; q)n

0, we have the orthog-

onality relation

and hence the little q-Jacobi polynomials are orthogonal on (0,l) with

respect to the q-beta integral (1.17).

Observe that

Using (2. ll), we have

Observe that

Using (6.6-8) and (6.8) with a replaced by

to multiply the q-Saalschutz

sum (1.2) by (ab)" (c; q), (club; q),, we obtain

which is a polynomial formulation of the q-Saalschutz sum (1.2).