Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.

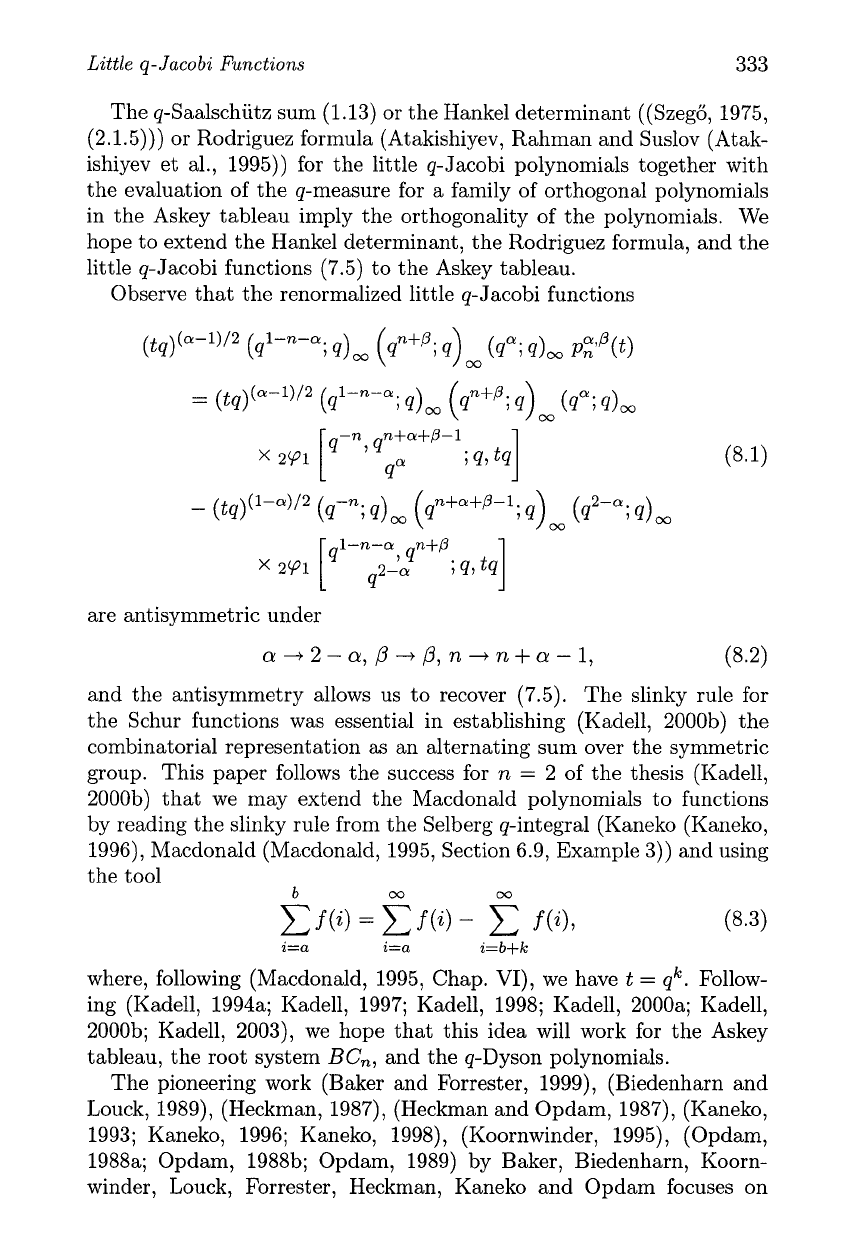
Little q-Jacobi Functions 333
The q-Saalschiitz sum (1.13) or the Hankel determinant ((Szega, 1975,
(2.1.5))) or Rodriguez formula (Atakishiyev, Rahman and Suslov (Atak-
ishiyev et al., 1995)) for the little q-Jacobi polynomials together with
the evaluation of the q-measure for a family of orthogonal polynomials
in the Askey tableau imply the orthogonality of the polynomials. We
hope to extend the Hankel determinant, the Rodriguez formula, and the
little q-Jacobi functions (7.5) to the Askey tableau.
Observe that the renormalized little q-Jacobi functions
are antisymmetric under
and the antisymmetry allows us to recover (7.5). The slinky rule for
the Schur functions was essential in establishing (Kadell, 2000b) the
combinatorial representation as an alternating sum over the symmetric
group. This paper follows the success for
n
=
2 of the thesis (Kadell,
2000b) that we may extend the Macdonald polynomials to functions
by reading the slinky rule from the Selberg q-integral (Kaneko (Kaneko,
1996)) Macdonald (Macdonald, 1995, Section 6.9, Example 3)) and using
the tool
b
00
00
where, following (Macdonald, 1995, Chap.
VI),
we have
t
=
qk. Follow-
ing (Kadell, 1994a; Kadell, 1997; Kadell, 1998; Kadell, 2000a; Kadell,
2000b; Kadell, 2003)) we hope that this idea will work for the Askey
tableau, the root system BCn, and the q-Dyson polynomials.
The pioneering work (Baker and Forrester, 1999), (Biedenharn and
Louck, l989), (Heckman, NU), (Heckman and Opdam, 1987)) (Kaneko,
1993; Kaneko, 1996; Kaneko, 1998)) (Koornwinder, 1995)) (Opdam,
1988a; Opdam, 1988b; Opdam, 1989) by Baker, Biedenharn, Koorn-
winder, Louck, Forrester, Heckman, Kaneko and Opdam focuses on

334
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
the multivariable basic hypergeometric functions and orthogonal poly-
nomials associated with root systems. We hope to extend the Mac-
donald (Macdonald, 1995, Chap. VI), Bidenharn-Louck (Biedenharn
and Louck, l989), Heckman-Opdam (Heckman, l987), (Heckman and
Opdam, 1987), (Opdam, 1988a; Opdam, 1988b), Koornwinder (Koorn-
winder, 1995), and Sahi-Knop (Sahi and Knop, 1996) polynomials to
functions of complex argument and to extend the properties of orthog-
onal polynomials and q-series identities. We hope to follow Section 5 of
this paper and combine the transformation formulas of Baker and For-
rester (Baker and Forrester, 1999) with Kaneko7s multivariable sum
(Kaneko, 1998) to give a multivariable nonterminating q-Chu-Vandermonde
sum and a multivariable Rogers-Fine symmetric function. We hope to
use Ismail's argument (Ismail, 1977) to prove Heine's
291
transformation
(1.11) and extend this to the multivariable setting.
We hope to give a Vinet operator (see (Vinet and Lapointe, 1995))
of complex order which extends the Rodriguez formulas for the Askey
tableau.
References
Anderson,
G.
(1991).
A short proof of Selberg's generalized beta formula. Forum
Math.,
3:415-417.
Andrews,
G.
E.
(1965).
A simple proof of Jacobi's triple product identity. Proc. Amer.
Math. Soc.,
16:333-334.
Andrews,
G.
E.
(1969).
On Ramanujan's summation of l&(a; b;
2).
Proc. Amer. Math.
Soc.,
22:552-553.
Andrews, G.
E.
(1972).
Two theorems of Gauss and allied identities proved arithmeti-
cally. Pacific
J.
Math.,
41:563-568.
Andrews,
G.
E.
(1998).
The Theory of Partitions. Cambridge Mathematical Library.
Cambridge University Press, Cambridge. Reprint of the
1976
original.
Andrews, G. E. and Askey, R.
(1977).
Enumeration of partitions: the role of Eulerian
series and q-orthogonal polynomials. In Aigner, M., editor, Higher Combinatorics,
pages
3-26.
Reidel, Boston, Mass.
Andrews,
G.
E.
and Askey, R.
(1978).
A simple proof of Ramanujan's summation of
the
I$J~.
Aequationes Math.,
18:333-337.
Andrews,
G.
E. and Askey, R.
(1981).
Another q-extension of the beta function. Proc.
Amer. Math. Soc.,
81:97-102.
Aomoto,
K.
(1987).
Jacobi polynomials associated with Selberg's integral. SIAM
J.
Math. Anal.,
18:545-549.
Askey, R.
(1978).
The q-gamma and q-beta functions. Appl. Anal.,
8:125-141.
Askey, R.
(1980).
Some basic hypergeometric extensions of integrals of Selberg and
Andrews. SIAM
J.
Math. Anal.,
11:938-951.
Askey,
R.
and Ismail, M. E.-H.
(1979).
The very well poised
6&.
Proc. Amer. Math.
Soc.,
77:218-222.

Little
q-
Jacobi Functions
335
Askey, R. and Wilson,
J.
(1985).
Some basic hypergeometric orthogonal polynomials
that generalize Jacobi polynomials. Mem. Amer. Math. Soc.,
54(319):iv+55.
Atakishiyev,
N.
M.,
Rahman, M., and Suslov, S.
K.
(1995).
On classical orthogonal
polynomials. Constr. Approx.,
11:181-226.
Baker,
T.
H.
and Forrester, P.
J.
(1999).
Transformation formulas for multivariable ba-
sic hypergeometric series. Methods Appl. Anal.,
6(2):147-164.
Dedicated to Richard
A. Askey on the occasion of his
65th
birthday, Part 11.
Biedenharn,
L.
C. and Louck,
J.
D.
(1989).
A new class of symmetric polynomials
defined in terms of tableaux. Adv. in Appl. Math.,
10:396-438.
Evans, R.
(1994).
Multidimensional beta and gamma integrals. Contemp. Math.,
166:341-357.
Fine,
N.
J.
(1988).
Basic Hypergeometric Series and Applications, volume
27
of Math-
ematical Surveys and Monographs. Amer. Math. Soc., Providence, RI.
Garvan,
F.
and Gonnet, G.
(1991).
Macdonald's constant term conjectures for excep-
tional root systems. Bull. Amer. Math. Soc. (N.S.),
24:343-347.
Garvan, F. G.
(1989a).
A beta integral associated with the root system gz. SIAM
J.
Math. Anal.,
19:1462-1474.
Garvan, F. G.
(1989b).
A
proof of the Macdonald-Morris root system conjecture for
f4.
SIAM
J.
Math. Anal.,
21:803-821.
Gasper, G.
(1997).
Elementary derivations of summation and transformation formulas
for q-series. In Ismail,
M.
E. H., Masson,
D.
R., and Rahman, M., editors, Special
Functions, q-Series and Related Topics (Toronto, ON,
1995),
volume
14
of Fields
Instit. Commun., pages
55-70.
Amer. Math. Soc., Providence, RI.
Gasper, G. and Rahman, M.
(1990).
Basic Hypergeometric Series. Cambridge Univ.
Press, Cambridge.
Gustafson, R.
A.
(1990).
A
generalization of Selberg's beta integral. Bull. Amer. Math.
SOC. (N.S.),
22:97-105.
Habsieger,
L.
(1986).
La q-conjecture de Macdonald-Morris pour
gz.
C.
R.
Acad. Sci.
Paris Sh. I Math.,
303:211-213.
Habsieger,
L.
(1988).
Une q-intbgrale de Selberg-Askey. SIAM
J.
Math. Anal.,
19:1475-
1489.
Hahn, W.
(1949).
~ber polynome, die gleichzeitig zwei verschieclen orthogonalsyste-
men angehoren. Math. Nachr.,
2:263-278.
Heckman, G.
J.
(1987).
Root systems and hypergeometric functions 11. Compositio
Math.,
64:353-373.
Heckman, G.
J.
and Opdam, E. M.
(1987).
Root systems and hypergeometric func-
tions
I.
Compositio Math.,
64:329-352.
Heine, E.
(1847).
Untersuchungen iiber die Riehe
. . ..
J.
reine angew. Math.,
34:285-
328.
Heine, E.
(1878).
Handbuch der Kugelfunctionen, Theorie und Anwendungen, vol-
ume
1.
Reimer, Berlin.
Hille, E.
(1973).
Analytic Function Theory, volume
1.
Chelsea, New York, second
edition.
Hua,
L.
K.
(1963).
Harmonic Analysis of Functions of Several Complex Variables
in the Classical Domains, volume
6
of Transl. Math. Monogr. Amer. Math. Soc.,
Providence, RI.
Ismail, M. E.-H.
(1977).
A simple proof of Ramanujan's
~$1
summation formula.
Proc. Amer. Math. Soc.,
63:185-186.

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Ismail, M. E.-H. and Rahman, M. (1995). Some basic bilateral series and integrals.
Pacific J. Math., 170:497-515.
Jackson,
F.
H. (1910). On q-definite integrals. Quart. J. Pure Appl. Math., 41
:
193-203.
Joichi,
J.
T. and Stanton, D. (1989). An involution for Jacobi's identity. Discrete
Math., 73:261-271.
Kadell, K.
W.
J.
(1987a). Path functions and generalized basic hypergeometric func-
tions. Mem. Amer. Math. Soc., 65(360):iv+54.
Kadell, K.
W.
J.
(198713). A probabilistic proof of Ramanujan's
~$l
sum. SIAM J.
Math. Anal., 18:1539-1548.
Kadell, K. W. J. (1988a).
A
proof of Askey's conjectured q-analogue of Selberg's
integral and a conjecture of Morris. SIAM J. Math. Anal., 19:969-986.
Kadell, K.
W.
J.
(1988b). The q-Selberg polynomials for
n
=
2. Trans. Amer. Math.
SOC., 310(2):535-553.
Kadell, K.
W.
J.
(1993). An integral for the product of two Selberg-Jack symmetric
polynomials. Compositio Math., 87:5-43.
Kadell, K.
W.
J.
(1994a).
A
proof of the q-Macdonald-Morris conjecture for
bc,.
Mem.
Amer. Math. Soc., 108(516):vi+80.
Kadell, K.
W.
J.
(1994b). A simple proof of an Aomoto type extension of the q-Morris
theorem. Contemp. Math., 166:167-181.
Kadell, K.
W.
J.
(1997). The Selberg-Jack symmetric functions. Adv. Math., 130:33-
102.
Kadell, K.
W.
J.
(1998).
A
simple proof of an Aomoto-type extension of Gustafson's
Askey-Wilson Selberg q-integral. Methods Appl. Anal., 5:125-142.
Kadell, K.
W.
J.
(2000a). A Dyson constant term orthogonality relation.
J.
Combin.
Theory Ser. A, 89(2):291-297.
Kadell, K.
W.
J.
(2000b). The Schur functions for partitions with complex parts. In
q-series from a contemporary perspective (South Hadley, MA,
1998),
volume 254
of Contemp. Math., pages 247-270. Amer. Math. Soc., Providence,
RI.
Kadell, K.
W.
J.
(2003). Selberg sums for the Sahi-Knop polynomials. Preprint.
Kaneko,
J.
(1993). Selberg integrals and hypergeometric series associated with Jack
polynomials. SIA M J. Math. Anal., 24:1086-1110.
Kaneko,
J.
(1996). q-Selberg integrals and Macdonald polynomials. Ann. Sci. dcole
Norm. Sup., 29(4):583-637.
Kaneko,
J.
(1998). A summation theorem for Macdonald polynomials. Ramanujan
J., 2:379-386.
Kendall, M.
G.
and Stuart, A. (1973). The Advanced Theory of Statistics, volume 2.
Hafner, New York.
Knop,
F.
and Sahi, S. (1997). A recursion and a combinatorial formula for Jack
polynomials. Invent. Math., 128:9-22.
Koekoek,
R.
and Swarttouw,
R.
F.
(1994). The Askey-scheme of hypergeometric or-
thogonal polynomials and its q-analogue. Faculty TWI 94-05, Delft University of
Technology. Electronic version: http: //aw
.
twi
.
tudelf
t
.nl/"koekoek/askey/.
Koornwinder, T. (1995). Jacobi polynomials of type bc, Jack polynomials, limit tran-
sitions and
o(oo).
Contemp. Math., 190:283-286.
Macdonald,
I.
G.
(1982). Some conjectures for root systems and finite reflection
groups. SIAM J. Math. Anal., 13:988-1007.

Little q-Jacobi Functions
337
Macdonald,
I.
G.
(1992). Schur functions: theme and variations. In Sdminaire Lotharingien
de Combinatoire, (Saint-Nabor, 1992), volume 498 of Publ. Inst. Rech. Math. Av.,
pages 5-39. Univ. Louis Pasteur, Strasbourg.
Macdonald, I.
G.
(1995). Symmetric Functions and Hall Polynomials. Oxford Univ.
Press, Oxford, 2nd edition.
Macdonald,
I.
G.
(1998). Symmetric Functions and Orthogonal Polynomials, vol-
ume 12 of Univ. Lecture Ser. Amer. Math. Soc., Providence,
RI.
Mehta, M. L. (1967). Random Matrices and the Statistical Theory of Energy Levels.
Academic Press, New York.
Morris, 11,
W.
G.
(1982). Constant term identities for finite and afine root systems:
conjectures and theorems. PhD thesis, University of Wisconsin-Madison.
Opdam, E. (1989). Some applications of hypergeometric shift operators. Invent. Math.,
98:l-18.
Opdam, E. (1995). Harmonic analysis for certain representations of graded Hecke
algebras. Acta Math., 175:75-121.
Opdam, E. M. (1988a). Root systems and hypergeometric functions 111. Compositio
Math., 67:21-49.
Opdam, E. M. (198813). Root systems and hypergeometric functions IV. Compositio
Math., 67:191-209.
Proctor,
R.
(1989). Equivalence of the combinatorial and the classical definitions of
the Schur functions.
J.
Combin. Theory Ser. A, 51:135-137.
Rahman, M. and Suslov, S. K. (1994a). Barnes and Ramanujan-type integrals on the
q-linear lattice. SIAM
J.
Math. Anal., 25:1002-1022.
Rahman, M. and Suslov, S. K. (1994b). The Pearson equation and the beta integral.
SIAM
J.
Math. Anal., 25:646-693.
Rahman, M. and Suslov, S. K. (1996). A unified approach to the summation and inte-
gration formulas for q-hypergeometric functions,
I.
J.
Statist. Plan. Infer., 54:lOl-
118.
Rahman,
M.
and Suslov, S.
K.
(1998a). A unified approach to the summation and inte-
gration formulas for q-hypergeometric functions, 11. Methods Appl. Anal., 5(4):399-
412.
Rahman, M. and Suslov, S.
K.
(199813). A unified approach to the summation and
integration formulas for q-hypergeometric functions, 111. Methods Appl. Anal.,
5(4):413-424.
Richards, D. S. P. (1989). Analogs and extensions of Selberg's integral. In q-series and
Partitions (Minneapolis,
MN,
1988), volume 18 of IMA Vol. Math. Appl., pages
109-137. Springer, New York.
Rogers, L.
J.
(1916). On two theorems of combinatory analysis and some allied iden-
tities. Proc. London Math. Soc., 16(2):315-336.
Rogers, L.
J.
(1983). On a three-fold symmetry in the elements of Heine's series. Proc.
London Math. Soc., 24:171-191.
Sahi, S. (1994). The spectrum of certain invariant differential operators associated to
a Hermitian symmetric space. In Brylinski, J.-L., Brylinski,
R.,
Guillemin,
V.,
and
Kac,
V.,
editors, Lie Theory and Geometry in Honor of Bertram Kostant, volume
123 of Progr. Math., pages 569-576. Birkhauser, Boston, Mass.
Sahi, S. and Knop,
F.
(1996). Difference equations and symmetric polynomials defined
by their zeroes. Internat. Math. Res. Notices, 10:473-486.

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Sears,
D.
B.
(1951).
Transformations of basic hypergeometric functions of special type.
Proc. London Math. Soc.,
53(2) :467-483.
Selberg,
A.
(1944).
Bemerkninger om et multipelt integral. Norsk Mat. Tidsskr.,
26:71-78.
Stanley, R.
P.
(1989).
Some combinatorial properties of Jack symmetric functions.
Adv. Math.,
77:76-115.
Starcher,
G.
W.
(1930).
On identities arising from solutions of q-difference equations
and some interpretations in number theory. Amer.
J.
Math.,
53:801-816.
Stembridge,
J.
(1988).
A short proof of Macdonald's root-system conjecture for the
root systems of type a. Proc. Amer. Math. Soc.,
102:777-786.
Suslov, S.
K.
(1998).
Multiparameter Ramanujan-type q-beta integrals. Ramanujan
J.,
2:351-369.
Szego,
G.
(1939).
Orthogonal Polynomials, volume
23
of Colloquium Publications.
Amer. Math. Soc.
Vinet,
L.
and Lapointe, L.
(1995).
A Rodriguez formula for the Jack polynomials and
the Macdonald-Stanley conjecture. Internat. Math. Res. Notices,
9:419-424.
Zeilberger,
D.
(1988).
A
unified approach to Macdonald's root-system conjectures.
SIAM
J.
Math. Anal.,
19:987-1013.
Zeilberger,
D.
(1989/90).
A Stembridge-Stanton style elementary proof of the Habsieger-
Kadell q-Morris identity. Discrete Math.,
79:313-322.
A SECOND ADDITION FORMULA FOR
CONTINUOUS Q-ULTRASPHERICAL
POLYNOMIALS
Tom
H.
Koornwinder
Korteweg-de Vries Institute
University of Amsterdam
Plantage Muidergracht
24
1018
TV Amsterdam
THE NETHER LANDS
thk@science.uva.nl
Abstract
This paper provides the details of Remark 5.4 in the author's paper
L'Askey-Wilson polynomials as zonal spherical functions on the
SU(2)
quantum group," SIAM
J.
Math. Anal.
24
(1993), 795-813. In formula
(5.9) of the 1993 paper a two-parameter class of Askey-Wilson polynomi-
als was expanded as a finite Fourier series with a product of two 3cpz's as
Fourier coefficients. The proof given there used the quantum group in-
terpretation. Here this identity will be generalized to a 3-parameter class
of Askey-Wilson polynomials being expanded in terms of continuous q-
ultraspherical polynomials with a product of two zcpz's as coefficients,
and an analytic proof will be given for it. Then Gegenbauer's addition
formula for ultraspherical polynomials and Rahman's addition formula
for q-Bessel functions will be obtained as limit cases. This q-analogue of
Gegenbauer's addition formula is quite different from the addition for-
mula for continuous q-ultraspherical polynomials obtained by Rahman
and Verma in 1986. Furthermore, the functions occurring as factors in
the expansion coefficients will be interpreted as a special case of a sys-
tem of biorthogonal rational functions with respect to the Askey-Roy
q-beta measure. A degenerate case of this biorthogonality are Pastro's
biorthogonal polynomials associated with the Stieltjes-Wigert polyno-
mials.
O
2005
Springer Science+Business Media, Inc.
340
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
1.
Introduction
Rahman and Verma (Rahman and Verma, 1986) obtained the follow-
ing addition formula for continuous q-ultraspherical polynomials:
1 1
pn (cos 8; a, aqi
,
-a, -aqi
I
q)
The formula is here written in the form given in (Gasper and Rahman,
1990, Exercise 8.11). Use (Gasper and Rahman, 1990) also for notation
of (q-)hypergeometric functions and (q-)shifted factorials. Throughout
it is supposed that 0
<
q
<
1.
Formula (1.1) is given in terms of Askey- Wilson polynomials (see
(Askey and Wilson, 1985) or (Gasper and Rahman, 1990, 57.5)):
P,(COS
8; a,
b,
c, d
I
q)
:=
a-n (ab, ac, ad; q), r,(cos 8; a,
b,
c, d
I
q)
(n
E
&o)
(1.2)
(symmetric in a,
b,
c, d), where
1
The continuous q-ultraspherical polynomials are the special case
b
=
aqs
,
1
c
=
-a, d
=
-aqs of the Askey-Wilson polynomials, often notated
as
follows (see (Gasper and Rahman, 1990, (7.4.14))):
13 13
A further specialization to a
=
i,
i.e., (a, b, c, d)
=
(a,
a,
-a,
-T),
yields
the continuous q-Legendre polynomials. For this case a
=
Koelink
was able to give two different poofs of the addition formula (1.1) from
A
second addition formula for continuous q-ultraspherical polynomials 341
a quantum group interpretation on SUq(2), see (Koelink, 1994) and
(Koelink, 1997).
If
a
is replaced by
qfX
in (1.1) and the limit is taken for q
I
1,
then a version of the addition formula for ultraspherical polynomials is
obtained:
"
22k (2X
+
2k
-
1) (n
-
k)! (A):
c;
(cos 0)
=
C
k=O
(2X
-
l)n+k+l
k
X+k
2
x
(sin
cp)
c:::
(cos cp) (sin
$)
Cn-* (cos
$)
Ct-'
Here ultraspherical polynomials are defined by
cos 0
-
cos
cp
cos
$
sin
cp
sin
$
(1.5)
By elementary substitution the addition formula (1.5) transforms into
the familiar addition formula for ultraspherical polynomials:
"
22k (2X
+
2k
-
1)
(n
-
k)! (A):
~~(coscpcos~
+
sincpsin$cos0)
=
C
k=O (2X
-
l)n+k+l
k
X+k A-112
~(sincp)~~~f~(coscp)(sin~)
Cn-,(cos$)Ck
(cos~),
(1.7)
see (Erdelyi et al., 1953, 10.9(34)), but watch out for the misprint 2m
which should be 22m; see also the references given in (Askey, 1975, Lec-
ture 4). For the removable singularity at
X
=
in (1.7) observe that
k
lim
-
cL-f
(cos~)
=
cos(kq (k
>
01;
~t-i(cos~)
=
I.
A+'
2X
-
1
2
(1.8)
An elementary transformation comparable to the passage from (1.5)
to (1.7) cannot be performed on the q-level. It is a generally observed
phenomenon in q-theory that, for a classical formula involving a param-
eter dependent function with an argument transformed by a parameter
dependent transformation,
a
possible q-analogue has the transformation
parameters occurring in the function parameters. Compare for instance
the pk factor in
(1.1)
with the
c:-+
factor in (1.5).
In (Koornwinder, 1993, (5.9)) I obtained the following formula as a
spin-off of the interpretation of certain Askey-Wilson polynomials as
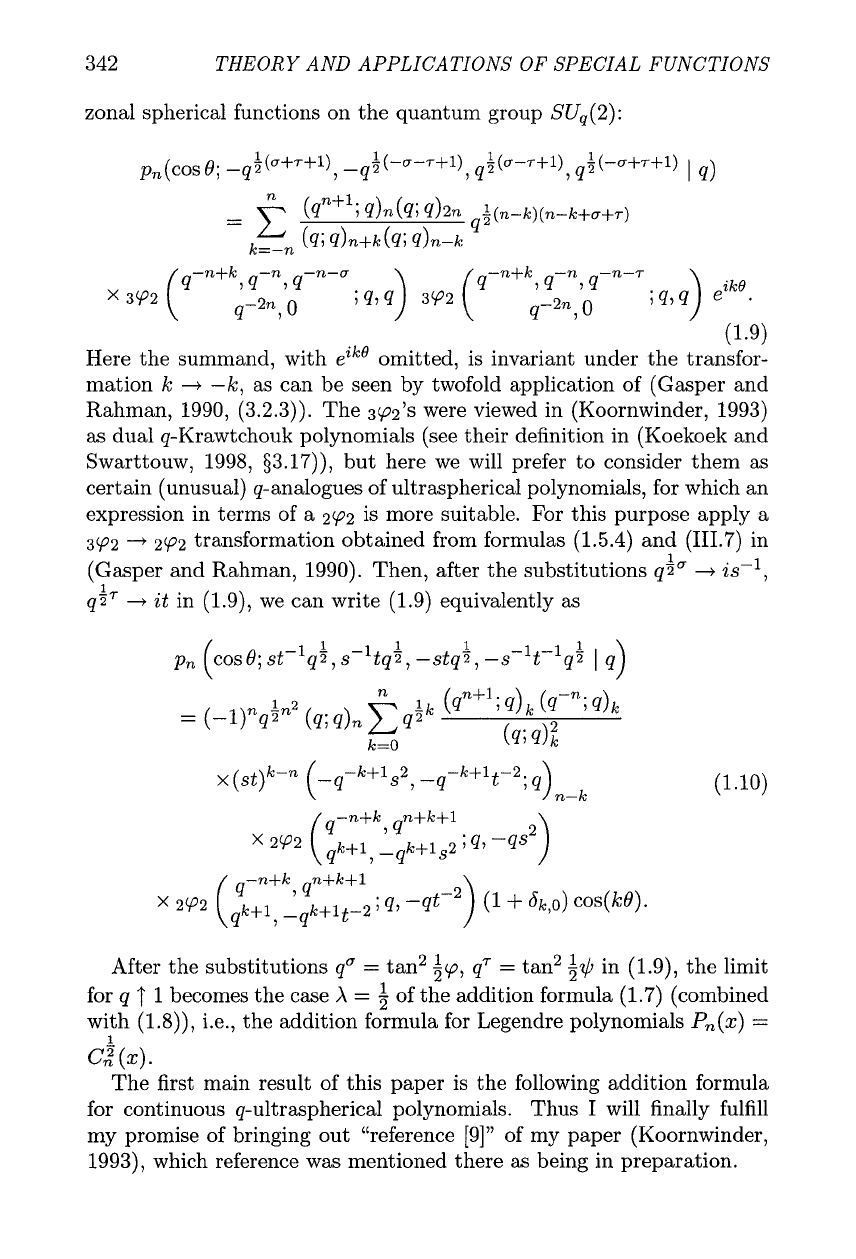
342
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
zonal spherical functions on the quantum group
SUq(2):
(1.9)
Here the summand, with
eike
omitted, is invariant under the transfor-
mation k
+
-k, as can be seen by twofold application of (Gasper and
Rahman, 1990, (3.2.3)). The
392)~
were viewed in (Koornwinder, 1993)
as dual q-Krawtchouk polynomials (see their definition in (Koekoek and
Swarttouw, 1998, §3.17)), but here we will prefer to consider them
as
certain (unusual) q-analogues of ultraspherical polynomials, for which an
expression in terms of a
292
is more suitable. For this purpose apply a
392
-f
292
transformation obtained from formulas (1.5.4) and (111.7) in
(Gasper and Rahman, 1990). Then, after the substitutions qiu
+
is-',
1
qiT
+
it in (1.9)) we can write (1.9) equivalently as
After the substitutions qu
=
tan2
$9,
qT
=
tan2
$$J
in (1.9)) the limit
for q
1
becomes the case
X
=
$
of the addition formula (1.7) (combined
with (IS)), i.e., the addition formula for Legendre polynomials Pn(x)
=
c"$
(5).
The first main result of this paper is the following addition formula
for continuous q-ultraspherical polynomials. Thus I will finally fulfill
my promise of bringing out "reference [9]" of my paper (Koornwinder,
1993), which reference was mentioned there as being in preparation.
