Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Little

q-

Jacobi Functions

323

Let

n,

a

and

b

be fixed with

n

2

0, a

#

0,

b

#

0

and

alb

not an

integer power of q. Since

(6.3)

gives

b

=

cqx,

we see taking

x

=

m

in the

orthogonality relation

(6.5)

that

Using the symmetry

we see that

(6.10)

becomes

We have

Sn(a,

b,

0)

=

(ab)".

Observe that

Sn(a,

b,

c)

has degree at most

2n

as a polynomial in

c

and

that

(6. lo), (6.12)

and

(6.13)

give the values, in agreement with

(6.9),

of

Sn(a,

b,

c)

at

2n

+

1

distinct values of

c.

We may remove the restrictions

on

a

and

b

by successively considering

Sn(a,

b,

c)

as a polynomial in

a

and

b.

Thus the orthogonality of the little q-Jacobi polynomials, which

is given by

(6.5),

implies the polynomial formulation

(6.9)

of the q-

Saalschiitz sum

(1.2).

Hence the orthogonality of the little q-Jacobi

polynomials is equivalent to the q-Saalschiitz sum

(1.2).

We may use

(2.11)

to reverse the q-Chu-Vandermonde sum

(1.3).

The

result is (Gasper and Rahman,

1990, (1.5.3))

Setting

y

=

n

-

x

-

1

in

(6.2)

and taking

a

=

qa+" and

b

=

q"

(6.15)

in the reversed q-Chu-Vandermonde sum

(6.14),

we obtain

Using the inverse q"

=

b

and qx

=

alb

of

(6.16),

we see that

(6.17)

ib

equivalent to the reversed q-Chu-Vandermonde sum

(6.14)

and hence is

equivalent to the q-Chu-Vandermonde sum

(1.3).

324

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Since 0

<

!

<

n

-

1

implies that (q-e; q),

=

0, we have the orthogo-

nality relation

Since we may use any basis for the polynomials in

t

with degree less than

or equal to

n

-

1,

we see by (6.18) that the little q-Jacobi polynomials

are orthogonal on (0,l) with respect to the q-beta integral (1.17).

Using (6.6) and (6.8) with

c

replaced by

b

to multiply the reversed

q-Chu-Vandermonde sum (6.14) by (b; q),, we obtain

(6.18)

which is a polynomial formulation of the reversed q-Chu-Vandermonde

sum (6.14).

Let

n

and a be fixed with

n

>

0,

a

#

0 and alb not an integer power

of q. Since (6.16) gives

a

=

bqx, we see taking x

=

e

in the orthogonality

relation (6.18) that

We have

[bn]

T,(a,

b)

=

(-l), q(;). (6.20)

Observe that (6.20) gives the values, in agreement with (6. lg), of

Tn

(a, b)

at

n

distinct values of

b

and that (6.21) gives the coefficient of the leading

term of Tn(a, b) as a polynomial in b. We may remove the restrictions on

a

by considering Tn(a, b)

as

a polynomial in a. Thus the orthogonality

of the little q-Jacobi polynomials, which is given by (6.18), implies the

polynomial formulation (6.19) of the reversed q-Chu-Vandermonde sum

(6.13) and hence implies the q-Chu-Vandermonde sum (1.3). Hence the

orthogonality of the little q-Jacobi polynomials is equivalent to the q-

Chu-Vandermonde sum (1.3).

Observe that the q-Chu-Vandermonde sum (1.3) is equivalent to and

hence implies the orthogonality of the little q-Jacobi polynomials, which

is equivalent to and hence implies the q-Saalschutz sum (1.2).

7.

The little

q-

Jacobi functions

In this section, we extend the little q-Jacobi polynomials naturally to

the little q-Jacobi functions of complex order. We show that the nonter-

minating q-Saalschutz (1 .l3) and q-Chu-Vandermonde (1.12) sums are

equivalent to the evaluations of the moments

I;'@(x,

0) and

I;'@(X,

n

-

Little q-Jacobi Functions 325

x-I), respectively, and, using the Liouville-Ismail argument (Hille, 1973,

Theorem 8.2.2), (Ismail, 1977), to two orthogonality relations. We show

that (1.12) implies (1.13).

Let

n,

p

be complex, let x

+

1

have positive real part, and let

m

2

0,

k'

2

0 be nonnegative integers. Let 0

#

lql

<

1,

t

#

0

with fixed

natural logarithms of q and t. Observe that (1.6) and (1.14) extend

the q-Pockhammer symbol (a; q),

=

(a; q),/ (aqn; q), to complex

n

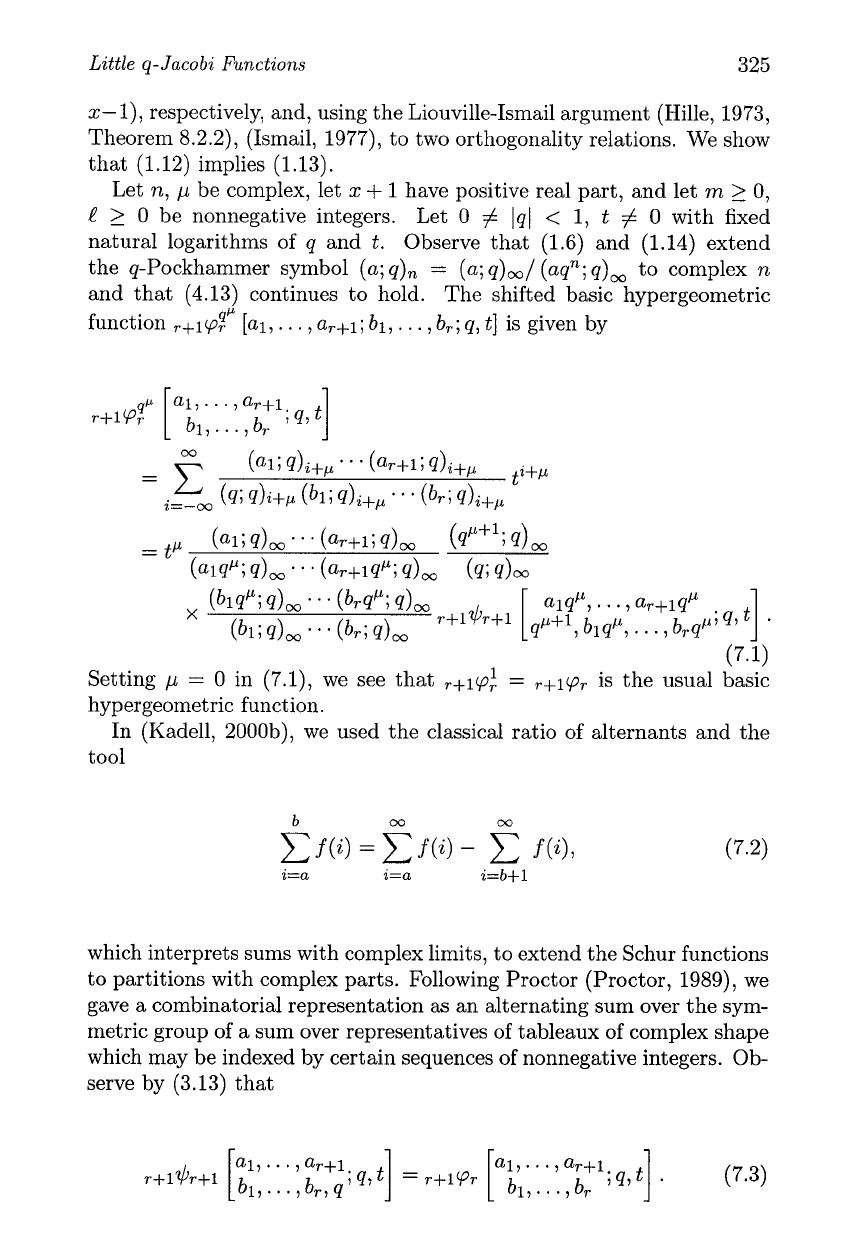
and that (4.13) continues to hold. The shifted basic hypergeometric

9'l

function T+lyT [al,

.

. .

,

aT+l;

bl,

.

,

br;

q, t] is given by

=

tp

(al; q),

.

(ar+l; q), (qp+l; 4)

,

(alp; q),

.

-

(aT+iqY q),

(q; q)~

(74

Setting

p

=

0

in (7.1)) we see that T+lyi

=

T+l(PT

is the usual basic

hypergeometric function.

In (Kadell, 2000b), we used the classical ratio of alternants and the

tool

which interprets sums with complex limits, to extend the Schur functions

to partitions with complex parts. Following Proctor (Proctor, 1989), we

gave a combinatorial representation as an alternating sum over the sym-

metric group of a sum over representatives of tableaux of complex shape

which may be indexed by certain sequences of nonnegative integers. Ob-

serve by (3.13) that

326

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Since (7.1) and (7.3) give

it is natural to extend the little q-Jacobi polynomials to functions of

complex order

n

by

1-n-a:

n+p

x

291

[q

q22

;

q,tq

1

I

(7.5)

which is a difference of shifted basic hypergeometric functions. We may

avoid poles by assuming that

n

+

a

-

1,

-n

-

P

and

-a

are not non-

negative integers.

Using (1.16), (1.17) and (6.1), we have

Little

q-

Jacobi Functions

Combining our results

(6.2),

(7.5) and (7.6), we obtain

Setting

y

=

0

in (7.7), we have

(9; q)m (qP+.+l.

1-n-a

n+p

X

lq),

392

[q

(qX+l; 41, (qP; q),

q2-a1

qp+x+l

;9,

Q

.

Taking

+

I

(7.8)

in the nonterminating q-Saalschiitz sum (1.13) and substituting into

(7.8), we obtain

Using the inverse qa

=

el qx

=

c/e, qfl

=

abqle and qn

=

l/a of (7.9),

we see that (7.10) is equivalent to the nonterminating q-Saalschiitz sum

(1.13).

Setting

y

=

n

-

x

-

1

in (7.7),

we

have

328

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Taking

a

=

qWn,

b

=

q"+" and c

=

q"

(7.12)

in the nonterminating q-Chu-Vandermonde sum (1.12) and substituting

into (7.11), we obtain

I:@(x,n

-

x

-

1)

=

(4; 4)m

(P;

(!Pn+%;

q)

00

(qa+P+n-l

;

dm

(ql-n-a;

q),

(qP+n-x-l.

,

dm

(qX+l;

41, (qa+"; 41,

'

(7.13)

Using the inverse qa

=

C,

qx

=

b/c and qn

=

l/a of (7.12), we see

that (7.13) is equivalent to the nonterminating q-Chu-Vandermonde sum

(1.12).

Observe that the evaluations (7.10) and (7.13) of the moments I:@(x, 0)

and

I,"@

(z,

n

-

a:

-

1) give the orthogonality relations

n

-

x

-

1,

a

-

1

or

-

n

-

a

-

,8

-

x is a nonnegative integer

(7.14)

+

I,"*P(X,O)

=

0

and

n

-

x

-

1,

a

-

1

or

-

n

-

a

-

p

+

1

is a nonnegative integer

(7.15)

+

I:~P(x,n-x- 1)

=O,

respectively.

We may rearrange the nonterminating q-Saalschiitz sum (1.13) as

~ ~

x

(q2+i/e; q)_ (abcq2+'/e2; q),

=

(e; q), (q/e; 4)w (abqle; q)w (a+; q), (bcqle; q),.

(7.16)

Since (7.9) gives elacq

=

qn-"-l, e/q

=

q"-l and elbcq

=

q-n-"-P-x,

we see that the orthogonality relation (7.14) gives

m

>

0

S

(a, b, c; acqmf

l)

(7.17)

=

S

(a,

b,

c;

*"+I)

=

S

(a, b, c; bcqm+l)

=

0.

Little q-Jacobi Functions 329

Observe that the functions on both sides of (7.16) satisfy

and they are both symmetric in a,

b

and c. Hence by (7.17), we have

m

2

0

+

S

(a,

b,

c; abqm+')

=

S

(a,

b,

c; ql-")

=

0.

(7.19)

Observe that (7.17) and (7.19) express the orthogonality relation (7.14)

in terms of the function S(a,

b,

c; e) and identify the factors on the right

side of (7.16).

We may rearrange the nonterminating q-Chu-Vandermonde sum (1.12)

as

=

(c; q)00 (dc; q)00 (abqlc;

dm.

(7.20)

Since (7.12) gives clabq

=

qnRX-' and c/q

=

qff-l, we see that the

orthogonality relation (7.15) gives

e

o

+

T

(a,

b;

abqe+'

=

I

a,

b;

qe+'

=

0.

)()

(7.21)

Observe that the functions on both sides of (7.21) satisfy

Hence by (7.21), we have

Observe that (7.21) and (7.23) express the orthogonality relation (7.15)

in terms of the function 7(a, b; c) and identify the factors on the right

side of (7.20).

Since

S(a,

b,

0; c)

=

7(a, b; c),

(7.24)

we see that the orthogonality relation (7.14), which is given by (7.17)

and (7.19), implies the orthogonality relation (7.15), which is given by

(7.21) and (7.23).

330

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

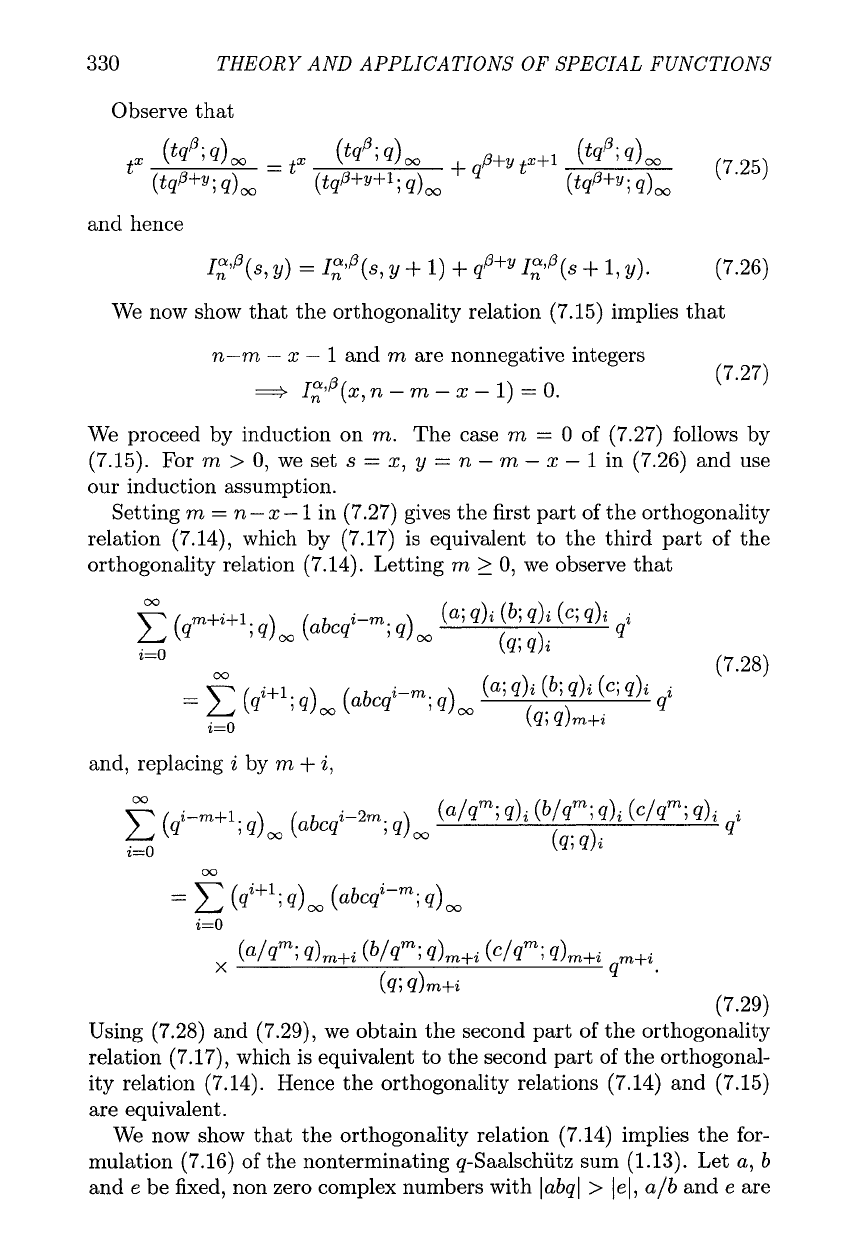
Observe that

and hence

We now show that the orthogonality relation

(7.15)

implies that

n-m

-

x

-

1

and

m

are nonnegative integers

(7.27)

I;@(X,

n

-

m

-

x

-

1)

=

0.

We proceed by induction on

m.

The case

m

=

0

of

(7.27)

follows by

(7.15).

For

m

>

0,

we set

s

=

x,

y

=

n-m-

x

-

1

in

(7.26)

and use

our induction assumption.

Setting

m

=

n-x- 1

in

(7.27)

gives the first part of the orthogonality

relation

(7.14),

which by

(7.17)

is equivalent to the third part of the

orthogonality relation

(7.14).

Letting

m

>

0,

we observe that

and, replacing

i

by

m

+

i,

. .

(7.29)

Using

(7.28)

and

(7.29),

we obtain the second part of the orthogonality

relation

(7.17),

which is equivalent to the second part of the orthogonal-

ity relation

(7.14).

Hence the orthogonality relations

(7.14)

and

(7.15)

are equivalent.

We now show that the orthogonality relation

(7.14)

implies the for-

mulation

(7.16)

of the nonterminating q-Saalschiitz sum

(1.13).

Let

a,

b

and

e

be fixed, non zero complex numbers with

labql

>

lei, alb

and

e

are

Little q-Jacobi Functions 331

not integer powers of

q,

and

abqle

is not the reciprocal of a nonnegative

integer power of

q.

Let

-

(7.30)

denote the sum on the left side of

(7.16)

divided by the product on the

right side of

(7.16).

Observe that the orthogonality relation

(7.14)

implies

(7.17)

and

(7.19),

which identify the factors on the right side of

(7.16).

Since the function

on the right side of

(7.16)

has at most simple zeroes, we see that

f

is an

entire function of

a, b

and

c.

Observe that

f

(c)

is analytic and hence bounded on the disc

fll

=

{c

1

lac1

<

\el

and

Ibcl

<

]el).

(7.31)

Let

E

>

0

be given and set

and

I

bcql+"/e

-

1

I

for all

m

2

0)

.

(7.32)

Let

c

E

f12.

Hence

c

#

0 and we may write

cqZ

=

w

where z is an integer and

lql

5

IwI

<

1.

(7.33)

Using the identity

(2.11)

for reversing the q-Pockhammer symbol and

(4.13),

we see that

(xcq/e; q),

=

(xcqle; q)z (~cq'+~le),

(7.34)

=

(-xcqle)'

q(;)

(e/xw; q), (xwq/e),.

Using

(7.33)

and

(7.34),

we have

(cq/e; q), (abcqle),

-

(e/w; q), (wq/e; q), (elah; q)~ (abwqle; q),

-

(acqle; q), (bcqle; q),

(claw;

q), (awqle;

(e/bw; q)~ (bwqle; q),

'

(7.35)

332

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Observe by (7.31-33) that z is bounded below. Using (7.35) and

(7.36), we see that both of the ratios of products on the right side of

(7.30) are bounded

as

functions of c. Using (7.33) and the identity (2.11)

for reversing the q-Pockhammer symbol, we have

Using (7.37), (7.37) with c replaced by cq/e, and the fact that lelabql

<

1,

we see that 3(~2[a,

b,

c; e, abcqle; q, q] and

3992

[aqle, We, cq/e; q2/e,

abcq2/e2; q,

q]

are bounded as functions of c. Thus

f

(c) is bounded on

a2

Recall Liouville's theorem Hille (Hille, 1973, Theorem 8.2.2) that a

bounded entire function is constant. The proof uses the Laurent series

representation Hille (Hille, 1973, Theorem 8.1 .l)

where the coefficients are given by Hille (Hille, 1973, (8.1.2), (8.2.3))

1

an

=

-

dz,

and the contour

C,

=

{z

I

lz

-

col

=

r)

and c are inside a disc with center

co on which

f

is analytic. If we take

E

<

1

-

lql1I4, then for each co there

exists a sequence

{T,),,~

of radii with lim

rn

=

oo

and

C,,

G

R1

U

R2,

-

n400

n

2

1. Using (7.39), we have a0

=

f

(co) and an

=

0,

n

>

1. Hence

(7.38) gives

f

(c)

=

f

(co). Taking co

=

e/q, we have

f

(c)

=

f

(elq)

=

1,

as

required. We may remove the restrictions on a,

b

and e since

f

is

entire and hence continuous in each of these parameters.

We call this argument the Liouville-Ismail argument.

We have come full circle since the nonterminating q-Chu-Vandermonde

sum (1.12) implies in turn (7.13), (7.15), (7.14) and the formulation

(7.16) of the nonterminating q-Saalschiitz sum (1.13), which implies

(1.12).

8.

Conclusion

In this section, we conclude with some thoughts on the Hankel deter-

minant, the Rodriguez formula, the slinky rule for the Schur functions,

the q-Dyson polynomials, q-series identities, and the Vinet operator.

Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.