Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.

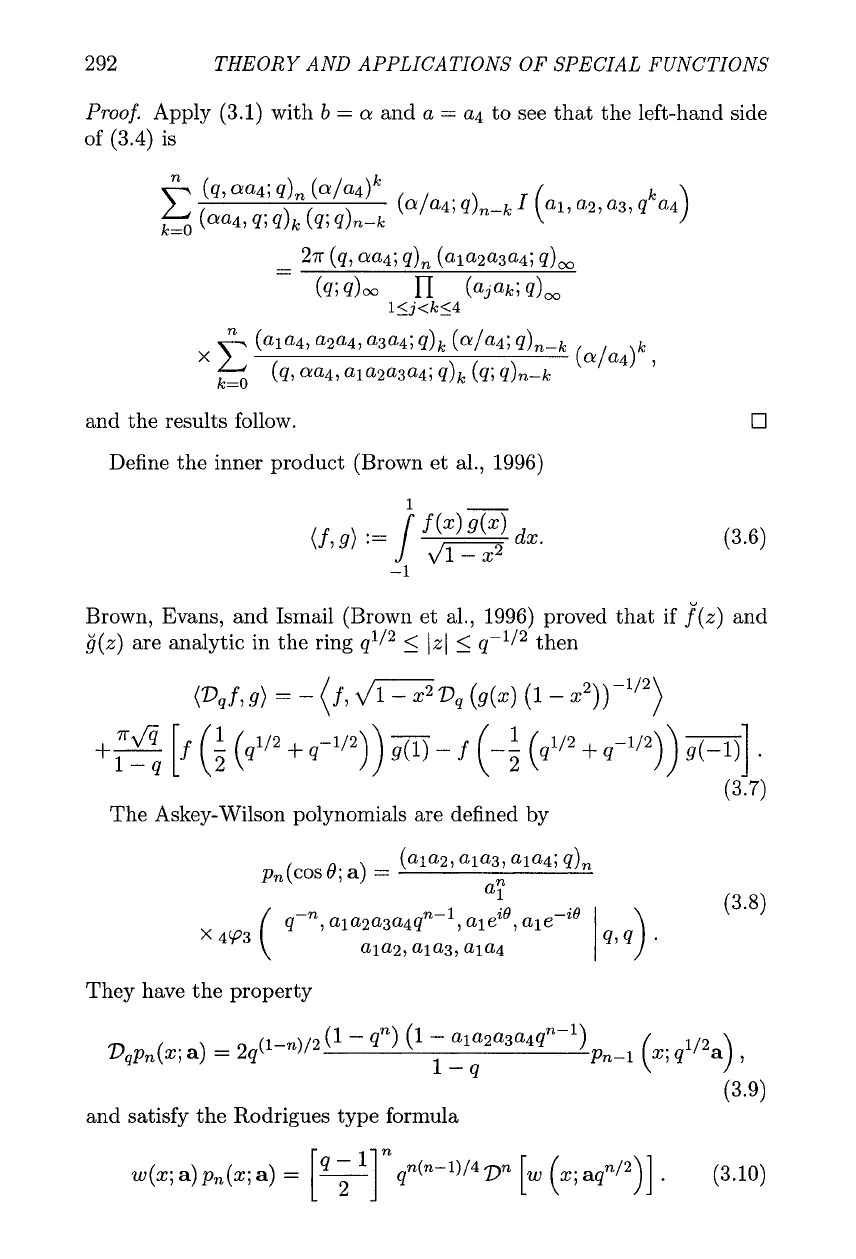
292

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Proof.

Apply (3.1) with

and

to see that the left-hand side

of (3.4) is

and the results follow.

Define the inner product (Brown et al., 1996)

Brown, Evans, and Ismail (Brown et al., 1996) proved that if f(z) and

g(z)

are analytic in the ring

q1I2

IzI

q-ll2

then

The Askey-Wilson polynomials are defined by

They have the property

and satisfy the Rodrigues type formula

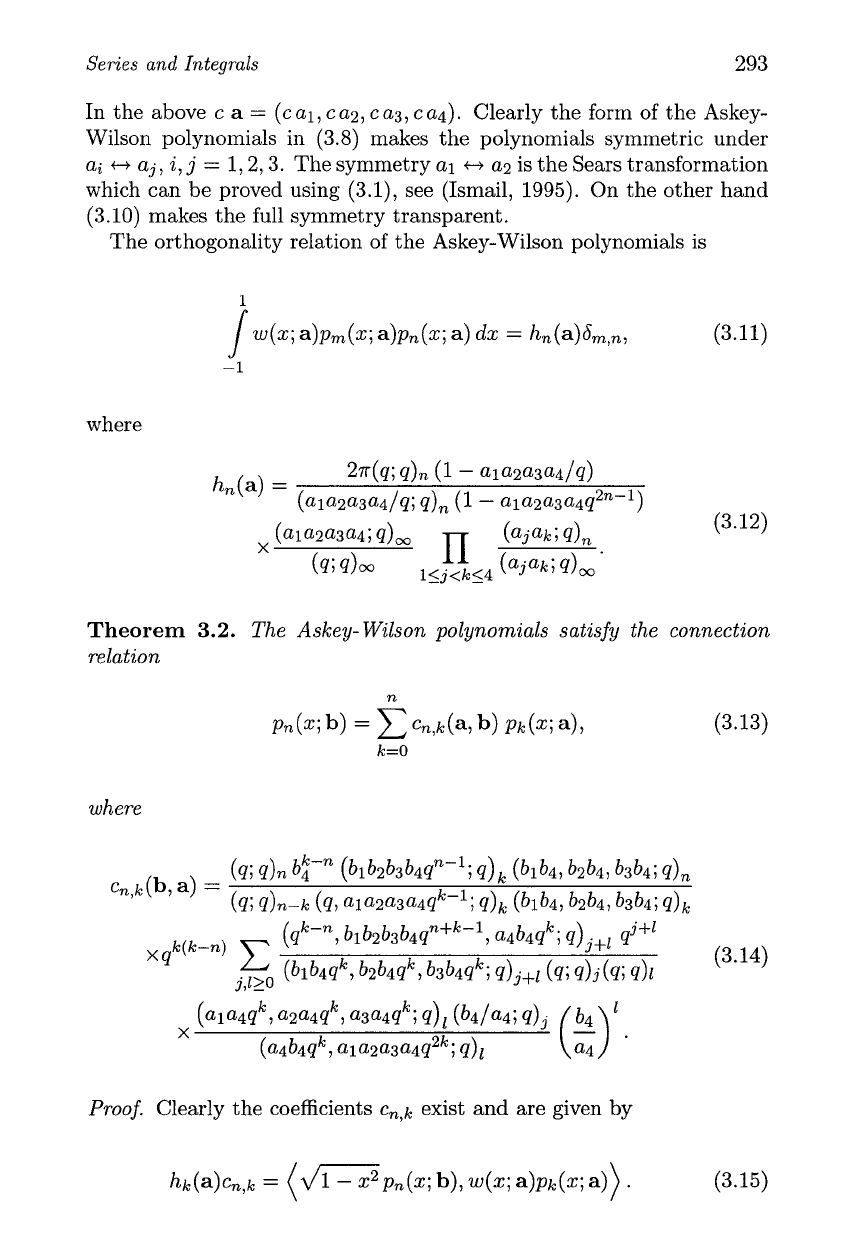
Series and Integrals 293

In the above

(c al, c a2, c a3, cad).

Clearly the form of the Askey-

Wilson polynomials in

(3.8)

makes the polynomials symmetric under

aj,

1,2,3.

The symmetry

is the Sears transformation

which can be proved using

(3.1),

see (Ismail,

1995).

On the other hand

(3.10)

makes the full symmetry transparent.

The orthogonality relation of the Askey-Wilson polynomials is

where

Theorem

3.2.

The Askey- Wilson polynomials satisfy the connection

relation

where

Proof.

Clearly the coefficients

cn,k

exist and are given by

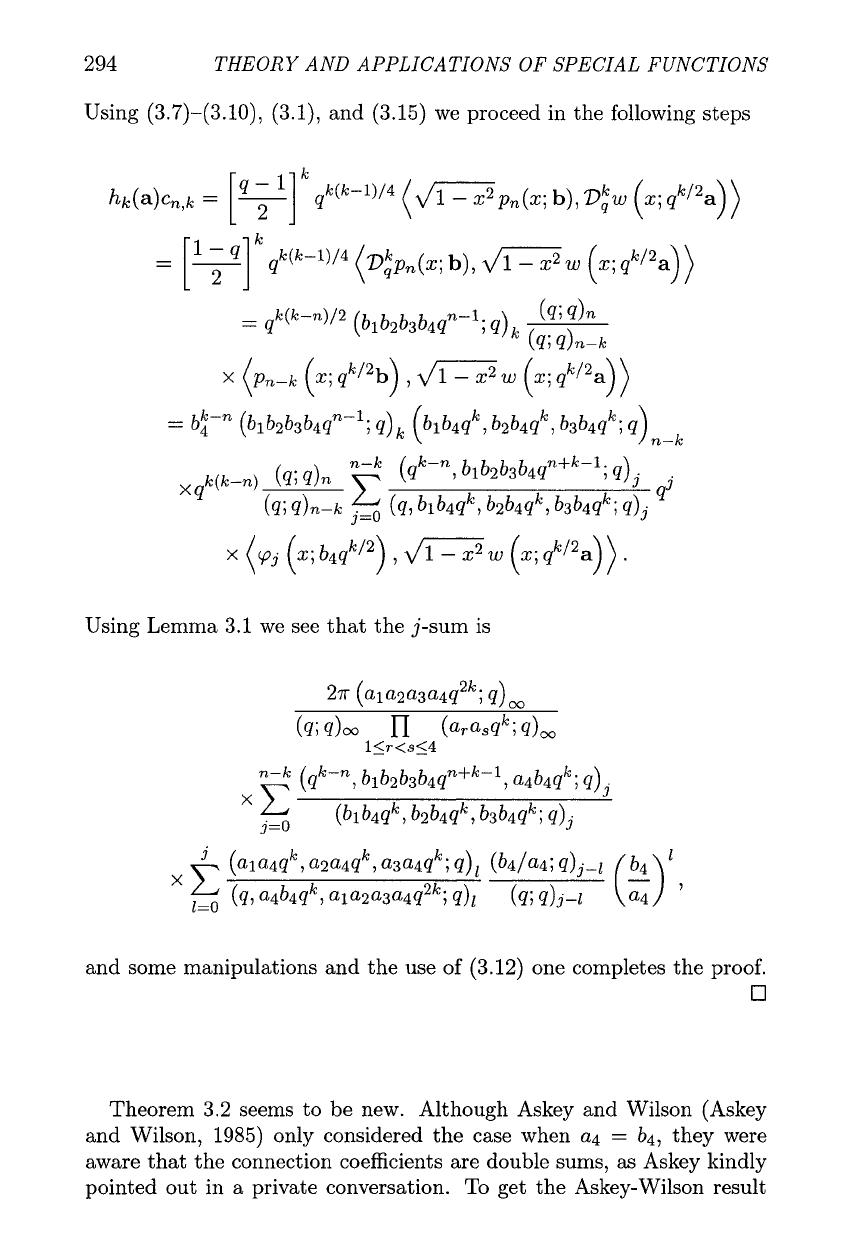
294

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Using

(3.7)-(3.10), (XI),

and

(3.15)

we proceed in the following steps

kl2b

J-w

x(pn-x(x;q

bt-" (blb2b3b4qn-l; q)k (blb4qk, b2hqk, b3b4qk; 4)

n-lc

(x; b4qkl2)

.\/=

(x; qkl2a))

Using Lemma

3.1

we see that the j-sum is

(ala4qk, a2a4qk, a3a4qk; qll (Wa4; q)j-l

(q, a4b4qk, ala2a3a4q21ci

(q; q)j-1

1=0

and some manipulations and the use of

(3.12)

one completes the proof.

Theorem

3.2

seems to be new. Although Askey and Wilson (Askey

and Wilson,

1985)

only considered the case when

b4,

they were

aware that the connection coefficients are double sums,

Askey kindly

pointed out in a private conversation. To get the Askey-Wilson result

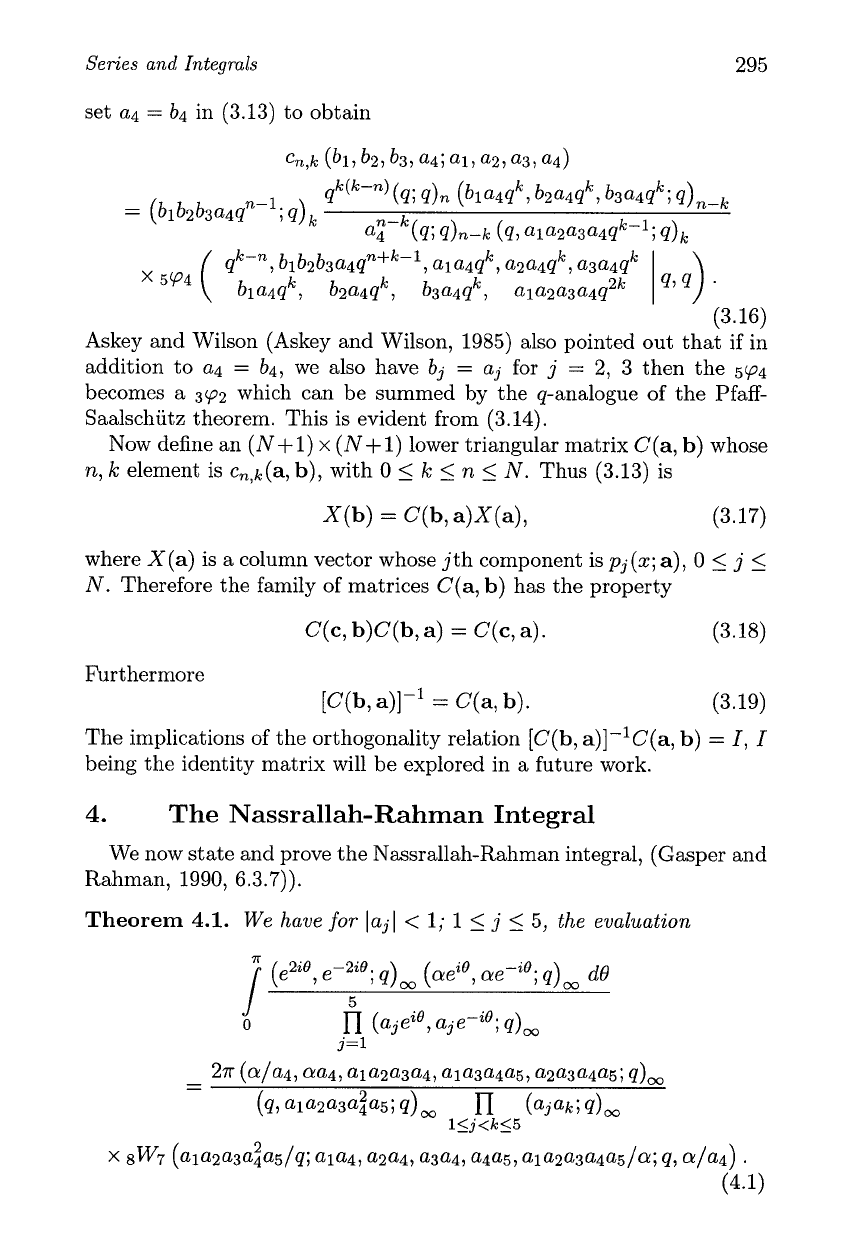
Series and Integrals

set

(3.13)

to obtain

q"k-n)(q; q)n (bla4qk,b2a4qk, b3a4qk; q)n-k

(blkha4qn-'; q)k

a~-~((q; q)n-k

(Q,

ala2a3a4qk-1; q)k

594

(

qk-n,

blb2b3aqqnf

ala4qk, a2a4qk, a3a4qk

ha4qk, b2a4qk, b3a4qk, ala2a3a4q2k

1w).

(3.16)

Askey and Wilson (Askey and Wilson,

1985)

also pointed out that if in

addition to

b4,

we also have

for

then the

594

becomes a

392

which can be summed by the q-analogue of the Pfaff-

Saalschiitz theorem. This is evident from

(3.14).

Now define an

lower triangular matrix

C(a,

whose

element is

cn,k(a,

b),

with

Thus

(3.13)

where

X(a)

is a column vector whose jth component is

pj(x;

a),

Therefore the family of matrices

C(a,

has the property

C(c, b)C(b,

C(c,

a).

(3.18)

Furthermore

[C(b,

a)]-'

C(a,

b).

(3.19)

The implications of the orthogonality relation

[C(b,

a)]-%'(a,

I, I

being the identity matrix will be explored in a future work.

The Nassrallah-Rahman Integral

We now state and prove the Nassrallah-Rahman integral, (Gasper and

Rahman,

1990, 6.3.7)).

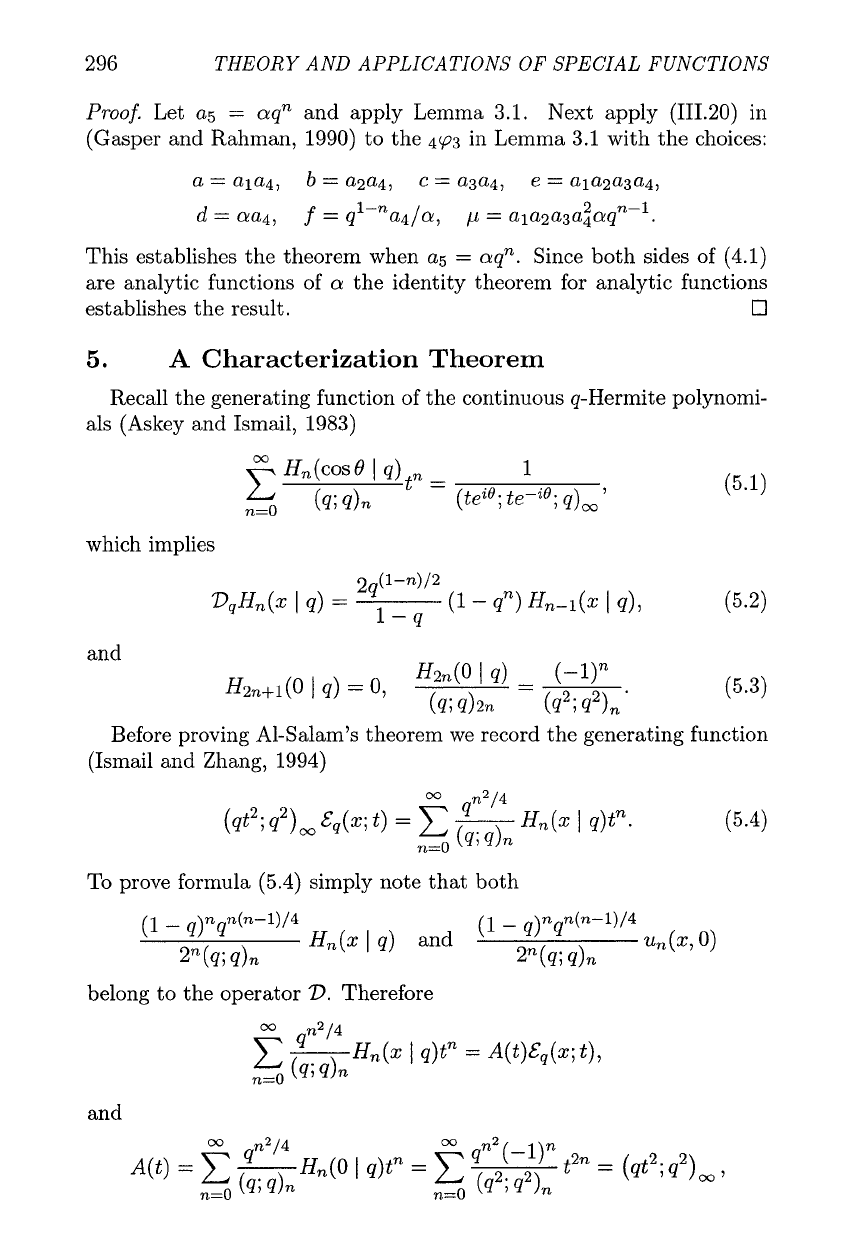
Theorem

4.1.

We have for laj/

1; 1

the evaluation

296

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Proof.

Let

aqn and apply Lemma 3.1. Next apply (111.20) in

(Gasper and Rahman, 1990) to the

493

in Lemma 3.1 with the choices:

This establishes the theorem when

aqn. Since both sides of (4.1)

are analytic functions of

the identity theorem for analytic functions

establishes the result.

Characterization Theorem

Recall the generating function of the continuous q-Hermite polynomi-

als (Askey and Ismail, 1983)

which implies

and

Before proving Al-Salam's theorem we record the generating function

(Ismail and Zhang, 1994)

To prove formula (5.4) simply note that both

belong to the operator

Therefore

and

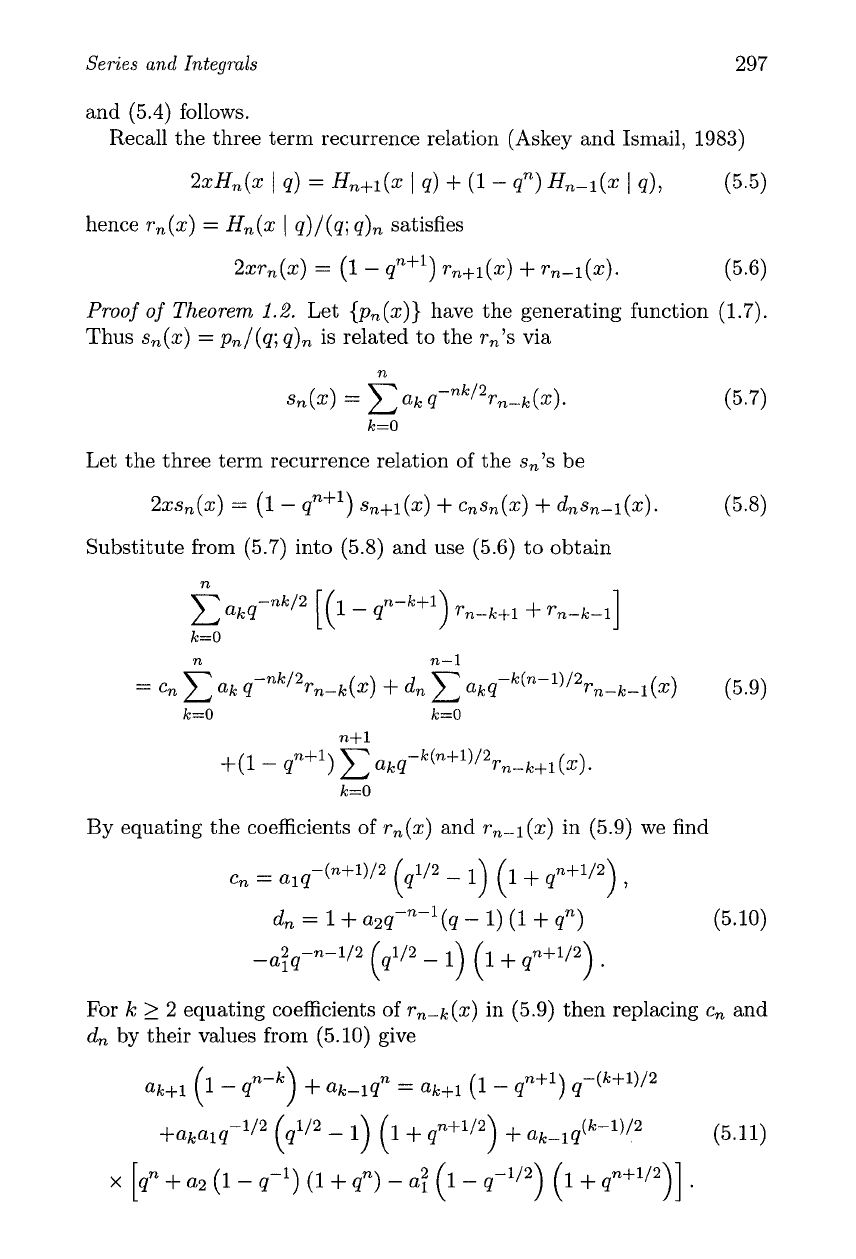
Series

and

Integrals

297

and

(5.4)

follows.

Recall the three term recurrence relation (Askey and Ismail,

1983)

2xHn

Hn+l

qn) Hn-1

q),

(5.5)

hence

rn(x)

Hn(x

q)/(q; q),

satisfies

2xrn

(2)

qnf

rn+~(x)

rn-l(x).

(5.6)

Proof of Theorem

1.2.

Let

{pn(x))

have the generating function

(1.7).

Thus

sn(x)

pn/(q; q),

is related to the

rn's

via

Let the three term recurrence relation of the sn's be

Substitute from

(5.7)

into

(5.8)

and use

(5.6)

to obtain

By equating the coefficients of

rn(x)

and

rn-l(x)

(5.9)

we find

For

equating coefficients of

rndk(x)

(5.9)

then replacing

and

by their values from

(5.10)

give

298

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Since the above equation must hold for all

3, the coefficients of qn

and the constant terms on both sides must be identical. This gives two

recurrence relations for ak, which must be compatible. If a1

a calculation proves ak

for all

3 and we conclude that pn(x)

Hn(x

q). When a1

but a2

then (5.11) shows that a2k+l

for

and

The consistence condition implies a2

q-l)

-1. Hence pn(x)

Hn(x

l/q). Through a change of variable the polynomials are orthog-

onal when q

on the imaginary axis (Askey, 1989). If ala2

the

compatibility condition leads to a contradiction, so this case does not

arise.

Dedication

It is very appropriate to dedicate this paper to our good friend Mizan

Rahman for his influence and seminal contributions to the subject of

q-series and for always being a friend who has always been there for us.

Acknowledgments

The authors gratefully acknowledge the page full of remarks made

by each of two referees who read the paper very carefully. The refer-

ees' comments improved both the presentation and accuracy of the final

version.

References

Al-Salam, W. A. (1995). Characterization of the Rogers q-Hermite polynomials. In-

ternat.

Math. Math. Sci., 18:641-647.

Andrews,

E.,

Askey, R. A., and Roy, R. (1999). Special Functions. Cambridge

University Press, Cambridge.

Askey, R.

(1989). Continuous q-Hermite polynomials when

1. In Stanton,

D.,

editor, q-Series and Partitions, IMA Volumes in Mathematics and Its Applications,

pages 151-158. Springer-Verlag, New York.

Askey, R. A. and Ismail, M. E.

(1983).

generalization of ultraspherical polyno-

mials. In ErdGs,

P.,

editor, Studies in Pure Mathematics, pages 55-78. Birkhauser,

Basel.

Askey,

and Wilson,

(1985). Some basic hypergeometric orthogonal polyno-

mials that generalize Jacobi polynomials. Memoirs Amer. Math. Soc., 54(319):iv+55.

Brown,

M., Evans, W.

D.,

and Ismail, M. E.

(1996). The Askey-Wilson polyno-

mials and q-Sturm-Liouville problems. Math. Proc. Cambridge Phil. Soc., 119: 1-16.

Cooper, S. (1996). The Askey-Wilson operator and the

69s

summation formula.

Preprint.

Series and Integrals

299

Gasper,

and Rahman,

(1990). Basic Hypergeometric Series. Cambridge Uni-

versity Press, Cambridge.

Ismail, M.

(1995). The Askey-Wilson operator and summation theorems. In

Ismail, M., Nashed,

Z., Zayed, A., and Ghaleb, A., editors, Mathematical Anal-

ysis, Wavelets, and Signal Processing, volume 190 of Contemporary Mathematics,

pages 171-178. American Mathematical Society, Providence.

Ismail, M.

(2001). An operator calculus for the Askey-Wilson operator. Annals

of Combinatorics, 5:333-348.

Ismail,

and Stanton,

(2003a). Applications of q-Taylor theorems.

Comp.

Appl. Math., 153:259-272.

Ismail, M.

and Stanton,

(2003b). q-Taylor theorems, polynomial expansions,

and interpolation of entire functions.

Approx. Theory, 123:125-146.

Ismail,

and Zhang,

(1994). Diagonalization of certain integral operators.

Advances

Math., 109:l-33.

Nassrallah,

and Rahman, M. (1985). Projection formulas, a reproducing kernel and

a generating function for q-Wilson polynomials. SIAM

Math. Anal., 16:186-197.

Rainville,

(1971). Special Functions. Chelsea Publishing Co., Bronx,

NY.

Rota, G.-C., Kahaner,

D.,

and Odlyzko, A. (1973). On the foundations of combina-

torial theory. VIII. Finite operator calculus.

Math. Anal. Appl., 42:684-760.

Suslov, S. K. (1997). Addition theorems for some q-exponential and trigonometric

functions. Methods and Applications of Analysis, 4:ll-32.

THE LITTLE Q-JACOB1 FUNCTIONS OF

COMPLEX ORDER

Kevin

Kadell

Department of Mathematics and Statistics

Arizona State University

Tempe,

85287-1804

Abstract

We use Ismail's argument and an elementary combinatorial identity to

prove the q-binomial theorem, the symmetry of the Rogers-Fine func-

tion, Ramanujan's

l$Jl

sum, and Heine's q-Gauss sum and give many

other proofs of these results. We prove a special case of Heine's

291

transformation and write Ramanujan's

141

sum

the nonterminating

q-Chu-Vandermonde sum. We show that the q-Saalschiitz and q-Chu-

Vandermonde sums are equivalent to the evaluations of certain moments

and to the orthogonality of the little q-Jacobi polynomials; hence the

q-Chu-Vandermonde sum implies the q-Saalschutz sum. We extend the

little q-Jacobi polynomials naturally to the little q-Jacobi functions of

complex order. We show that the nonterminating q-Saalschutz and

q-Chu-Vandermonde sums are equivalent to the evaluations of certain

moments and, using the Liouville-Ismail argument, to two orthogonality

relations. We show that the nonterminating q-Chu-Vandermonde sum

implies the nonterminating q-Saalschutz sum.

Keywords: little q-Jacobi polynomials, basic hypergeometric functions, bilateral ba-

sic hypergeometric functions, g-binomial theorem, Rogers-Fine symmet-

ric function, Ramanujan's sum, Heine's q-Gauss sum and

291

trans-

formation, q-Chu-Vandermonde sum, q-Saalschutz sum, nonterminat-

ing sum, and Macdonald, Bidenharn-Louck, Heckman-Opdam, Koorn-

winder and Sahi-Knop polynomials

Introduction and summary

The celebrated Askey tableau of orthogonal polynomials (see (Koekoek

and Swarttouw, 1994)) is founded on the Jacobi and little q-Jacobi poly-

nomials (see (Hahn, 1949)). It is naturally related to the standard results

for ordinary and basic hypergeometric functions surveyed by Gasper and

Rahman (Gasper and Rahman, 1990). See also (Andrews, 1965; An-

drew~, 1969; Andrews, 1972; Andrews, 1998), (Andrews and Askey,

1977; Andrews and Askey, 1978; Andrews and Askey, 1981), (Askey,

2005

Springer Science+Business Media, Inc.

302

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

1978), (Askey and Ismail, 1979)) (Askey and Wilson, 1985)) (Fine, 1988),

(Gasper, 1997), (Heine, 1847; Heine, 1878)) (Ismail, 1977)) (Ismail and

Rahman, 1995), (Joichi and Stanton, 1989), (Kadell, 1987a; Kadell,

1987b), (Rogers, 1983; Rogers, 1916)) (Rahman and Suslov, 1994b; Rah-

man and Suslov, 1994a; Rahman and Suslov, 1996; Rahman and Suslov,

1998a; Rahman and Suslov, 1998b), (Suslov, 1998) by Andrews, Askey,

Fine, Gasper, Heine, Ismail, Joichi, Kadell, Rahman, Rogers, Stanton,

Suslov and Wilson.

Askey (Askey, 1980) conjectured a number of extensions of Selberg's

integral (Selberg, 1944) which use the beta-type distributions of the

Askey tableau. Macdonald (Macdonald, 1982) and Morris (Morris,

11,

1982) conjectured certain constant term identities associated with root

systems. Anderson (Anderson, 1991) and Aomoto (Aomoto, 1987) devel-

oped alternative proofs of Selberg's integral. See (Evans, 1994), (Garvan,

1989a; Garvan, 198913)) (Garvan and Gonnet, 1991), (Gustafson, 1990),

(Habsieger, 1988)) (Habsieger, 1986)) (Kadell, 1988a; Kadell, 1994a;

Kadell, 199413; Kadell, 1998)) (Mehta, 1967), (Stembridge, 1988)) (Zeil-

berger, 1988; Zeilberger, 8990) by Evans, Garvan, Gonnet, Gustafson,

Habsieger, Kadell, Mehta, Stembridge and Zeilberger for proofs of these

conjectures and closely related results.

Heckman and Opdam (Heckman, 1987; Heckman and Opdam, 1987;

Opdam, 1988a; Opdam, 198813) introduced Jacobi polynomials and ba-

sic hypergeometric functions associated with root systems. Opdam (Op-

dam, 1989) gave a norm evaluation which eclipsed the Macdonald-Morris

constant term q-conjecture. Baker and Forrester (Baker and Forrester,

1999)) Kadell (Kadell, 198813; Kadell, 1993; Kadell, 1997; Kadell, 2000a;

Kadell, 2000b)) Kaneko (Kaneko, 1993; Kaneko, 1996; Kaneko, 1998))

Knop and Sahi (Knop and Sahi, 1997), Macdonald (Macdonald, 1995,

Chap. VI), Opdam (Opdam, 1995)) Richards (Richards, 1989)) Stanley

(Stanley, 1989)) and Vinet and Lapointe (Vinet and Lapointe, 1995) de-

veloped further aspects of the theory associated with the root system

An-1.

Sahi and Knop (Sahi, 1994; Sahi and Knop, 1996) introduced

polynomials related to the hypergeometric distribution which general-

ized the Biedenharn-Louck (Biedenharn and Louck, 1989) polynomials.

Koornwinder (Koornwinder, 1995) introduced polynomials associated

with the root system

BC,. See also Kadell (Kadell, 2003) and Macdon-

ald (Macdonald, 1998).

In (Kadell, 2000b), we used the classical ratio of alternants to extend

the ~chur functions to partitions with complex parts. Following Proctor

(Proctor, 1989)) we gave a combinatorial representation

an alternating

sum over the symmetric group, and established the Pieri formula and