Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.


A
bilateral series involving basic hypergeometric functions
363
Then the following identity holds:
4,
qybl
bk
)
+
idem (al; a2,.
. .
,
ak+l)
.
xal
. . .
ak+l
Here, idem (al; a2,.
.
.
,
ak+1) denotes the sum of the
k
terms obtained
by interchanging a1 in the second term with each of a2,.
. .
,
ak+l (thus,
we have in total
k
+
2 terms on the right-hand side of (2.3)). As is
customary, we implicitly assume that the parameters are such that one
never divides by zero. Note also that interchanging the roles of
k+lcpk
and
1+1yq gives an alternative expression for the series, which is valid when
(2.2) is replaced by the condition lqxdl
-
. .
dl
1
<
1
ycl
.
.
cl+11. These two
expressions are related by (Gasper and Rahman, 1990, (4.10.10)).
In (Koelink and Rosengren, 2002), we computed the sum when
k
=
1
=
1
and, crucially to the methods used there, (1.2) holds. The latter
condition means exactly that the three
493
series on the right-hand side
of (2.3) are balanced. In this case Theorem 2.1 reduces to (Koelink and
Rosengren, 2002, (3.13)), rather than the alternative expression as a sum
of two
8W7
series given in (Koelink and Rosengren, 2002, Proposition
3.1).
Our main tool is the following lemma from (Koelink and Rosengren,
2002), where it was used only in the case
k
=
5.
Here we need the
general case.
Lemma
2.2.
For

364
THEORY
AND APPLICATIONS OF SPECIAL FUNCTIONS
and x
E
(C
\
R>o
one has
-
-
-
(q, al,
,
ak+l,
2-4
!I14 bl/t,.
-.
,
bk/t; qIoo . (2.5)
(x,
q/x,
bl,
..
.
,
bk,
t, a1/t,..
.
,
ak+l/t;
In (Koelink and Rosengren, 2002), this was proved using the explicit
formula for analytic continuation of
k+lcpr,
series. It is possible to give
a much simpler proof using integral representations. Namely, the coeffi-
cient of
tn
in the Laurent expansion of the right-hand side in the annulus
(2.4) is given by
where the integral is over a positively oriented contour encircling thk
origin inside the annulus. This is an integral of the form (Gasper and
Rahman, 1990, (4.9.4)), which is computed there using residue calculus.
If
lxqnl
<
1,
its value is given by (Gasper and Rahman, 1990, (4.10.9))
as the
k+lcpk
series in (2.5). Since (2.6) is analytic in x, this also holds
for lxqnl
2
1
in the sense of analytic continuation.
Remark
2.3.
More generally, (Gasper and Rahman, 1990, (4.10.9))
may be used to express the Laurent coeficients of
in the annulus
max
l&l
<
14
<
min (I/ Iril) (2.8)
as sums of analytically continued basic hypergeometric series.
Proof of Theorem 2.1. Similarly as in (Koelink and Rosengren, 2002),
it is easy to check that (2.1) is the natural condition for absolute con-
vergence of the left-hand side of (2.3). We first rewrite this series as an
integral. For this we make the preliminary assumption that t is real with
Writing fk(t; a,
b,
x) for either side of (2.5), we consider the integral

A
bilateral series involving basic hypergeometric functions
365
Using the expression on the left-hand side of (2.5) together with orthog-
onality of the monomials, we find that it equals the sum in (2.1).
To compute the integral, we plug in the expressions from the right-
hand side of (2.5). Up to a constant, this gives an integral of the form
where
P
is as in (2.7), with
Note that (2.8) and
(2.9)
are equivalent. Thus, we again have an integral
of the form (Gasper and Rahman, 1990, (4.9.4)). The condition (2.2) is
precisely (Gasper and Rahman, 1990, (4.10.2)), which ensures that the
singularity at
z
=
0 does not contribute to the integral. The value of
the integral is then given by (Gasper and Rahman, 1990, (4.10.8)) as a
sum of
k
+
2 terms, each being a
k+1+49k+1+3
series. However, because
of the condition
a&
=
a2P2
=
q,
they all reduce to type
k+l+29k+l+l.
This proves Theorem 2.1 under the assumption (2.9). By analytic con-
tinuation in
t,
this may be replaced with the weaker condition (2.1).
Remark
2.4.
Using instead of Lemma
2.2
the Laurent expansion of
the general product
(2.7)
gives a generalization of Theorem
2.1,
where
the two basic hypergeometric series on the left-hand side of
(2.3)
are
replaced by finite sums of such series.
In the case
I
=
0, one may use the q-binomial theorem to sum the
series
190
in Theorem 2.1. The condition
y
E
(C
\
is then superflu-
ous. We find this special case interesting enough to write out explicitly.
Compared to Theorem 2.1 we have made the change of variables
y
I+
c,
cl
I+
dlc.
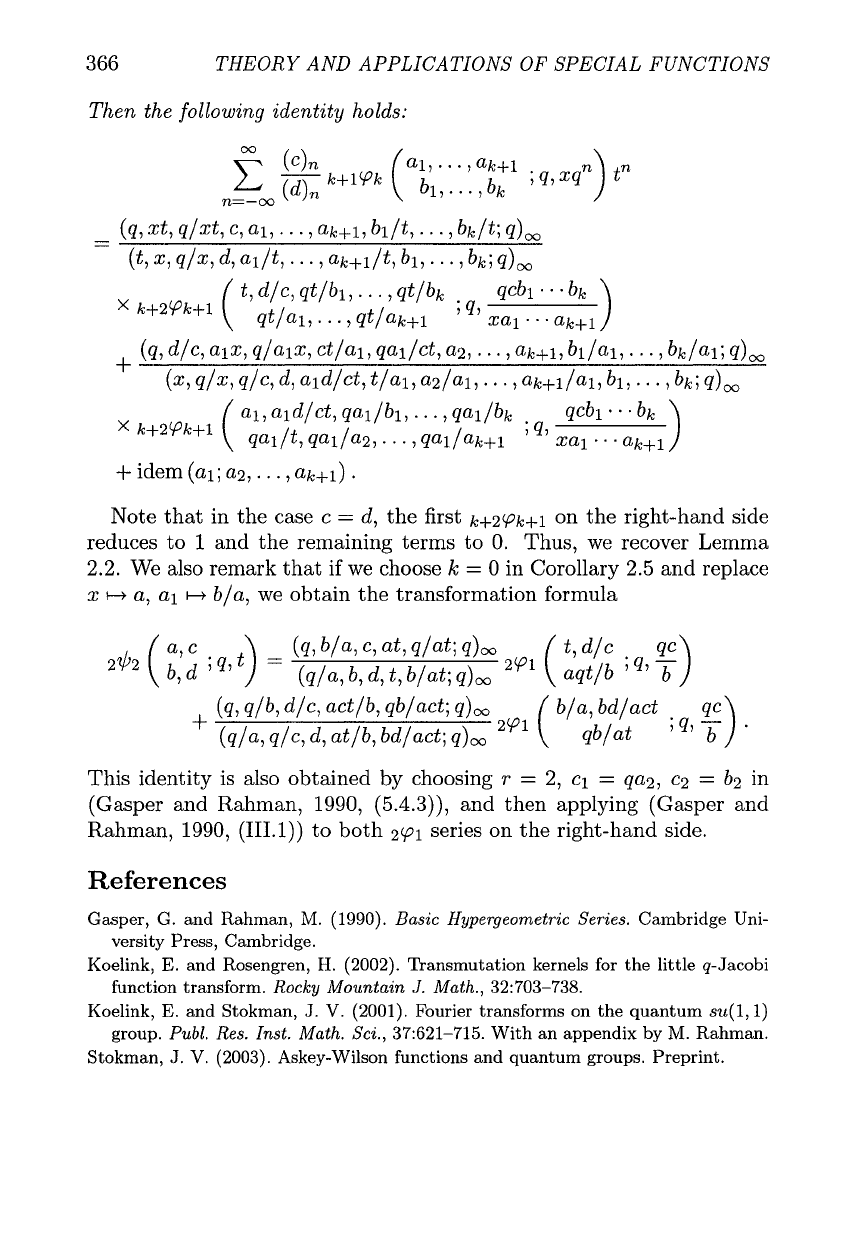
Corollary
2.5.
Let x
E
C
\
E%>o,
-
and assume that
366
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Then the following identity holds:
+
idem
(al; a2,
. .
.
,
ak+l)
.
Note that in the case
c
=
d,
the first
k+29k+l
on the right-hand side
reduces to
1
and the remaining terms to
0.
Thus, we recover Lemma
2.2.
We also remark that if we choose
lc
=
0
in Corollary
2.5
and replace
x
H
a, a1
H
bla,
we obtain the transformation formula
(q, bla, c, at, qlat;
4,
t,
dlc
22
(
q7
)
=
(qla, b, d,
t,
blat; q),
291
(
aqt/b
;
4,
T)
This identity is also obtained by choosing
r
=
2,
cl
=
qua, c2
=
b2
in
(Gasper and Rahman,
1990, (5.4.3)),
and then applying (Gasper and
Rahman,
1990, (111.1))
to both
291
series on the right-hand side.
References
Gasper, G. and Rahman,
M.
(1990). Basic Hypergeometric Series. Cambridge Uni-
versity Press, Cambridge.
Koelink,
E.
and Rosengren,
H.
(2002). Transmutation kernels for the little q-Jacobi
function transform. Rocky Mountain
J.
Math., 32:703-738.
Koelink,
E.
and Stokman, J.
V.
(2001). Fourier transforms on the quantum su(1,l)
group.
Publ.
Res. Inst. Math. Sci., 37:621-715. With an appendix by
M.
Rahman.
Stokman, J.
V.
(2003). Askey-Wilson functions and quantum groups. Preprint.

THE HILBERT SPACE ASYMPTOTICS OF
A CLASS OF ORTHONORMAL
POLYNOMIALS ON A BOUNDED
INTERVAL
S.
N.
M.
Ruijsenaars
Centre for Mathematics and Computer Science
P.O.
Box
94079
1090
GB Amsterdam
THE NETHERLANDS
Abstract
We study the asymptotics of orthonormal polynomials {pn(cosx))~=o,
associated with a certain class of weight functions w(x)
=
l/c(x)c(-x)
on
[O,.rr].
Our principal result is that the norm of the difference of
p, (cos x) and
D,
(x)
=
c(~)e~~"+c(-x)e-~~" in
L~
([o,
TI,
(2~)-'w(x)dx)
vanishes exponentially as
n
+
co.
The decay rate is determined by an-
alyticity properties of the c-function.
1.
Introduction
Suppose w(x) is a continuous function on
[O,
T]
that is positive on
(0,
T)
.
Then the functions
form a total set in the Hilbert space
By the Gram-Schmidt procedure, we can therefore construct an or-
thonormal base of the form
Pn
(x)
=
PnBn
(x)
+
C
bnmBm (x)
Pn
>
0,
brim
E
B,
n
E
N.
(1.3)
m<n
We may view Bn(x) as a polynomial of degree
n
in cos x (the Tchebichev
polynomial of the first kind). Doing so, it is clear that there are polyno-
mials pn(v) such that
pn (cos x)
=
Pn
(5).
(1.4)
O
2005
Springer Science+Business Media, Inc.

368
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
As is well known (and easily verified), these polynomials satisfy a self-
adjoint recurrence relation
with
a-1-0, anE(0,2), b,E(-2,2), n€N.
(le6)
In this paper we study the n
-+
oo
asymptotics of P,(x),
p,,
a,
and
b,.
Our primary interest is in the n
-+
oo
asymptotics of the functions
P,(x) in the Hilbert space
3-t.
We allow weight functions of the form
where c(x) has certain analyticity properties specified in Section 2. Defin-
ing the dominant asymptotics function
our principal result is an exponential decay bound
for weight functions w(x) in the class
We
(2.18). Here,
11
.
11
denotes the
norm derived from the scalar product of
3-t
(1.2), and the decay rate
d
is
any positive number smaller than a number d+ in (0,
oo]
that depends
on the choice of c-function. (It is given by (2.2).)
We obtain no information on the pointwise decay of IP,(x)
-
Dn(x)l
for x
E
(0,
r),
but we believe this is also governed by an O(exp(-2nd))-
bound. Our interest in obtaining a sharp L2-bound was triggered by
the possibility to use such a bound for an approximation argument. The
application we have in mind concerns what may be viewed as 'relativistic'
Lam6 functions. To prove L2-completeness of a suitable countable set
of the latter, we need a sufficiently strong bound
IIP,
-
D,ll
5
P,
on
orthonormal polynomials associated to certain weight functions in
We.
Specifically, the sequence
{P,)
must be in 12. It is however beyond our
scope to elaborate on this; we return to this issue elsewhere (Ruijsenaars,
2003).
As it has turned out, the methods we have developed to prove the
desired L2-bounds can be generalized to the multi-variable case. The
pertinent results have a striking application to the theory of quantum
soliton systems, and have already been reported in our paper Ref. (Rui-
jsenaars, 2002). (For further generalizations, see work by van Diejen (van
Diejen, 2003).)
In the one-variable case at issue here, the proofs are quite elementary
and transparent. Furthermore, we can allow a larger class of weight

Hilbert space asymptotics for Orthogonal Polynomials
369
functions than the one obtained by specializing Ref. (Ruijsenaars, 2002).
It therefore seems useful to have an independent treatment of the one-
variable case available.
At the outset, we should mention that the subclass
WoYo
of
W
(cf. (2.15))
belongs to the huge class of weight functions for which Szeg6 has ob-
tained uniform pointwise bounds on
I
Pn
(x)
-
Dn
(x)
I,
cf. Theorem 12.1.4
in his monograph (Szeg6, 1975). The latter Lw-bounds are however
0
((ln(n))-') for some
X
>
0, a decay that is far too slow to be in 12.
(To be sure, SzegB's bounds are presumably quite sharp for the class he
handles.)
Another point of special note is that our class
We
of weight functions
contains the weight function of the Askey-Wilson polynomials (Askey
and Wilson, 1985; Gasper and Rahman, 1990). More precisely, setting
CAW
(x)
-
(ae-"
";)
_
(be-"; 4)
_
(ce-"
;
4)
_
(de-'x; 4)
_
/
(e-2"; 4)
_
,
(1.10)
the weight function
WAW(X)
=
~/CAW(X)CAW(-x)
(1.11)
belongs to
W1,l
(2.15) for
We would like to emphasize that for this special case the exponen-
tial decay of
llPn
-
Drill
is an obvious corollary of previous results by
Ismail and Wilson (Ismail and Wilson, 1982; Ismail, 1986). Indeed, via
generating function techniques they obtained an Lw([O,
T])
exponential
decay bound on
Pn
-
Dn.
It is an open question whether the generating
functions of the much larger class of polynomials arising from
We
can
again be exploited to obtain exponential decay in Lw. (Note this would
yield a stronger result than our L2-decay, since we restrict attention to
bounded weight functions.)
2.
Main
results
Our starting point consists of the space
A
of functions
f
(z) that are
analytic and zero-free in the closed unit disk, real-valued for z
E
[-I,
11,
and normalized by
f
(0)
=
1.
(2.1)
Thus the convergence radii R+ and R- of the power series expansions
of
f
(z) and
l/
f
(z) are greater than
1,
and the decay parameters
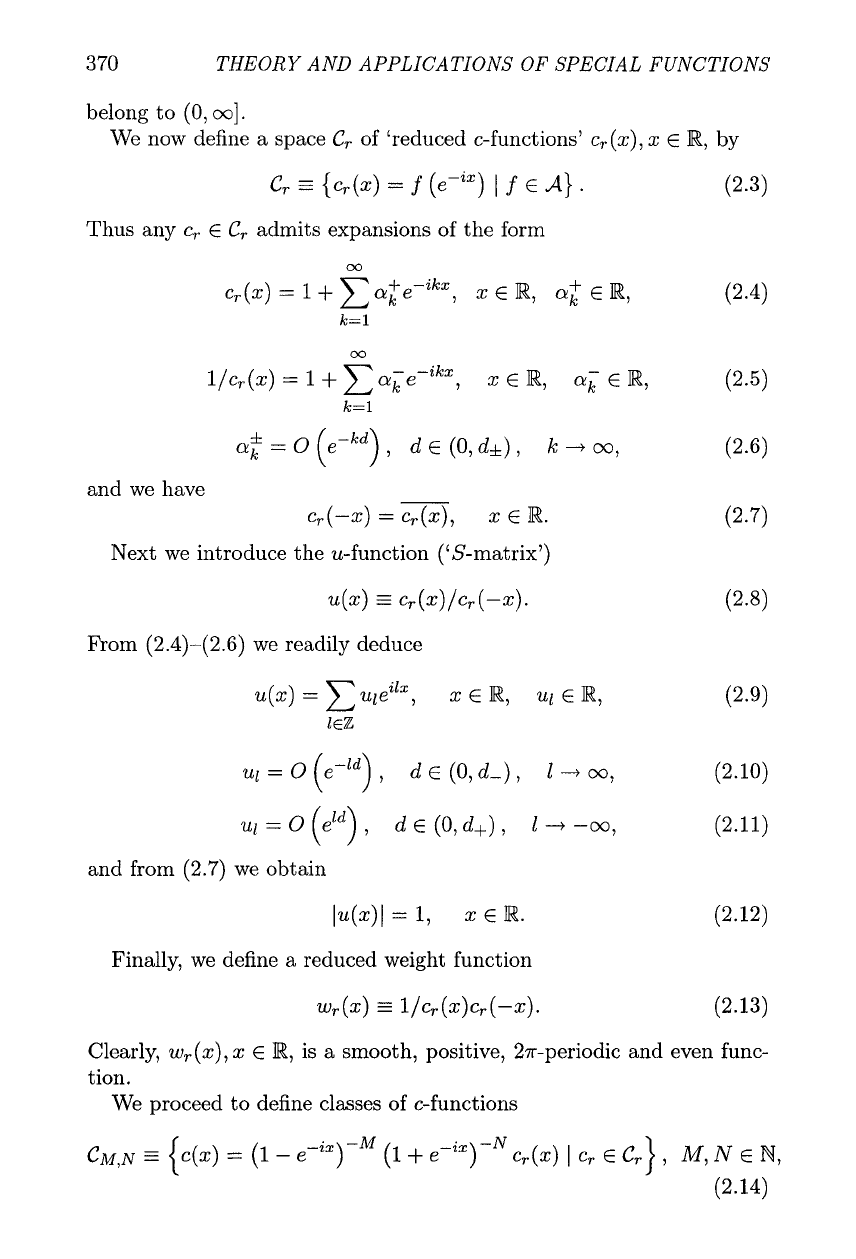
370
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
belong to (0, m].
We now define a space
Cr
of 'reduced c-functions' c, (x),
x
E
W,
by
Thus any c,
E
Cr admits expansions of the form
00
~(x)=l+xa:e-"%,
XEW,
k=l
00
I/+ (x)
=
1
+
a;e-"x, x
E
ER,
a;
E
P,
k=l
a:
=
o
(e-kd)
,
d
E
(0, d+),
ii
+
m,
and we have
G(-x)=c~(x))
XEW.
Next we introduce the u-function ('5'-matrix')
From (2.4)-(2.6) we readily deduce
and from (2.7) we obtain
Finally, we define a reduced weight function
Clearly, w,(x), x
E
W,
is a smooth, positive, 2n-periodic and even func-
tion.
We proceed to define classes of c-functions
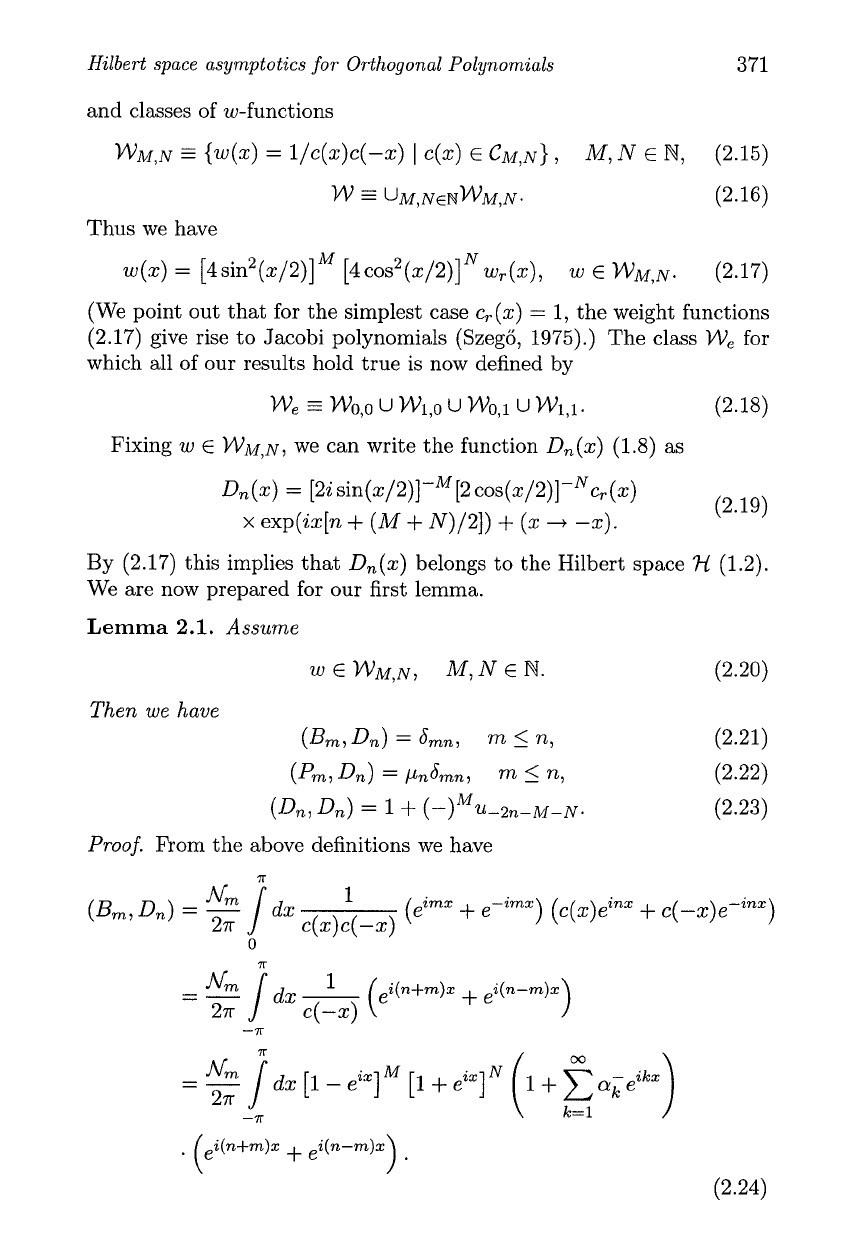
Hilbert space asymptotics for Orthogonal Polynomials
371
and classes of w-functions
W
--
UM,NEN~M,N.
Thus we have
W(X)
=
[4
sin2 (x/2)]
[4
cos2 (x/2)] w, (x), w
E
WM,N. (2.17)
(We point out that for the simplest case c,(x)
=
1,
the weight functions
(2.17) give rise to Jacobi polynomials (Szeg6, 1975).) The class We for
which all of our results hold true is now defined by
Fixing w
E
WM,~, we can write the function Dn(x) (1.8) as
By (2.17) this implies that Dn(x) belongs to the Hilbert space
'H
(1.2).
We are now prepared for our first lemma.
Lemma
2.1.
Assume
Then we have
(Bm,Dn)=dmn, mIn, (2.21)
(Pm, Dn)
=
~namn,
m
I
n, (2.22)
(Dn, Dn)
=
1
+
(-)"~-2n-M-N.
(2.23)
Proof.
From the above definitions we have

372
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Since the sequence
{ai)
has exponential decay, we may interchange
the summation and the integration. For
m
<
n each of the resulting
integrals has an integrand proportional to exp(i1x) with 1
E
N*,
so they
all vanish. For
m
=
n we pick up the constant term and obtain
1.
Hence
(2.21) follows.
Clearly, (2.22) follows from (2.21) and the definition (1.3) of
Pn,
so it
remains to prove (2.23). To this end we calculate
where we used (2.8)-(2.11).
Notice that this lemma solely concerns equalities,
as
opposed to in-
equalities. As an obvious corollary, we obtain a further equality
Using (2.1 I), the following inequality is also immediate from (2.23)
:
IlDnll
=
1
+
O(exp(-2nd)), d
E
(0, d+)
,
n
+
oo.
(2.27)
From (2.22) and the Schwarz inequality we now get
Next, we combine (2.26) and the upper bound (2.28) to deduce that
we have an equivalence
llPn
-
Drill
=
O(exp(-nd))
*
pn
2
1-C1exp(-2nd), C'
>
0, Vn
E
N.
(2.29)
However, we will show in Section 3 that
llPn
-
Drill
does not decay ex-
ponentially for
w
€
W
\
We.
We now pass to an auxiliary result that is
still valid for all
w
E
W.
To this end we introduce a truncated +function
L
ciL)
(x)
=
1
+
x
ate-i1x,
L
E
N*,
(2.30)
k1
